Figure - PMC

Skip to main content

An official website of the United States government

Here's how you know

Here's how you know

Official websites use .gov
A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS
A lock ( ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

View full-text article in PMC

. 2020 Jul 3;10:10973. doi: 10.1038/s41598-020-67413-8

Search in PMC
Search in PubMed
View in NLM Catalog
Add to search

© The Author(s) 2020

Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.

PMC Copyright notice

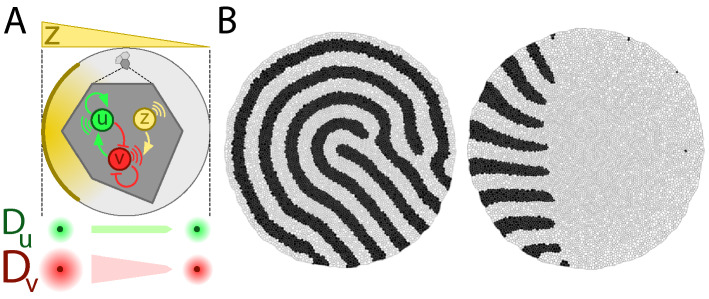
Fig. 4 — Stripe alignment mechanism in growing tissues. (A) In tissues where cells actively grow/divide, if each cell is driven by a regulatory network that just involves an activator, u, and an inhibitor, v, then the resulting Turing pattern displays rotational symmetry (panel B left). If an additional species, z, is released from the “tip” (left side of the tissue in this example) and set a polarity gradient such that the diffusivity of v is spatially modulated, then stripes align following the directionality of the gradient (panel B right). (B) Final snapshot of simulations without (left) and with (right) diffusivity modulation (constant cellular adhesion). The black (white) cellular domains account for regions where $u > v$ ( $v > u$ ). Since diffusive transport relies on tissue topology (“Methods”) we avoided a possible bias in patterning by using in both simulations the same random sequences that determine the variability of cellular growth/division in order to reproduce the same cellular growth/division events and cell/tissue topologies.