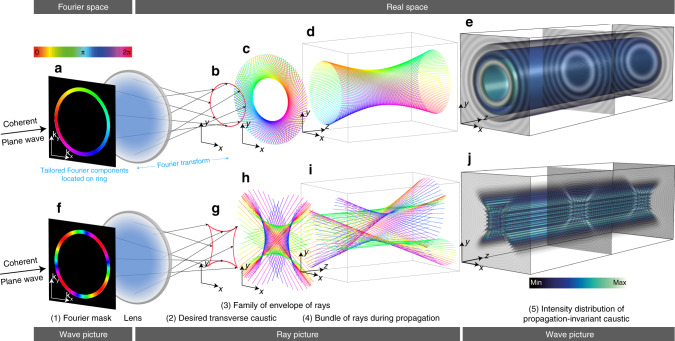
Fig. 1. Caustics in propagation-invariant beams.

a–e A Bessel beam J₁ with OAM of charge ℓ = 1 has a circular caustic. a Fourier phase pattern, with azimuthally linearly increasing phase Φ = ℓϕ, confined to an infinitesimal thin ring. Fourier transform by a lens. Fourier wavefront shaping determines the direction of the propagating rays, and the caustic (b) forms in real space as the envelope of the rays in a transverse plane (c). A subset of the rays, each coming from a specific point on the ring, is shown in (d). The complete family of rays is composed of a continuum of bundles like the one shown in d but displaced in z. The corresponding field has a propagation-invariant transverse intensity (e) with a pronounced central ring according to the circular caustic. f–j A more complex phase function $\mathrm{\Phi }=\ell \varphi -q/2\sin \left(2\varphi \right)$ yields an astroid caustic, which includes four cusp caustics.