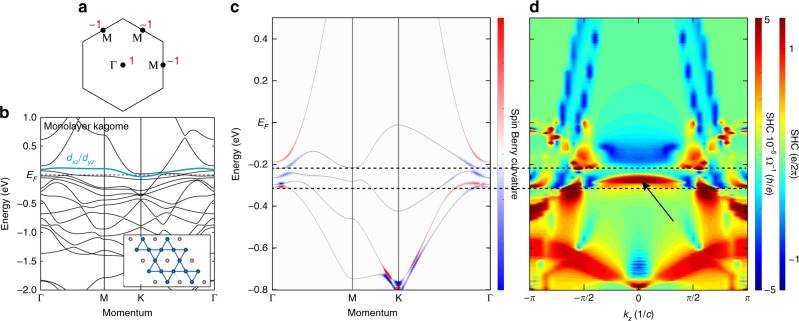
Fig. 5. Nontrivial topology of the kagome flat bands in CoSn.
a Parity eigenvalues of the flat bands of the bulk CoSn at the time-reversal invariant momenta of kz = 0 plane. b Electronic band structure for a single kagome layer limit of CoSn (inset). The dxz/dyz flat band locates exactly at Fermi level and retains nonzero Z2 index in the monolayer limit (parity eigenvalues are same with a). ck-resolved spin Hall conductivity in the kz = 0 plane. The dominant contribution to SHC is concentrated near the spin–orbit coupling gap between the flat band and Dirac bands at Γ as well as at the massive Dirac points at Κ. dkz dependence of SHC of CoSn. The in-plane momentum (kx, ky) contribution to the SHC is integrated in this map, up to a given energy. As marked by the black arrow, the SHC is sharply enhanced inside the nontrivial SOC gap between the flat and Dirac bands (horizontal dashed lines). Due to the two-dimensionality of the flat band, its contribution to SHC is fairly kz independent across ~40% of the Brillouin zone.