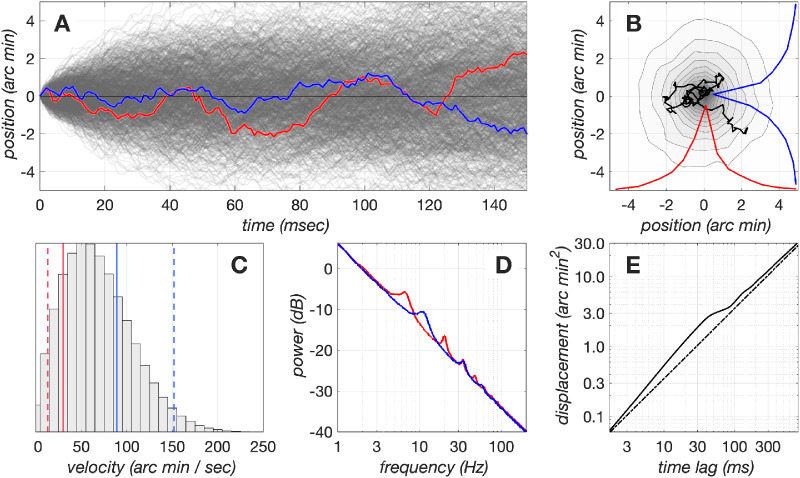
Figure 7.

Dynamics of drift fixational eye movements generated by our model. (A) x and y components of a set of 1,024 eye movement trajectories during a period of 150 ms. For visualization purposes, the paths always start at (0,0), but in the simulations, each path starts at a random location, and the centroid of each path is constrained to be at (0,0). Red and blue lines denote the x and y, respectively, trajectories of a single eye movement trajectory. (B) The contour plot depicts the fixation span of the set of 1,024 eye movement paths depicted in A, and the red and blue lines depict the marginal distributions of x and y eye positions of this data set. The superimposed black line depicts the eye movement path whose x and y trajectory components are depicted in A as the red and blue traces. (C) Distribution of instantaneous velocities in the examined set of 1,024 eye movement trajectories. The red and blue solid lines depict the mean drift velocities from 2 human subjects who had the lowest and highest velocity, respectively, in a pool of 12 subjects (Cherici, Kuang, Poletti, & Rucci, 2012). The red and blue dashed lines represent −1σ and +1σ of the drift velocity distributions for these subjects, respectively. (D) Power spectra of the x and y drift trajectory components. The spectral peaks are due to the oscillatory behavior of the modeled delayed negative feedback mechanism, which has slightly different delays for the x and y drift components. (E) Displacement analysis of model drift eye movements. The solid line depicts the mean squared displacement, $\overline{\Delta p^2}$, as a function of time lag for the examined set of eye movement paths. The dashed line depicts $\overline{\Delta p^2}$ for a purely Brownian process. Notice the persistent behavior between 2 and 30 ms, which causes the eye to diffuse more than it would have if it were under the control of a purely Brownian process, and the antipersistent behavior that stabilizes eye position at longer time delays (100 to 500 ms).