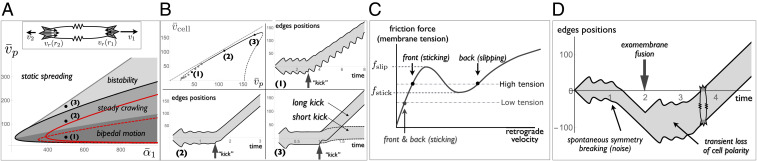
Fig. 3.

Polarization and crawling of a 1D elastic cell. (A) (Top) Sketch of a 1D cell with protrusions at both ends coupled through the elastic cell tension (springs with a dimensionless stiffness ${\overline{k}}_{\sigma }$). (Bottom) Crawling phase diagram with the adhesion parameter ${\overline{\alpha }}_1$ and the polymerization velocity ${\overline{v}}_p$. The symmetric spreading state is oscillatory within the thick solid lines (Eq. 6, black for ${\overline{k}}_{\sigma }\to 0$ and red for ${\overline{k}}_{\sigma }=10$). A crawling state exists within the regions shaded gray. Three regions can be distinguished: a steady crawling state, where the cell moves without changing its shape, coexists with the static spreading state in the bistability region, and coexists with the oscillatory spreading state in the steady crawling region. In the bipedal motion region, no true steady state exists and a bipedal crawling state—where the cell’s leading and trailing edges follow different limit cycles—coexists with the oscillatory spreading state. The dashed red line is the boundary between steady crawling and bipedal motion for ${\overline{k}}_{\sigma }=10$. (B) (Top) Variation of the cell velocity with the polymerization velocity. The solid part of the curve corresponds to steady crawling and the dashed parts are unstable steady states. Gray dots correspond to bipedal motion. The other panels are examples of “kymographs” showing the position of the two cells ends as a function of time in the three different regimes. The cell is first allowed to spread isotropically and is given an asymmetric “kick” which transiently removes all bound linkers on one side of the cell at a prescribed time (arrows). In the bistable region, the binder may rebind immediately after the kick (short kick) or after a short delay $\mathrm{\Delta }t=0.05/k_{\text{off}}^0$ (long kick). (C) Role of membrane tension on cell polarity. The coexistence of a sticking and a slipping regime, necessary for cell polarization, requires high enough tension. The slipping state is inaccessible if membrane tension is decreased. (D) Cell edge position as a function of time. Isotropic spreading starts at $t=0$ and is followed by a spontaneous breaking of symmetry due to intrinsic noise on $r_{\text{on}}$. Membrane tension is abruptly halved at $t=2/k_{\text{off}}^0$, e.g., following the fusion of exocellular vesicles, resulting in a transient loss of cell polarity. High tension is restored after further spreading, leading to a new symmetry-breaking event. Parameters: $r_{\text{on}}=10$, (B) ${\overline{\alpha }}_1=500$ and ${\overline{k}}_{\sigma }=10$, (D) ${\overline{v}}_p=100$, and $r_{\text{on}}$ is a Gaussian random variable varying within $1\%$ around $r_{\text{on}}=10$.