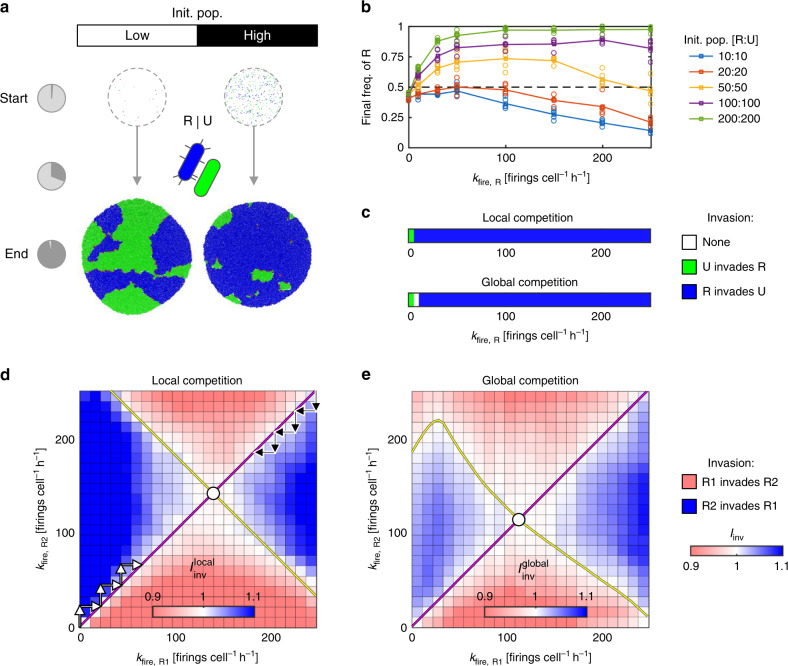
Fig. 1. The evolution of random T6SS firing.

a Simulation snapshots showing initial and final cell configurations for surficial competition between T6SS− Unarmed strain (U, green) and a Random-firing T6SS+ strain (R, blue). Simulations are carried out for both low and high initial cell densities (left and right columns; initial cell populations 10 vs. 10 and 200 vs. 200 cells, respectively); pie charts (left) indicate the consumption of patch resources. Firing rate k_fire,R = 50 firings cell⁻¹ h⁻¹. b Competition outcomes, measured by final R cell proportion, as a function of firing rate k_fire,R for increasing initial cell densities (see legend, right). Circles and lines correspond to data points and their means, respectively. c Invasion plots showing outcomes of local and global invasion analyses for R vs. U competition (see “Methods”), as a function of firing rate k_fire,R, for high initial cell density (200 vs. 200 cells); additional cases are shown in Supplementary Fig. 3. d, e Pairwise invasion plots for competing R-type strategists (R1, R2), showing invasion outcomes for local (d) and global (e) competition scales for intermediate cell density (50 vs. 50 cells). Arrows illustrate progression of evolving firing rates k_fire,R, converging on evolutionary stable strategy firing rates (ESS, white circles). Simulation parameters used throughout: N_hits = 2, c = 0.001; n = 5 simulation replicates per case in b, c and n = 10 per case in d, e. Source data are provided as a Source Data file.