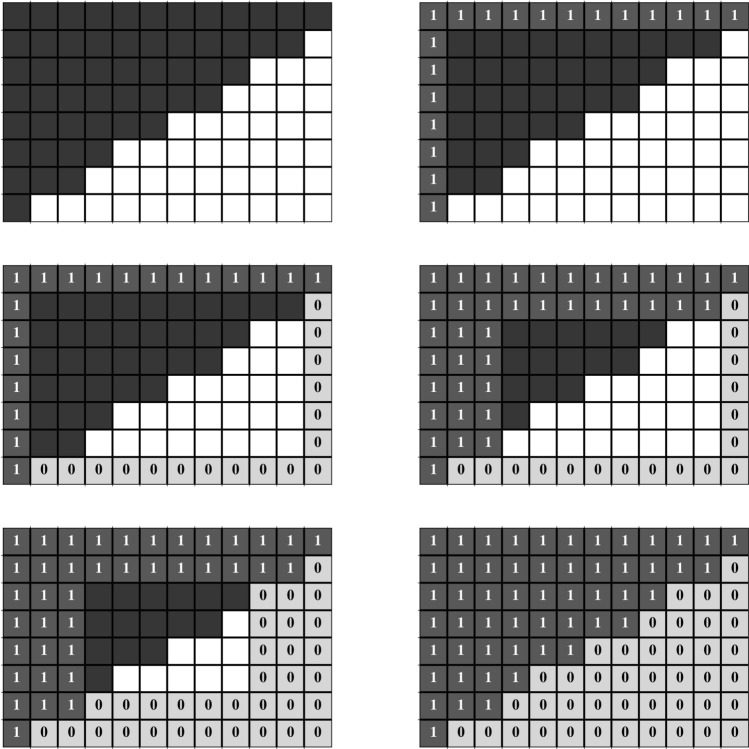
Figure 2.

An example of a perfectly nested network with its probabilities per link from the BiCM: at the first step, the first row and column are full, and the degree is respectively 12 and 8. So the link probabilities must be exactly one, for preserving the row sum and the column sum. At the second step, since the last row and column have degree 1, the remaining entries must sum to 0, yielding all zeros. Again, at the third and fourth steps the rows and columns that are completely full or empty univocally determine the respective probabilities to be 1 or 0. At the end of this process, the link probabilities are all set to 0 or 1, so the corresponding canonical ensemble contains only one matrix.