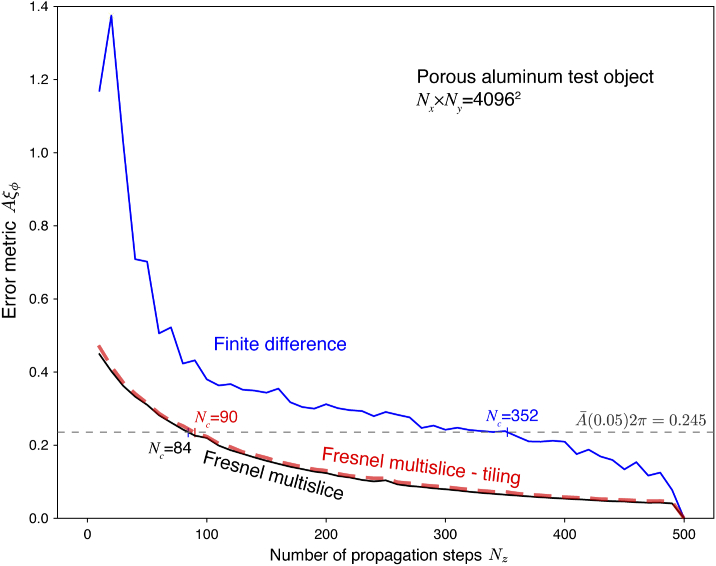
Fig. 7.

Convergence test for the three algorithms of Sec. 2 using the porous aluminum test object. For this test, the ${4096}^2$ transverse subarray of the object was selected, and the thickness $t=147.5$ $\mathrm{\mu }$m was bilinearly re-sampled onto a variable number of slices $N_z$. Using the convergence criterion of Eq. (26) giving a tolerance for this sample of ${[\overline{A}{\xi }_{\varphi }]}_{\text{Al}}=0.245$ (Eq. (30)), the full-array Fresnel multislice approach reached convergence with $n_C=84$ slices with ${\mathrm{\Delta }}_z=1.64$ $\mathrm{\mu }$m while the tiling-based short-distance Fresnel multislice approach required $n_C=90$ slices with ${\mathrm{\Delta }}_z=1.64$ $\mathrm{\mu }$m. The finite difference method required $n_C=352$ slices with ${\mathrm{\Delta }}_z=0.42$ $\mathrm{\mu }$m for this irregular object.