Skip to main content
. 2020 Nov 6;9:e54835. doi: 10.7554/eLife.54835

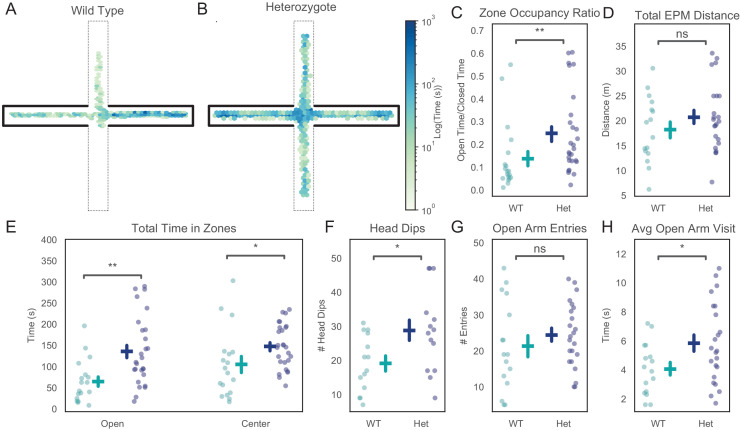
Figure 1. Pogz+/- mice exhibit reduced avoidance in the elevated plus maze (EPM).

(A, B) Occupancy plot for a 15 min EPM session for a representative wildtype (A) and Pogz+/- (B) mouse. (C) Ratio of time spent in open vs. closed arms of the EPM. Wilcoxon rank-sum test, U = −2.8857, p=0.003, WT N = 18, Het N = 27. (D) Total distance traveled during EPM sessions. Wilcoxon rank-sum test U = −1.9434, p=0.35, WT N = 16, Het N = 23. (E) Total time spent in exposed areas of EPM, open arms: statistic = −3.0753, p=0.001, center: U = −2.2112, p=0.02. Wilcoxon rank-sum test, WT N = 18, Het N = 27. (F) Total number of head dips for each mouse, U = −1.9434, p=0.03. Wilcoxon rank-sum test, WT N = 14, Het N = 14. (G) Number of open arm entries, U = −0.9993, p=0.32. Wilcoxon rank-sum test, WT N = 16, Het N = 23. (H) Average duration of each open arm visit, U = −1.984, p=0.047. Wilcoxon rank-sum test, WT N = 16, Het N = 23.

Figure 1—source data 1. Source data for Figure 1.

Figure 1.

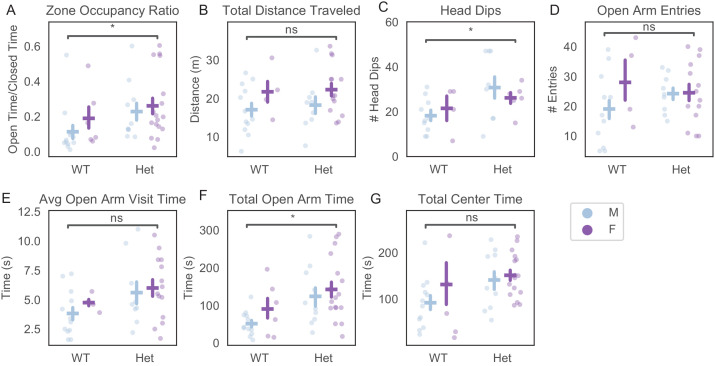
Figure 1—figure supplement 1. Sex differences do not account for elevated-plus maze (EPM) phenotypes.

Figure 1—figure supplement 1.

EPM metrics divided by sex. For normally distributed data, two-way ANOVA was used to separate effects of genotype and sex. For non-normal data, effects of sex were tested by correcting all values by the median wildtype value for that sex. (A) Ratio of time in open vs closed arms. Pearson’s normality test, statistic = 8.4985, p=0.014. Genotype rank-sum test for sex-adjusted values, U = −2.4455, p=0.014. Rank-sum test for sex difference in Het mice, U = 0.52698, p=0.60. WT N = 12 males, 4 females. Het N = 10 males, 17 females. (B) Total distance traveled in EPM. Pearson’s normality test, statistic = 14.70185, p=0.00064. Genotype rank-sum test for sex-adjusted values, U = −0.2594, p=0.79. WT N = 12 males, four females. Het N = 9 males, 14 females. (C) Head dips. Pearson’s normality test, statistic = 2.0271, p=0.44. Two-way ANOVA, effect of genotype, F = 4.776, p=0.02, effect of sex, F = 0.88, p=0.81, interaction, F = 0.365, p=0.36. Rank-sum test for sex difference in Het mice, U = −0.6123, p=0.54. WT N = 10 males, 4 females. Het N = 8 males, 6 females. (D) Number of open arm entries. Pearson’s normality test, statistic = 3.02627, p=0.22. Two-way ANOVA, effect of genotype, F = 0.264, p=0.61, effect of sex, F = 0.85, p=0.32, interaction, F = 1.31, p=0.26. WT N = 12 males, 4 females. Het N = 9 males, 14 females. (E) Average open arm visit length. Pearson’s normality test, statistic = 3.16358, p=0.22. Two-way ANOVA, effect of genotype, F = 3.42, p=0.07, effect of sex, F = 0.479, p=0.49, interaction, F = 0.092, p=0.76. WT N = 12 males, 4 females. Het N = 9 males, 14 females. (F) Total time in open arms. Pearson’s normality test, statistic = 6.4703, p=0.040. Genotype rank-sum test for sex-adjusted values, U = −2.479, p=0.013. Rank-sum test for sex difference in Het mice, U = 0.6527, p=0.61. WT N = 12 males, 4 females. Het N = 10 males, 17 females. (G) Total time in center. Pearson’s normality test, statistic = 1.2337, p=0.54. Two-way ANOVA, effect of genotype, F = 3.06, p=0.087, effect of sex, F = 1.14, p=0.29, interaction, F = 0.50, p=0.48. WT N = 12 males, 4 females. Het N = 10 males, 17 females.
Figure 1—figure supplement 2. Other behavioral assays in Pogz+/- mice.

Figure 1—figure supplement 2.

(A) Time that Pogz+/- mice or wild-type littermates spend interacting with a novel juvenile conspecific, U = 0.96, p=0.34. Wilcoxon rank sum, WT N = 7, Het N = 7. (B) Time that Pogz+/- mice or wild-type littermates spend interacting with a novel object, U = −0.063, p=0.95. Wilcoxon rank sum, WT N = 7, Het N = 7. (C) Number of marbles buried by Pogz+/- mice or wild-type littermates during 20 min, U = 0.7522, p=0.45. Wilcoxon rank sum, WT N = 8, Het N = 7. (D) Distance traveled in an open field by Pogz+/- mice or wild-type littermates, U = −1.4289, p=0.15. Wilcoxon rank sum, WT N = 14, Het N = 17. (E) Schematic of the T-maze delayed match to sample task. Mice must recall the direction of the forced run during the sample phase to successfully obtain reward from the opposite arm during the choice phase. (F) Number of trials Pogz+/- mice or wild-type littermates need to reach a learning criterion (80% accuracy) in the T-maze task, U = −0.5222, p=0.60. Wilcoxon rank sum, WT N = 5, Het N = 5. (G) Schematic of the odor-texture rule shift task. Mice must initially learn that a texture cue signals the location of a hidden food reward. Once they learn this initial rule, there is an extra-dimensional rule shift such that an odor now signals the reward location. (H) Number of trials Pogz+/- mice or wild-type littermates need to reach a learning criterion (80% accuracy) during the initial association or rule shift, IA: U = −0.1443, p=0.89; RS: U = 0.1443, p=0.89. Wilcoxon rank sum, WT N = 4, Het N = 4.
Figure 1—figure supplement 3. Distributions of sex and age for WT and Pogz Het mice used in all experiments.

Figure 1—figure supplement 3.

Age and sex breakdown for all experimental animals; age listed at date of testing. (A) Elevated-plus maze (EPM) sex. WT males: 13, Het males: 10, WT females: 6, Het females: 17. (B) EPM age. WT: 72 ± 13 days, Het: 72 ± 22 days. (C) Rule shift sex. WT males: 3, Het males: 2, WT females: 2, Het females: 2. (D) Rule shift age. WT: 68 ± 3 days, Het: 68 ± 3 days. (E) Marble burying sex. WT males: 6, Het males: 5, WT females: 2, Het females: 2. (F) Marble burying age. WT: 108 ± 69 days, Het: 115 ± 72 days. (G) Social interaction and novel object exploration sex. WT males: 4, Het males: 4, WT females: 3, Het females: 3. (H) Social interaction and novel objection exploration age. WT: 69 ± 8 days, Het: 65 ± 7 days. (I) Open field sex. WT males: 10, Het males: 8, WT females: 4, Het females: 9. (J) Open field age. WT: 69 ± 14 days, Het: 67 ± 11 days. (K) T-maze sex. WT males: 3, Het males: 3, WT females: 2, Het females: 2. (L) T-maze age. WT: 61 ± 40 days, Het: 61 ± 40 days. (M) Patch clamp recordings sex. WT males: 10, Het males: 8, WT females: 10, Het females: 6. (N) Patch clamp recordings age. WT: 110 ± 18 days, Het: 11 ± 22 days. (O–P) Local field potential recordings sex. WT males: 4, Het males: 3, WT females: 2, Het females: 4. (P) Local field potential recordings age. WT: 99 ± 36 days, Het: 121 ± 34 days.