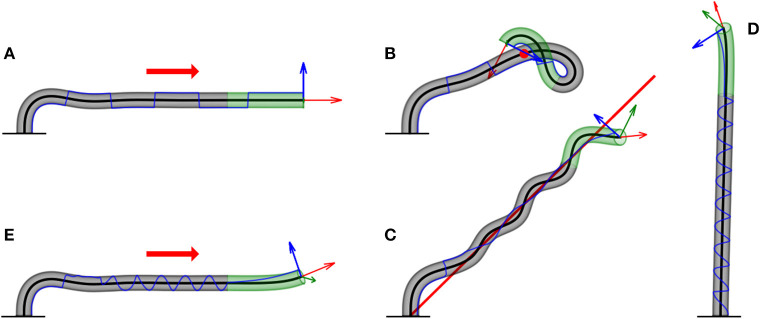
Figure 6.
Examples of numerical simulations for various scenarios. Here, we showcase snapshots of simulations for various cases. The subapical active growth zone is in green, while no growth occurs below that in gray. The arrows on the apex are the apical tangent direction (red), normal direction (blue), and bi-normal direction (green). The blue line marks the history of the direction of along the organ. The details of the simulations are given in section 3. We note that elasticity is not implemented here, and therefore the organ grows through itself. (A) Infinitely distant constant stimulus (red arrow). The organ reaches a steady state, growing in the direction of the stimulus. switches directions due to damped oscillations in the solution (Video 1). (B) Point stimulus (red dot). Illustrates the different dynamics between a distant vs. nearby stimulus (Video 2). (C) General geometry: twining around a line stimulus (red line). Any geometry for the source stimulus can be implemented. Here, we chose a line geometry, which, together with a signal in the direction of the line (to prevent self-intersections), yields dynamics similar to the twining of a climbing plant (Video 3). (D) Circumnutations. We implement the growth response to an internal cue rather than external cues, yielding inherent periodic movements of plants called circumnutations, generally associated with search processes. The periodic trajectory of visualizes the rotational movement of the growing tip (Video 4). (E) Superposition of internal and external stimuli. We combine circumnutations with an infinitely distant external stimulus (Video 5).