Figure 3.

Cortical turbo codes.

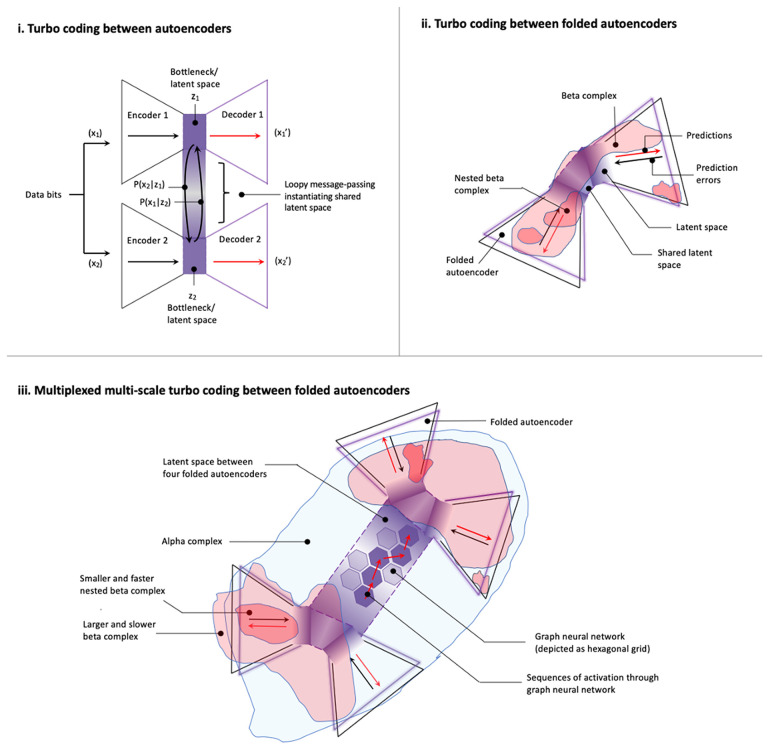
(i) Turbo coding between autoencoders.

Turbo coding allows signals to be transmitted over noisy channels with high fidelity, approaching the theoretical optimum of the Shannon limit. Data bits are distributed across two encoders, which compress signals as they are passed through a dimensionality reducing bottleneck—constituting a noisy channel—and are then passed through decoders to be reconstructed. To represent the original data source from compressed signals, bottlenecks communicate information about their respective (noisy) bits via loopy message passing. Bottleneck z₁ calculates a posterior over its input data, which is now passed to Bottleneck z₂ as a prior for inferring a likely reconstruction (or posterior) over its data. This posterior is then passed back in the other direction (Bottleneck z₂ to Bottleneck z₁) as a new prior over its input data, which will then be used to infer a new posterior distribution. This iterative Bayesian updating repeats multiple times until bottlenecks converge on stable joint posteriors over their respective (now less noisy) bits. IWMT proposes that this operation corresponds to the formation of synchronous complexes as self-organizing harmonic modes (SOHMs), entailing marginalization over synchronized subnetworks—and/or precision-weighting of effectively connected representations—with some SOHM-formation events corresponding to conscious “ignition” as described in Global Neuronal Workspace Theory (Dehaene, 2014). However, this process is proposed to provide a means of efficiently realizing (discretely updated) multi-modal sensory integration, regardless of whether “global availability” is involved. Theoretically, this setup could allow for greater data efficiency with respect to achieving inferential synergy and minimizing reconstruction loss during training in both biological and artificial systems. In terms of concepts from variational autoencoders, this loopy message passing over bottlenecks is proposed to entail discrete updating and maximal a posteriori (MAP) estimates, which are used to parameterize semi-stochastic sampling operations by decoders, so enabling the iterative generation of likely patterns of data, given past experience (i.e., training) and present context (i.e., recent data preceding turbo coding). Note: In turbo coding as used in industrial applications such as enhanced telecommunications, loopy message passing usually proceeds between interlaced decoder networks; within cortex, turbo coding could potentially occur with multiple (potentially nested) intermediate stages in deep cortical hierarchies.

(ii) Turbo coding between folded autoencoders.

This panel shows turbo coding between two folded autoencoders connected by a shared latent space. Each folded autoencoder sends predictions downwards from its bottleneck (entailing reduced-dimensionality latent spaces), and sends prediction errors upwards from its inputs. These coupled folded autoencoders constitute a turbo code by engaging in loopy message passing, which when realized via coupled representational bottlenecks is depicted as instantiating a shared latent space via high-bandwidth effective connectivity. Latent spaces are depicted as having unclear boundaries—indicated by shaded gradients—due to their semi-stochastic realization via the recurrent dynamics. A synchronous beta complex is depicted as centered on the bottleneck latent space—along which encoding and decoding networks are folded—and spreading into autoencoding hierarchies. In neural systems, this spreading belief propagation (or message-passing) may take the form of traveling waves of predictions, which are here understood as self-organizing harmonic modes (SOHMs) when coarse-grained as standing waves and synchronization manifolds for coupling neural systems. Relatively smaller and faster beta complexes are depicted as nested within—and potentially cross-frequency phase coupled by—this larger and slower beta complex. This kind of nesting may potentially afford multi-scale representational hierarchies of varying degrees of spatial and temporal granularity for modeling multi-scale world dynamics. An isolated (small and fast) beta complex is depicted as emerging outside of the larger (and slower) beta complex originating from hierarchically higher subnetworks (hosting shared latent space). All SOHMs may be understood as instances of turbo coding, parameterizing generative hierarchies via marginal maximum a posteriori (MAP) estimates from the subnetworks within their scope. However, unless these smaller SOHMs are functionally nested within larger SOHMs, they will be limited in their ability to both inform and be informed by larger zones of integration (as probabilistic inference).

(iii) Multiplexed multi-scale turbo coding between folded autoencoders.

This panel shows turbo coding between four folded autoencoders. These folded autoencoders are depicted as engaging in turbo coding via loopy message passing, instantiated by self-organizing harmonic modes (SOHMs) (as beta complexes, in pink), so forming shared latent spaces. Turbo coding is further depicted as taking place between all four folded autoencoders (via an alpha complex, in blue), so instantiating further (hierarchical) turbo coding and thereby a larger shared latent space, so enabling predictive modeling of causes that achieve coherence via larger (and more slowly forming) modes of informational integration. This shared latent space is illustrated as containing an embedded graph neural network (GNN) (Liu et al., 2019; Steppa and Holch, 2019), depicted as a hexagonal grid, as a means of integrating information via structured representations, where resulting predictions can then be propagated downward to individual folded autoencoders. Variable shading within the hexagonal grid-space of the GNN is meant to indicate degrees of recurrent activity—potentially implementing further turbo coding—and red arrows over this grid are meant to indicate sequences of activation, and potentially representations of trajectories through feature spaces. These graph-grid structured representational spaces may also afford reference frames at various levels of abstraction; e.g., space proper, degrees of locality with respect to semantic distance, abductive connections between symbols, causal relations, etc. If these (alpha- and beta-synchronized) structured representational dynamics and associated predictions afford world models with spatial, temporal, and causal coherence, these processes may entail phenomenal consciousness. Even larger integrative SOHMs may tend to center on long-distance white matter bundles establishing a core subnetwork of neuronal hubs with rich-club connectivity (van den Heuvel and Sporns, 2011). If hippocampal-parietal synchronization is established (typically at theta frequencies), then bidirectional pointers between neocortex and the entorhinal system may allow decoders to generate likely patterns of data according to trajectories of the overall system through space and time, potentially enabling episodic memory and imagination. If frontal-parietal synchronization is established (potentially involving theta-, alpha-, and beta- synchrony), these larger SOHMs may also correspond to “ignition” events as normally understood in Global Neuronal Workspace Theory, potentially entailing access consciousness and volitional control.