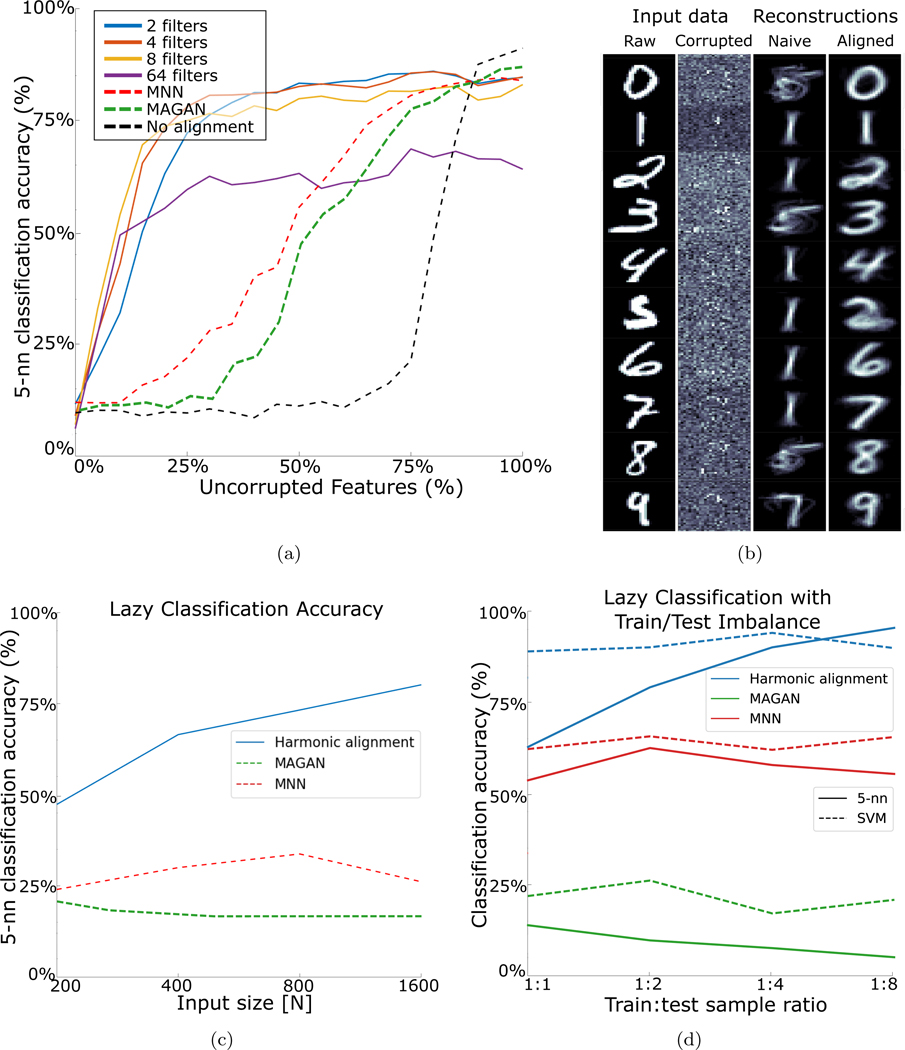
Figure 2:

Recovery of k-nearest neighborhoods under feature corruption. Mean over 3 iterations is reported for each method. (a) At each iteration, two sets X and Y of 1000 points were sampled from MNIST. Y was then distorted by a 784 × 784 corruption matrix O_p for various identity percentages p (§5.1). Subsequently, a lazy classification scheme was used to classify points in Y O_p using a 5-nearest neighbor vote from X. Results for harmonic alignment with $\ell \in \{2,4,8,64\}$ (§4.1), mutual nearest neighbors (MNN), and classification without alignment are shown. (b) Reconstruction of digits with only 25% uncorrupted features. Left: Input digits. Left middle: 75% of the pixels in the input are corrupted. Right middle: Reconstruction without harmonic alignment. Right: Reconstruction after harmonic alignment. (c) Lazy classification accuracy relative to input size with unlabeled randomly corrupted digits with 35% preserved pixels. (d) Transfer learning performance. For each ratio, 1K uncorrupted, labeled digits were sampled from MNIST, and then 1K, 2K, 4K, and 8K (x-axis) unlabeled points were sampled and corrupted with 35% column identity.