Fig. 1.
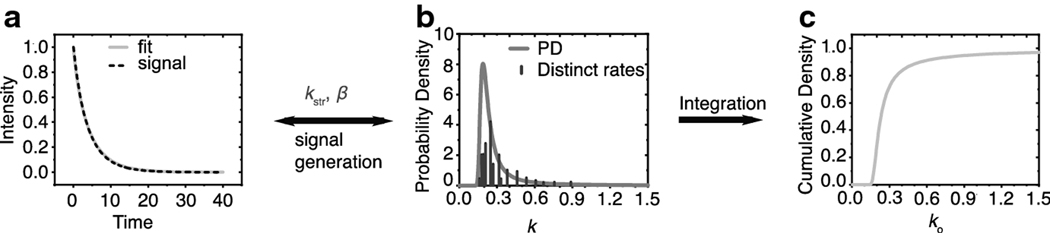
Illustration of the principle of using the SEF as a sum of exponentials. In (a), the dashed line is a signal generated from the sum of 15 random exponential decay constants, which are represented as the vertical lines in (b). The relative heights of these lines reflect their fractional contribution to the signal, and the position the value of the rate constant. The solid line in (a) represents the SEF of the signal with two parameters, kstr and β. These parameters were used to construct the continuous probability distribution as described in Stein et al. [43]. This distribution covers all the rate constants within the signal. (c) The cumulative distribution function was obtained by integrating the area under the probability distribution and can be used to assess the probability of finding a range of rate constants within the signal.