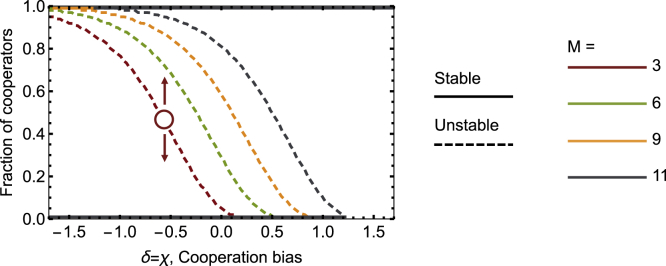
Figure 2.

Under-trust and over-trust (homodirectional biases) impact the likelihood of reaching optimal coordination

In a coordination dilemma (f > 1), when there is no bias (δ = χ = 0), the dynamics of the population are characterized by a coordination threshold that corresponds to the fraction of cooperators above which the population will evolve toward full cooperation and below which it will evolve toward defection. That coordination threshold depends on the threshold within the interacting group, М, necessary for getting the reward. The dashed lines represent unstable equilibria: below them, there are insufficient cooperators, and the population evolves to a state of full defection; above, the population evolves to a state of full cooperation. Full lines at 0 and 1 represent stable equilibria in the fraction of cooperators. The left side of the figure, with negative biases toward cooperation (δ = χ < 0), is part of the under-trust region. The right side, with positive biases toward cooperation (δ = χ > 0), is part of the over-trust region. Over-trust promotes the coordination of a population into a cooperative state, whereas under-trust does the opposite. Effectively, biases toward the existence of cooperators reduce the coordination threshold, facilitating cooperation. Parameters: N = 11, c = 1, b = 10, f = 1.5, and χ = δ.