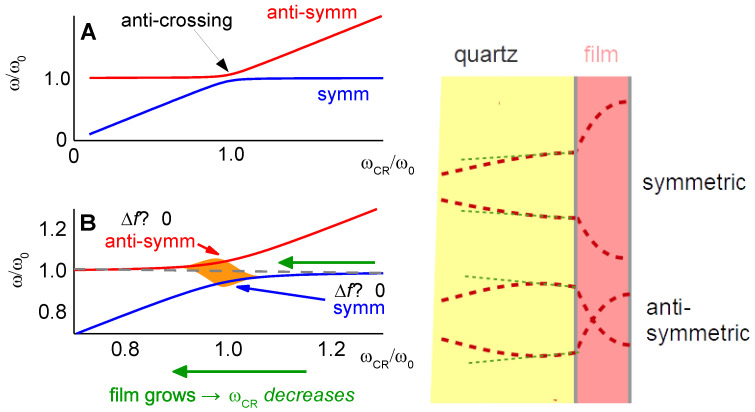
Figure 19.

If the small-load approximation is abandoned, a film resonance produces two separate peaks in the conductance trace, $G_{\mathrm{el}}\left(\mathsf{\omega }\right)$, corresponding to two separate modes of vibration. The shear gradients inside the film have opposite sign for the two modes, hence the labels “symmetric” and “antisymmetric”. Far away from the coupling condition, ${\mathsf{\omega }}_0$ and ${\mathsf{\omega }}_{\mathrm{CR}}$ are not affected by coupling (to the right and to the left in panel (A)). If the two frequencies match and if the two modes indeed couple, anticrossing results. For a more quantitative treatment see Chapter 4.63 in [5]. Panel (B) shows an enlargement of panel (A) in the region of anti-crossing. If the bandwidth is large, the two modes are not actually resolved (sketched in orange in panel (B)). When the film becomes thicker, ${\mathsf{\omega }}_{\mathrm{CR}}$ approaches the coupling condition from above (green arrow). Δf is less than zero, following Sauerbrey. Because the two modes are not resolved at the coupling condition, the peak in $G_{\mathrm{el}}\left(\mathsf{\omega }\right)$ is broad. The center of the peak gradually moves up, because the antisymmetric mode becomes stronger. Eventually, the peak sharpens again and returns to the original frequency from above. A similar behavior is seen in Figure 16, based on Equation (41).