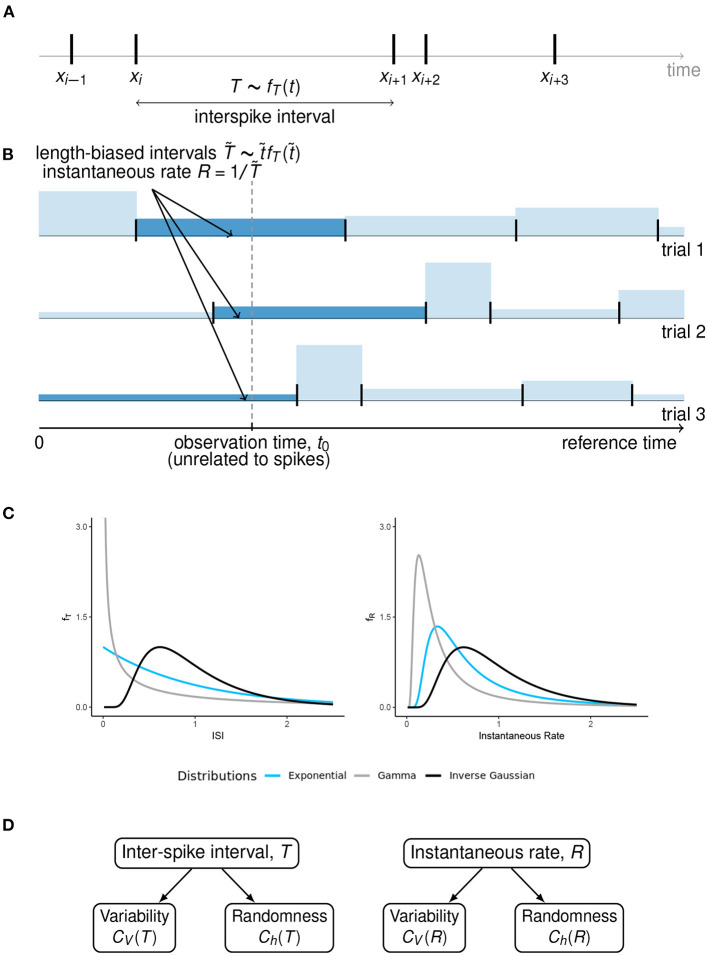
Figure 1.

Length-biased ISIs and instantaneous rate. (A) An overview of the ISIs when the spikes occur at times X_i−1, X_i, X_i+1, …. We assume that the ISIs are independent and identically distributed with pdf f_T(t), under steady state conditions. (B) When the observation time t₀ is fixed with respect to some reference time, unrelated to the spike times, the probability of observing a particular ISI is proportional to its length. These “length-biased” intervals ($\tilde{T}$) are used to define the instantaneous rate R with the property 𝔼(R) = 1/𝔼(T). (C) A graphical representation of how the ISI distributions can visually differ from the instantaneous rate distributions, for some well-known ISI models with equal mean firing rate. (D) A summary of the concepts of variability and randomness for the two ways of spike train description that we have considered in this article.