Figure 5. A lattice model exhibits key characteristics of liquid‐phase condensation with a polymeric subregion as a surface.
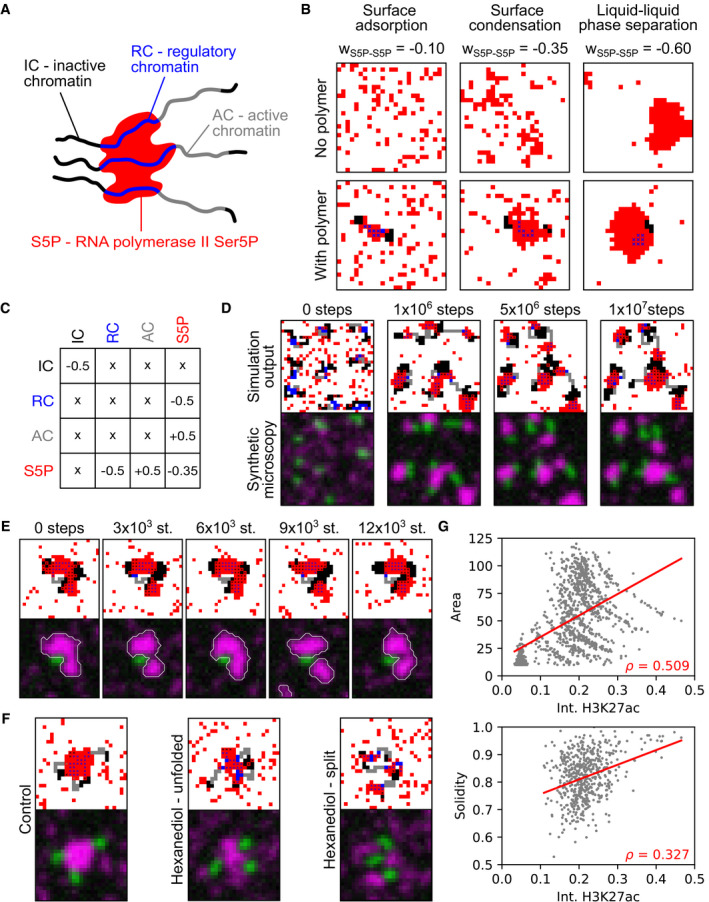
- Sketch of cluster nucleation with the different species involved in the model.
- Examples of lattice configurations obtained from simulations containing only red particles with increasing self‐affinity (w S5P‐S5P, as indicated). Lattice simulations containing a polymer chain of length L polymer = 20 with N IC = 12 black monomers (black‐black affinity w IC‐IC = −0.5) and N RC = 8 blue monomers (blue‐red affinity w RC‐S5P = −0.5, adjustment see Appendix Fig S10), same w S5P‐S5P values as for simulations without polymer chain. All simulations on 25‐by‐25 lattices, N S5P = 100 red particles, for adjustment of N S5P, see Appendix Fig S10A.
- Interaction matrix for different species in the lattice model. Affinity is represented by negative and repulsion by positive values.
- Long time behavior (total of 1 × 107 iteration steps) of the model shown as lattice output and synthetic microscopy images, 30‐by‐30 lattice, N S5P = 140, nine chains, extent of blue and gray regions chosen randomly for each chain at initialization.
- Time‐lapse showing transient separation and merging events of a S5P cluster. 25‐by‐25 lattice, N S5P = 100, four chains.
- Simulated hexanediol treatment. 25‐by‐25 lattices, N S5P = 100, three chains, N RC = 8, N AC = 6, modified parameter values in hexanediol simulations: w S5P‐S5P = −0.15, w S5P‐RC = −0.25.
- Correlations between H3K27ac intensity (RC) and cluster area (in units of lattice cells, n = 1,132) and cluster solidity (only including clusters with area greater 50, n = 551, ρ is the Pearson correlation coefficient, red line—linear fit to guide the eye). Analysis based on 30 simulations of 25‐by‐25 lattices, N S5P = 100, four chains per lattice, extent of blue and gray regions randomly assigned per chain at initialization of each simulation.
