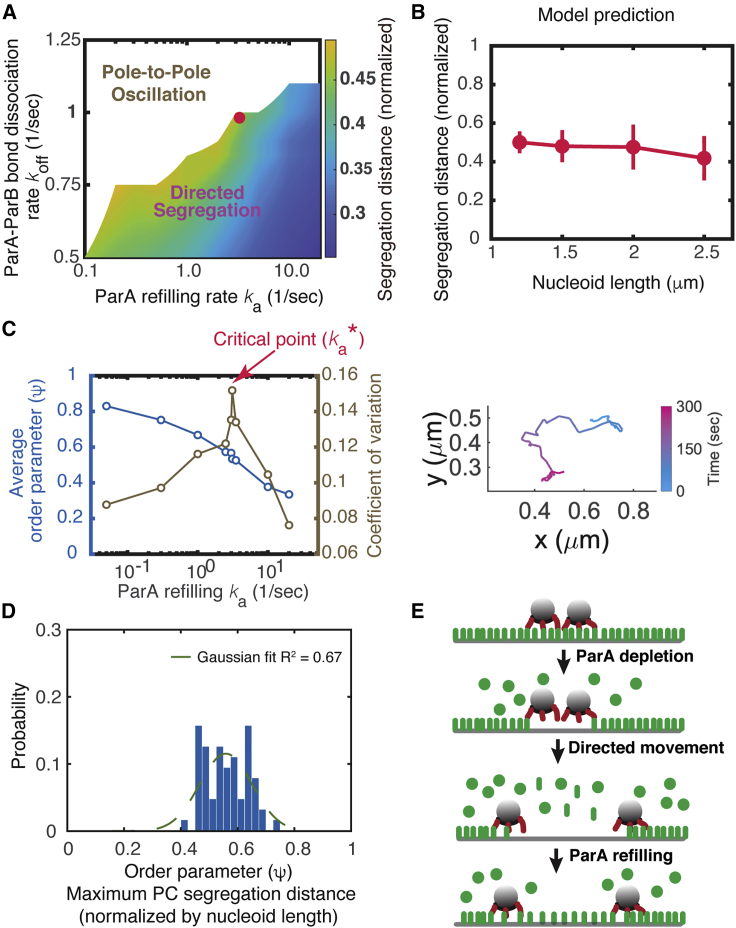
Figure 2.

Predicted feature of near-critical-point operation of ParA-mediated PC partition. (A) Predicted phase diagram of PC motility controlled by (k_a and k_off). Here, the ParA concentration is kept constant as ~3500 molecules per micron of nucleoid length, and other parameters are kept fixed (see Table S1 for details). In our phase diagram calculation, k_off and k_a were varied by changing their absolute values, i.e., k_off = 0.5/s, 0.75/s, 0.85/s, 0.9/s, 1.0/s, etc., and k_a = 0.1/s, 0.2/s, 0.5/s, 1.0/s, 2.0/s, 2.5/s, 3.0/s, 4.0/s, etc. The log scale of k_a reflects the fact that the dependence of model result on k_a is, in general, less sensitive than k_off. The relative smooth phase boundary in the phase diagram reflects a balance between the quality of the figure and computational load. The red dot marks the critical point, which is further defined in (B). (B) Segregation distance adapts to 1/2 of the nucleoid length at the critical point in the parameter space. (C) Predicted characteristics of near-critical-point operation. Left: average order parameter ψ (i.e., averaged maximal segregation distance between PCs normalized by the nucleoid length over ≥36 trajectories of 10-min evolution) increases continuously as the ParA refilling rate decreases, whereas the variance of the order parameter peaks around the critical point $\left(k_{\mathrm{a}}^{\ast }\right)$. Note that the order parameter evolves similarly as a function of the ParA-ParB dissociation rate, k_off. Right: corresponding representative simulation trajectory of PC excursion with the parameter set at this critical point. (D) Predicted statistical distribution of order parameter ψ at the critical point (n = 64). (E) Schematic illustration of the physical nature of near-critical-point partitioning. For (A) and (B), the segregation distance refers to the instantaneous distance between the two PCs at 10 min after they started to segregate.