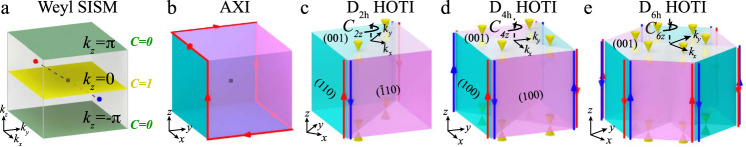
Fig. 5. The five families of 3D symmetry-indicated, spinful, strong topological phases.

In this work, we have computed the complete set of symmetry-indicated spinful topological phases of 3D magnetic and nonmagnetic crystalline solids (see SN 26). We find that, for spinful bands in 3D crystals that satisfy the insulating the compatibility relations along all high-symmetry lines and planes [see SN 16], there are only five families of symmetry-indicated strong topological phases: (a) Smith-index Weyl SMs (Weyl SISMs), (b) axion insulators (AXIs) and 3D TIs defined by the nontrivial axion angle^{19–21,55,63} θ = π [e.g., MnBi₂Te₄^41,42], (c) helical TCIs and higher-order TCIs (HOTIs) equivalent to two superposed AXIs with the same orbital hybridization and twofold rotation or rotoinversion symmetry [e.g., bismuth³³ and MoTe₂³⁴], (d) helical TCIs and HOTIs equivalent to four superposed AXIs with the same orbital hybridization⁵⁴ and fourfold rotation or screw symmetry [e.g. SnTe^24,28], and (e) helical TCIs and HOTIs equivalent to six superposed AXIs with the same orbital hybridization and sixfold rotation or screw symmetry. Through the double SIs calculated for this work (Table 2 and SN 31 and 32), we have discovered the existence of helical magnetic HOTIs with mirror-protected hinge states and bulk topology respectively enforced by the mirror and rotation symmetries of (c) double MPG 8.1.24 mmm [i.e., D_2h, see ref. ¹¹], (d) double MPG 15.1.53 4/mmm [D_4h], and (e) double MPG 27.1.100 6/mmm [D_6h], where we have labeled MPGs using the notation of the CorepresentationsPG tool (see SN 18). The magnetic HOTIs in (c–e) are respectively indicated by the minimal double SIs (c) z₄ = 2 in double MSG 47.249 Pmmm, (d) z₈ = 4 in double MSG 123.339 P4/mmm, and (e) z₁₂ = 6 in double MSG 191.233 P6/mmm [as well as trivial values for all other independent minimal double SIs, see Table 2 and SN 33 for further details].