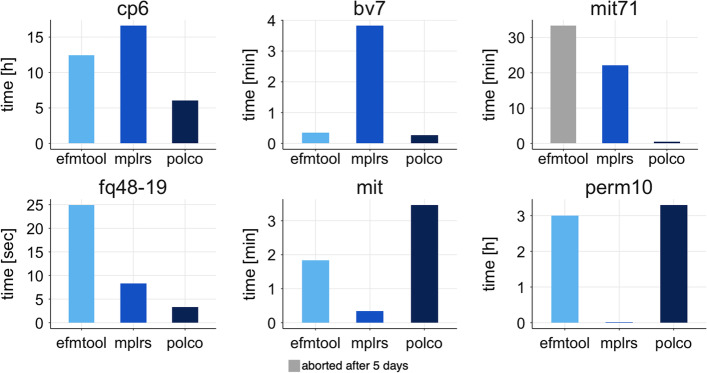
Fig. 5.

Comparison of the run times for six general polyhedra with different grades of degeneracy of mplrs [1] and two representatives of the DDM—efmtool and polco [12]. The y-axis shows the measured wall time in units suitable for the respective calculations, varying from seconds to hours. The x-axis shows the results of the three tools. 20 threads were used for all calculations. For calculations with polco and mplrs, the models were taken in the H-representation as provided on the lrs homepage. Only for use with efmtool, the input matrices first needed to be transformed into a flux cone (9) as described in the method section. To make the comparison as fair as possible no compressions–neither through EFMlrs nor through internal compression methods of polco or efmtool, were applied and no output files were written. The models are sorted in descending order according to their degree of degeneracy. From cp6 and bv7 being highly degenerate to perm10 a simple 9-dimensional polytope. The DDM-based tools were faster for the high to moderate degenerate models, while mplrs outperformed both efmtool and polco on the simple models. For the calculations of the mit71 polco needed 33 s and mplrs 22 min. Unfortunately calculations with efmtool could not be finished and were aborted after 5 days. In the plot this is indicated by a gray column. All in all, our results confirm the widespread assumption that the DDM is faster for degenerate polyhedra, whereas mplrs was faster for the simple polyhedra. This can be seen particularly well in the perm10 model for which mplrs required less than a minute while the DDM based methods needed a bit more than 3 h