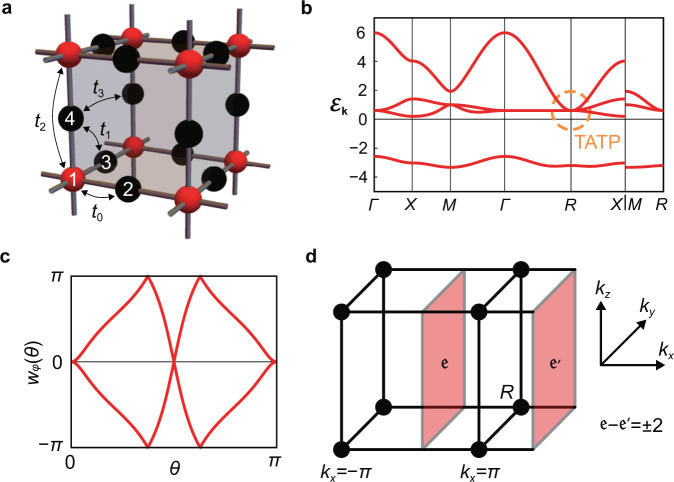
Fig. 4. Avoiding the doubling theorem in 3D electronic Lieb model.

a 3D Lieb lattice structure with four sites in a unit cell. b The electronic band structure with a single TATP at R. Here Γ = (0, 0, 0), X = (π, 0, 0), M = (π, π, 0), and R = (π, π, π). We refer to the highest energy mode forming the TATP as the L mode, and the lower two energy modes forming the TATP as the T modes. c Wilson loop spectrum for the second and third lowest bands, corresponding to T mode of the TATP, computed over a sphere with radius 0.1π centered at R. The winding structure shows that $\mid \mathfrak{e}\mid =2$. d With a single TATP at R (black dots), it is not possible to define $\mathfrak{e}$ in the (k_y, k_z) plane since it conflicts with the periodicity of the Brillouin zone. This contradiction is resolved by noticing the π Zak phases along the k_x, k_y, k_z directions, so that $\mathfrak{e}$ is ill defined.