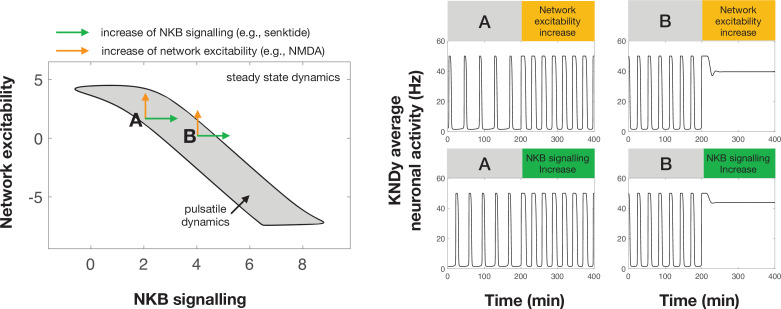
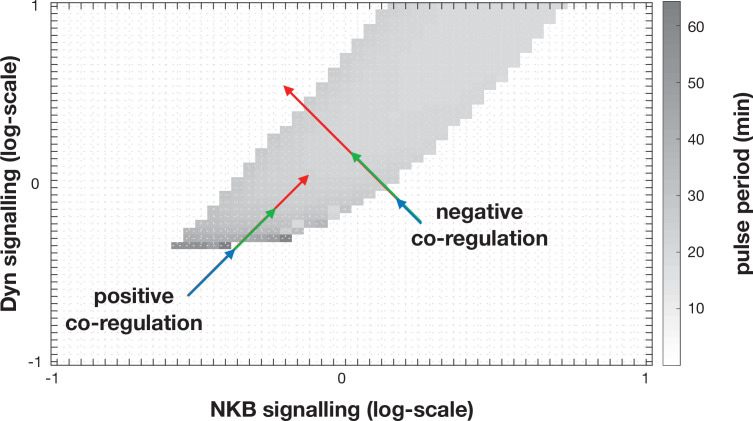
Figure 6. Differential effect of perturbations on the dynamics of the pulse generator.

Two-parameter (NKB signalling and network excitability) bifurcation diagram showing the region in the parameter space for which the system exhibits pulsatile dynamics (grey area). Two points (denoted by A and B) illustrate how an increase in NKB signalling or network excitability could have a differential effect on the dynamics of the system. For point A an increase in network excitability or NKB signalling could lead to an increase in the frequency and width of pulses. However, for point B a similar increase leads to pulse inhibition and steady state system dynamics. Furthermore, a negative correlation in how NKB signalling and network excitability co-vary (i.e. aligned with the direction of the pulsatile regime) make the system dynamics less sensitive to small perturbations and enable more robust control over the cyle.

Figure 6—figure supplement 1. Dynamic behaviour of the KNDY system as a function of dynorphin and NKB singalling.

Positive correlation in the regulation of these two parameters (see positive co-regulation arrows) allows robust control over the system’s dynamics, that is, large enough changes (regardless their actual magnitude) will move the system from the quiescent into the pulsatile regime. Negative correlation in the regulation of the two parameters (see negative co-regulation arrows) makes system dynamics more sensitive to the magnitude of the change (arrow length), for example, large changes can fail to trigger LH pulses.