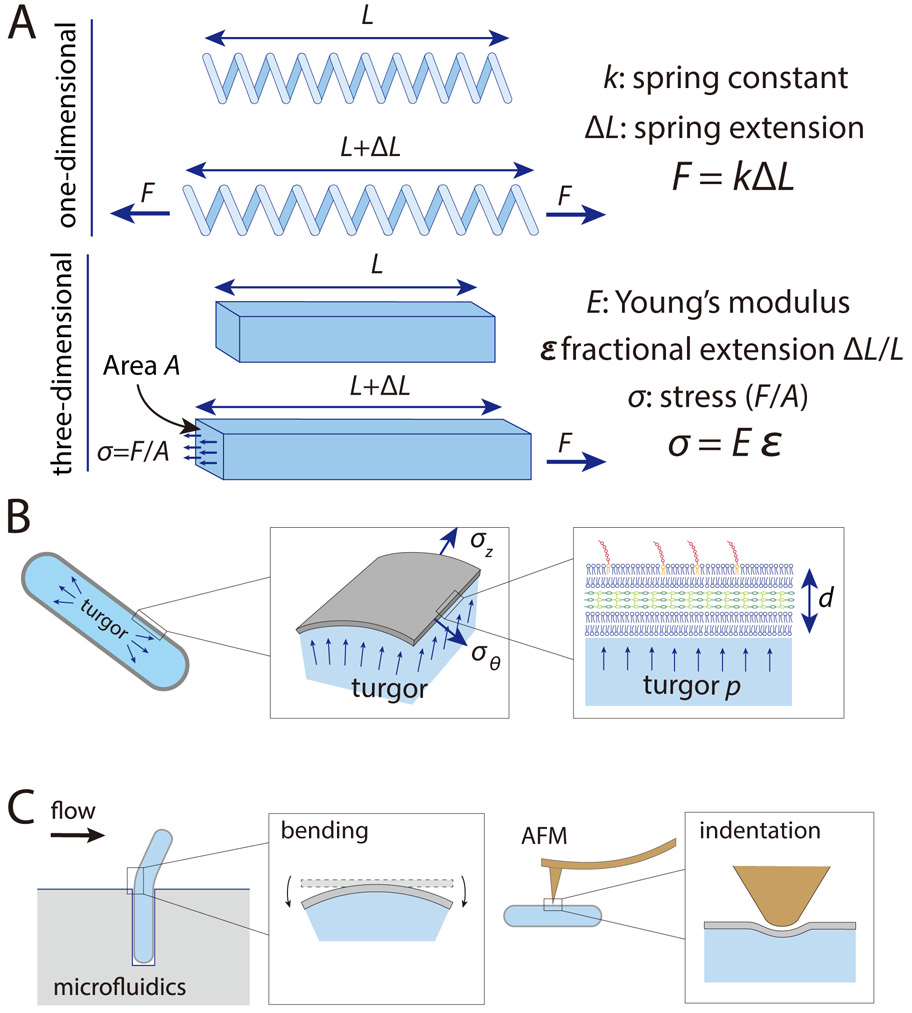
Box Figure: The mechanics of cylindrical thin shells.

A) For a one-dimensional spring with spring constant k, the force required to extend the spring by an amount ΔL is F = kΔL. For a three-dimensional material, Young’s modulus E is the analog of the spring constant, and is the ratio of the stress σ to the fractional extension ε.

B) The cell envelope of a rod-shaped bacterial cell with cross-sectional radius r can be modeled as a thin shell of thickness d (which is much smaller than r) under turgor pressure p. Along the cylindrical portion the stresses in the axial and circumferential directions are approximately ${\sigma }_z=\frac{pr}{2d}$ and ${\sigma }_{\theta }=\frac{pr}{d}$.

C) Bending in a microfluidic chamber (Figure 3B) and AFM indentation (Figure 3C) explore other modes of deformation such as bending and indentation, respectively.