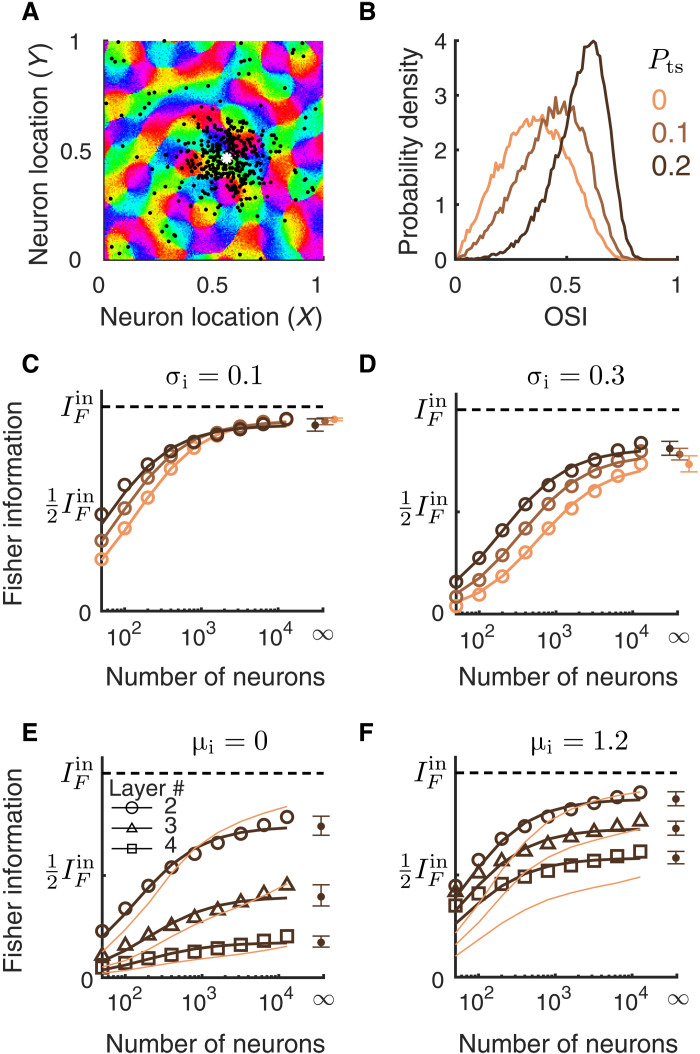
Fig. 10. Networks with tuning-dependent connectivity.

(A) Locations of postsynaptic excitatory neurons (black dots) of one example presynaptic excitatory neuron (white asterisk). The connection probability is defined similar to the networks described previously, except that a percentage (P_ts) of recurrent excitatory connections were rewired to connect similarly tuned neurons across the whole network (see Materials and Methods). (B) The probability density distributions of OSI of the excitatory neurons from L2/3 for varying the percentage of tuning-dependent connections (P_ts). The network parameters are the same as in (C) with σ_i = σ_e = 0.1. (C to F) The linear Fisher information as a function of the number of neurons sampled from the excitatory population for networks with different P_ts [color scheme the same as in (B)]. The asymptotic values of I_F at large N limit (dots at N = ∞) are estimated by fitting Eq. 10 (see Materials and Methods). Solid curves are the fits of I_F. Open circles are the numerical estimation of the linear Fisher information (Eq. 8). Error bars are the 95% confidence intervals. (C) Networks with σ_i = σ_e = 0.1; (D) networks with σ_i = 0.3 and σ_e = 0.1; (E) multilayer networks with P_ts = 0.2 (dark color) or P_ts = 0 (light color), and μ_i = 0; (F) the same as (E) with μ_i = 1.2. The information curves of networks with P_ts = 0 (light color) in (E) and (F) are the same as those in Fig. 9 (B and C).