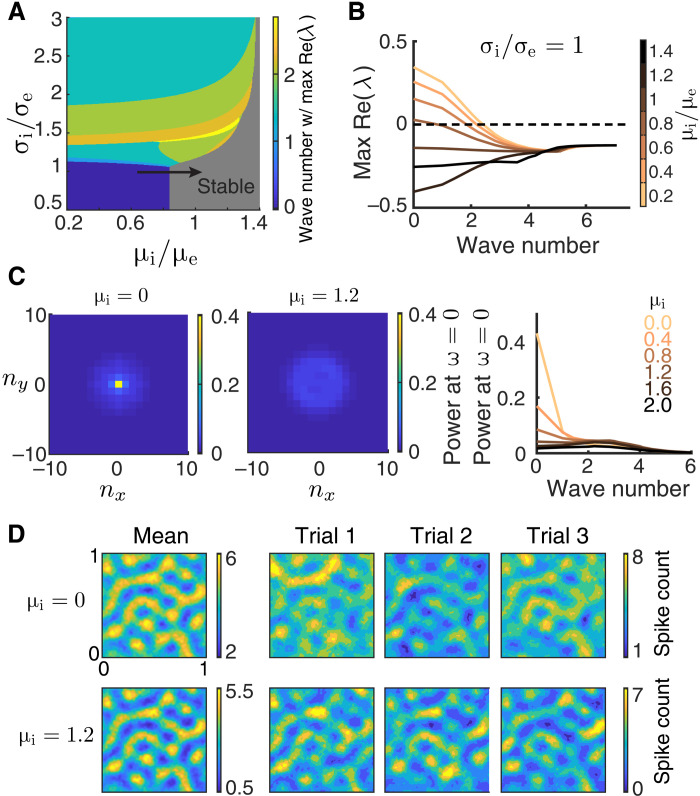
Fig. 7. Lack of inhibitory drive gives rise to network-wide fluctuations.

(A) Bifurcation diagram of a firing rate model (Eq. 6) as a function of the inhibitory projection width σ_i and the depolarization current to the inhibitory neurons. The same as Fig. 5A. (B) The real part of eigenvalues as a function of wave number for increasing μ_i (μ_e = 0.5) when σ_i = σ_e. The zero wave number loses stability when μ_i is small, indicating that the network will exhibit network-wide nonlinear dynamics. (C) The power spectrum at temporal frequency ω = 0 for different spatial Fourier modes (n_x, n_y) of the spontaneous spiking activity from the spiking neuron networks with μ_i = 0 (left) and μ_i = 1.2 (middle). Right: The average power at ω = 0 across wave number ($k=\sqrt{n_x^2+n_y^2}$). The power spectra are computed using a 200-ms time window. (D) Activities of spiking neuron networks with different μ_i when driven by a Gabor image (right, three trials of spike counts within the 200-ms window; left, mean spike counts). Images are smoothed with a Gaussian kernel of width 0.01. Other parameters for the spiking neuron networks (C and D) are σ_e = σ_i = 0.1, α_ffwd = 0.05, J_eF = 140 mV, and J_iF = 0 mV, and the tonic current to excitatory neurons is μ_e = 0. This corresponds to an average current of 1.56 to each excitatory neuron.