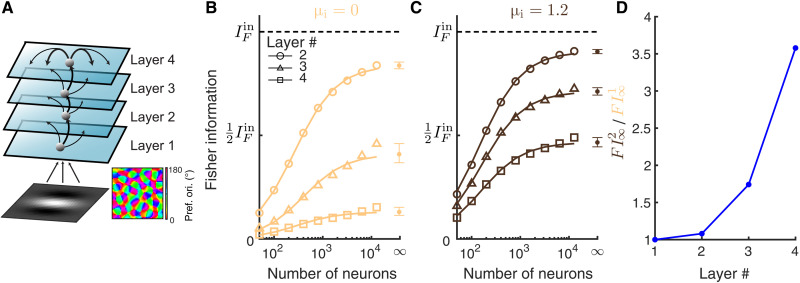
Fig. 9. Information deteriorates in a multilayer model with internal dynamics.

(A) Schematic of a multilayer model. Layer 2 to 4 are recurrent networks of excitatory and inhibitory neurons, with the same projection widths of both recurrent and feedforward connections (α_rec = 0.1 and α_ffwd = 0.05). Recurrent connections of layer 2 and layer 3 are not shown for better illustration. The layer 1 network is the same as the V1 L4 network in Fig. 1A. (B) The linear Fisher information is much more reduced in the higher-order layers when there is no input to the inhibitory neurons (μ_i = 0). The black dashed line is the total input Fisher information from layer 1 (FI_ctin). The asymptotic values of I_F at N = ∞ (dots) are estimated by fitting Eq. 10 (see Materials and Methods). Solid curves are the fits of I_F (the linear fits shown in fig. S1E). Open circles are the numerical estimation of the linear Fisher information (Eq. 8). Error bars are the 95% confidence intervals. (C) The same as B with larger input to the inhibitory neurons (μ_i = 1.2). The linear fits of 1/I_F versus 1/N are shown in fig. S1F. (D) The ratio between the Fisher information estimated at N = ∞ in networks with μ_i = 1.2 (FI₂) and that with μ_i = 0 (FI₁) across layers. The feedforward strengths are adjusted such that the firing rates are similar between the two conditions of μ_i and are maintained across layers (fig. S5C).