Fig 3. Alternative models.

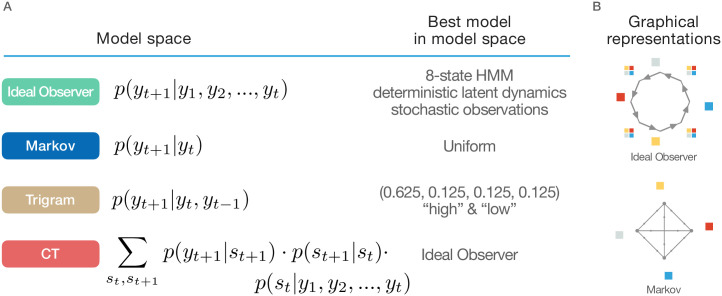
A Table of models and the maximum likelihood parameter sets for the stimuli in our experiment. The ideal observer model (the true generative model of the stimuli) can be formalized as an 8-state HMM with states Pattern1, Random1, Pattern2, Random2, Pattern3, Random3, Pattern4, Random4 where the pattern states produce the corresponding sequence element with probability 1 and all the random states produce any of the four observations with equal probability independently. The Markov model (where predictions are produced by conditioning only on the previous observation) fits the observations best when it predicts all observations with equal probability, since the marginal probabilities of any one stimulus is equal regardless what the previous observation was, because every other trial is random. The trigram model produces a “high triplet” prediction, where the next stimulus is the successor of the stimulus two trials ago in the pattern sequence (the current observation is either a random or a pattern element, each with 50% probability, with conditional probabilities of 100% or 25%, respectively). All alternatives have equal probability of 0.125. Note that the exact probabilities in this case are not relevant since the trials are categorized into two groups (high and low) and therefore the parameters of the response time model and these probabilities are underspecified. The CT model produces a prediction for the next stimulus via filtering. A latent state of the sequence is estimated from previous observations using a Hidden Markov Model. This flexible model space includes the ideal observer model as well as the Markov model as special cases. B Structure of the ideal observer model (top panel) and that of the Markov model (bottom panel). For the description of the graphical elements as Fig 2A.