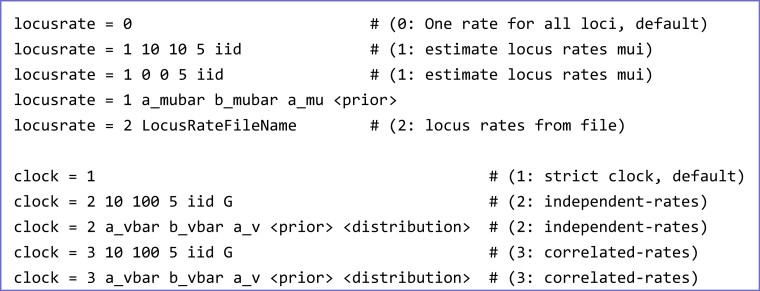
Fig. 2.

The relaxed-clock models are specified using two control variables in bpp: (i) locusrate concerning the overall rate ${\mu }_i$ for locus $i$ and (ii) clock concerning the rate variance parameter ${\nu }_i$ for locus $i$. The locusrate variable is used with any of the three clock models (clocks 1, 2, 3). In the example ${\alpha }_{\overline{\mu }}=10$ and ${\beta }_{\overline{\mu }}=10$ specify the mean overall rate $\overline{\mu }\sim \mathrm{G}({\alpha }_{\overline{\mu }},{\beta }_{\overline{\mu }})$. When there are no fossil calibrations on the species tree, $\overline{\mu }=1$ is fixed, specified using ${\alpha }_{\overline{\mu }}={\beta }_{\overline{\mu }}=0$. Given the mean overall rate $\overline{\mu }$, the overall rates for loci (${\mu }_i$) are generated from the conditional-independence model (iid) or the gamma-Dirichlet model (dir), with the shape parameter ${\alpha }_{\mu }$ ($=$ 5 in the example) specifying how similar ${\mu }_i$ are among loci. The clock variable specifies the three clock models: clock 1 (strict clock), clock 2 (independent-rates model), and clock 3 (correlated-rates model). Under both clock 2 and clock 3, the average rate variance parameter is specified as $\overline{\nu }\sim \mathrm{G}({\alpha }_{\overline{\nu }},{\beta }_{\overline{\nu }})$; in the example ${\alpha }_{\overline{\nu }}=10$ and ${\beta }_{\overline{\nu }}=100$ with mean 0.1. Given $\overline{\nu }$, the variance ${\nu }_i$ for locus $i$ is similarly generated from the iid or dir models. Given the overall rate ${\mu }_i$ and the rate variance parameter ${\nu }_i$ for locus $i$, rates for branches at locus $i$ are specified for clock 2 and clock 3 using either the gamma (G) or log-normal (LN) distributions.