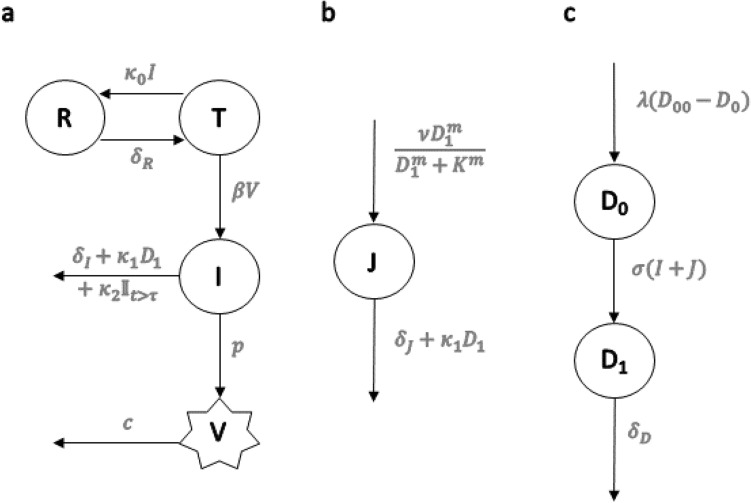
Figure 1.

Schematic representation of the model described by Eqs. (1–7). Target cells ($T$) transition to a productively infected state ($I$) after successful infection by virions ($V$) at rate β. Virions are cleared at per capita rate c, while new virions are produced by infected cells at rate p. Target cells become refractory to infection ($R$) at rate ${\kappa }_{0}I$ (we assume target cells are exposed to a concentration of type-I IFN that is proportional to $I$ and that puts the cells into an antiviral state). Resting innate immune cells ($D_0$) become activated ($D_1$) at rate σ (I + J), where I and J are the number of infected and damaged bystander cells, respectively (we assume the extent of PAMP and DAMP signaling is proportional to $I$+$J)$. Also, activated immune cells ($D_1$) die at per capita rate ${\delta }_D$. Damaged bystander cells ($J$) are generated from an extensive proinflammatory response at a rate that is a Hill function of the number of activated immune cells $D_1$. Infected cells die due to viral cytopathic effects at rate ${\delta }_I$ and damaged cells die from their injury at rate ${\delta }_J$. The clearance of these cells also occurs by the action of activated innate immune cells at rate ${\kappa }_1{D}_1$. The effect of the adaptive immune response is modeled by adding a constant term κ₂ to the clearance of infected cells at time τ after infection. Finally, homeostatic processes allow replenishment of the population of resting cells $\left(D_0\right)$ at rate $\mathrm{\lambda }(D_{00}-D_0)$, where D₀₀ is their homeostatic level_.