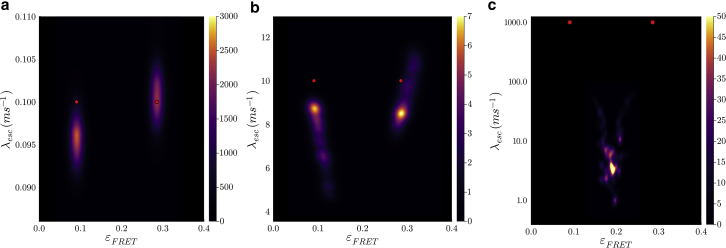
Figure 7.

Learned bivariate posterior for the system state escape rates ${\lambda }_{esc}$ (log-scale in (c)) and FRET efficiencies ${ϵ}_{FRET}$ from synthetic data. For all synthetic smFRET traces, we use an excitation rate of 10 ms⁻¹ and FRET efficiences of 0.29 and 0.09 for the two system states, respectively. The three panels correspond to different timescales being probed with transitions rates: (a) 0.1 ms⁻¹; (b) 10 ms⁻¹; and (c) 1000 ms⁻¹. The ground truth values are shown with red dots. The bias in the parameter estimates increases as faster kinetics are probed, demonstrating deterioration of the information content of the collected data resulting in expectedly poor estimation assuming a fixed photon budget of 670,000. This can also be seen quantitatively by calculating the confidence intervals reported below for each case. The FRET efficiencies estimated by our sampler for the slowest case in (a) are ${0.286}_{-0.002}^{+0.002}$ and ${0.091}_{-0.001}^{+0.001}$ ms⁻¹, and the corresponding escape rates are ${0.101}_{-0.005}^{+0.004}$ and ${0.096}_{-0.004}^{+0.004}$ ms⁻¹. For the intermediate case in (b), FRET efficiencies estimated by our sampler are ${0.200}_{-0.110}^{+0.117}$ and ${0.102}_{-0.014}^{+0.022}$, and predicted escape rates are ${8.47}_{-3.17}^{+2.42}$ ms⁻¹ and ${7.67}_{-2.66}^{+1.32}$ ms⁻¹. For the fastest case in (c), FRET efficiencies estimated by our sampler are ${0.189}_{-0.027}^{+0.025}$ and ${0.189}_{-0.029}^{+0.016}$, and predicted escape rates are ${5.00}_{-3.63}^{+26.9}$ ms⁻¹ and ${3.49}_{-2.49}^{+27.21}$ ms⁻¹. Poorer confidence intervals for larger escape rates reflect larger uncertainty due to lack of information.