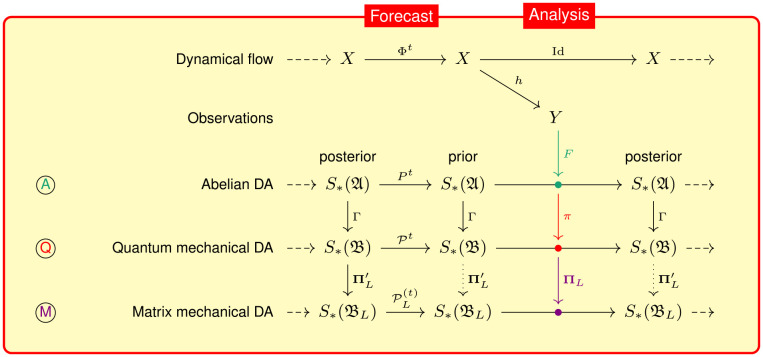
Fig. 1.
Schematic representation of the abelian and nonabelian formulations of sequential data assimilation (DA), showing a forecast–analysis cycle. The Top row of the diagram shows the dynamical flow Φt : X → X. The second row shows the observation map h : X → Y used to update the state of the DA system in the analysis step. The rows labeled  ,
,  , and
, and  show the abelian, infinite-dimensional nonabelian (quantum mechanical), and finite-dimensional nonabelian (matrix mechanical) DA systems, respectively. In
show the abelian, infinite-dimensional nonabelian (quantum mechanical), and finite-dimensional nonabelian (matrix mechanical) DA systems, respectively. In  , the forecast step is carried out by the transfer operator acting on states of the abelian algebra . The analysis step (green dot) is represented by an effect-valued map that updates the state given observations in Y. In
, the forecast step is carried out by the transfer operator acting on states of the abelian algebra . The analysis step (green dot) is represented by an effect-valued map that updates the state given observations in Y. In  , the forecast step is carried out by the transfer operator acting on states of the nonabelian operator algebra . The analysis step (red dot) is carried out by an effect given by the composition of F with the regular representation π of into (red arrow). The state space is embedded into by means of a map Γ, which is compatible with both forecast and analysis; Eqs. 4 and 9. This compatibility is represented by the commutative loops between
, the forecast step is carried out by the transfer operator acting on states of the nonabelian operator algebra . The analysis step (red dot) is carried out by an effect given by the composition of F with the regular representation π of into (red arrow). The state space is embedded into by means of a map Γ, which is compatible with both forecast and analysis; Eqs. 4 and 9. This compatibility is represented by the commutative loops between  and
and  having Γ as a vertical arrow. To arrive at the matrix mechanical DA,
having Γ as a vertical arrow. To arrive at the matrix mechanical DA,  , we project into an L2-dimensional operator algebra using a positivity-preserving projection . The composition of this projection with ℱ leads to an effect employed in the analysis step (purple arrow and dot). Moreover, induces a state space projection and a projected transfer operator employed in the forecast step. Vertical dotted arrows indicate asymptotically commutative relationships that hold as L → ∞.
, we project into an L2-dimensional operator algebra using a positivity-preserving projection . The composition of this projection with ℱ leads to an effect employed in the analysis step (purple arrow and dot). Moreover, induces a state space projection and a projected transfer operator employed in the forecast step. Vertical dotted arrows indicate asymptotically commutative relationships that hold as L → ∞.