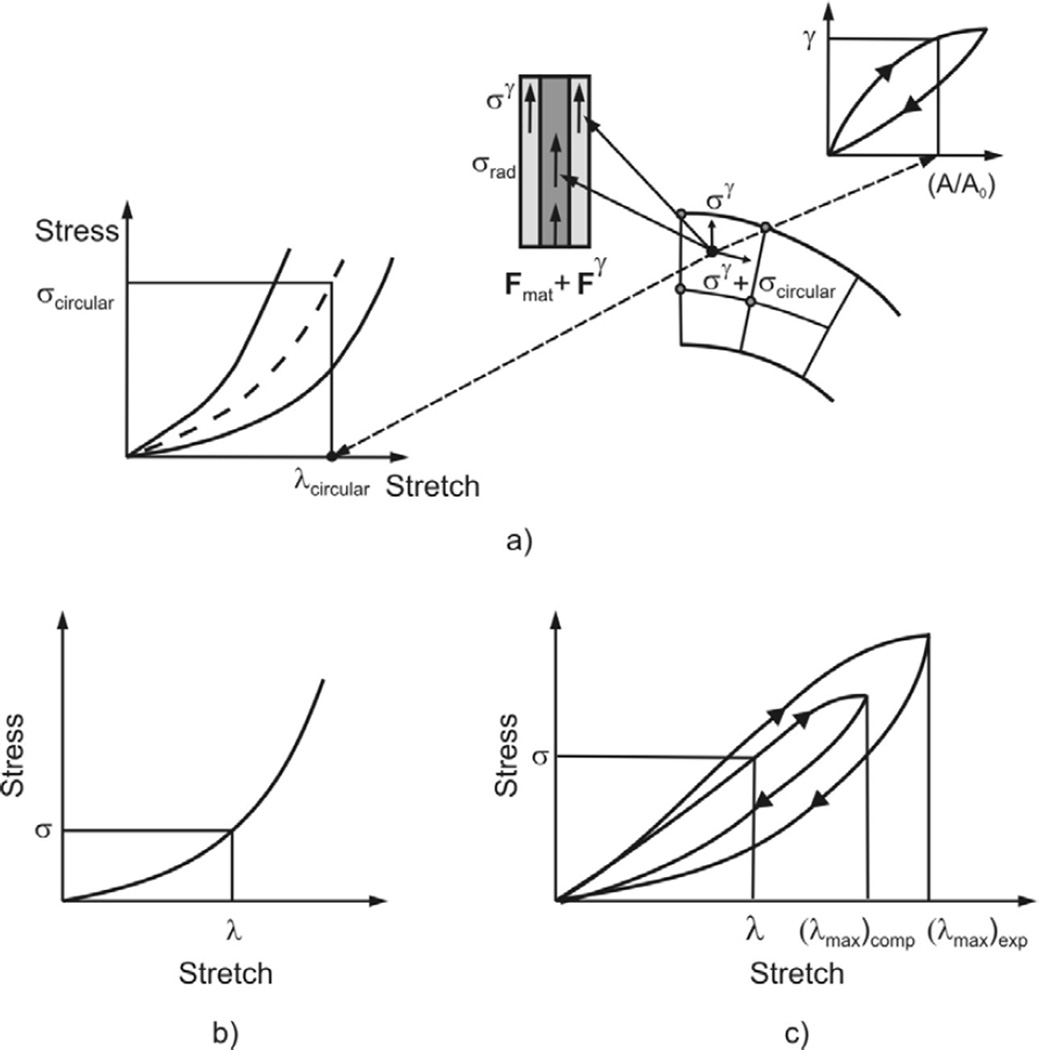
Figure A1.
Sketch of stress calculation. Stresses correspond to the previous iteration (indexed by i-1 in equation A1). a) At each material point of the radial septum the stress is represented as a sum of the stresses due to material deformation (radial stress σrad and circumferential stress σcircular) and due to surfactant σγ. These stresses are evaluated from constitutive laws for material (biaxial model) and surfactant (hysteretic model). The stresses produce the finite element nodal forces Fint = Fmat + Fγ entering the equilibrium equations for the finite element assemblage. b) and c) Stresses within the ring evaluated for nonlinear elastic and hysteretic material models.