Ten‐year longitudinal changes in muscle power, force, and velocity in young, middle‐aged, and older adults

Abstract

Background

Maximum muscle power (P_max) is a biomarker of physical performance in all ages. No longitudinal studies have assessed the effects of aging on P_max obtained from the torque‐velocity (T‐V) relationship, which should be considered the ‘gold standard’. This study evaluated the longitudinal changes in the T‐V relationship and P_max of the knee‐extensor muscles in young, middle‐aged, and older adults after 10 years of follow‐up.

Methods

Four hundred eighty‐nine subjects (311 men and 178 women; aged 19–68 years) were tested at baseline and after a 10‐year follow‐up. Anthropometric data, daily protein intake, physical activity level (PAL), and knee‐extension muscle function (isometric, isokinetic, and isotonic) were evaluated. A novel hybrid equation combining a linear and a hyperbolic (Hill‐type) region was used to obtain the T‐V relationship and P_max of the participants, who were grouped by sex and age (young: 20–40 years; middle‐aged: 40–60 years; and old: ≥60 years). Linear mixed‐effect models were used to assess effects of time, sex, and age on T‐V parameters, P_max, and body mass index (BMI). Additional analyses were performed to adjust for changes in daily protein intake and PAL.

Results

P_max decreased in young men (−0.6% per year; P < 0.001), middle‐aged men and women (−1.1% to −1.4% per year; P < 0.001), and older men and women (−2.2% to −2.4% per year; P ≤ 0.053). These changes were mainly related to decrements in torque at P_max at early age and to decrements in both torque and velocity at P_max at older age. BMI increased among young and middle‐aged adults (0.2% to 0.5% per year; P < 0.001), which led to greater declines in relative P_max in those groups. S/T₀, that is, the linear slope of the T‐V relationship relative to maximal torque, exhibited a significant decline over time (−0.10%T₀·rad·s⁻¹ per year; P < 0.001), which was significant among middle‐aged men and old men and women (all P < 0.05). Annual changes in PAL index were significantly associated to annual changes in P_max (P = 0.017), so the overall decline in P_max was slightly attenuated in the adjusted model (−5.26 vs. −5.05 W per year; both P < 0.001).

Conclusions

P_max decreased in young, middle‐aged, and older adults after a 10‐year follow‐up. The early declines in P_max seemed to coincide with declines in force, whereas the progressive decline at later age was associated with declines in both force and velocity. A progressively blunted ability to produce force, especially at moderate to high movement velocities, should be considered a specific hallmark of aging.

Introduction

Muscle power is defined as the rate at which mechanical work is performed and is one of the main biomarkers of physical performance in humans during actions that can be determining for success in sport activities in the case of athletes ¹ or in activities of daily living in older individuals. ² In the latter case, muscle power is negatively and independently associated with the risk of cognitive decline, ³ mobility limitations and disability, ⁴ , ⁵ hospitalization, ⁶ and mortality in older adults. ⁶ , ⁷ The clinical relevance of low muscle power seems to exceed that shown by sarcopenia (i.e., low muscle mass and strength). ⁸ , ⁹ Previous studies have shown that the decline in muscle power initiates after the age of 40 years in both women and men, although between‐sex differences can be observed during certain stages of life, ¹⁰ , ¹¹ which makes it necessary to investigate men and women separately. Evidence from studies conducted in master athletes even suggests that, despite their greater muscle power levels throughout the lifespan when compared with their sedentary counterparts, the age‐related decline in power is inevitable. ¹²

The amount of muscle power that an individual produces varies as a function of movement velocity, showing lower values under either relatively slow or fast contractions (i.e., heavy and light loading conditions, respectively) and higher values (maximum muscle power (P_max)) during moderate contraction velocities (i.e., moderate loading conditions). ¹³ Therefore, P_max (i.e., apex of the power‐velocity (P‐V) curve) can occur at different absolute and relative loading conditions among study participants. Unfortunately, age‐related declines in muscle power are usually derived from studies in which muscle power was assessed under a single loading condition, ¹⁰ , ¹¹ , ¹⁴ so it is possible that differences in muscle power among participants and over time are at least partially due to the chosen loading conditions. A ‘gold standard’ approach to determine the effect of aging on P_max would be the assessment of the force‐velocity (F‐V) or torque‐velocity (T‐V) relationship and recording of derived muscle power values across contraction velocities. This method can also help discern whether decrements in P_max are related to changes in force, velocity, or both. ¹⁵ Results derived from a cross‐sectional study suggest that the force component is already relevant in mid‐life, whereas the velocity component becomes relevant at an older age. ¹⁶ Importantly, these changes are driven by different physiological mechanisms (e.g., decrease in muscle size ¹⁷ vs. slower single muscle fibre phenotype ¹⁸ ), having important implications for designing effective countermeasures. Nevertheless, no previous longitudinal studies using the above‐mentioned approach have been found in the literature. ¹⁹ The evidence about changes in muscle power with aging is limited to either cross‐sectional studies, ¹⁰ , ¹² longitudinal studies that assessed upper‐limb, but not lower‐limb, muscle power, ¹¹ or studies that did not measure P_max obtained from the F‐V or T‐V relationship. ¹⁹

Therefore, the main goal of the present investigation was to assess the longitudinal changes in the T‐V and P‐V relationships, and in P_max of the knee extensors in community‐dwelling young, middle‐aged, and older men and women. Our main hypotheses were that P_max would decrease with aging in young, middle‐aged, and older participants, whereas these decrements would be motivated mainly by torque‐related factors in young individuals and by both torque‐ and velocity‐related factors in middle‐aged and older participants.

Methods

Study design

This is a longitudinal study in which data were collected in the framework of the first (2002–2004; baseline) and third (2012–2015; follow‐up) waves of the Flemish Policy Research Center on Sport. The study sample was randomly selected among community‐dwelling people aged 18 to 80 years living in the region of Flanders (Belgium), as previously described in detail. ²⁰ , ²¹

Participants

A total of 1569 subjects (923 men and 646 women) were tested at baseline, of which a total of 652 subjects (420 men and 232 women) were tested again at follow‐up. Exclusion criteria, reasons for dropping out, and differences between the drop‐out and follow‐up participants have been previously reported. ²¹ , ²² Briefly, subjects were excluded in the case of cardiovascular disease or acute thrombosis, recent surgery, neuromuscular disease, infection or fever, diabetes, and/or pregnancy. In the current study, only those participants that presented the full set of data from the anthropometric and T‐V assessments were included. Thus, a total 489 subjects (178 women and 311 men; aged from 19 to 68 years at baseline) participated in this longitudinal study (Table 1). Median follow‐up time and inter‐quartile range was 9.6 [9.3–10.4] years. All the subjects gave their written informed consent; all the procedures were performed in accordance with the Declaration of Helsinki, and the study was approved by the Ethics Committee Research UZ/KU Leuven.

Table 1.

Main characteristics of the study participants.

	Age groups
	Young (20.0–39.9 years)	Middle‐aged (40.0–59.9 years)	Old (≥60.0 years)
Women, n	44	124	10
Age, years	33.2 ± 5.8	46.9 ± 5.2*, a	62.9 ± 2.8ab
Height, m	1.65 ± 0.05*	1.65 ± 0.06*	1.61 ± 0.10*
Body mass, kg	61.4 ± 8.1*	63.9 ± 8.9*	59.5 ± 6.6*
BMI, kg/m2	22.4 ± 2.4*	23.3 ± 3.0*	22.9 ± 3.4*
Protein, g·kg−1	1.24 ± 0.35	1.29 ± 0.38	1.37 ± 0.32
PAL index	1.73 ± 0.25	1.71 ± 0.16*	1.59 ± 0.13
EEsports, MET·h·week−1	26.0 ± 37.4	24.7 ± 30.1	14.8 ± 14.3
Men, n	85	202	24
Age, years	33.1 ± 5.9	47.9 ± 4.9*, a	63.9 ± 2.5ab
Height, m	1.78 ± 0.06*	1.77 ± 0.06*	1.73 ± 0.06* ab
Body mass, kg	76.2 ± 10.6*	79.8 ± 10.0*, a	78.6 ± 8.3*
BMI, kg/m2	23.9 ± 2.8*	25.4 ± 2.7*, a	26.2 ± 2.8*, a
Protein, g·kg−1	1.25 ± 0.35	1.23 ± 0.32	1.31 ± 0.30
PAL index	1.80 ± 0.21	1.79 ± 0.22*	1.70 ± 0.20
EEsports, MET·h·week−1	22.2 ± 23.8	23.6 ± 23.7	20.3 ± 20.7

Note: Baseline differences were assessed using linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID).

Abbreviations: BMI, body mass index. PAL, physical activity level. EE_sports, energy expenditure in sports.

^*Significant differences between men and women (P < 0.05).

^a Significant differences compared with young adults (P < 0.05).

^b Significant differences compared with middle‐aged adults (P < 0.05).

Outcomes

Anthropometrics

The participants were barefoot and with minimal clothing for the anthropometric measurements. Height was measured to the nearest 0.01 m using a stadiometer (Holtain, Crymych, UK), and body mass was measured to the nearest 0.1 kg with a digital scale (Seca 841, Seca GmbH, Hamburg, Germany). Body mass index (BMI) was calculated as the ratio between body mass and height squared (kg·m⁻²).

Protein intake

Protein intake was assessed by asking the participants to register all food and drinks consumed in a validated 3‐day diet record (two weekdays and one weekend day). ²³ The food diary was sent to the participants prior to the test session in the laboratory, so that they could fill it in beforehand. The participants had to weigh the amount of food and drinks or otherwise estimate the amounts by using standard household measures (e.g., spoon and cup). Then, daily protein intake relative to body mass (g·kg⁻¹) was calculated using Becel Nutrition software (Unilever CO., Rotterdam, the Netherlands).

Physical activity

Physical activity and participation in sports was assessed by the Flemish Physical Activity Computerized Questionnaire. ²⁴ Briefly, this questionnaire registers information on the participants' engagement in leisure activities and sports participation, household and garden activities, transportation, occupation, sleeping, and sedentary behaviour in a normal week. From these data, energy expenditure due to sport activities (EE_sports) was estimated based on the Compendium of Physical Activity as the average metabolic equivalents of energy expenditure hours per week (MET·h·week⁻¹). ²⁵ Physical activity level (PAL) was assessed as the ratio between daily total energy expenditure and basal metabolic rate. ²⁴

Knee extension torque‐velocity relationship and muscle power

Unilateral knee extension muscle function of the right leg (left leg in case of history of injury) was assessed using an isokinetic dynamometer (Biodex System Pro 3, Biodex Medical Systems, USA). Torque and angular velocity signals were sampled at 100 Hz and processed offline through a commercial software package (Matlab R2015b; The MathWorks Inc., USA). Subjects were seated upright on the dynamometer chair with the axis of the dynamometer corresponding to the knee joint axis. The participants' shoulder, waist, thigh, and lower leg were secured with straps, and arms were kept crossed over the chest during all the trials. After an adequate familiarization and warm‐up, several isometric, isotonic, and isokinetic trials were carried out. Briefly, peak torque values were recorded during maximal isometric contractions (3–4 s) at knee joint angles of 30°, 60°, and 90° (full extension = 0°). Two trials were performed for each isometric condition and the highest peak torque (Nm) registered among the six trials was defined as the maximum isometric torque. In addition, peak torque and corresponding angular velocity values were recorded during maximal isotonic contractions against loads corresponding to 40%, 20%, 0%, and 60% of maximum isometric torque (three trials per each loading condition), and during maximal isokinetic contractions performed at 1.05 rad·s⁻¹ (four trials) and 4.19 rad·s⁻¹ (six trials) (i.e., 60° and 240°·s⁻¹, respectively). Torque (Nm) and angular velocity (rad·s⁻¹) at peak power registered against each load/angular velocity was used for further analysis. All the participants received the instruction to perform every repetition as fast and strong as possible through the whole range of movement (90° to full extension). Strong verbal encouragement was provided during each trial, and adequate resting periods (20–120 s) were allowed between trials and loading conditions.

Torque‐velocity equation

In recent years, the linear F‐V relationship has been suggested as a featured property of only multi‐joint muscle actions. However, the linear F‐V relationship is a characteristic also shared by single‐joint muscle actions. ¹⁸ , ²⁶ In both multi‐joint and single‐joint muscle actions, force decreases linearly as a function of velocity in a specific limited region (from high to moderate forces), whereas force decreases as velocity increases in a curvilinear (convex) fashion in other region (from moderate to null forces). ²⁷ Therefore, a hybrid equation ²⁸ (Equation 1 ) that combines a linear and a curvilinear (Hill‐type) region was used in the current study. Briefly, this equation includes the classical linear ²⁹ and hyperbolic ³⁰ equations, as well as an associated coefficient to each of the two [c ₁ (Equation 2 ) and c ₂ (Equation 3 ), respectively] that provides different albeit complementary weights (c ₁ + c ₂ = 1) to each of them as a function of relative intensity (i.e., in this case torque relative to maximum isometric torque). ²⁸

$$\mathrm{V}=c_1\left[\frac{\mathrm{T}-{\mathrm{T}}_0}{S}\right]+c_2\left[\frac{\left({\mathrm{T}}_0-\mathrm{T}\right)\mathrm{b}}{\mathrm{T}+\mathrm{a}}\right]$$ (1)

$$c_1=\frac{1}{1+{\mathrm{e}}^{{\left(\mathrm{k}-\mathrm{T}/{\mathrm{T}}_0\right)}^S}}$$ (2)

$$c_2=\frac{1}{1+{\mathrm{e}}^{{\left(\mathrm{T}/{\mathrm{T}}_0-\mathrm{k}\right)}^S}}$$ (3)

where V is velocity (angular velocity in this case), c ₁ is the coefficient associated to the linear equation, c ₂ is the coefficient associated to the hyperbolic equation, T is torque, T ₀ is estimated maximum isometric torque, S is the slope of the linear region, a and b are Hill‐type constants, k is a constant that determines the point of transition from the linear to the hyperbolic equation (i.e., when c ₁ and c ₂ are both 0.5), and s is a constant that determines how smooth the model runs from the linear to the hyperbolic region. Estimated maximal unloaded velocity (V₀) was calculated as the intercept of the velocity axis, and S/T₀ and a/T₀ were calculated to describe the decrease in torque as a function of contraction velocity in the linear (i.e., slope) and hyperbolic (i.e., curvature) regions, respectively, of the T‐V relationship. Power was calculated as the product of torque and angular velocity; maximum muscle power (P_max) was identified at the apex of the P‐V relationship, and optimal torque (T_opt) and optimal velocity (V_opt) as those produced at P_max. With the torque and angular velocity values measured in the current experiments, and k = 0.45 based on previous evidence, ³¹ a commercial software package (Solver VBA, Microsoft Excel, USA) was used to calculate the rest of the constants of the T‐V equation (non‐linear least squares method). To ensure an optimal assessment of the T‐V relationship, and based on basic physiological principles, the following automatized instructions were implemented in the software for the selection of suitable torque and angular velocity data (Figure 1): Maximum isometric torque values lower than any of the registered dynamic torque values were discarded; dynamic trials showing a lower torque value for a corresponding angular velocity compared with a faster contraction were discarded; and dynamic trials showing a lower power for a corresponding angular velocity compared with both a slower and a faster contraction were discarded. In addition, those isometric or dynamic trials that by visual inspection showed diminished torque compared with the one that would be expected according to the rest of the trials were discarded only if after excluding those trials the difference between measured and estimated values reached ≥10%. Participants that did not have at least three suitable trials (at least one trial below 45% and two trials above 45% of T₀) were excluded from further analysis. The average number of T‐V data points included was 5.4 ± 1.1 (excluded: 1.6 ± 1.0), coefficient of determination (R²) was 0.99 ± 0.02, and standard error of the estimate (SEE) was 0.44 ± 0.32. A total of 50 participants (baseline and follow‐up; i.e., 100 T‐V tests) were randomly selected and assessed independently by two different evaluators to assess the inter‐rater reliability of this procedure. Reliability values (intraclass correlation coefficient (ICC_2,1) for absolute agreement [95% confidence interval]) for the main outcomes derived from the T‐V relationship were T₀: 0.99 [0.98–0.99]; V₀: 0.85 [0.77–0.90]; and P_max: 0.99 [0.99–0.99].

Figure 1.

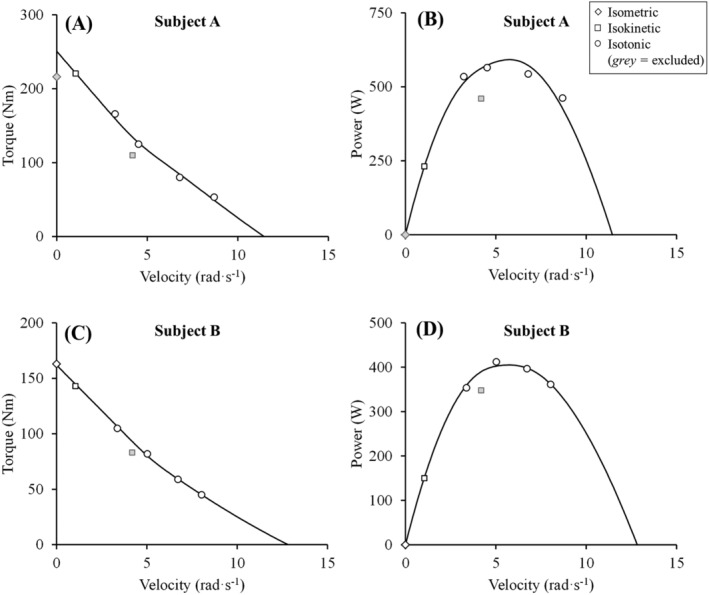
Analysis of the torque‐velocity (A and C) and power velocity (B and D) relationships in two participants (subject 1: A and B; subject 2: C and D). A hybrid equation ²⁸ was applied to measured data after excluding those data that fulfilled the following criteria: Maximum isometric torque values lower than any of the registered dynamic torque values (in A); dynamic trials showing a lower torque value for a corresponding velocity compared with a faster contraction (in A); and dynamic trials showing a lower power for a corresponding velocity compared with both a slower and a faster contraction (in B and D).

Data analyses

Next to the analyses of absolute values, torque and power outcomes were normalized to body mass (i.e., relative T₀ and relative P_max). Relative values have a greater functional relevance compared with absolute values. ² , ³² Longitudinal yearly changes (i.e., ∆·year⁻¹) in daily protein intake, physical activity behaviour, and outcomes derived from the T‐V and P‐V relationships (both absolute and relative to body mass) were calculated as the difference between follow‐up and baseline values divided by follow‐up time in years; then, percentage changes (i.e., %∆·year⁻¹) were calculated relative to baseline levels.

Statistical analyses

Data were presented as mean ± standard deviation or 95% confidence interval unless otherwise stated. Cross‐sectional baseline or follow‐up differences regarding anthropometry, daily protein intake, physical activity behaviour, and outcomes derived from T‐V and P‐V relationship between men and women and between age groups were assessed using linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID). Then, longitudinal changes (i.e., absolute and relative change scores as dependent variable) were assessed with linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID). Maximum likelihood estimation and the best‐fitting covariance structure were applied. Pairwise comparisons were corrected by the Bonferroni method. For reasons of clarity and readability, the results reported in the text refer to longitudinal absolute changes and are reported as overall changes over time (i.e., all participants merged), main effect of sex (i.e., all age groups merged), main effect of age (i.e., men and women merged), sex‐by‐age interaction and subgroup comparisons (i.e., classified by sex and age). Tables 2, 3, 4 also include longitudinal percentage changes and subgroup comparisons. In addition, to assess the influence of changes in protein intake and physical activity behaviour on changes in the outcomes derived from the T‐V and P‐V relationships, the above‐mentioned analyses were adjusted by changes in protein intake and physical activity behaviour. Finally, regression analyses (2nd order polynomials) were used to represent the continuum of longitudinal percentage changes as a function of baseline age. All statistical analyses were carried out with SPSS, version 24 (SPSS Inc., USA), and the level of significance was set at α = 0.05.

Table 2.

Changes in parameters obtained from the torque‐velocity relationship.

	Women			Men
	Young	Middle‐aged	Old	Young	Middle‐aged	Old
T0 (Nm)
Pre	159.5 ± 27.9*	153.4 ± 27.7*	115.6 ± 23.7* ab	242.7 ± 47.8*	234.0 ± 47.3*	203.6 ± 41.2* ab
Post	154.6 ± 32.0*	138.3 ± 23.8*	103.8 ± 28.6* ab	234.1 ± 41.8*	219.3 ± 39.9*	178.8 ± 35.6* ab
∆ (year−1)	−0.45 ± 3.12	−1.57 ± 2.27	−1.26 ± 1.79*	−0.82 ± 3.88	−1.52 ± 3.75	−2.58 ± 2.60
∆% (year−1)	−0.28 ± 1.96	−1.02 ± 1.48 * , a	−1.09 ± 1.55	−0.34 ± 1.60	−0.65 ± 1.60 *	−1.27 ± 1.28 a
P‐value	0.368	<0.001	0.256	0.022	<0.001	<0.001
V0 (rad·s−1)
Pre	11.6 ± 2.1*	10.6 ± 1.7*	10.2 ± 2.2	12.9 ± 2.8*	12.1 ± 2.9*	10.8 ± 2.0ab
Post	11.8 ± 3.6*	10.5 ± 1.9*, a	9.4 ± 1.2 a	13.3 ± 3.4*	11.1 ± 2.4*, a	9.8 ± 1.7 a
∆ (year−1)	0.03 ± 0.37	−0.01 ± 0.24*	−0.10 ± 0.25	0.03 ± 0.37	−0.11 ± 0.33 * , a	−0.10 ± 0.28
∆% (year−1)	0.26 ± 3.19	−0.09 ± 2.26*	−0.98 ± 2.45	0.23 ± 2.87	−0.91 ± 2.73 * , a	−0.93 ± 2.59
P‐value	0.609	0.740	0.365	0.388	<0.001	0.105
S/T0 (%T0·rad·s−1)
Pre	−11.5 ± 1.8	−12.5 ± 1.8*, a	−13.7 ± 2.8 a	−10.9 ± 1.9	−11.6 ± 2.1*, a	−12.5 ± 2.2 a
Post	−12.0 ± 2.2	−12.7 ± 2.4	−15.3 ± 2.9ab	−11.4 ± 1.9	−12.8 ± 2.6 a	−14.0 ± 2.8ab
∆ (year−1)	−0.05 ± 0.23	−0.03 ± 0.24*	−0.19 ± 0.44	−0.05 ± 0.23	−0.13 ± 0.26 *	−0.16 ± 0.39
∆% (year−1)	−0.43 ± 2.00	−0.24 ± 1.92	−1.39 ± 3.21	−0.46 ± 2.11	−1.12 ± 2.24	−1.28 ± 3.12
P‐value	0.240	0.200	0.028	0.052	<0.001	0.002
a/T0
Pre	2.45 ± 1.35	2.48 ± 1.10	1.94 ± 0.69	2.35 ± 1.45	2.43 ± 1.45	2.49 ± 1.30
Post	2.06 ± 1.10	2.46 ± 0.99	2.00 ± 1.15	2.04 ± 1.30	2.49 ± 1.28	2.32 ± 1.25
∆ (year−1)	−0.04 ± 0.18	<0.01 ± 0.15	0.01 ± 0.07	−0.03 ± 0.17	0.01 ± 0.19	−0.02 ± 0.19
∆% (year−1)	−1.63 ± 7.35	<0.01 ± 6.05	0.52 ± 3.61	−1.28 ± 7.23	0.41 ± 7.82 a	−0.80 ± 7.63
P‐value	0.115	0.944	0.904	0.110	0.659	0.583

Note: Linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID) were performed and reported. The P‐value in the table represents the time effect within each subgroup for absolute changes. Bold values indicate a significant change over time (P < 0.05).

Abbreviations: T₀, estimated maximum isometrid torque; V₀, estimated maximum unloaded velocity; S/T₀, linear slope of the torque‐velocity relationship; a/T₀, curvature of the torque‐velocity relationship.

^*Significant differences between men and women (P < 0.05).

^a Significant differences compared with young adults (P < 0.05).

^b Significant differences compared with middle‐aged adults (P < 0.05).

Table 3.

Changes in maximum muscle power, optimal force, and optimal velocity.

	Women			Men
	Young	Middle‐aged	Old	Young	Middle‐aged	Old
Pmax (W)
Pre	358.4 ± 68.2*	319.4 ± 59.9*	228.8 ± 70.6* ab	591.1 ± 131.1*	536.4 ± 122.9*, a	429.9 ± 96.7* ab
Post	338.5 ± 71.5*	286.1 ± 58.1*	178.4 ± 33.8* ab	557.8 ± 114.2*	464.5 ± 102.9*, a	340.6 ± 78.4* ab
∆ (year−1)	−2.02 ± 5.76	−3.48 ± 5.00 *	−5.50 ± 4.90	−3.54 ± 11.38	−7.56 ± 9.56 * , a	−9.47 ± 8.62 a
∆% (year−1)	−0.56 ± 1.61	−1.09 ± 1.57	−2.40 ± 2.14 a	−0.60 ± 1.93	−1.41 ± 1.78 a	−2.20 ± 2.01 a
P‐value	0.117	<0.001	0.053	<0.001	<0.001	<0.001
Topt (Nm)
Pre	69.8 ± 12.5*	67.2 ± 11.7*	49.2 ± 11.1* ab	102.3 ± 19.5*	98.9 ± 20.6*	87.8 ± 18.4* ab
Post	66.0 ± 15.7*	60.3 ± 10.7*	42.6 ± 11.3* ab	95.3 ± 20.0*	92.2 ± 18.9*	75.6 ± 14.7* ab
∆ (year−1)	−0.38 ± 1.40	−0.72 ± 1.18	−0.71 ± 0.73	−0.68 ± 2.09	−0.70 ± 2.04	−1.28 ± 1.38
∆% (year−1)	−0.54 ± 2.01	−1.07 ± 1.76 *	−1.44 ± 1.48	−0.66 ± 2.04	−0.71 ± 2.06 *	−1.46 ± 1.57
P‐value	0.157	<0.001	0.229	<0.001	<0.001	<0.001
Vopt (rad·s−1)
Pre	5.20 ± 0.87*	4.79 ± 0.72*	4.63 ± 0.99	5.84 ± 1.27*	5.51 ± 1.13*	4.96 ± 0.89ab
Post	5.30 ± 1.41*	4.78 ± 0.81*, a	4.29 ± 0.53 a	5.99 ± 1.34*	5.12 ± 1.01*, a	4.54 ± 0.79ab
∆ (year−1)	0.01 ± 0.15	<0.01 ± 0.10*	−0.04 ± 0.11	0.01 ± 0.15	−0.04 ± 0.13 * , a	−0.05 ± 0.12
∆% (year−1)	0.19 ± 2.88	<0.01 ± 2.09*	−0.86 ± 2.38	0.17 ± 2.57	−0.73 ± 2.36 * , a	−1.01 ± 2.42
P‐value	0.646	0.909	0.367	0.367	<0.001	0.082

Note: Linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID) were performed and reported. The P‐value in the table represents the time effect within each subgroup for absolute changes. Bold values indicate a significant change over time (P < 0.05).

Abbreviations: P_max, maximum muscle power; T_opt, optimal torque; V_opt, optimal velocity.

^*Significant differences between men and women (P < 0.05).

^a Significant differences compared with young adults (P < 0.05).

^b Significant differences compared with middle‐aged adults (P < 0.05).

Table 4.

Changes in body mass index, relative T₀ and relative P_max.

	Women			Men
	Young	Middle‐aged	Old	Young	Middle‐aged	Old
BMI (kg·m−2)
Pre	22.4 ± 2.4*	23.3 ± 3.0*	23.1 ± 2.9*	23.9 ± 2.8*	25.4 ± 2.7*, a	26.2 ± 2.8*, a
Post	23.4 ± 2.9*	23.9 ± 3.4*	23.2 ± 3.5*	24.7 ± 3.0*	25.8 ± 3.1*, a	25.9 ± 3.3*
∆ (year−1)	0.10 ± 0.19	0.06 ± 0.16	0.01 ± 0.18	0.08 ± 0.17	0.04 ± 0.15	−0.03 ± 0.13 a
∆% (year−1)	0.45 ± 0.85	0.26 ± 0.69	0.04 ± 0.78	0.33 ± 0.71	0.16 ± 0.59	−0.11 ± 0.50 a
P‐value	<0.001	<0.001	0.878	<0.001	<0.001	0.396
Relative T0 (Nm·kg−1)
Pre	2.62 ± 0.48*	2.43 ± 0.44*	1.94 ± 0.37* ab	3.20 ± 0.58*	2.94 ± 0.53*, a	2.60 ± 0.47* ab
Post	2.41 ± 0.47*	2.14 ± 0.39*, a	1.74 ± 0.39*, a	2.99 ± 0.56*	2.73 ± 0.45*, a	2.32 ± 0.39* ab
∆ (year−1)	−0.02 ± 0.05	−0.03 ± 0.04	−0.02 ± 0.02	−0.02 ± 0.05	−0.02 ± 0.05	−0.03 ± 0.04
∆% (year−1)	−0.76 ± 1.91	−1.23 ± 1.65 *	−1.03 ± 1.03	−0.63 ± 1.56	−0.68 ± 1.70 *	−1.15 ± 1.54
P‐value	0.002	<0.001	0.135	<0.001	<0.001	0.001
Relative Pmax (W·kg−1)
Pre	5.84 ± 0.84*	5.04 ± 0.93*, a	3.85 ± 1.24* ab	7.79 ± 1.54*	6.72 ± 1.24*, a	5.49 ± 1.17* ab
Post	5.28 ± 1.04*	4.43 ± 0.92*, a	3.03 ± 0.65* ab	7.11 ± 1.41*	5.77 ± 1.17*, a	4.44 ± 1.03* ab
∆ (year−1)	−0.06 ± 0.10	−0.06 ± 0.07 *	−0.09 ± 0.08	−0.07 ± 0.13	−0.10 ± 0.11 *	−0.11 ± 0.11
∆% (year−1)	−1.03 ± 1.71	−1.19 ± 1.39	−2.34 ± 2.08	−0.90 ± 1.67	−1.49 ± 1.64 a	−2.00 ± 2.00 a
P‐value	<0.001	<0.001	0.009	<0.001	<0.001	<0.001

Note: Linear mixed‐effect models with two fixed factors (sex and age group) and one random factor (subject ID) were performed and reported. The P‐value in the table represents the time effect within each subgroup for absolute changes. Bold values indicate a significant change over time (P < 0.05).

Abbreviations: BMI, body mass index; T₀, estimated maximum isometric torque; P_max, maximum muscle power.

^*Significant differences between men and women (P < 0.05).

^a Significant differences compared with young adults (P < 0.05).

^b Significant differences compared with middle‐aged adults (P < 0.05).

Results

Table 1 gives an overview of the main characteristics and subgroup comparisons at baseline.

Longitudinal changes in lifestyle factors

Daily protein intake decreased significantly from baseline to follow‐up (mean [95% CI] = −0.01 [−0.016 to −0.004] g·kg⁻¹ per year; P = 0.001), but no effects of sex (P = 0.998), age group (P = 0.508), or sex‐by‐age interaction (P = 0.513) were noted. However, post hoc within‐subgroup analyses showed significant changes only in middle‐aged women (mean [95% CI] = −0.01 [−0.018 to −0.003] g·kg⁻¹ per year; P = 0.007) and old men (mean [95% CI] = −0.02 [−0.035 to −0.003] g·kg⁻¹ per year; P = 0.017). There were no significant differences between subgroups (all P > 0.05). No significant changes were noted in PAL index (mean [95% CI] = −0.002 [−0.006 to 0.001] per year; P = 0.176) or EE_sports (mean [95% CI] = −0.25 [−0.65 to 0.16] MET·h·week⁻¹ per year; P = 0.234), and no main effects of sex (P = 0.911 and 0.331, respectively), age group (P = 0.477 and 0.586, respectively), or sex‐by‐age interaction (P = 0.384 and 0.673, respectively) were found. However, post hoc within‐subgroup analyses showed that PAL index diminished significantly in middle‐aged men (mean [95% CI] = −0.004 [−0.007 to −0.001] per year; P = 0.004), whereas EE_sports did in middle‐aged women (mean [95% CI] = −0.52 [−0.98 to −0.07] MET·h·week⁻¹ per year; P = 0.023). There were no significant differences between subgroups (all P > 0.05). In general, changes in protein intake (all P ≥ 0.133), PAL index (P ≥ 0.100) or EE_sports (all P ≥ 0.372) did not contribute significantly to changes in the T‐V and P‐V relationships over the follow‐up, with a few exceptions that are reported below.

Longitudinal changes in the torque‐velocity and power‐velocity relationships

Individual changes and regression lines observed in the main T‐V and P‐V outcomes after the 10‐year follow‐up in men and women as a function of age are shown in Figure 2.

Figure 2.

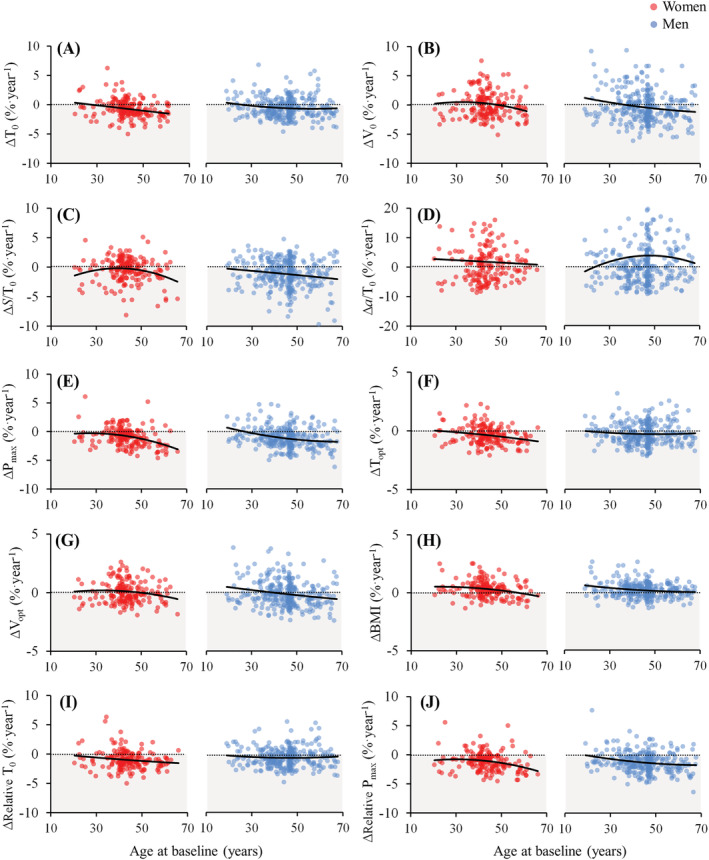
Individual longitudinal annual percentage changes in the study outcomes as a function of baseline age in women and men. Longitudinal changes were calculated as the difference between follow‐up and baseline values divided by follow‐up time in years, and then relative to baseline levels. The dotted lines represent no change (i.e., zero) whereas the solid lines were calculated using regression analyses (2nd order polynomials) to represent the continuum of longitudinal percentage changes as a function of baseline age. ∆, change in a respective outcome; T₀, estimated maximum isometric torque; V₀, estimated maximum unloaded contraction velocity; S/T₀, slope of the linear part of the torque‐velocity relationship; a/T₀, curvature of the hyperbolic part of the torque‐velocity relationship; P_max, maximum muscle power; T_opt, optimal torque; V_opt, optimal velocity; BMI, body mass index.

Knee extension torque‐velocity relationship

The results from each study subgroup and multiple comparisons regarding T₀, V₀, S/T₀, and a/T₀ are provided in Table 2. T₀ decreased significantly over time (mean [95% CI] = −1.37 [−1.85 to −0.88] Nm per year; P < 0.001). There was no main effect of sex (P = 0.268), but there was a main effect of age group (P = 0.027). Specifically, T₀ decreased significantly in middle‐aged women, and in young, middle‐aged, and old men (all P < 0.05). Also, the T₀ decrement was significantly higher in middle‐aged compared with young participants (P = 0.036). No sex‐by‐age interaction was observed (P = 0.541).

Changes in V₀ over time did not reach statistical significance (mean [95% CI] = −0.05 [−0.091 to 0.001] %T₀ rad·s⁻¹ per year; P = 0.057), and no effect of sex was detected (P = 0.452), whereas a significant main effect of age group was observed (P = 0.022). V₀ diminished significantly in middle‐aged men only (P < 0.001). Moreover, the middle‐aged group exhibited a significantly greater decrease in V₀ than the young group (P = 0.030). No sex‐by‐age interaction was observed (P = 0.258).

S/T₀ exhibited a significant decline over time (mean [95% CI] = −0.10 [−0.14 to −0.06] %T₀·rad·s⁻¹ per year; P < 0.001), but no significant main effects of sex (P = 0.501) or age group (P = 0.080) were detected. The decline in S/T₀ was statistically significant among old women, middle‐aged men and old men (all P < 0.05). No sex‐by‐age interaction was observed (P = 0.190).

No significant changes for a/T₀ were found (mean [95% CI] = −0.01 [−0.04 to 0.01] per year; P = 0.312) nor main effects of sex (P = 0.901) or age group (P = 0.872). No sex‐by‐age interaction was observed (P = 0.872). Figure 3 shows the changes observed in the T‐V relationship for each subset of participants.

Figure 3.

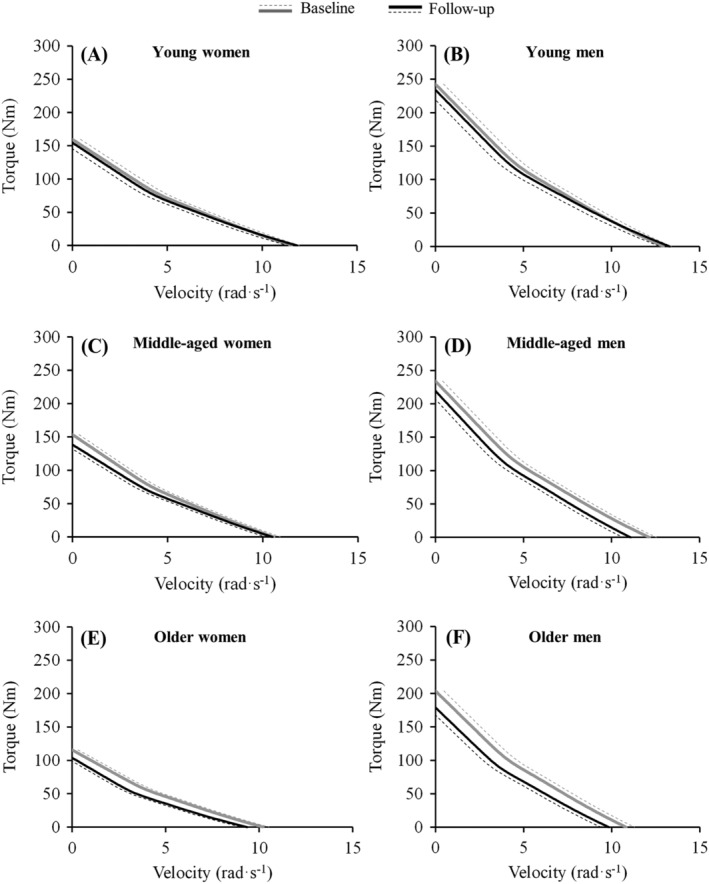
Longitudinal changes observed after the 10‐year follow‐up in the torque‐velocity relationship in young women and men, middle‐aged women and men, and older women and men. The dotted lines represent standard error of the estimate values, and the solid lines represent the hybrid equation used for modelling the torque‐velocity relationship.

Knee extension maximum muscle power

The results from each study subgroup and multiple comparisons regarding P_max, T_opt, and V_opt are provided in Table 3. P_max was found to decrease significantly over time (mean [95% CI] = −5.26 [−6.52 to −4.00] W per year; P < 0.001), whereas significant main effects of sex (P = 0.013) and age group (P = 0.004) were also noted. P_max decreased significantly in middle‐aged women, and in young, middle‐aged, and old men (all P < 0.001). Also, P_max declined more in men compared with women (P = 0.013), and more in middle‐aged and old adults compared with young participants (P = 0.010 and 0.034, respectively). No sex‐by‐age interaction was observed (P = 0.385). Annual changes in PAL index were significantly associated to annual changes in P_max (P = 0.017), so the overall decline in P_max was slightly attenuated in the adjusted model (mean [95% CI] = −5.05 [−6.39 to −3.72] W per year; P < 0.001), but it did not influence comparisons between sex or age groups (Table  S1 ).

In addition, absolute T_opt declined significantly over time (mean [95% CI] = −0.74 [−1.00 to −0.49] Nm per year; P < 0.001), but no main effects of sex (P = 0.273) or age group (P = 0.416) were observed. Specifically, it decreased significantly in middle‐aged women, and in young, middle‐aged and old men (all P < 0.001). No sex‐by‐age interaction was observed (P = 0.540). In terms of T_opt relative to T₀, there was a significant decrement (from 43.0 ± 4.2 to 42.3 ± 4.2% of T₀; mean [95% CI] = −0.11 [−0.21 to −0.02] % of T₀ per year, P < 0.017) with no main effects of sex (P = 0.914) or age group (P = 0.115). No sex‐by‐age interaction was observed (P = 0.883).

Changes in absolute V_opt did not reach statistical significance (mean [95% CI] = −0.02 [−0.036 to 0.001] rad·s⁻¹ per year; P = 0.064), although there was a significant main effect of age group (P = 0.035), but not sex (P = 0.449). V_opt decreased significantly in middle‐aged men only (P < 0.001). Despite the higher V_opt decrements observed in middle‐aged compared with young adults, the difference was not statistically significant (P = 0.059). No sex‐by‐age interaction was observed (P = 0.265). Furthermore, V_opt as a percentage of V₀ increased significantly over time (from 45.5 ± 1.6 to 45.9 ± 1.8% of V₀; mean [95% CI] = 0.04 [0.001 to 0.070] % of V₀ per year; P = 0.043), although no significant main effects of sex (P = 0.825) or age group (P = 0.129) were reported. Figure 4 shows the changes observed in the P‐V relationship for each subset of participants. No sex‐by‐age interaction was observed (P = 0.522).

Figure 4.

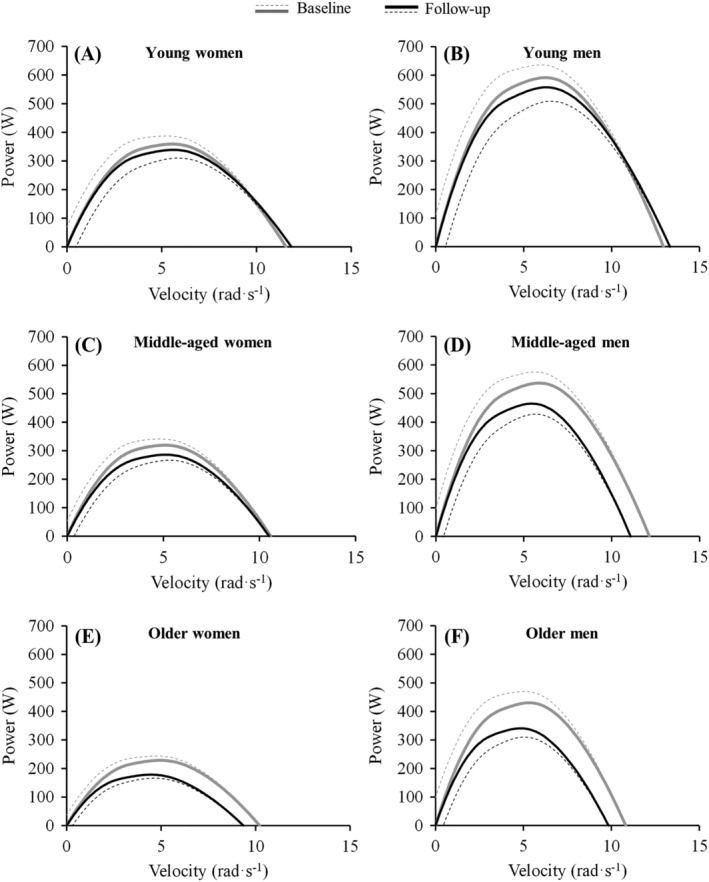
Longitudinal changes observed after the 10‐year follow‐up in the power‐velocity relationship in young women and men, middle‐aged women and men, and older women and men. The dotted lines represent standard error of the estimate values, and the solid lines represent the hybrid equation used for modelling the torque‐velocity relationship that resulted in the power‐velocity relationship.

Longitudinal changes in body mass index and muscle function measures relative to body mass

The results from each study subgroup and multiple comparisons regarding BMI, relative T₀, and relative P_max are provided in Table 4. There was a significant increase in BMI (mean [95% CI] = 0.04 [0.02 to 0.07] kg·m⁻² per year; P < 0.001), and a significant main effect of age group (P = 0.010), but not sex (P = 0.263). BMI increased significantly in young and middle‐aged women and men (all P < 0.001). The young group experienced a significant increase in BMI compared with a non‐significant decline in the old group (P = 0.016). Annual changes in protein intake were significantly associated to annual changes in BMI (P < 0.001), although with no apparent variation in the overall increase in BMI in the adjusted model (mean [95% CI] = 0.04 [0.01 to 0.06] kg·m⁻² per year; P < 0.001) nor in comparisons between sex or age groups (Table  S2 ).

Both relative T₀ and relative P_max decreased significantly (mean [95% CI] = −0.02 [−0.03 to −0.02] Nm·kg⁻¹ per year, and −0.08 [−0.10 to −0.07] W·kg⁻¹ per year; both P < 0.001), but no effects of sex (P = 0.972 and 0.133, respectively) or age group (P = 0.492 and 0.147, respectively) were noted. Finally, the declines were statistically significant for relative T₀ in young and middle‐aged women, and in young, middle‐aged, and old men; whereas regarding relative P_max, the declines were statistically significant for all the subgroups (all P < 0.01). No sex‐by‐age interaction was observed (P = 0.597). Annual changes in protein intake were significantly associated to annual changes in relative P_max (P = 0.010), but the overall decline in relative P_max remained the same in the adjusted model (mean [95% CI] = −0.08 [−0.10 to −0.07] W·kg⁻¹ per year; P < 0.001) and did not influence comparisons by sex or age groups (Table  S2 ).

Discussion

The main findings of the present longitudinal investigation were that after 10 years, P_max decreased at different rates in young (−0.6%·year⁻¹) compared with middle‐aged (−1.1% to −1.4%·year⁻¹) and older (−2.2% to −2.4%·year⁻¹) adults. These changes were in general accompanied by decreases in T_opt and V_opt. Due to concomitant changes in BMI, relative P_max was found to decline in young (−0.9% to −1.0%·year⁻¹), middle‐aged (−1.2% to −1.5%·year⁻¹), and older (−2.0% to −2.3%·year⁻¹) adults. Of note, an overall longitudinal decline in protein intake was detected, whereas physical activity and sports participation remained unchanged in the whole cohort of participants. Changes in physical activity were associated to changes in absolute P_max, whereas changes in protein intake were associated to changes in BMI and relative P_max.

Early cross‐sectional studies demonstrated that older people show a downward shift in the F‐V relationship (i.e., lower force production at given movement velocities, lower maximal isometric force, and lower maximal unloaded contraction velocity) ³³ , ³⁴ , ³⁵ when compared with their younger counterparts. Of note, muscle power is the product of force and velocity, so changes in the F‐V relationship impact muscle power output severely. In addition, age‐related percentage decrements in the F‐V relationship seem to be greater at higher versus lower contraction velocities, ⁶ , ³⁶ , ³⁷ , ³⁸ , ³⁹ and so dynamic muscle function is more affected by aging than isometric muscle function. ³⁷ , ⁴⁰ By analysing changes in the slope of the T‐V relationship relative to maximum isometric torque (i.e., S/T₀), we were able to examine how torque decreases when movement velocity increases. Our results show that (i) torque decreased as a function of velocity more abruptly in middle‐aged and older adults compared with young adults and (ii) significant longitudinal decreases were observed in older women and in middle‐aged and older men. Basically, these results confirm that middle‐aged and especially older people exhibit a more compromised muscle function with aging as the required movement velocity increases. Another study has also shown that older people exhibit a lower resistance to fatigue compared with young people during fast muscle actions, ³⁸ which would impair even more their ability to conduct repeated fast dynamic actions. Therefore, as previously noted, ⁴¹ the inclusion of explosive exercises within resistance training programmes might be paramount for the prevention and treatment of this hallmark of aging.

Regarding the potential mechanisms leading to these changes, one strong candidate might be the loss of skeletal muscle. Nevertheless, muscle force is less dependent on muscle size as muscle contraction velocity increases, ^{38 , 42} and no significant longitudinal changes in whole‐body skeletal muscle mass assessed by bioelectrical impedance analysis have been previously reported for the present cohort of participants. ²² Although skeletal muscle mass was diminished by ~3% after the 10‐year follow‐up in the older participants (i.e., ~0.3% per year), other factors should be considered to explain the observed 21–23% decrease in P_max. For example, lower overall single muscle fibre ATPase activity ⁴³ or a fast‐to‐slow shift in the single muscle fibre phenotype ⁴⁴ with aging would fit with the observed changes. In addition, an age‐related decrease in tendon stiffness and skeletal muscle fascicle length would cause a decrease in torque at given velocities, especially during fast contractions. ^45,46 Also, considering the time constraints inherent to fast contractions, a slower ability to maximally evoke voluntary contractions, convey action potentials through motor nerves and translate the electrical signal into a mechanical signal (excitation‐contraction coupling) might explain a greater decline in high‐ versus low‐velocity actions. A possible disproportionate coactivation of the antagonist muscles during fast contractions, or impaired muscle gearing, may also account for the greater decrements observed during fast versus slow muscle actions. Among these hypotheses, specific atrophy and loss of type II fibres, ⁴⁷ reduced shortening velocity within fibre types due to slowed rates of myosin head detachment, ⁴⁸ muscle fascicles operating in a faster region of the F‐V relationship for a given external velocity, ⁴⁹ reduced muscle fibre conduction velocity, ⁵⁰ decreased tendon stiffness ⁵¹ and muscle fascicle length, ⁵² and lower rate of EMG rise ⁵³ and decreased neural drive during the early phase of the muscle contraction ⁵⁴ have been found to be associated with aging in previous studies. The assessment of the potential role of the other proposed factors and the finding of molecular markers ^55,56 might be of relevance for future studies.

Apart from the changes in absolute P_max values, we noted a significant decline in relative P_max at all age and sex groups. These declines in relative P_max values apparently exceeded the decline in absolute P_max values in younger individuals, whereas they were slightly lower in older adults. This results from concomitant changes in BMI occurring during aging. Of note, BMI was found to augment in the young and middle‐aged participants (0.3–0.5% and 0.2–0.3%·year⁻¹, respectively), whereas no significant changes were reported in our 60‐ to 70‐year‐old adults. This matches with a previous cross‐sectional study showing an increase in BMI up to the age of 65 years in men and 75 years in women, after which it starts to decline. ¹⁰ Likewise, sex differences in changes in BMI have been confirmed in a previous 10‐year longitudinal follow‐up study, ⁵⁷ and the current data show that older women and men in their 60s increased (0.04% year⁻¹, not significant) and decreased (−0.1% year⁻¹, not significant) in BMI, respectively. Important to note is that the potential loss of BMI at advancing age cannot compensate the progressively larger loss of muscle power as people age, which can reach up to 4–9%·year⁻¹ after the eighth decade of life. ^{10 , 58} As low relative muscle power is more strongly associated with several negative outcomes occurring at older age (e.g., mobility limitations, hospitalization, or mortality) than sarcopenia, low handgrip strength, or absolute muscle power per se, ⁶ , ⁸ , ⁹ , ^{14 , 59–61} it should be evaluated and diagnosed in older people according to a proposed operational algorithm and cut‐off points. ⁶²

The temporal sequence of the declines in the different parameters can be viewed in Figure 2, that is, by investigating when the regression lines crossed the zero line and changes became negative. Maximum isometric torque (i.e., T₀) started to decline after the age of 27 years in men and 28 years in women, whereas P_max declined after the age of 27 years in men and 20 years in women. Although longitudinal changes in power were greater than those found in maximum isometric torque, both started approximately in the same period in life. In addition, torque produced at P_max (i.e., T_opt) started to decline after the age of 20 years in men and 22 years in women, whereas velocity at P_max (i.e., V_opt) decreased after the age of 39 years in men and 49 years in women. This denotes that early decrements in P_max are associated with mechanisms directly related to torque production, whereas in middle‐aged and older adults, both torque‐ and velocity‐related mechanisms are associated with the age‐related loss of P_max. A previous cross‐sectional study also reported that the main determinant of the lower maximum power observed in older versus young women was a decreased optimal force, whereas optimal velocity was reduced to a lesser extent. ⁶³ In any case, the great interindividual variability observed in our study indicates that evaluation and individualization should be the preferred choice to establish a correct diagnosis and treatment for each individual.

Finally, there are several considerations and limitations that deserve to be mentioned to interpret adequately the current findings. Firstly, the present study assessed knee extension muscle function, so the findings can only apply for this muscle action. Secondly, in some subgroups, there were changes in physical activity level, sports energy expenditure, and protein intake over the 10‐year follow‐up period, which can influence the changes in muscle function. Although we have performed additional analyses to adjust for those variables, we should be aware that these measurements only address two specific points in the 10‐year period (i.e., baseline and follow‐up) and might not represent what occurred during follow‐up period. In addition, there might be other covariates that may have influenced our results, such as co‐morbidity. Although we registered some disease‐related data for the exclusion criteria and risk stratification for a maximal graded exercise test within the overall study protocol, these were not collected in sufficient detail to include co‐morbidity as a covariate in the study. Thirdly, the results derived from older women are based on a relatively small group of participants, which may explain the lack of statistical significance in some outcomes. Finally, longitudinal studies are limited by a potential survival bias, and thus, our finding may underestimate the actual changes occurring during aging in the whole set of participants evaluated at baseline.

Conclusions

Maximum muscle power of the knee extensors declined significantly after a period of 10 years in young men, middle‐aged women and men, and older women and men. The early declines in muscle power seemed to coincide with declines in force production, whereas the progressive decline in muscle power was associated with declines in both force and velocity production. The analysis of the torque‐velocity relationship also showed a progressively blunted ability to produce force at moderate‐to‐high movement velocities (i.e., maximum muscle power) as a specific hallmark of aging.

Conflict of interest

The authors declare no conflicts of interest.

Funding

This study was conducted under the authority of the First and Third waves of the Flemish Policy Research Center on Sport and was funded by the Flemish Government. E. Van Roie was supported by the Research Foundation Flanders, Belgium (senior postdoctoral fellowship 12Z5720N). J. Alcazar was supported by the Biomedical Research Networking Center on Frailty and Healthy Aging (CIBERFES) and FEDER funds from the European Union (CB16/10/00477). C. Rodriguez‐Lopez was supported by the Ministerio de Economía y Competitividad of the Government of Spain (DEP2015‐69386‐R and BES‐2016‐077199).

Supporting information

Data S1. Supporting Information

Table S1. Changes in maximum muscle power adjusted by changes in PAL index.

Table S2. Changes in body mass index and relative P_max adjusted by changes in daily protein intake.

Alcazar J., Rodriguez‐Lopez C., Delecluse C., Thomis M., and Van Roie E. (2023) Ten‐year longitudinal changes in muscle power, force, and velocity in young, middle‐aged, and older adults, Journal of Cachexia, Sarcopenia and Muscle, 14, 1019–1032, 10.1002/jcsm.13184