Abstract
Transition-metal-doped boron nanoclusters exhibit unique structures and bonding in chemistry. Using the experimentally observed seashell-like borospherenes C2 B28−/0 and Cs B29− as ligands and based on extensive first-principles theory calculations, we predict herein a series of novel transition-metal-centered endohedral seashell-like metallo-borospherenes C2 Sc@B28− (1), C2 Ti@B28 (2), C2 V@B28+ (3), and Cs V@B292− (4) which, as the global minima of the complex systems, turn out to be the boron analogues of dibenzenechromium D6h Cr(C6H6)2 with two B12 ligands on the top and bottom interconnected by four or five corner boron atoms on the waist and one transition-metal “pearl” sandwiched at the center in between. Detailed molecular orbital, adaptive natural density partitioning (AdNDP), and iso−chemical shielding surface (ICSS) analyses indicate that, similar to Cr(C6H6)2, these endohedral seashell-like complexes follow the 18-electron rule in bonding patterns (1S21P61D10), rendering spherical aromaticity and extra stability to the systems.
Keywords: first-principles theory, seashell-like metallo-borospherenes, structures, bonding, spherical aromaticity
1. Introduction
Extensive joint photoelectron (PE) spectroscopy and first-principles theory investigations in the past two decades have unveiled a great structural diversity in boron nanoclusters featuring multi-center-two-electron (mc-2e, m ≥ 3) bonding, including the planar or quasi-planar (2D) Bn−/0 (n = 3–38, 41, 42) [1,2,3], cage-like D2d B40−/0 and C3/C2 B39− [4,5], and bilayer D2h B48−/0[6], with the smallest seashell-like C2 B28−/0 [7] and Cs B29− [8] observed in gas phases competing with their 2D counterparts in experiments. Based on the experimentally observed cage-like B40−/0 and B39−, the borospherene family have been extended to the Bnq series (n = 36−42, q = n − 40) in theory [9,10,11]. Theoretical investigations have shown that metal-decorated seashell-like B28 may serve as effective potential hydrogen storage materials [12]. The first theoretically predicted perfect cage-like B80 in 2007 [13,14] spurred renewed interest in all-boron fullerenes although it was later proved to favor core–shell structures. The bilayer structural motif observed in B48−/0 has been extended to B48-B72 and B84–B98 at the density functional theory (DFT) level, with a bilayer bottom-up approach based on the experimentally observed C6v B36 proposed for the observed bilayer BL-α+ borophenes on Ag (111) [15,16,17,18,19]. Mononuclear core–shell B68, B74, B80, B84, B96, B100, B101, B102, and B112 and binuclear core–shell Cs B180 ((B12)2@B156), Cs B182 ((B12)2@B158), and Cs B184 ((B12)2@B160) with two interconnected icosahedral B12 cores at the center have also been predicted at DFT, with Cs B112 and Cs B184 as the most stable mononuclear and binuclear species reported to date in thermodynamics [20,21,22,23,24,25,26,27], respectively. Transition-metal doping proves to induce dramatic structural changes in boron nanoclusters. Perfect transition-metal-centered wheel-like D8h Co©B8−, D9h M©B9−(M = Rh, Ir, Re), and D10h M©B10−(M = Ta, Nb), [28,29,30] half-sandwich C3v CoB12− and IrB12−, and double–ring tubular drum-like D8d CoB16−, MnB16−, and RhB18−, Cs B2–Ta@B18−, and D10d Ta@B20− have been observed in experiments [31,32,33,34], with Ta@B20− possessing the highest coordination number of CN = 20 in tubular species [35]. Perfect lanthanide-metal-doped inverse sandwich Dnh La2Bn− (n = 7–9) and spherical trihedral metallo-borospherenes La3B18− and Tb3B18− have also been reported in experiments [36,37]. With inspirations from these experimental observations, our group predicted the smallest core–shell spherical trihedral metallo-boronospherene D3h La3[B2@B18]−, perfect spherically aromatic tetrahedral metallo-borospherenes Td La4B24 and core–shell Td La4B290/+/− (La4[B@B4@B24]0/+/−), endohedral metallo-borospherenes Oh La6&[La@B24]+/0, and the spherically aromatic trihedral metallo-borospherene D3h La6B30 in a series of recent papers [38,39,40,41]. Spherical trihedral metallo-borospherenes and endohedral Complexes of B20TMn (TM = Sc, Y; n = 3, 4) were predicted recently [42]. The Ta-centered metallo-borospherenes Ta@B22− and Ta@Bnq (n = 23–28, q = −1–3) which follow the 18-electron rule, the smallest trihedral metallo-borospherene D3h Ta3B12− with three equivalent octacoordinate Ta centers in three η8–B8 rings, and spherical tetrahedral metallo–borospherene Td Ta4B18 with four equivalent nonacoordinate Ta centers in four η9–B9 rings conforming to the 18-electron principle were proposed recently [43,44,45,46]. Alkaline-earth-metal-centered M@B40 (M = Ca, Sr) [47] and actinide-metal-centered U@B40 [48] were also predicted in theory. However, to the best of our knowledge, spherically aromatic transition-metal-centered endohedral metallo-borospherenes based on the experimentally observed seashell-like C2 B28−/0 and Cs B29− as the global minima (GM) of the systems have not been reported in the literature.
As boron analogues of benzene (D6h C6H6), the experimentally observed quasi-planar C3v B12 with three delocalized π bonds was first utilized as ligands to form the perfect sandwich-like complex D3d Cr(B12)2 [49,50]. Unfortunately, such a manually designed complex appears to be a high-lying local minimum of the system unlikely to be produced in experiments. Using the experimentally observed smallest seashell-like borospherenes C2 B28−/0 and Cs B29− as ligands which contain two B12 ligands on the top and bottom interconnected by four or five corner boron atoms on the waist and based on extensive GM searches augmented with first-principles theory calculations, we predict in this work a series of transition-metal-centered seashell-like metallo-borospherenes C2 Sc@B28− (1), C2 Ti@B28 (2), C2 V@B28+ (3), and Cs V@B292−(4) which, as the GMs of the systems with two interconnected B12 ligands on the top and bottom and one transition metal center as the “pearl” sandwiched in between, follow the 18-electron rule in bonding patterns, making the transition-metal-doped boron complexes spherically aromatic in nature, highly stable in both thermodynamics and dynamics and possible to be targeted in future experiments.
2. Results and Discussions
2.1. Structures and Stabilities
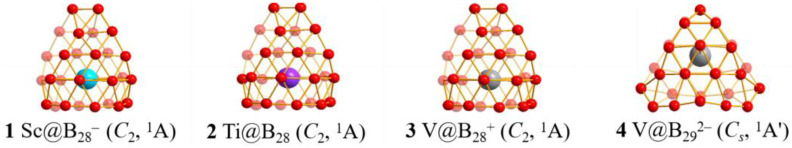
The obtained transition-metal-centered seashell-like metallo-borospherenes C2 Sc@B28− (1), C2 Ti@B28 (2), C2 V@B28+ (3), and Cs V@B292− (4) as the GMs of the systems at PBE0/6-311+G(d) [51], TPSSh/6-311+G(d) [52,53], and CCSD(T)/6-31G(d) [54,55] levels are collectively depicted in Figure 1, with more alternative low-lying isomers summarized in Figures S1–S4 (ESI†). The isovalent Sc@B28− (1), Ti@B28 (2), and V@B28+ (3) with the calculated coordination energies of Ec = 9.56, 7.83, 7.57 eV and lowest calculated vibration frequencies of 181.13, 186.63, 184.70 cm−1 at PBE0, respectively, turn out to have similar seashell-like structures in the same symmetry as their parent C2 B28 ligand [7], with two B12 ligands on the top and bottom interconnected by four corner boron atoms on the waist and one transition metal pearl comfortably sandwiched in between. These axially chiral endohedral metallo-borospherene complexes contain a slightly distorted C2 B16 double-ring tube as the basis of the seashell-like structures, two heptagonal windows on the right and left, and thirty-six B3 triangles on the cage surface, with a transition metal center sandwiched comfortably inside the B28 cage along the C2 molecular axis on the upper end of the B16 double-ring tube (see detailed coordination bond lengths tabulated in Table S1). C2 Sc@B28− (1), Ti@B28 (2), V@B28+ (3) possess the large calculated HOMO-LUMO energy gaps of ΔEgap = 2.10, 2.97, and 3.20 eV at PBE0, respectively, well supporting their high chemical stabilities. It is noticed that the second isomer C2 Sc&B28− (1b) in Figure S1, an exohedral metallo-borospherene with an octacoordinate Sc atom at the lower end of the B16 double-ring tube, is actually iso-energetic with Sc@B28− (1) at CCSD(T), suggesting that the two degenerate C2 isomers may coexist in experiments, while, as shown in Figures S2 and S3, the endohedral Ti@B28 (2) and V@B28+ (3) are 0.18 eV and 0.04 eV more stable than their second lowest-lying isomers at CCSD(T), respectively. Triplet and quintet isomers prove to be at least 0.85 eV less stable than their singlet GMs.
Figure 1.
Optimized structures of the transition metal-doped seashell-like endohedral metallo-borospherenes C2 Sc@B28−(1), C2 Ti@B28 (2), C2 V@B28+ (3), and Cs V@B292− (4) at PBE0/6-311+G(d) level.
The optimized V-centered Cs V@B292− (4) also possesses a seashell-like endohedral structure in the same symmetry as its parent ligand Cs B29− [8]. It contains two B12 ligands on the top and bottom interconnected by five corner boron atoms on the waist, two equivalent octagonal windows on the right and left sides, and thirty-eight B3 triangles on the cage surface, with a vanadium center coordinated inside. With a large calculated HOMO-LUOM energy gap of ΔEgap = 2.39 eV, coordination energy of Ec = 4.79 eV and one small imagery vibrational frequency at −54.30 cm−1, Cs V@B292−(4) appears to be the vibrationally averaged GM of the system between two slightly distorted C1 V@B292− isomers (4b in Figure S4) in an a″ vibrational mode in which the top B atom and V center swinging left and right reversibly. With zero-point corrections included, Cs V@B292−(4) turns out to be 0.02 eV and 0.06 eV more stable than the second seashell-like isomer C1 V@B292− (4b) and third tubular isomer Cs V@B292− (4c) at CCSD(T), respectively (Figure S4). Triplet and quintet isomers are found to be 0.74 eV and 1.81 eV less stable than singlet Cs V@B292−(4) at PBE0 level, respectively, and all the other isomers lying at least 0.15 eV higher than the Cs GM (4).
Detailed natural bonding orbital (NBO) [56] analyses indicate that transition metal centers in Sc@B28− (1), Ti@B28 (2), V@B28+ (3), and V@B292− (4) possess the net atomic charges 0.76, 0.36, -0.33, and -0.37 |e|, electronic configurations of Sc ([Ar]4s0.193d1.42), Ti ([Ar]4s0.213d2.02), V ([Ar]4s0.223d4.26), and V ([Ar]4s0.203d4.48), and total Wiberger bond orders of 4.03, 6.02, 6.70, and 6.44, respectively. Obviously, transition metal coordination centers in these complexes donate their 4s2 electrons almost completely to the boron ligands, while in return, accept partial electrons in their partially filled 4d orbitals from the boron ligands via effective π→3d back-donations, enhancing the thermodynamical stabilities of systems.
Extensive Born–Oppenheimer molecular dynamics (BOMD) [57] simulations on Sc@B28− (1) at 600 K, Ti@B28 (2) at 700 K, and V@B292− (4) at 700 K in Figure S5 clearly indicate that these seashell-like transition metal boron complexes are highly dynamically stable at high temperatures, as evidenced by their small calculated root-mean-square-deviations of RMSD = 0.09, 0.10, 0.10 Å and maximum bond length deviations of MAXD = 0.30, 0.32, 0.33 Å, respectively. No high-lying isomers were observed during the simulations in 30 ps, with the basic structural motifs of the complex systems well maintained in reversible thermal vibrations.
2.2. Bonding Pattern Analyses
To better comprehend the high stabilities of these seashell-like endohedral complexes, detailed adaptive natural density partitioning (AdNDP) [58,59] bonding analyses are performed on Ti@B28 (2) and V@B292− (4) in Figure 2, in comparison with that of the prototypic sandwich complex D6h (C6H6)2Cr. As indicated in Figure 2a, D6h (C6H6)2Cr possesses 12 2c-2e C-C σ bonds and 12 2c–2e C–H σ bonds on the two C6H6 ligands with the occupation numbers ON = 1.95 |e|. Its remaining nine delocalized coordination bonds include 3 7c–2e C6 (π)–Cr (dπ/σ) bonds between the Cr center and C6H6 ligand on the top, 3 7c-2e C6 (π)–Cr (dπ/σ) bonds between the Cr center and C6H6 ligand at the bottom, and 3 13c C6 (π)–Cr (dπ/σ)–C6 (π) bonds between Cr center and the two C6H6 ligands with ON = 1.93~2.00 |e|, well demonstrating that D6h (C6H6)2Cr satisfies the 18-electron rule.
Figure 2.
AdNDP bonding patterns of (a) D6h Cr(C6H6)2, (b) C2 Ti@B28 (2), and (c) Cs V@B292− (4), with the occupation numbers (ON) indicated.
Detailed AdNDP analyses presented in Figure 2b indicate that neutral seashell-like C2 Ti@B28 (2) contains 34 3c–2e σ bonds on 34 B3 triangles on the cage surface and 1 4c–2e σ bond shared by two edge-sharing B3 triangles on the upper end, forming the σ-framework of the seashell-like complex. Its remaining nine delocalized coordination bonds include three 13c–2e B12 (π)–Ti (dπ/σ) bonds between the Ti center and B12 ligand on the top, three 13c–2e B12 (π)–Ti (dπ/σ) between the Ti center and B12 ligand at the bottom, and three 27c–2e B13 (π)–Ti (dπ/σ)–B13 (π) bonds mainly between Ti and its two B12 ligands on the top and bottom with ON = 1.88~2.00 |e|. Such a delocalized coordination bonding pattern possesses a one-to-one correspondence relationship with that of D6h (C6H6)2Cr in Figure 2a, indicating that, similar to (C6H6)2Cr, Ti@B28 (2) follows the 18-electron principle in coordination bonding pattern. Both the isovalent C2 Sc@B28− (1) and C2 V@B28+ (3) are found to follow similar bonding patterns (Figure S6).
Cs V@B292− (4) appears to possess a similar bonding pattern. As shown in Figure 2c, it has 38 3c–2e σ bonds on 38 B3 triangles on the cage surface, forming the σ-framework of the B29− ligand. The remaining nine delocalized coordination bonds include three 13c–2e B12 (π)–V(dπ/σ) bonds between the V center and B12 ligand on the top, three 13c–2e B12 (π)–V (dπ/σ) between the V center and B12 ligand at the bottom, and three 27c–2e B14 (π)–V (dπ/σ)–B12 (π) bonds mainly between V and its two B12 ligands on the top and bottom with ON = 1.91~1.99 |e|, again well corresponding to bonding pattern of D6h (C6H6)2Cr in Figure 2a, showing that V@B292− (4) also matches the 18-electron rule in coordination bonding pattern.
The eigenvalue spectra of D6h (C6H6)2Cr, C2 Ti@B28 (2), and Cs V@B292− (4) compared in Figure S7 indicate that these transition metal-centered complexes possess nine delocalized atomic-like canonical molecular orbitals (CMOs) in the pseudo-superatomic [60] electronic configuration of 1S21P61D10 via effective spd-π interaction/hybridizations, indicating that they follow the 18-electron principle and match the 2(n + 1)2 electron counting rule (n = 2), making them spherically aromatic in nature and chemically stable both thermodynamically and dynamically.
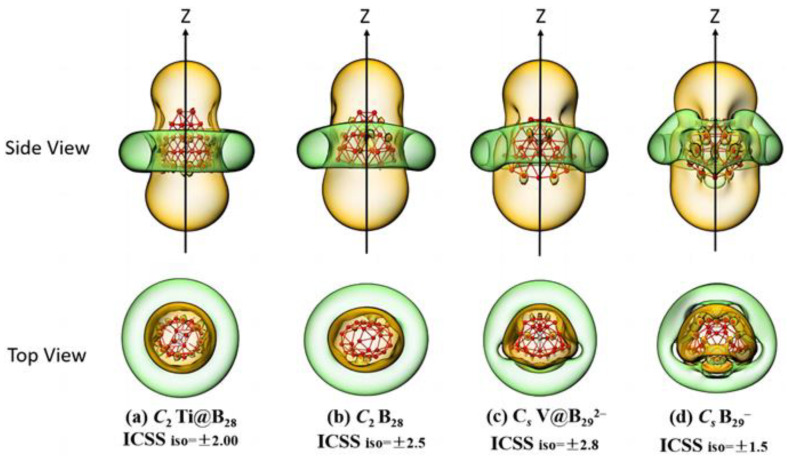
The calculated iso-chemical shielding surfaces (ICSSs) [61] of Ti@B28 (2) and V@B292− (4) based on the ZZ components of the calculated nuclear-independent chemical shifts (NICS-ZZ) shown in Figure 3a,c appear to be similar with that of the experimentally known spherically aromatic C2 B28 (Figure 3b) [7] and Cs B29− (Figure 3d) [8], respectively, well supporting the spherical aromaticity of these endohedral seashell-like endohedral complexes. The spaces inside the boron cage or within 1 Å above the cage surface in vertical directions with negative NICS–ZZ values belong to chemical shielding regions (highlighted in yellow), while the belt-like region outside the cage in the horizontal direction around the waist belongs to the chemical de-shielding area (highlighted in green).
Figure 3.
Calculated iso−chemical shielding surfaces (ICSSs) of (a) C2 Ti@B28 (2) and (c) Cs V@B292− (4), compared with that of the experimentally known spherically aromatic (b) C2 B28 and (d) Cs B29−, respectively.
2.3. IR, Raman, and PE Spectral Simulations
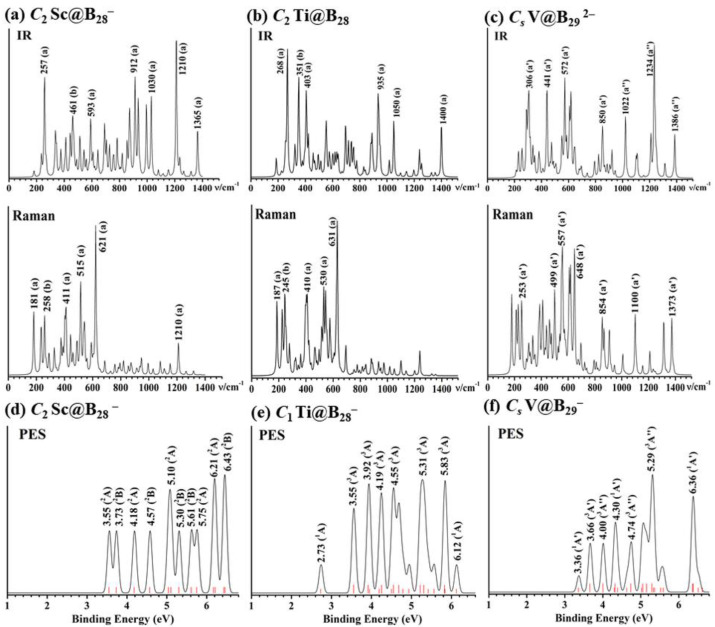
Joint experimental spectroscopic and first-principles theory investigations have proven to be the most effective method to characterize gas phase clusters [62]. The infrared (IR) and Raman spectra of C2 Sc@B28− (1), C2 Ti@B28 (2), and Cs V@B292− (3) are simulated at PBE0/6-311+G(d) in Figure 4 to facilitate their future spectroscopic characterizations. As shown in Figure 4a, C2 Sc@B28− (1) exhibits strong IR active peaks at 257 (a), 461 (b), 593 (a), 872 (a), 912 (a), 936 (b), 1030 (a), 1210 (a), and 1365 (a) cm−1 which mainly belong to the vibrational modes of the B28 skeleton, while its strong Raman active vibrations occur at 181 (a), 258 (b), 411 (a), 515 (a), 621 (a), 1210 cm−1 (a), with the 411 cm−1 (a) peak corresponding to typical “radial breathing mode” (RBM) [63] of the C2 B28 ligand which can be used to characterize hollow boron nanostructures. The IR and Raman spectra of Ti@B28 (Figure 4b) is similar to that of Sc@B28−, with the IR active vibrational modes at 268 (a), 351 (b), 403 (a), 935 (a), 1050 (a), and 1400 (a) and Raman active vibrations at 187 (a), 245 (b), 410 (a), 530 (a), and 631(a) cm−1, respectively, with the 530 cm−1 (a) peak belonging to typical RBM. The strong IR peaks of V@B292− (4) occur at 306 (a′), 441 (a′), 572 (a′), 850 (a′), 1022 (a″), 1234 (a″), and 1386 (a″), while its Raman features are located at 253 (a′), 499 (a′), 557 (a′), 648 (a′), 854 (a″), 1100 (a′), and 1373 (a″) (Figure 4c). Simulated IR and Raman spectra of (a) C2 V@B28+ are shown in Figure S8.
Figure 4.
Simulated IR and Raman spectra of (a) C2 Sc@B28− (1), (b) C2 Ti@B28 (2), and (c) Cs V@B292− (4) and PE spectra of (d) C2 Sc@B28−, (e) C1 Ti@B28−, and (f) Cs V@B29− at PBE0/6-311+G(d) level. The red bars in (d), (e,f) stand for the positions of calculated PE features, with the long and short red bars in (e,f) representing triplet and singlet final states in the neutrals, respectively.
The simulated PE spectra of C2 Sc@B28− (1) and C1 Ti@B28− and Cs V@B29 − derived from C2 Ti@B28 (2) and Cs V@B292− (4) are shown in Figure 4d–f using the time-dependent TD-PBE0/6-311+G(d) approach [64,65], with their first calculated vertical detachment energies (VDEs) located at 3.55, 2.73, and 3.36 eV and first adiabatic detachment energies (ADEs) located at 3.33, 2.41, and 3.21 eV, respectively. Detachment of one electron from singlet C2 Sc@B28− (1) leads to doublet final states in its neutral, with the major spectroscopic features at 3.55, 3.73, 4.18, 4.57, 5.10, 5.30, 5.61, 5.75, 6.21, and 6.43 eV, respectively (Figure 4d). Detachment of one electron from the open-shell doublet C1 Ti@B28− and Cs V@B29− generates both singlet or triplet final states in their neutrals, with the major spectral peaks located at 2.73, 3.55, 3.92, 4.19, 4.55, 5.31, 5.83, and 6.12 eV for Ti@B28− and 3.36 3.66, 4.00, 4.30, 4.74, 5.29, and 6.36 eV for V@B29−, respectively (Figure 4e,f).
3. Computational Details
Extensive GM searches were performed on Sc@B28−, Ti@B28, and V@B28+, V@B292− at DFT level with electronic multiplicities considered, using both the TGmin2 [66,67] and Minima Hopping (MH) [68,69] codes, in conjunction with manual constructions based on the experimentally observed C2 B28−/0 and Cs B29− at PBE/DZVP, with about 3500 stationary points probed for each species on its potential surface. The low-lying isomers were then fully optimized at both PBE0/6-311+G(d) [51] and TPSSh/6-311+G(d) [52,53] levels using the Gaussian 09 program, with vibrational frequencies checked to make sure all the obtained low-lying isomers are true minima of the systems. Single point CCSD(T)/6-31G(d) calculations were performed on the five lowest–lying isomers to further refine their relative energies employing the Molpro (2013) program [54,55], with the T1 diagnostics checked to make sure that multi-reference interactions make non-significant contributions in these closed-shell complexes. Natural bonding orbital (NBO) analyses were carried out using the NBO 6.0 program [56]. Extensive Born–Oppenheimer molecular dynamics (BOMD) simulations were performed on C2 Sc@B28−(1) at 600 K, C2 Ti@B28 (2) at 700 K, and V@B292−(4) at 700 K for 30 ps using the CP2K program [57] utilizing the hybrid Gaussian and plane waves method, with the GTH–PBE pseudopotential and DZVP–MOLOPT–SR–GTH basis set for boron and transition metal, respectively. Detailed bonding analyses were carried out utilizing the adaptive natural density partitioning (AdNDP) approach [58,59]. Iso-chemical shielding surfaces (ICSS) [61] were calculated using the Multiwfn 3.8 software [70]. Bonding analyses and ICSS surfaces were visualized using the visual molecular dynamics (VMD) [71] software. The IR and Raman spectra of C2 Sc@B28− (1), C2 Ti@B28 (2), Cs V@B292− (4) were simulated at PBE0/6-311+G(d). The PE spectra of C2 Sc@B28− (1), C1 Ti@B28− and Cs V@B29− were simulated using the time-dependent DFT approach (TD-DFT) at PBE0/6-311+G(d) level [64,65]. An overall calculation scheme used in this work is presented in Figure S9.
4. Conclusions
Based on the experimentally observed seashell-like C2 B28−/0 and Cs B29− and extensive first-principles theory calculations, we propose in this work the transition-metal-centered endohedral seashell-like metallo-borospherenes Sc@B28− (1), Ti@B28 (2), V@B28+ (3), and V@B292− (4) which, as the boron analogues to the well-known sandwich complex Cr(C2H6)2 highly stable both thermodynamically and dynamically, follow the 18-electron rule in coordination bonding patterns and are spherically aromatic in nature. The IR, Raman, and PE spectra of the concerned species are theoretically simulated to facilitate their future spectroscopic characterizations in gas-phase experiments via laser ablations of boron-transition-metal mixed binary targets. Further combined theoretical and experimental investigations on metal-doped boron complexes are expected to unveil novel structures and bonding in chemistry and materials science and shed new insights on boron-based nano-devices.
Supplementary Materials
The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28093892/s1, Figure S1: Low-lying isomers of C2 Sc@B28− with their relative energies; Figure S2: Low-lying isomers of C2 Ti@B28 with their relative energies; Figure S3: Low-lying isomers of C2 V@B28+ with their relative energies; Figure S4: Low-lying isomers of Cs V@B292− with their relative energies; Figure S5: Molecular dynamics simulations of (a) Sc@B28− (1) at 600 K, (b) Ti@B28 (2) at 700 K, and (c) V@B292− (4) at 700 K; Figure S6: AdNDP Analysis of (a) C2 Sc@B28− and (b) C2 V@B28+; Figure S7: Molecular orbital energy levels of (a) D6h (C6 H6)2Cr, (b) C2 Ti@B28 and (c) Cs V@B292−; Figure S8: Simulated IR and Raman spectra of (a) C2 V@B28+; Figure S9: An overall scheme of the theoretical procedures adapted in this work. Table S1: The bond lengths rSc-B of C2 Sc@B28−, rTi-B of C2 Ti@B28, rV-B of C2 V@B28 and r’V-B of Cs V@B292−; Table S2: Cartesian coordinates of the optimized low-lying isomers.
Author Contributions
Conceptualization and finalization, S.-D.L.; Validation, T.Z., M.Z., X.-Q.L., Q.-Q.Y. and X.-N.Z.; Writing, T.Z., S.-D.L. All authors have read and agreed to the published version of the manuscript.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
Sample Availability
Samples of the compounds are not available from the authors.
Funding Statement
This work was supported by the National Natural Science Foundation of China (21973057 and 21720102006 to S.-D. L).
Footnotes
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
References
- 1.Jian T., Chen X.-N., Li S.-D., Boldyrev A.-I., Li J., Wang L.-S. Probing the structures and bonding of size-selected boron and doped-boron clusters. Chem. Soc. Rev. 2019;48:3550–3591. doi: 10.1039/C9CS00233B. [DOI] [PubMed] [Google Scholar]
- 2.Wang L.-S. Photoelectron spectroscopy of size-selected boron clusters: From planar structures to borophenes and borospherenes. Int. Rev. Phys. Chem. 2016;35:69–142. doi: 10.1080/0144235X.2016.1147816. [DOI] [Google Scholar]
- 3.Bai H., Chen T.-T., Chen Q., Zhao X. -Y.; Zhang, Y.-Y.; Chen, W.-J.; Li, W.-L.; Cheung, L.-F.; Bai, B.; Cavanagh, J.; Huang, W.; et al. Planar B41− and B42− clusters with double-hexagonal vacancies. Nanoscale. 2019;11:23286–23295. doi: 10.1039/C9NR09522E. [DOI] [PubMed] [Google Scholar]
- 4.Zhai H.-J., Zhao Y.-F., Li W.-L., Chen Q., Bai H., Hu H.-S., Piazza Z.-A., Tian W.-J., Lu H.-G., Wu Y.-B., et al. Observation of an all-boron fullerene. Nat. Chem. 2014;6:727–731. doi: 10.1038/nchem.1999. [DOI] [PubMed] [Google Scholar]
- 5.Chen Q., Li W.-L., Zhao Y.-F., Zhang S.-Y., Hu H.-S., Bai H., Li H.-R., Tian W.-J., Lu H.-G., Zhai H.-J., et al. Experimental and theoretical evidence of anaxially chiral borospherene. ACS Nano. 2015;9:754–760. doi: 10.1021/nn506262c. [DOI] [PubMed] [Google Scholar]
- 6.Chen W.-J., Ma Y.-Y., Chen T.-T., Ao M.-Z., Yuan D.-F., Chen Q., Tian X.-X., Mu Y.-W., Li S.-D., Wang L.-S. B48−: A bilayer boron cluster. Nanoscale. 2021;13:3868–3876. doi: 10.1039/D0NR09214B. [DOI] [PubMed] [Google Scholar]
- 7.Wang Y.-J., Zhao Y.-F., Li W.-L., Jian T., Chen Q., You X.R., Ou T., Zhao X.-Y., Zhai H.-J., Li S.-D., et al. Observation and characterization of the smallest borospherene, B28− and B28. J. Chem. Phys. 2016;144:064307. doi: 10.1063/1.4941380. [DOI] [PubMed] [Google Scholar]
- 8.Li H.-R., Jian T., Li W.-L., Miao C.-Q., Wang Y.-J., Chen Q., Luo X.-M., Wang K., Zhai H.-J., Li S.-D., et al. Competition between quasi-planar and cage-like structures in the B29− cluster: Photoelectron spectroscopy and ab initio calculations. Phys. Chem. Chem. Phys. 2016;18:29147–29155. doi: 10.1039/C6CP05420J. [DOI] [PubMed] [Google Scholar]
- 9.Tian W.-J., Chen Q., Li H.-R., Yan M., Mu Y.-W., Lu H.-G., Zhai H.-J., Li S.-D. Saturn-like charge-transfer complexes Li4&B36, Li5&B36+, and Li6&B362+: Exohedral metalloborospherenes with a perfect cage-like B364− core. Phys. Chem. Chem. Phys. 2016;18:9922–9926. doi: 10.1039/C6CP01279E. [DOI] [PubMed] [Google Scholar]
- 10.Liu H., Mu Y.-W., Li S.-D. Axially Chiral Cage-like B38+ and B382+: New aromatic members of the borospherene family. J. Cluster Sci. 2022;33:81–87. doi: 10.1007/s10876-020-01943-z. [DOI] [Google Scholar]
- 11.Chen Q., Zhang S.-Y., Bai H., Tian W.-J., Gao T., Li H.-R., Miao C.-Q., Mu Y.-W., Lu H.-G., Zhai H.-J., et al. Cage-like B41+ and B422+: New chiral members of the borospherene family. Angew. Chem. Int. Ed. 2015;54:8160–8164. doi: 10.1002/anie.201501588. [DOI] [PubMed] [Google Scholar]
- 12.Si L., Tang C.-M. The reversible hydrogen storage abilities of metal Na (Li, K, Ca, Mg, Sc, Ti, Y) decorated all-boron cage B28. Int. J. Hydrogen Energ. 2017;42:16611–16619. doi: 10.1016/j.ijhydene.2017.05.181. [DOI] [Google Scholar]
- 13.Szwacki N.-G., Sadrzadeh A., Yakobson B.-I. Erratum: B80 Fullerene: An ab initio prediction of geometry, stability, and electronic structure. Phys. Rev. Lett. 2007;98:166804. doi: 10.1103/PhysRevLett.98.166804. [DOI] [PubMed] [Google Scholar]
- 14.Research highlights. Nature. 2007;447:4–5. doi: 10.1038/447004a. [DOI] [Google Scholar]
- 15.Pei L., Yan Q.-Q., Li S.-D. Predicting the structural transition in medium-sized boron nanoclusters: From bilayer B64, B66, B68, B70, and B72 to Core-Shell B74. Eur. J. Inorg. Chem. 2021;2021:2618–2624. doi: 10.1002/ejic.202100328. [DOI] [Google Scholar]
- 16.Yan Q.-Q., Pei L., Li S.-D. Predicting bilayer B50, B52, B56 and B58: Structural evolution in bilayer B48–B72 clusters. J. Mol. Model. 2021;27:364. doi: 10.1007/s00894-021-04954-3. [DOI] [PubMed] [Google Scholar]
- 17.Yan Q.-Q., Zhang T., Ma Y.-Y., Chen Q., Mu Y.-W., Li S.-D. A bottom-up approach from medium-sized bilayer boron nanoclusters to bilayer borophene nanomaterials. Nanoscale. 2022;14:11443–11451. doi: 10.1039/D2NR02950B. [DOI] [PubMed] [Google Scholar]
- 18.Chen Q., Wei G.-F., Tian W.-J., Bai H., Liu Z.-P., Zhai H.-J., Li S.-D. Quasi-planar aromatic B36 and B36− clusters: All-boron analogues of coronene. Phys. Chem. Chem. Phys. 2014;16:18282–18287. doi: 10.1039/C4CP02032D. [DOI] [PubMed] [Google Scholar]
- 19.Ma Y.-Y., Zhao X.-Y., Zan W.-Y., Mu Y.-W., Zhang Z.-H., Li S.-D. Prediction of freestanding semiconducting bilayer borophenes. Nano Res. 2022;15:5752–5757. doi: 10.1007/s12274-022-4169-x. [DOI] [Google Scholar]
- 20.Prasad D.-L., Jemmis E.-D. Stuffing improves the stability of fullerenelike boron clusters. Phys. Rev. Lett. 2008;100:165504. doi: 10.1103/PhysRevLett.100.165504. [DOI] [PubMed] [Google Scholar]
- 21.Li H., Shao N., Shang B., Yuan L.-F., Yang J., Zeng X.C. Icosahedral B12-containing core–shell structures of B80. Chem. Commun. 2010;46:3878–3880. doi: 10.1039/c002954h. [DOI] [PubMed] [Google Scholar]
- 22.Zhao J.-J., Wang L., Li F.-Y., Chen Z.-F. B80 and other medium-sized boron clusters: Core−shell structures, not hollow cages. J. Phys. Chem. A. 2010;114:9969–9972. doi: 10.1021/jp1018873. [DOI] [PubMed] [Google Scholar]
- 23.Sai L.-W., Wu X., Yu F.-Y. B96: A complete core–shell structure with high symmetry. Phys. Chem. Chem. Phys. 2022;24:15687–15690. doi: 10.1039/D2CP01865A. [DOI] [PubMed] [Google Scholar]
- 24.Li F.-Y., Jin P., Jiang D.-E., Wang L., Zhang S.-B., Zhao J.-J., Chen Z.-F. B80 and B101–103 clusters: Remarkable stability of the core-shell structures established by validated density functionals. J. Chem. Phys. 2012;136:074302. doi: 10.1063/1.3682776. [DOI] [PubMed] [Google Scholar]
- 25.Sai L.-W., Wu X., Gao N., Zhao J.-J., King R.-B. Boron clusters with 46, 48, and 50 atoms: Competition among the core–shell, bilayer and quasi-planar structures. Nanoscale. 2017;9:13905–13909. doi: 10.1039/C7NR02399E. [DOI] [PubMed] [Google Scholar]
- 26.Zhang M., Lu H.-G., Li S.-D. B111, B112, B113, and B114: The most stable core-shell borospherenes with an icosahedral B12 core at the center exhibiting superatomic behaviors. Nano Res. 2021;14:4719–4724. doi: 10.1007/s12274-021-3411-x. [DOI] [Google Scholar]
- 27.Zhang M., Jia W.-P., Zhang T., Pei B.-B., Xu J., Tian X.-X., Lu H.-G., Li S.-D. Superatomic icosahedral-CnB12-n (n = 0, 1, 2) stuffed mononuclear and binuclear borafullerene and borospherene nanoclusters with spherical aromaticity. Sci. Rep. 2022;12:19741. doi: 10.1038/s41598-022-21809-w. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 28.Romanescu C., Galeev T.-R., Li W.-L., Boldyrev A.-I., Wang L.-S. Aromatic metal-centered monocyclic boron rings: Co©B8− and Ru©B9−. Angew. Chem. 2011;50:9334–9337. doi: 10.1002/anie.201104166. [DOI] [PubMed] [Google Scholar]
- 29.Chen T.-T., Li W.-L., Bai H., Chen W.-J., Dong X.-R., Li J., Wang L.-S. Re©B8− and Re©B9−: New members of the transition-metal-centered borometallic molecular wheel family. J.Phys. Chem. A. 2019;123:5317–5324. doi: 10.1021/acs.jpca.9b03942. [DOI] [PubMed] [Google Scholar]
- 30.Romanescu C., Galeev T.-R., Li W.-L., Boldyrev A.-I., Wang L.-S. Transition-metal-centered monocyclic boron wheel clusters (M©Bn): A new class of aromatic borometallic compounds. Acc. Chem. Res. 2013;46:350–358. doi: 10.1021/ar300149a. [DOI] [PubMed] [Google Scholar]
- 31.Popov I.-A., Li W.-L., Piazza Z.-A., Boldyrev A.-I., Wang L.-S. Complexes between planar boron clusters and transition metals: A photoelectron spectroscopy and ab initio study of CoB12− and RhB12−. J. Phys. Chem. A. 2014;118:8098–8105. doi: 10.1021/jp411867q. [DOI] [PubMed] [Google Scholar]
- 32.Popov I.-A., Jian T., Lopez G.-V., Boldyrev A.-I., Wang L.-S. Cobalt-centred boron molecular drums with the highest coordination number in the CoB16− cluster. Nat. Commun. 2015;6:8654. doi: 10.1038/ncomms9654. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Jian T., Li W.-L., Popo I.-A., Lope G.-V., Chen X., Boldyrev A.-I., Li J., Wang L.-S. Manganese-centered tubular boron cluster–MnB16−: A new class of transition-metal molecules. J. Chem. Phys. 2016;144:154310. doi: 10.1063/1.4946796. [DOI] [PubMed] [Google Scholar]
- 34.Jian T., Li W.-L., Chen X., Chen T.-T., Lopez G.-V., Li J., Wang L.S. Competition between drum and quasi-planar structures in RhB18−: Motifs for metallo-boronanotubes and metallo-borophenes. Chem. Sci. 2016;7:7020–7027. doi: 10.1039/C6SC02623K. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Li W.-L., Jian T., Chen X., Li H.-R., Chen T.-T., Luo X.-M., Li S.-D., Li J., Wang L.-S. Observation of a metal-centered B2-Ta@B18− tubular molecular rotor and a perfect Ta@B20− boron drum with the record coordination number of twenty. Chem. Commun. 2017;53:1587–1590. doi: 10.1039/C6CC09570D. [DOI] [PubMed] [Google Scholar]
- 36.Chen T.-T., Li W.-L., Li J., Wang L.-S. [La(ηx-Bx)La]− (x = 7–9): A new class of inverse sandwich complexes. Chem. Sci. 2019;10:2534–2542. doi: 10.1039/C8SC05443F. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Chen T.-T., Li W.-L., Chen W.-J., Yu X.-H., Dong X.-R., Li J., Wang L.-S. Spherical trihedral metallo-borospherenes. Nat. Commun. 2020;11:2766. doi: 10.1038/s41467-020-16532-x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Zhao X.-Y., Yan M., Wei Z.-H., Li S.-D. Donor–acceptor duality of the transition-metal-like B2 core in core–shell-like metallo-borospherenes La3&[B2@B17]− and La3&[B2@B18]−. RSC Adv. 2020;10:34225–34230. doi: 10.1039/D0RA06769E. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 39.Lu X.-Q., Gao C.-Y., Wei Z.-H., Li S.-D. Cage-like La4B24 and Core-Shell La4B290/+/−: Perfect spherically aromatic tetrahedral metallo-borospherenes. J. Mol. Model. 2021;27:130. doi: 10.1007/s00894-021-04739-8. [DOI] [PubMed] [Google Scholar]
- 40.Lu X.-Q., Ao M.-Z., Tian X.-X., Zan W.-Y., Mu Y.-W., Li S.-D. Perfect cubic La-doped boron clusters La6&[La@B24]+/0 as the embryos of low-dimensional lanthanide boride nanomaterials. RSC Adv. 2020;10:12469–12474. doi: 10.1039/D0RA01616K. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Ao M.-Z., Lu X.-Q., Mu Y.-W., Zan W.-Y., Li S.-D. La@[La5&B30]0/−/2−: Endohedral trihedral metallo-borospherenes with spherical aromaticity. Phys. Chem. Chem. Phys. 2022;24:3918–3923. doi: 10.1039/D1CP05644A. [DOI] [PubMed] [Google Scholar]
- 42.Yan L.-J. Large B7 triangles in hollow spherical trihedral metallo-borospherenes and their endohedral complexes of B20TMn (TM = Sc, Y; n = 3, 4): A theoretical characterization. Inorg. Chem. 2022;61:10652–10660. doi: 10.1021/acs.inorgchem.2c00624. [DOI] [PubMed] [Google Scholar]
- 43.Li H.-R., Liu H., Lu X.-Q., Zan W.-Y., Tian X.-X., Lu H.-G., Wu Y.-B., Mu Y.-W., Li S.-D. Cage-like Ta@Bnq complexes (n = 23–28, q = −1– +3) in 18-electron configurations with the highest coordination number of twenty-eight. Nanoscale. 2018;10:7451–7456. doi: 10.1039/C8NR01087K. [DOI] [PubMed] [Google Scholar]
- 44.Li H.-R., Liu H., Tian X.-X., Zan W.-Y., Mu Y.-W., Lu H.-G., Li J., Wang Y.-K., Li S.-D. Structural transition in metal-centered boron clusters: From tubular molecular rotors Ta@B21 and Ta@B22+ to cage-like endohedral metalloborospherene Ta@B22−. Phys. Chem. Chem. Phys. 2017;19:27025–27030. doi: 10.1039/C7CP05179D. [DOI] [PubMed] [Google Scholar]
- 45.Zhang Y., Zhao X.-Y., Yan M., Li S.-D. From inverse sandwich Ta2B7+ and Ta2B8 to spherical trihedral Ta3B12−: Prediction of the smallest metallo-borospherene. RSC. Adv. 2020;10:29320–29325. doi: 10.1039/D0RA05570K. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 46.Zhang Y., Lu X.-Q., Yan M., Li S.-D. Perfect spherical tetrahedral metallo-borospherene Ta4B18 as a superatom following the 18-electron rule. ACS Omega. 2021;6:10991–10996. doi: 10.1021/acsomega.1c00828. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 47.Bai H., Chen Q., Zhai H.-J., Li S.-D. Endohedral and exohedral metalloborospherenes: M@B40 (M=Ca, Sr) and M&B40 (M=Be, Mg) Angew Chem Int Ed. 2015;54:941–945. doi: 10.1002/anie.201408738. [DOI] [PubMed] [Google Scholar]
- 48.Yu T.-R., Gao Y., Xu D.-X., Wang Z.-G. Actinide endohedral boron clusters: A closed-shell electronic structure of U@B40. Nano Res. 2018;11:354–359. doi: 10.1007/s12274-017-1637-9. [DOI] [Google Scholar]
- 49.Czekner J., Cheung L.-F., Wang L.-S. Probing the structures of neutral B11 and B12 using high-resolutionphotoelectron imaging of B11− and B12−. J. Phys. Chem. C. 2017;121:10752–10759. doi: 10.1021/acs.jpcc.6b10958. [DOI] [Google Scholar]
- 50.Yuan Y., Cheng L.-J. Ferrocene analogues of sandwich B12·Cr·B12: A theoretical study. J. Chem. Phys. 2013;138:024301. doi: 10.1063/1.4773281. [DOI] [PubMed] [Google Scholar]
- 51.Adamo C., Barone V. Toward reliable density functional methods without adjustable parameters: The PBE0 model. J. Chem. Phys. 1999;110:6158–6170. doi: 10.1063/1.478522. [DOI] [Google Scholar]
- 52.Staroverov V.-N., Scuseria G.-E., Tao J., Perdew J.-P. Comparative assessment of a new nonempirical density functional: Molecules and hydrogen-bonded complexes. J. Chem. Phys. 2003;119:12129–12137. doi: 10.1063/1.1626543. [DOI] [Google Scholar]
- 53.Krishnan R., Binkley J.-S., Seeger R.-J., Pople A. Self-consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 1980;72:650–654. doi: 10.1063/1.438955. [DOI] [Google Scholar]
- 54.Purvis G.-D., III, Bartlett R.-J. A full coupled-cluster singles and doubles model: The inclusion of disconnected triples. J. Chem. Phys. 1982;76:1910–1918. doi: 10.1063/1.443164. [DOI] [Google Scholar]
- 55.Raghavachari K., Trucks G.-W., Pople J.-A., Head-Gordon M. A fifth-order perturbation comparison of electron correlation theories. Chem. Phys. Lett. 1989;157:479–483. doi: 10.1016/S0009-2614(89)87395-6. [DOI] [Google Scholar]
- 56.Glendening E.-D., Landis C.-R., Weinhold F. NBO 6.0: Natural bond orbital analysis program. J. Comput. Chem. 2013;34:1429–1437. doi: 10.1002/jcc.23266. [DOI] [PubMed] [Google Scholar]
- 57.Vondele J.-V., Krack M., Mohamed F., Parrinello M., Chassaing T.-S., Hutter J. Quickstep: Fast and accurate density functional calculations using a mixed Gaussian and plane waves approach. Comput. Phys. Commun. 2005;167:103–128. doi: 10.1016/j.cpc.2004.12.014. [DOI] [Google Scholar]
- 58.Zubarev D.-Y., Boldyrev A.-I. Developing paradigms of chemical bonding: Adaptive natural density partitioning. Phys. Chem. Chem. Phys. 2008;10:5207–5217. doi: 10.1039/b804083d. [DOI] [PubMed] [Google Scholar]
- 59.Tkachenko N.-V., Boldyrev A.-I. Chemical bonding analysis of excited states using the adaptive natural density partitioning method. Phys. Chem. Chem. Phys. 2019;21:9590–9596. doi: 10.1039/C9CP00379G. [DOI] [PubMed] [Google Scholar]
- 60.Kang S.-Y., Nan Z.-A., Wang Q.-M. Superatomic orbital splitting in coinage metal nanoclusters. J. Phys. Chem. Lett. 2022;13:291–295. doi: 10.1021/acs.jpclett.1c03563. [DOI] [PubMed] [Google Scholar]
- 61.Kleinpeter E., Klod S., Koch A. Visualization of through space NMR shieldings of aromatic and anti-aromatic molecules and a simple means to compare and estimate aromaticity. J. Mol. Struc: Theochem. 2007;811:45–60. doi: 10.1016/j.theochem.2007.02.049. [DOI] [Google Scholar]
- 62.Wang G.-J., Zhou M.-F., Goettel J.-T., Schrobilgen G.-J., Su J., Li J., Schloeder T., Riedel S. Identification of an iridium-containing compound with a formal oxidation state of IX. Nature. 2014;514:475–477. doi: 10.1038/nature13795. [DOI] [PubMed] [Google Scholar]
- 63.Ciuparu D., Klie R.-F., Zhu Y.-M., Pfefferle L. Synthesis of pure boron single-wall nanotubes. J. Phys. Chem. B. 2004;108:3967–3969. doi: 10.1021/jp049301b. [DOI] [Google Scholar]
- 64.Bauernschmitt R., Ahlrichs R. Treatment of electronic excitations within the adiabatic approximation of time dependent density functional theory. Chem. Phys. Lett. 1996;256:454–464. doi: 10.1016/0009-2614(96)00440-X. [DOI] [Google Scholar]
- 65.Casida M.-E., Jamorski C., Casida K.-C., Salahub D.-R. Molecular excitation energies to high-lying bound states from time-dependent density-functional response theory: Characterization and correction of the time-dependent local density approximation ionization threshold. J. Chem. Phys. 1998;108:4439–4449. doi: 10.1063/1.475855. [DOI] [Google Scholar]
- 66.Zhao Y.-F., Chen X., Li J. TGMin: A global-minimum structure search program based on a constrained basin-hopping algorithm. Nano Res. 2017;10:3407–3420. doi: 10.1007/s12274-017-1553-z. [DOI] [Google Scholar]
- 67.Chen X., Zhao Y.-F., Zhang Y.-Y., Li J. TGMin: An efficient global minimum searching program for free and surface-supported clusters. J. Comput. Chem. 2019;40:1105–1112. doi: 10.1002/jcc.25649. [DOI] [PubMed] [Google Scholar]
- 68.Goedecker S. Minima hopping: An efficient search method for the global minimum of the potential energy surface of complex molecular systems. J. Chem. Phys. 2004;120:9911–9917. doi: 10.1063/1.1724816. [DOI] [PubMed] [Google Scholar]
- 69.Goedecker S., Hellmann W., Lenosky T. Global Minimum Determination of the Born-Oppenheimer Surface within Density Functional Theory. Phys. Rev. Lett. 2005;95:055501. doi: 10.1103/PhysRevLett.95.055501. [DOI] [PubMed] [Google Scholar]
- 70.Lu T., Chen F.-W. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012;33:580–592. doi: 10.1002/jcc.22885. [DOI] [PubMed] [Google Scholar]
- 71.Humphrey W., Dalke A., Schulten K. VMD: Visual molecular dynamics. J. Mol. Graphics. 1996;14:33. doi: 10.1016/0263-7855(96)00018-5. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
Not applicable.