Insights into the photovoltaic properties of indium sulfide as an electron transport material in perovskite solar cells

Abstract

According to recent reports, planar structure-based organometallic perovskite solar cells (OPSCs) have achieved remarkable power conversion efficiency (PCE), making them very competitive with the more traditional silicon photovoltaics. A complete understanding of OPSCs and their individual parts is still necessary for further enhancement in PCE. In this work, indium sulfide (In₂S₃)-based planar heterojunction OPSCs were proposed and simulated with the SCAPS (a Solar Cell Capacitance Simulator)-1D programme. Initially, OPSC performance was calibrated with the experimentally fabricated architecture (FTO/In₂S₃/MAPbI₃/Spiro-OMeTAD/Au) to evaluate the optimum parameters of each layer. The numerical calculations showed a significant dependence of PCE on the thickness and defect density of the MAPbI₃ absorber material. The results showed that as the perovskite layer thickness increased, the PCE improved gradually but subsequently reached a maximum at thicknesses greater than 500 nm. Moreover, parameters involving the series resistance as well as the shunt resistance were recognized to affect the performance of the OPSC. Most importantly, a champion PCE of over 20% was yielded under the optimistic simulation conditions. Overall, the OPSC performed better between 20 and 30 °C, and its efficiency rapidly decreases above that temperature.

Introduction

The scientific community has shown a great deal of interest in researching perovskite solar cells (OPSCs), which are mainly comprised of organic–inorganic metal halide compounds and are used to produce high-efficiency and inexpensive photovoltaic (PV) technologies^1–3. These semiconductors have a number of important characteristics, including high charge carrier mobility, long carrier diffusion length, adjustable bandgaps, and a high absorption coefficient^4–7. Due to such exceptional properties, photoconversion efficiency (PCE) values spiked substantially, from 3.8% in 2009 to over 25% in 2021^8–11. In order, an OPSC has a front electrode, an electron-transport material (ETM), a light harvesting layer, a hole-transport material (HTM), and a back electrode. The harvester material of an OPSC generates charge carriers when exposed to sunlight^12–15. These photocarriers are delivered to the appropriate electrodes by ETMs and HTMs. The relevance of charge transport materials is crucial to the entire PV performance of OPSCs, in addition to the perovskite layer's role. For instance, titanium dioxide (TiO₂), a common ETM, is not suitable for fabricating large devices since it demands an operating temperature of more than 400 °C. The use of TiO₂ in high-efficiency OPSCs is further limited by the material's poor electron mobility (µ_e) and UV instability^16–18. This highlights the need to look for a candidate ETM layer with appropriate properties, such as high µ_e, good electrical conductivity (σ), and low-temperature manufacturing.

Compact ETM-based planar PSCs have a simplified layout and are easier to fabricate. TiO₂ and ZnO have been widely used as ETMs for planar n-i-p OPSCs^19–23. Nevertheless, planar OPSCs based on compacted TiO₂ and ZnO often exhibit low stability because of the materials' limited carrier mobility, offset energy level alignment with perovskites, and defect traps at the surface^24–28. As a result, it is important to provide cutting-edge ETM components for OPSCs. Indium sulfide (In₂S₃) is an n-type semiconductor with excellent carrier mobility, nontoxicity, an adequate bandgap, adjustable electrical properties, and good thermal durability^29,30, all of which are ideal for utilization as an ETM in solar cells^31,32. By adjusting the period of chemical bath deposition to 2 h, Hou et al. were able to construct an In₂S₃ nanoflakes array as ETMs for CH₃NH₃PbI₃ OPSCs, achieving a performance of 18.22%. However, the long-term stability of In₂S₃-OPSC was not examined in this work³⁰. One year later, Xu et al. prepared In₂S₃ sheets as ETMs for CH₃NH₃PbI₃ devices using a solvent-thermal approach for 2 h and achieved an efficiency of 18.83%³³. Subsequently, Yang et al. made further efforts to use In₂S₃ film and developed a spray-assisted deposition technique as an ETM for semitransparent CsPbIBr₂ OPSCs. The optimized devices obtained a performance of 5.59% with improved ambient stability³⁴. Meanwhile, as far as we can tell, no theoretical studies relevant to adopting In₂S₃ as the ETM in perovskite solar cells have been reported.

In this work, the first ever conventional n-i-p planar architecture of OPSCs using In₂S₃ as the electron transport material has been simulated and optimized. To verify our data, we recreated the findings of an experimentally published 18.83% robust and stable single-cation OPSC (FTO/In₂S₃/MAPbI₃/Spiro-OMeTAD/Au)³³. To improve the efficiency of the control OPSC, the thickness variation of the perovskite (t_p) was further investigated. Along with thickness variation, the effects of defect density (N_T), series resistance (R_s), shunt resistance (R_sh), and operating temperature on OPSC performance were studied. Our research can offer some key advice for OPSC design and optimization based on theoretical principles.

Method and simulation

The numerical modeling of the devices enables us to understand the solar cell dynamics without the need for actual manufacturing. It also provides a high-level outline of the device's functionality. The one-dimensional SCAPS (version 3.3.07) was used in this simulation study. In 2000, researchers at the University of Gent in Belgium created this open-source program, which can be downloaded at any time³⁵. The SCAPS software assists in the modeling of planar and graded PV structures up to seven components, with the additional functionality of calculating the band alignment graph, current–voltage (J–V) behavior, quantum efficiency (QE), recombination and generation currents, and other essential PV characteristics. SCAPS-1D relies primarily on the well-established Poisson's formula and the continuity laws for electrons and holes to perform its calculations^36–39. SCAPS is very powerful software for performing solar cell and a description of the programme, and the algorithms it uses, is found in the literature^40,41 and in its user manual⁴².

$$\mathrm{Poisson}\mathrm{equation}:-\frac{\partial }{\partial x}\left(\epsilon ,\left(x\right),\frac{\partial V}{\partial x}\right)=q[p\left(x\right)n\left(x\right)+N_D^{+}\left(x\right)-N_A^{-}\left(x\right)+p_t\left(x\right)-n_t\left(x\right)]$$ 1

$$\mathrm{Continuity}\mathrm{equation}\mathrm{for}\mathrm{the}\mathrm{hole}:\frac{\partial p}{\partial t}=\frac{1}{q}\frac{\partial J_p}{\partial x}+G_p-R_p$$ 2

$$\mathrm{Continuity}\mathrm{equation}\mathrm{for}\mathrm{the}\mathrm{electron}:\frac{\partial n}{\partial t}=\frac{1}{q}\frac{\partial J_n}{\partial x}+G_n-R_n$$ 3

where q is the charge, V is the potential, p(x) is the free hole concentration, n(x) is the free electron concentration, ε is the dielectric permittivity, $N_D^{+}\left(x\right)$ is the donor density, $N_A^{-}\left(x\right)$ is the acceptor density, p_t(x) is the hole trap concentration, n_t(x) is the trap concentration of an electron, J_n is the current density of an electron, J_p is the current density of a hole, G_n is the electron generation rate, G_p is the holes generation rate, R_n is the recombination rate of electrons, R_p is the recombination rate of holes.

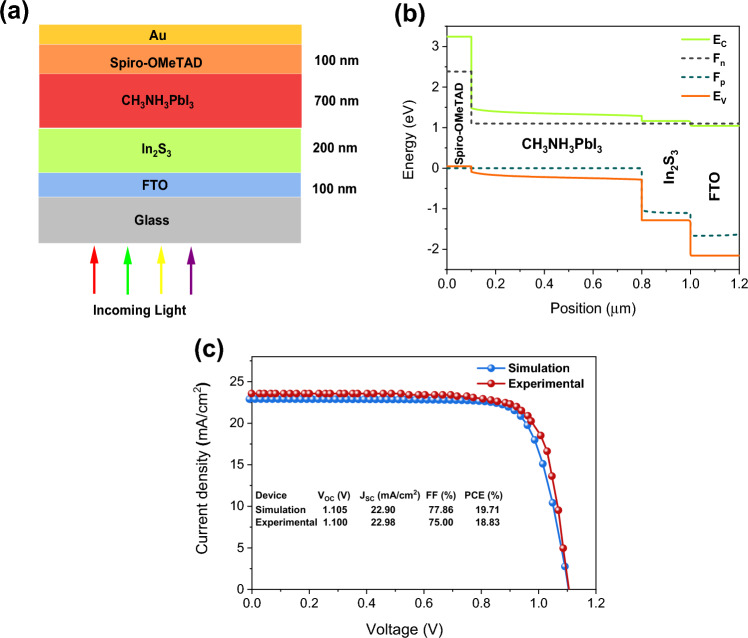
Here, we simulated a typical n-i-p PV architecture with CH3NH3PbI3 perovskite as the photoactive film, compact In₂S₃ as the ETM, and Spiro-OMeTAD organic film as the HTM, with fluorine-containing SnO₂ (FTO) and gold (Au) as the front and back electrodes, respectively. In Fig. 1a, we have a graphical diagram of the FTO/In₂S₃/CH₃NH₃PbI₃/Spiro-OMeTAD/Au device assembly. Tables 1 and 2 summarize the fundamental device parameters of several materials utilized in this analysis that were acquired from the theoretical and experimental literature. The work functions for the front and back electrodes were 4.4 eV and 5.2 eV, respectively. The SCAPS software calculated the absorption spectrum of each layer based on the optical merits of the materials and the geometry of the device.

Figure 1.

(a) Solar cell structure utilized in simulation. (b) Band alignment diagram of the proposed OPSC. (c) A representation comparison between experimental and modeled parameters of our control OPSC³³.

Table 1.

The input parameters of simulated perovskite solar cells.

Device layer properties	Device layers
	Unit	In2S3	IGZO	MAPbI3	Spiro-OMeTAD
Thickness	nm	200	200	700 (varied)	100
Energy gap	eV	2.45	3.05	1.57	3.2
Electron affinity energy	eV	3.98	4.16	3.86	2.1
µe	cm2/V s	400	15.0	8	2 × 104
µh	cm2/V s	210	0.1	4	2 × 104
Concentration of the shallow acceptor (NA)	1/cm3	0	0.0	1 × 1019 (varied)	1 × 1020
Concentration of the shallow donor (ND)	1/cm3	1.47 × 1018	1.0 × 1018	1 × 1019	0
CB effective density of states (NC)	1/cm3	1.8 × 1019	5.8 × 1018	1 × 1018	2.8 × 1018
VB effective density of states (NV)	1/cm3	4 × 1013	5.8 × 1018	1 × 1018	1.8 × 1019
Dielectric permittivity	–	6.5	10.0	28	3
Defect type	–	Neutral	Neutral	Neutral	Neutral
Capture cross section of electrons	cm2	1 × 10–15	1 × 10–15	1 × 10–15	1 × 10–15
Capture cross section of holes	cm2	1 × 10–15	1 × 10–15	1 × 10–15	1 × 10–15
NT	1/cm3	1 × 1016 (varied)	1.0 × 1015	2.45 × 1015 (varied)	1 × 1014
Energetic distribution	–	Single	Single	Single 0.6 eV above Ev	Single 0.6 eV above Ev
References		43	44	45	46

Table 2.

Interface parameters of FTO/ In₂S₃/MAPbI₃/Spiro-OMeTAD/Au OPSCs.

Parameters/interfaces	In2S3/MAPbI3	MAPbI3/spiro-OMeTAD
Defect type	Neutral	Neutral
Total defect density (cm−2)	1 × 1010	1 × 1010
Capture cross section electrons (cm2)	1 × 1010	1 × 10−18
Capture cross section holes (cm2)	1 × 10−19	1 × 10−18
Energetic distribution	Single	single
Reference for defect energy level Et	Above the highest Ev	Above the highest Ev
Energy with respect to reference (eV)	0.600	0.600
Total density (1/cm2)	1 × 1010	1 × 1010

The defects were used 0.6 eV above the valence band with a particular energy of 0.1 eV, taking into account the Gaussian energy distribution and the capture cross-section of carriers of 10^–15 cm². The radiative recombination coefficient for perovskite was 2.3 × 10^–9 cm³/s, which was taken into consideration. The modeling analysis added imperfections at the HTM/perovskite and perovskite/ETM interfaces about 10¹⁰ cm⁻². The conventional AM 1.5 G spectrum and a temperature of 300 K were used for the computations.

Figure 1b displays the band structure diagram for the suggested n-i-p OPSC layout. At the conduction band interface of In₂S₃ and CH₃NH₃PbI₃, a potential barrier of 0.12 eV exists, which is a beneficial barrier for the better transport of electrons from perovskite to ETM, whereas at the junction of the valence band of perovskite and HTM, holes have to contend with a large barrier of 0.13 eV. The J–V plot of the suggested cell architecture was analyzed after the appropriate layer parameters and operational conditions were determined (as covered in this section). Figure 1c displays the calculated J–V graph and its initial output parameters. We attained a power conversion efficiency (PCE) of 19.71%, which is close to the PCE of 18.83% that has been published experimentally³³. A slight mismatch between the experimental and computed results is that in the present research, the FTO and Au layers were utilized as front and back electrodes, where the thickness of front and back contacts cannot be changed. In the experimental research, however, they were employed as layers with appropriate thicknesses.

Ethics approval and consent to participate

This article does not contain any studies with human participants or animals performed by the authors. We comply with the ethical standards. We provide our consent to take part.

Results and discussion

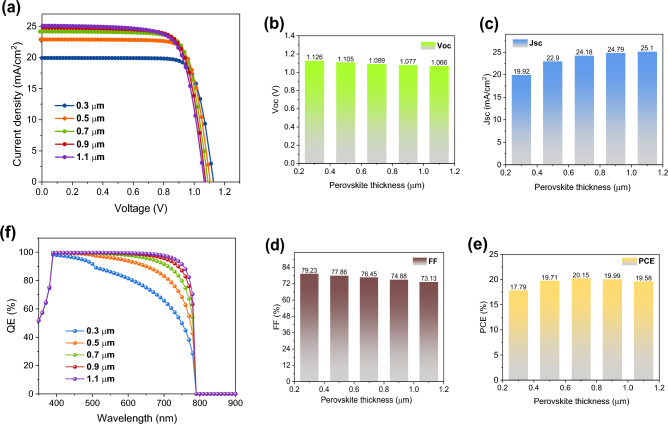
Increasing the device's efficiency is highly dependent on the thickness of the absorber layer. Nevertheless, using a very thick photoactive layer leads to a low charge carrier extraction rate and considerable losses owing to charge recombination; finding the right equilibrium between these two variations is crucial. Therefore, optimizing the light-absorbing thickness becomes essential for determining photocarrier production and spectrum response in photovoltaics⁴⁷. The obtained J–V graphs are shown in Fig. 2a, with perovskite thickness variations ranging from 0.3 to 1.1 µm, whereas Fig. 2b–e show the variations in the J_SC, V_OC, FF, and PCE parameters. According to Fig. 2, an increment in the perovskite thickness causes a rise in the J_SC and a reduction in the V_OC. The trend of rising J_SC values is a result of increased photocarrier production. Thin perovskite film results in lower long-wavelength photon absorption rates, which results in less photocarrier formation and worse J_SC values⁴⁸. Furthermore, the poor recombination due to the thin perovskite creates a high V_OC. Increasing the absorber perovskite's thickness also boosts the layer's ability to absorb light with longer wavelengths. As a result, more charge carriers are produced, which leads to a rise in the value of the J_SC⁴⁹. However, with higher absorbance, the recombination rate of photocarriers also increases since photocarriers have to cover a longer distance before approaching the corresponding electrodes. The increase in perovskite thickness raises the R_s, which causes a decrease in FF. The improvement in efficiency is attributable to the steady rise in J_SC. Our calculations suggest that the ideal value for the perovskite thickness should be 0.7 µm for the highest performance of MAPbI₃-based single-cation OPSC. Therefore, optimizing the thickness of the perovskite layer is crucial for achieving the highest efficiency in a perovskite solar cell. By carefully balancing the absorption of light and the extraction of charge carriers, an optimal thickness can be found that maximizes the photocurrent and minimizes recombination, leading to the best performance of the device.

Figure 2.

(a) J–V characteristics of the OPSCs with different MAPbI₃ thicknesses. Variations of OPSC performance parameters with various thicknesses of perovskite: (b) V_OC, (c) J_SC, (d) FF, and (e) PCE. (f) QE of devices with various MAPbI₃ thicknesses.

Figure 2f illustrates the external QE (EQE) of devices with varying MAPbI₃ film thicknesses. The EQE of the device was clearly improved when the MAPbI₃ light harvester thickness was less than 0.7 µm, which indicates that the improvement in absorption was high. Nevertheless, the EQE of the device rose less when the thickness of MAPbI₃ was more than 0.7 µm, indicating that the rise in absorption was less significant. As the MAPbI₃ film thickness increased, it was better able to absorb light of longer wavelengths⁵⁰. The profile of carrier generation rate is also obtained and reported in Fig. 3 to validate the higher penetration of generation rate in the absorber layer at higher thicknesses.

Figure 3.

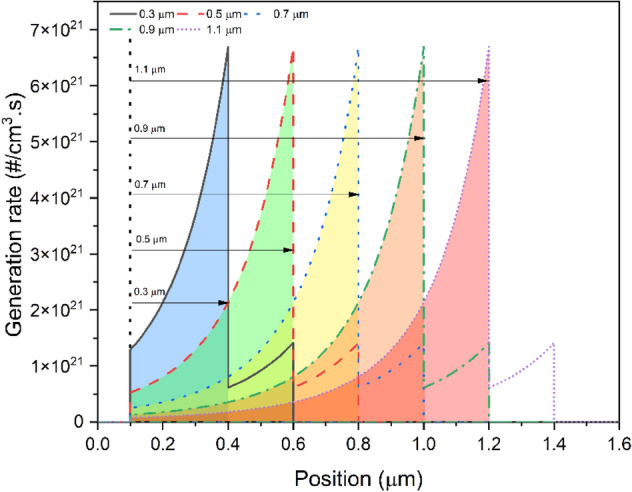
Generation rate inside the device with at different thicknesses of the absorber layer.

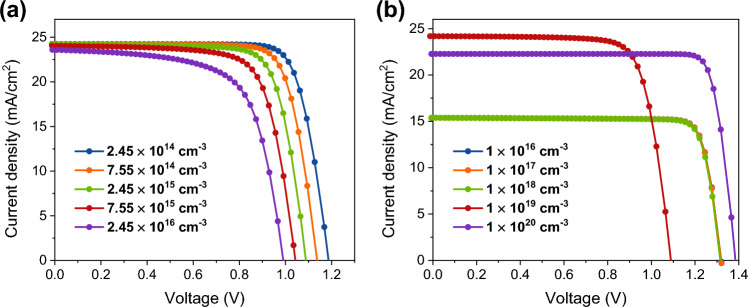
The number of defects in the photoactive MAPbI₃ has a significant impact on the output quality of perovskite solar cells. The V_OC of the device may be optimized by controlling the generation-recombination rate of the photocarriers inside the perovskite. Shockley–Read–Hall (SRH) recombination may provide a more adequate explanation for the correlation between N_T and OPSC performance^37,49. The perovskite defect density in this analysis ranges from 2.45 × 10¹⁴ to 2.45 × 10¹⁶ cm⁻³, and its impact on how well our computed work performs is investigated. Figure 4a displays J–V graphs that have been plotted with varying N_T values. Results show that a minor decrease in J_SC—from 24.241 to 23.582 mA/cm² and a major reduction in V_OC—from 1.188 to 0.991 V—are found when the N_T is increased from 2.45 × 10¹⁴ to 2.45 × 10¹⁶ cm⁻³ (Table 3). Since FF is dependent on V_OC, there is a significant decrease in FF values (from 79.163 to 66.498%). The efficiency was dramatically reduced from 22.79 to 15.55% because of these decreases in J_SC, V_OC, and FF values. This suggests that a rise in the N_T values leads to a greater number of imperfections, which in turn raises the recombination process, as shown in Fig. 5. According to the experimentally stated value, we chose the N_T for the perovskite here to be 2.45 × 10¹⁵ cm⁻³, which accounts for carrier diffusion lengths (L_p) of photocarriers of about 0.65 µm³³.

Figure 4.

J–V plots of the OPSCs obtained with varying (a) total defect density and (b) concentration of the shallow acceptor in CH₃NH₃PbI₃.

Table 3.

PV device parameters of OPSCs with varying total defect density in CH₃NH₃PbI₃.

NT (cm−3)	Lp (µm)	VOC (V)	JSC (mA/cm2)	FF (%)	PCE (%)
2.45 × 1014	2.1	1.188	24.241	79.163	22.79
7.55 × 1014	1.2	1.138	24.227	78.347	21.60
2.45 × 1015	0.65	1.089	24.180	76.456	20.15
7.55 × 1015	0.37	1.043	24.039	73.047	18.32
2.45 × 1016	0.21	0.991	23.582	66.498	15.55

Figure 5.

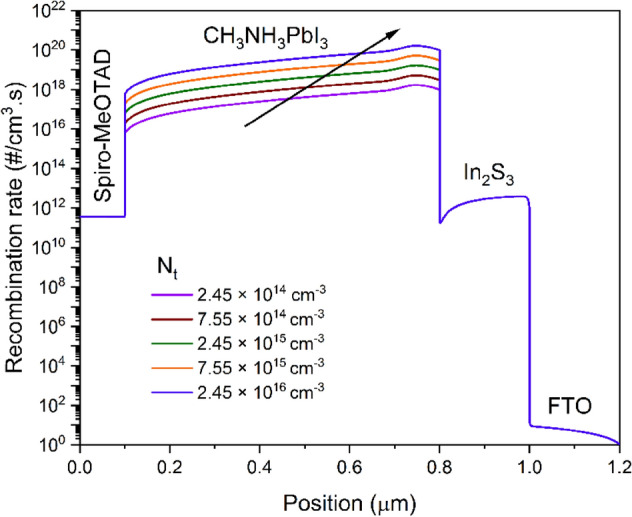
Recombination rate profile at different defect density in absorber layer.

The efficiency of OPSC is significantly affected by the amount of doping used. Doping can be categorized as either n-type or p-type, depending on the dopants used. Thus, improving OPSC efficiency relies on setting the appropriate value of N_A. Doping concentration levels can be adjusted experimentally in many different ways⁵¹. Doping concentrations and defect density values, for example, can be experimentally modified by adding different dopants or adjusting their concentrations in the perovskite material. Experimentally changing doping ratios and minimizing defects may also be accomplished by adjusting the relative amounts of cesium (Cs), methylammonium iodide (MAI), formamidinium iodide (FAI), and lead iodide (PbI₂)⁵².

Furthermore, the N_A of the perovskite was adjusted from 10¹⁶ to 10²⁰ cm⁻³, and the results are shown in Fig. 4b to help understand the impact of doping on the OPSC performance. According to our findings, the J–V characteristics are unchanged at low N_A levels. Nevertheless, the inherent built-in electric field (V_bi) rises when N_A surpasses 10¹⁸ cm⁻³. The performance of the cell is enhanced by an increase in V_bi because it leads to improved separation of photocarriers. J_SC was shown to decrease with increasing N_A levels (Table 4). Auger recombination might explain a decline in J_SC value with rising N_A. Auger recombination rises with increasing doping ratios, which lowers device efficiency^53,54. Here, a further decline in J_SC was shown if the N_A was raised above 10¹⁹ cm⁻³. As a result, we decided to set the highest value for N_A in the current simulation at 10¹⁹ cm⁻³.

Table 4.

PV device parameters of OPSCs with varying concentration of the shallow acceptor in CH₃NH₃PbI₃.

NA (cm−3)	VOC (V)	JSC (mA/cm2)	FF (%)	PCE (%)
1 × 1016	1.319	15.382	85.548	17.36
1 × 1017	1.319	15.382	85.523	17.35
1 × 1018	1.316	15.381	85.236	17.26
1 × 1019	1.090	24.180	76.469	20.16
1 × 1020	1.386	22.270	86.143	26.60

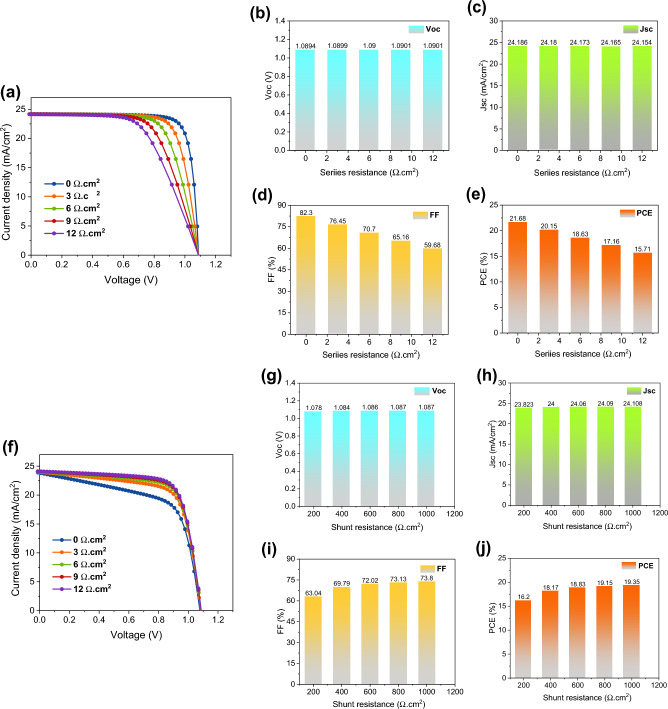
The series resistance (R_s) has a major effect on the operation of the OPSC, particularly the FF and short circuit current (I_SC). When the resistance of a series circuit rises, FF drops. Therefore, for higher levels of R_s, the I_SC begins to decrease as well. Hence, a device's efficiency suffers when R_s is quite high⁵⁵. This led researchers to examine how the PCE and FF of perovskite photoactive material changed with variations in R_s. We evaluate the performance of the OPSC while changing the R_s from 0 to 12 Ω cm² to examine the impact of R_s on OPSC performance. The J–V profiles for various resistances are depicted in Fig. 6a. Our research shows that the photovoltaic has superior performance and a higher FF at lower R_s (Fig. 6b–e). The efficiency of the devices deteriorates rapidly as the R_s rises. These findings are consistent with those reported in other studies^36,56.

Figure 6.

(a) J–V characteristics of the OPSCs with different series resistances. Variations of OPSC performance parameters with various series resistances: (b) V_OC, (c) J_SC, (d) FF, and (e) PCE. (f) J–V characteristics of the OPSCs with different shunt resistances with constant series resistance of 3 Ω cm². Variations of OPSC performance parameters with various shunt resistances: (g) V_OC, (h) J_SC, (i) FF, and (j) PCE.

Shunt resistance (R_sh) is caused by the several pathways for charge recombination in the OPSC⁵⁷. We simulate the device's operation, changing the R_sh from 0 to 1000 Ω cm², to examine the impact of R_sh on OPSC performance. Changing R_sh affects several different device characteristics, as seen in Fig. 6f,j. The performance of OPSC is found to improve as R_sh rises. PCE = 19.15% and FF = 73.13% at 800 Ω cm², and at 1000 Ω cm² we obtain PCE = 19.35% and FF = 73.8%, respectively. Therefore, we determine that an R_sh of 800 Ω cm² is optimal.

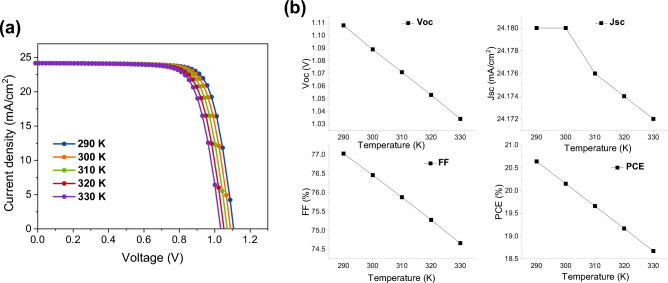
Figure 7a illustrates how altering the ambient temperature from 17 to 57 °C has an impact on the J–V plots of the OPSC device. It turns out that both V_OC and FF suffer when the temperature goes up. However, there are not any noticeable changes at J_SC. Efficiency gradually drops because both V_OC and FF are impacted by rising temperatures. This investigation demonstrates that OPSC in an ambient environment gives better efficiency, which is over 25%; however, as the temperature rises, this efficiency gradually declines, as shown in Fig. 7b. An increase in temperature increases the recombination and reverse saturation currents, which further reduce the V_OC and device performance. In addition, when the device is running at a higher temperature, the bandgap gets smaller, which may lead to more exciton recombination and less efficiency⁵⁸. This observation may be extremely important when choosing OPSC in tropical areas.

Figure 7.

(a) Effect of operational temperature with respect to (a) J–V curves and (b) PV parameters (J_SC, V_OC, FF, and PCE).

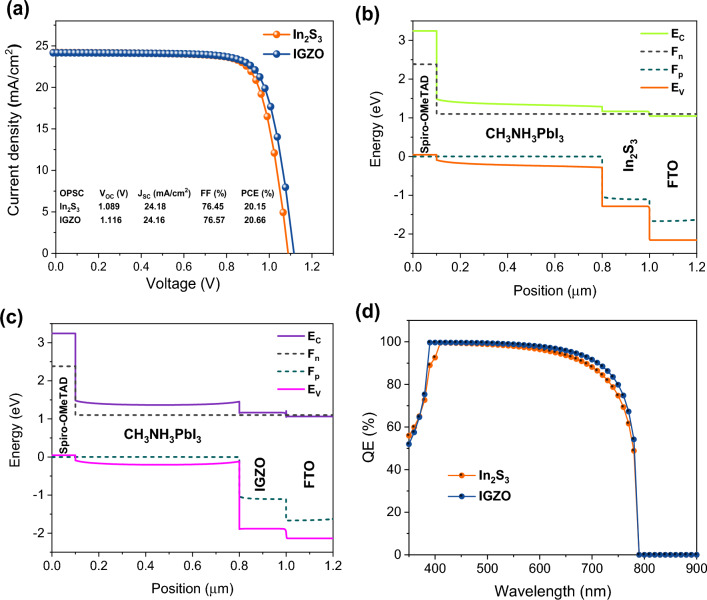
Finally, the performance of the optimized OPSC was compared to that of an OPSC made of indium gallium zinc oxide (IGZO) as an ETM layer (see Fig. 8a). Recently, IGZO has been used as an ETM; it offers great promise because of its high µ_e, environmental stability, low processing temperatures, and comparable electron affinity to perovskite^37,44,59. As we can see in inset table of Fig. 8, In₂S₃-based device showed comparable photovoltaic parameters to the IGZO-based device. The findings from this study are expected to facilitate the manufacturing of high-efficiency perovskite solar cells in the near future. The energy level layout is constructed by incorporating an ETM, a MAPbI₃ absorbing layer, and Spiro-OMeTAD as HTM. This arrangement affects the valence/conduction band offset, which refers to the variation in the valence band between the HTM and the perovskite, as well as the conduction band between the ETM and the perovskite. The energy level offset at the ETM/MAPbI₃ and the MAPbI₃/HTM interfaces greatly affects the solar cell’s performance³⁶. Figure 8b,c shows that quasi-Fermi levels F_n and F_p coexist with E_C and E_V in the OPSCs based on In₂S₃ and IGZO layers. As shown, the In₂S₃- and IGZO-based structures showed a small conduction band offset (CBO) of 0.121 eV and 0.294 eV at ETM/MAPbI₃ interface, indicating that In₂S₃ ETM provides better interface for electron transportation. However, IGZO film showed larger valence band offset at ETM/MAPbI₃ interface, which is significant for blocking the backflow of holes and suppressing the recombination rate in the OPSC.

Figure 8.

(a) J–V curves of perovskite solar cells with different ETMs, including In₂S₃ and IGZO films. Band offset behaviour of the proposed OPSC based on (b) In₂S₃ and (c) IGZO. The diagram was calculated using optimized thicknesses of ETMs (200 nm) and MAPbI₃ layer (700 nm). (d) EQE of OPSCs with various ETMs at a thickness of 200 nm.

Finally, we estimated The EQE spectra of In₂S₃ and IGZO ETM-based OPSCs, as shown in Fig. 8d. The EQE could vary depending on the specific features of the semiconductors and the design of the cell. IGZO ETM-based OPSC has been proven to demonstrate relatively higher QE spectrum across the visible spectrum than In₂S₃ ETM-based OPSC. This is because IGZO has a wide bandgap, which allows it to absorb a minimal amount of visible light while still effectively extracting electrons from the MAPbI₃ film. In general, it can be concluded that the utilization of both In2S3 and IGZO ETMs can effectively enhance the EQE of OPSCs. However, the selection of a suitable ETM is dependent upon the specific needs of the device and the preferred wavelength range for optimal performance.

We have provided insights into the relationship between the device's performance and the defects' density, which could be useful for optimizing the fabrication process and improving the device's performance. One possible approach to address this issue is to optimize the growth conditions during the fabrication process to minimize the defect density. For example, by carefully controlling the temperature, pressure, and some other important parameters of spin coating method during the growth process, it is possible to reduce the number of defects in the device. Interface passivation and anion/cation engineering can also be done to reduce the defect density. Additionally, post-growth processing techniques such as annealing could also reduce the density of defects in the material. In summary, we agree that the feasibility of tuning the property of the device at the fabrication or industrial level is an important consideration.

Conclusions

For the first time, the SCAPS-1D model has explored the potential of In₂S₃ as an alternate ETM film in OPSCs in an effort to increase PV stability, boost efficiency, and reduce hysteresis behavior. Problems with imperfections and high temperatures are fundamental to the simulation analysis. Theoretically, In₂S₃ can substitute TiO₂ as ETL in OPSC, and the results showed that defect states have a significant impact on OPSC efficiency at defect densities higher than 2.45 × 10¹⁵ cm⁻³. Finally, OPSC works best between 20 and 30 °C. The optimized design with an efficiency of 20.15% (V_OC = 1.089 V, J_SC = 24.18 mA/cm², and FF = 76.45%) sheds light on the possibility of In₂S₃ as a suitable ETL. This study paves the way towards practical implementation of indium sulfide as the potential ETL for MAPbI₃ perovskite solar cells.

Author contributions

Conceptualization, methodology, software, validation, A.K.A., A.K., S.Q.S., M.K.A.M.; formal analysis, investigation, resources, data curation, writing—original draft preparation, M.K.H., Z.M.Y., D.S.A.; supervision, project administration, D.D., R.P., P.S.J. All authors have read and agreed to the published version of the manuscript. All the authors are giving consent to publish.

Data availability

The datasets used and/or analysed during the current study available from the corresponding author on reasonable request.

Competing interests

The authors declare no competing interests.