Room-temperature hyperpolarization of polycrystalline samples with optically polarized triplet electrons: pentacene or nitrogen-vacancy center in diamond?

Abstract

We demonstrate room-temperature ${}^{\mathrm{13}}$ C hyperpolarization by dynamic nuclear polarization (DNP) using optically polarized triplet electron spins in two polycrystalline systems: pentacene-doped [carboxyl- ${}^{\mathrm{13}}$ C] benzoic acid and microdiamonds containing nitrogen-vacancy (NV ${}^{-}$ ) centers. For both samples, the integrated solid effect (ISE) is used to polarize the ${}^{\mathrm{13}}$ C spin system in magnetic fields of 350–400 mT. In the benzoic acid sample, the ${}^{\mathrm{13}}$ C spin polarization is enhanced by up to 0.12 % through direct electron-to- ${}^{\mathrm{13}}$ C polarization transfer without performing dynamic ${}^{\mathrm{1}}$ H polarization followed by ${}^{\mathrm{1}}\mathrm{H}{-}^{\mathrm{13}}\mathrm{C}$ cross-polarization. In addition, the ISE has been successfully applied to polarize naturally abundant ${}^{\mathrm{13}}$ C spins in a microdiamond sample to 0.01 %. To characterize the buildup of the ${}^{\mathrm{13}}$ C polarization, we discuss the efficiencies of direct polarization transfer between the electron and ${}^{\mathrm{13}}$ C spins as well as that of ${}^{\mathrm{13}}\mathrm{C}{-}^{\mathrm{13}}\mathrm{C}$ spin diffusion, examining various parameters which are beneficial or detrimental for successful bulk dynamic ${}^{\mathrm{13}}$ C polarization.

1. Introduction

Nuclear magnetic resonance (NMR) spectroscopy and magnetic resonance imaging (MRI) are powerful tools covering fields from physics, chemistry, and biology to medicine. However, the poor sensitivity remains the Achilles heel to all magnetic resonance experiments. Dynamic nuclear polarization (DNP), a technique to transfer spin polarization from electrons to nuclei, has been extensively studied since its early discovery and has seen an impressive revival in the current century . In DNP using unpaired electrons as the sources of polarization, the polarization enhancement factor $\mathit{ϵ}$ is limited to ${\mathit{\gamma }}_{\mathrm{e}}/{\mathit{\gamma }}_{\mathrm{n}}$ , where ${\mathit{\gamma }}_{\mathrm{e}\left(\mathrm{n}\right)}$ are the gyromagnetic ratios of the electron (nuclear) spins. To attain nuclear polarization of as high as $\sim \mathrm{10}$  %, DNP needs to be performed at very low temperature ( $<\mathrm{20}$  K) and in high magnetic fields ( $>\mathrm{3}$  T).

Conversely, the spins of optically polarized electrons can have much higher polarization than the thermal equilibrium value. DNP using such attractive sources of polarization leads to nuclear hyperpolarization beyond the limit of DNP using thermal electron polarization. Moreover, the spin polarization of the optically polarized electrons does not depend on the temperature and the magnetic field, except that the magnetic field happens to be such that level (anti)crossings take place . It follows that nuclear hyperpolarization is expected even at ambient temperatures and in relatively low magnetic fields. In times of global liquid helium shortage, this could become an elegant method without requiring liquid helium for sample cooling. Microwave-induced DNP using optically polarized electron spins in the triplet state, triplet DNP, was demonstrated in a single crystal of $p$ -dibromobenzene doped with $p$ -dichlorobenzene , fluorene doped with phenanthrene , and naphthalene doped with pentacene , and this DNP technique using pentacene achieved ${}^{\mathrm{1}}$ H polarization of 34 % at room temperature in 0.4 T . DNP using pentacene has been extended to polycrystalline samples , and dissolution DNP using pentacene-doped powder samples has been implemented. Furthermore, the range of molecules, hyperpolarized by triplet DNP, was successfully expanded . In these DNP experiments, the integrated solid effect (ISE) was used as a method for transferring the polarization. The ISE employs microwave irradiation and external magnetic-field sweep, so that the Hartmann–Hahn matching is implemented between the electron spins in the rotating frame and the nuclear spins in the laboratory frame .

In proton-rich organic solids or frozen solutions, a demonstrated strategy to polarize dilute or low- $\mathit{\gamma }$ nuclear spins is first to transfer the electron polarization to the protons, then to let the protons undergo spin diffusion to spatially transport the enhanced polarization away from the source electron spins, and finally to perform local polarization transfer from the protons to the target spin species. The protons in rigid solids are known to be efficient carriers of spin polarization. However, when, as is often the case, it is not the protons but other dilute or low- $\mathit{\gamma }$ spin species that are of NMR-spectroscopic interest, ${}^{\mathrm{1}}$ H hyperpolarization can be costly, because the relatively large heat capacity of the ${}^{\mathrm{1}}$ H reservoir consumes considerable resources of electron polarization. Then, a question arises: would direct dynamic polarization of dilute/low- $\mathit{\gamma }$ spins using the electrons in the triplet state be feasible without the protons being involved in the polarization-buildup process, and if it is, how efficient would that be? In this context, dynamic polarization of dilute/low- $\mathit{\gamma }$ spins in proton-free inorganic solids is also of interest.

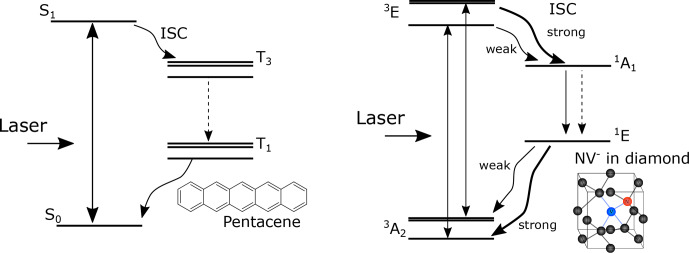
Here, we study dynamic ${}^{\mathrm{13}}$ C polarization using optically polarized electron spins in the triplet state through direct electron-to- ${}^{\mathrm{13}}$ C polarization transfer, i.e., without being mediated by the ${}^{\mathrm{1}}$ H spins. In this work, we deal with pentacene doped into a host matrix and negatively charged nitrogen-vacancy (NV ${}^{-}$ ) color centers in diamond. In both cases, the optically polarized triplet electron spins are the source for DNP. The electronic structures for these systems are shown in Fig.  . In the case of the NV ${}^{-}$ center, laser irradiation excites the electronic state from the ground triplet state ${}^{\mathrm{3}}{A}_{\mathrm{2}}$ to the excited triplet state ${}^{\mathrm{3}}E$ (Fig. ), which then undergoes intersystem crossing (ISC) to the singlet state ${}^{\mathrm{1}}{A}_{\mathrm{1}}$ . Importantly, this transition is highly spin selective: while the $m_{\mathrm{S}}=\mathrm{0}$ state has a low ISC probability, the $m_{\mathrm{S}}=\pm \mathrm{1}$ state has a high ISC probability. The singlet state ${}^{\mathrm{1}}{A}_{\mathrm{1}}$ further decays into a meta-stable singlet state ${}^{\mathrm{1}}{E}_{\mathrm{1}}$ , which preferentially decays into the $m_{\mathrm{S}}=\mathrm{0}$ spin sublevel of the ground triplet state ${}^{\mathrm{3}}{A}_{\mathrm{2}}$ , hyperpolarizing the $m_{\mathrm{S}}=\mathrm{0}$ state. In the case of pentacene, the ground singlet state $S_{\mathrm{0}}$ is photo-excited to the excited singlet state $S_{\mathrm{1}}$ . The subsequent transition to the triplet state $T_{\mathrm{3}}$ is caused by ISC, and then to the lowest triplet state $T_{\mathrm{1}}$ by internal conversion, where the spin-orbit coupling selectively populates the triplet sublevels. Moreover, the triplet population depends on the host molecule.

Figure 1.

Schematic diagrams of the electronic structure for NV ${}^{-}$ and pentacene. The optical transitions are denoted by solid straight arrows, the transitions caused by intersystem crossing are denoted by wavy arrows, and the transitions caused by internal conversion are denoted by dashed straight arrows.

Even though the relevant electron spins are in the triplet state ( $S=\mathrm{1}$ ) for both the pentacene molecules and the NV ${}^{-}$ centers, they differ in many aspects. For the NV ${}^{-}$ centers, the ground state is the triplet state, while in the case of pentacene, the ground state is the singlet state ( $S=\mathrm{0}$ ). The zero-field splitting (ZFS) parameters $D$ and $E$ are 2870 MHz and $\sim \mathrm{0}$  MHz for the NV ${}^{-}$ center and $\sim \mathrm{1350}$ and $\sim \mathrm{42}$  MHz for pentacene in the excited state, the latter of which may vary depending on the host molecule . The differences between these two systems are summarized in Table .

Table 1.

Differences between the two samples, NV ${}^{-}$ in microdiamonds and pentacene in [carboxyl- ${}^{\mathrm{13}}$ C] benzoic acid ( ${\mathit{\alpha }}^{\mathrm{13}}$ CBA).

Sample	NV ${}^{-}$ in microdiamonds	Pentacene in ${\mathit{\alpha }}^{\mathrm{13}}$ CBA
ZFS  $D$  (MHz)	2870	1350
ZFS  $E$  (MHz)	$\sim \mathrm{0}$	$-\mathrm{42}$
Electron spin	Defect in crystal	“Doped molecule”
Ground state	Triplet state	Singlet state
Meta-stable state	Singlet state	Triplet state
Average ${}^{\mathrm{13}}$ C distance $r_{\mathrm{C}}$  ( ${\mathit{\rho }}_{\mathrm{C}}^{\mathrm{1}/\mathrm{3}}$ ) (Å)	$\sim \mathrm{8.0}$	$\sim \mathrm{5.4}$

Microwave-free optical hyperpolarization for a bulk ensemble of ${}^{\mathrm{13}}$ C nuclear spins using NV ${}^{-}$ centers in a diamond single crystal was first demonstrated at cryogenic temperature in a magnetic field of 9.4 T . Optically pumped electron spins were used for nuclear hyperpolarization by exploiting an excited-state level anticrossing at 50 mT, followed by sample shuttling to a magnetic field of 4.7 T for NMR detection . The method was generalized to a broader range of magnetic fields and correspondingly different orientations of the NV ${}^{-}$ center by adding microwave irradiation . For a single crystal of diamond, the ${}^{\mathrm{13}}$ C polarization of 6 % at room temperature was achieved via DNP as a combination of the thermal mixing and the solid effect . Recently, DNP using the frequency-swept ISE on NV ${}^{-}$ in powdered microdiamonds in a magnetic field of as low as ca. 30 mT has been reported by , who took advantage of the reduced width of the anisotropic electron paramagnetic resonance (EPR) powder pattern of the NV ${}^{-}$ centers.

In the following, we report on optical DNP of ${}^{\mathrm{13}}$ C spins in microdiamonds containing NV ${}^{-}$ centers and in pentacene-doped [carboxyl- ${}^{\mathrm{13}}$ C] benzoic acid (PBA) microcrystals. While for the diamond samples the ${}^{\mathrm{13}}$ C isotope is naturally abundant, the carboxyl carbons are isotopically ${}^{\mathrm{13}}$ C-enriched in PBA microcrystals. We study the behavior of the buildup of the ${}^{\mathrm{13}}$ C polarization in terms of the efficiency of the polarization transfer from the electron to nuclear spins and that of ${}^{\mathrm{13}}$ C spin diffusion. As demonstrated below, we obtained ${}^{\mathrm{13}}$ C polarization of 0.01 % in the microdiamonds and 0.12 % in PBA at room temperature in a magnetic field of $\sim \mathrm{0.4}$  T by the ISE scheme.

In a slightly different context, both the pentacene and NV ${}^{-}$ systems can be used for single-spin optically detected magnetic resonance (ODMR). Pentacene in $p$ -terphenyl was the first single molecule which was detected by magnetic resonance . While these experiments were performed at cryogenic temperatures, the NV ${}^{-}$ center in diamond opened the door for single-spin experiments at room temperature . In this article, we will focus on the conventional induction detection of EPR/NMR on ensembles of spins.

2. Theory

Dynamic nuclear polarization using the integrated solid effect

Let us suppose that pulsed laser irradiation creates hyperpolarized electron spins in the triplet state. In the ISE, microwave irradiation and magnetic-field sweep are applied in such a way that the resonances of the individual electron spin packets between two triplet sublevels are adiabatically swept over and the Hartmann–Hahn condition is fulfilled at some point during the sweep, causing the contact between the electron spins in the rotating frame and the nuclear spins in the laboratory frame . The Hartmann–Hahn condition is expressed as

$${\mathit{\omega }}_{\mathrm{eff},\mathrm{e}}={\mathit{\omega }}_{\mathrm{0},\mathrm{C}}.$$ 1

Here, ${\mathit{\omega }}_{\mathrm{eff},\mathrm{e}}$ represents the electron nutation frequency around the effective field and is given by $\sqrt{{\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{2}}+(\mathrm{\Delta }{\mathit{\omega }}_{\mathrm{e}}{)}^{\mathrm{2}}}$ , where ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}$ is the microwave intensity and $\mathrm{\Delta }{\mathit{\omega }}_{\mathrm{e}}$ is the time-dependent resonance-offset frequency. ${\mathit{\omega }}_{\mathrm{0},\mathrm{C}}$ is the Larmor precession frequency of the nuclear spin species of interest, which is ${}^{\mathrm{13}}$ C in the present case. The exchange of the spin states and thereby of spin polarization is driven by the dipolar interaction between them through the same mechanism as that of cross-polarization . Thus, the source electron spins need to be locked along the effective field for polarization transfer to take place. This is ensured when the adiabatic sweep starts from far-off resonance, so that the effective field is initially aligned nearly along the static field and gradually tilts.

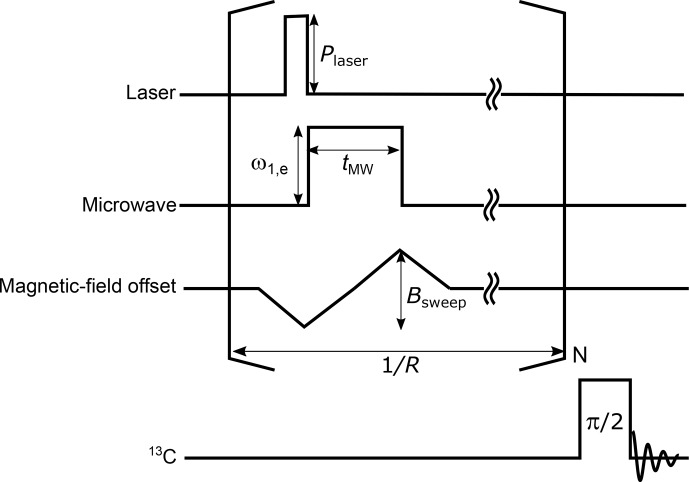
Since the polarization transfer is driven by the local dipolar interaction, direct hyperpolarization is limited to those nuclei which happen to be located in the vicinity of the electrons. Nevertheless, such a locally hyperpolarized ${}^{\mathrm{13}}$ C spin state can be transported away by spin diffusion. Thus, by repeating the ISE sequence as described in Fig. , the ${}^{\mathrm{13}}$ C polarization can be accumulated until the DNP buildup and the nuclear spin-lattice relaxation balance and a steady state is established.

Figure 2.

The pulse sequence for the ISE followed by NMR detection. A laser pulse is used for photoexcitation and optical polarization of the triplet electron spins. Then, a microwave pulse is applied together with a magnetic-field sweep. The ISE pulse sequence is repeated before the enhanced ${}^{\mathrm{13}}$ C magnetization is detected by applying a radiofrequency $\mathit{\pi }$ /2 pulse. During the acquisition of the ${}^{\mathrm{13}}$ C signal, ${}^{\mathrm{1}}$ H decoupling is to be applied in the case of the benzoic acid sample. The definitions of the symbols used in the figure are as follows. $P_{\mathrm{laser}}$ : laser-beam intensity, ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}$ : microwave intensity, $t_{\mathrm{MW}}$ : microwave-pulse width, $B_{\mathrm{sweep}}$ : field-sweep width, $R$ : ISE repetition rate, and $N$ : ISE repetition number.

In general, both the direct electron-to- ${}^{\mathrm{13}}$ C polarization transfer by the ISE and spin diffusion among the ${}^{\mathrm{13}}$ C spins contribute to the overall buildup of the bulk ${}^{\mathrm{13}}$ C polarization. Conversely, when ${}^{\mathrm{13}}$ C spin diffusion is fast such that the ${}^{\mathrm{13}}$ C polarization is smoothed out within the interval of the repeated ISE sequences, the buildup behavior of the bulk ${}^{\mathrm{13}}$ C spin polarization $P_{\mathrm{C}}$ becomes independent of the spin-diffusion rate. In this rapid-diffusion limit , the time evolution of $P_{\mathrm{C}}$ is governed by

$${\displaystyle \frac{\mathrm{d}}{\mathrm{d}t}}{P}_{\mathrm{C}}\simeq R\mathit{\xi }{\displaystyle \frac{{\mathit{\eta }}_{\mathrm{t}}{\mathit{\rho }}_{\mathrm{e}}}{{\mathit{\rho }}_{\mathrm{C}}}}({\mathit{\eta }}_{\mathrm{p}}\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}-P_{\mathrm{C}})-{\displaystyle \frac{\mathrm{1}}{T_{\mathrm{1},\mathrm{C}}}}{P}_{\mathrm{C}},$$ 2

where $R$ is the repetition rate of the ISE sequence, ${\mathit{\eta }}_{\mathrm{t}}$ is the fraction of the triplet electrons in two of the triplet sublevels and is obtained as $\frac{\mathrm{2}}{\mathrm{3}-P_{\mathrm{e}}}$ by considering that the populations in the $m_{\mathrm{S}}=+\mathrm{1}$ and $m_{\mathrm{S}}=-\mathrm{1}$ states are the same, $\mathit{\xi }$ is the exchange probability, i.e., the probability of the spin states being exchanged between the electron and the nuclear spins during a single ISE sequence, ${\mathit{\rho }}_{\mathrm{e}}$ is the density of the electrons in the triplet state, ${\mathit{\rho }}_{\mathrm{C}}$ is the density of ${}^{\mathrm{13}}$ C spins, and $T_{\mathrm{1},\mathrm{C}}$ is the longitudinal relaxation time of the ${}^{\mathrm{13}}$ C spins. ${\mathit{\eta }}_{\mathrm{p}}(\le \mathrm{1})$ , called the active spin-packet fraction, is introduced to deal with such situations where not all but some of the electron spin packets participate in the process of polarization transfer. This is indeed the case for polycrystalline samples, where the anisotropy of the ZFS tensor causes significant broadening to such an extent that the resonance line can only partly be excited. $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}$ is the electron polarization between two of the triplet sublevels averaged over the ISE sweep time $t_{\mathrm{MW}}$ , which is given as

$$\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}={\displaystyle \frac{\mathrm{1}}{t_{\mathrm{MW}}}}\underset{\mathrm{0}}{\overset{t_{\mathrm{MW}}}{\int }}{P}_{\mathrm{e}}\exp (-t/T_{\mathrm{1},\mathrm{e}})\mathrm{d}t.$$ 3

Here, $P_{\mathrm{e}}$ is the initial electron polarization between the relevant two triplet sublevels. $T_{\mathrm{1},\mathrm{e}}$ is the time constant introduced to take either spin-lattice relaxation or lifetime decay of the triplet state into account.

Neglecting the thermal polarization of ${}^{\mathrm{13}}$ C spins, we obtain the solution of this differential equation as

$$\begin{array}{}\text{4}& {\displaystyle }{P}_{\mathrm{C}}\left(t\right)=P_{\mathrm{fin}}(\mathrm{1}-\exp (-t/T_{\mathrm{b}}\left)\right),\text{5}& {\displaystyle \frac{\mathrm{1}}{T_{\mathrm{b}}}}={\displaystyle \frac{R\mathit{\xi }{\mathit{\eta }}_{\mathrm{t}}{\mathit{\rho }}_{\mathrm{e}}}{{\mathit{\rho }}_{\mathrm{C}}}}+{\displaystyle \frac{\mathrm{1}}{T_{\mathrm{1},\mathrm{C}}}},P_{\mathrm{fin}}={\displaystyle \frac{T_{\mathrm{1},\mathrm{C}}{\mathit{\eta }}_{\mathrm{p}}\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}}{\frac{{\mathit{\rho }}_{\mathrm{C}}}{R\mathit{\xi }{\mathit{\eta }}_{\mathrm{t}}{\mathit{\rho }}_{\mathrm{e}}}+T_{\mathrm{1},\mathrm{C}}}}.\end{array}$$

Here, $P_{\mathrm{fin}}$ is the maximum attainable nuclear spin polarization and $T_{\mathrm{b}}$ is the DNP buildup time constant. We note that the initial buildup rate, given as

$${\displaystyle \frac{\mathrm{d}}{\mathrm{d}t}}{P}_{\mathrm{C}}(t=\mathrm{0})=R{\displaystyle \frac{{\mathit{\rho }}_{\mathrm{e}}}{{\mathit{\rho }}_{\mathrm{C}}}}{\mathit{\eta }}_{\mathrm{t}}{\mathit{\eta }}_{\mathrm{p}}\mathit{\xi }\stackrel{\mathrm{‾}}{P_{\mathrm{e}}},$$ 6

is independent of the longitudinal relaxation time $T_{\mathrm{1},\mathrm{C}}$ .

In the following, we will use Eqs. (4)–(6) for data analysis.

The rapid-diffusion limit can be made valid by performing experiments with a sufficiently slow repetition rate $R$ . Buildup experiments with the slow repetition rate, albeit not advantageous for attaining the highest possible nuclear polarization, give helpful data that allow us to characterize the parameters.

3. Experimental

3.1. Sample preparation

We used the following three samples: (i) 70 mg of 500  $\mathrm{\mu }\mathrm{m}$ microdiamonds, with $\mathrm{8.9}\times {\mathrm{10}}^{\mathrm{17}}$  cm ${}^{-\mathrm{3}}$ (5 ppm in atomic ratio) NV ${}^{-}$ concentration and $\mathrm{4.6}\pm \mathrm{0.1}\times {\mathrm{10}}^{\mathrm{18}}$  cm ${}^{-\mathrm{3}}$ (26 ppm) P1 center concentration. So-called “P1 centers” are another type of electron spin defects in diamond with $S=\mathrm{1}/\mathrm{2}$ and represent single substitutional nitrogen atoms. (ii) 5.6 mg of 100 nm nanodiamonds, with $\mathrm{5.3}\times {\mathrm{10}}^{\mathrm{17}}$  cm ${}^{-\mathrm{3}}$ (3 ppm) NV ${}^{-}$ concentration, $\mathrm{1.2}\times {\mathrm{10}}^{\mathrm{19}}$  cm ${}^{-\mathrm{3}}$ (70 ppm) P1 center concentration, and a concentration of $\mathrm{3.1}\times {\mathrm{10}}^{\mathrm{19}}$  cm ${}^{-\mathrm{3}}$ (177 ppm) for the total $S=\mathrm{1}/\mathrm{2}$ electron spins (P1 centers, dangling bonds, etc.); 100 nm sized nanodiamonds “MICRON+ MDA M0.10” were purchased from Element Six, UK, and electron irradiated at a fluence of ${\mathrm{10}}^{\mathrm{19}}$  e ${}^{-}$ /cm ${}^{\mathrm{2}}$ at room temperature to form vacancies. To create the NV ${}^{-}$ centers, the nanodiamond samples were annealed at 800  ${}^{\circ }$ C under vacuum ( $<{\mathrm{10}}^{-\mathrm{4}}$  Pa). The temperature was increased to 400  ${}^{\circ }$ C over 1 h and kept for 4 h (“baking”). After that, the temperature was increased to 800  ${}^{\circ }$ C over 11 h, where the sample was annealed for 2 h. The temperature was decreased to 350  ${}^{\circ }$ C in 1 h and then down to room temperature. These “dark” nanodiamonds (due to amorphous sp ${}^{\mathrm{2}}$ carbon on the surface) were “made white” by oxidation in air at 550  ${}^{\circ }$ C for 2 h, followed by boiling acid cleaning in ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{HNO}}_{\mathrm{3}}$ ( $\mathrm{3}:\mathrm{1}$ , 125  ${}^{\circ }$ C) for 3 d . Both diamond samples contain 1.1 % natural abundance ${}^{\mathrm{13}}$ C. (iii) 4 mg of PBA powder, with 0.04 mol% ( $\mathrm{2.6}\times {\mathrm{10}}^{\mathrm{18}}$  cm ${}^{-\mathrm{3}}$ ) concentration of pentacene. The [carboxyl- ${}^{\mathrm{13}}$ C] benzoic acid was purchased from Cambridge Isotope Laboratories. The characteristics of the samples are summarized in Table . All the samples were packed in a glass tube, and the pictures of these samples are shown in the Supplement.

Table 2.

Summary of sample conditions and the optimized parameters of the DNP experiments.

Sample	Nanodiamonds	Microdiamonds	Pentacene-doped benzoic acid
Density of NV ${}^{-}$	$\mathrm{5.3}\times {\mathrm{10}}^{\mathrm{17}}$  cm ${}^{-\mathrm{3}}$ (3 ppm)	$\mathrm{8.9}\times {\mathrm{10}}^{\mathrm{17}}$  cm ${}^{-\mathrm{3}}$ (5 ppm)	–
Density of P1	$\mathrm{1.2}\times {\mathrm{10}}^{\mathrm{19}}$  cm ${}^{-\mathrm{3}}$ (70 ppm)	$\mathrm{4.6}\pm \mathrm{0.1}\times {\mathrm{10}}^{\mathrm{18}}$  cm ${}^{-\mathrm{3}}$ (26 ppm)	–
Density of pentacene	–	–	$\mathrm{2.6}\times {\mathrm{10}}^{\mathrm{18}}$  cm ${}^{-\mathrm{3}}$ (0.04 mol%)
Particle size	$\sim \mathrm{100}$  nm	$\sim \mathrm{500}$   $\mathrm{\mu }\mathrm{m}$	$<\mathrm{100}$   $\mathrm{\mu }\mathrm{m}$
$B_{\mathrm{sweep}}$ (mT)	(No DNP performed)	5	20
$t_{\mathrm{MW}}$ ( $\mathrm{\mu }\mathrm{s}$ )	(No DNP performed)	1250	30

3.2. Experimental setup

The procedure and experimental setup of triplet DNP used in this work are similar to those described by .

A solid-state laser with a wavelength of 527 nm, pulse length of 200 ns, and pulse energy of 30 mJ was used as a light source for the excitation of NV ${}^{-}$ centers, and a dye laser with a wavelength of 594 nm, pulse length of 200 ns, and pulse energy of 6 mJ was used as a light source for the excitation of pentacene. A static magnetic field from 0.3 to 0.5 T was generated by an electromagnet. All experiments were carried out at room temperature.

The EPR experiments were done with a home-built spectrometer, similar to that reported by , where an RF pulse at 400 MHz generated by an arbitrary waveform generator was converted to 11.6 GHz in a superheterodyne architecture. The microwave pulse was amplified to 1 W and led into the cavity.

4. Results and discussion

4.1. EPR measurements

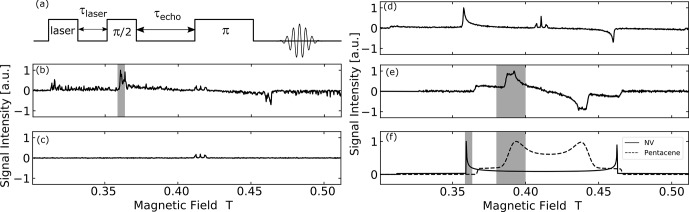
Using the pulse sequence depicted in Fig. a, magnetic-field dependencies of the amplitude of the spin echoes of the electrons in the triplet state were measured for the three samples described above.

Figure 3.

(a) EPR echo pulse sequence used in the measurement. ${\mathit{\tau }}_{\mathrm{laser}}$ is the delay time used for the lifetime decay measurement and ${\mathit{\tau }}_{\mathrm{echo}}$ is the echo time. (b–f) EPR spectra of polycrystalline samples under study obtained by experiment and simulation: (b) optically polarized EPR powder spectrum of microdiamonds with MW frequency of 11.63 GHz. (c) EPR powder spectrum of microdiamonds with MW frequency of 11.63 GHz without the laser pulse irradiation. (d) Optically polarized EPR powder spectrum of nanodiamonds with MW frequency of 11.52 GHz. (e) EPR powder spectrum of powder BA doped with 0.04 mol% pentacene with MW frequency of 11.66 GHz. (f) Simulated EPR powder spectra of thermal state triplet electron in NV ${}^{-}$ center and pentacene at 11.6 GHz. The shaded area is the magnetic field-sweep range $B_{\mathrm{sweep}}$ for each system.

The optically excited NV ${}^{-}$ spectrum of microdiamonds in Fig. b does not show a smooth polycrystalline pattern (powder spectrum) but rather a partially averaged crystalline pattern due to the average diameter of the particles around 500  $\mathrm{\mu }\mathrm{m}$ and the number of microdiamonds in the sample tube being only $\simeq \mathrm{200}$ . The echo time ${\mathit{\tau }}_{\mathrm{echo}}$ was set to 2.3  $\mathrm{\mu }\mathrm{s}$ and the measurement took 3 h.

By comparing the signal intensity with and without laser irradiation (see the Supplement), we estimate an enhancement of ca. 170. This corresponds to an electron spin polarization of ca. 16 %, derived from a thermal polarization of ca. 0.095 %.

The three lines in the center of the field (around $g=\mathrm{2}$ ) do not stem from the NV ${}^{-}$ center, but from the P1 centers and other $S=\mathrm{1}/\mathrm{2}$ electron spin defects. The hyperfine structure observed arises from the coupling of the electron spin to the adjacent ${}^{\mathrm{14}}$ N nucleus in the P1 centers. This signal is not optically polarized and is also present “in the dark” as shown in Fig. c for the case of microdiamonds.

Figure d shows the optically polarized NV ${}^{-}$ powder spectrum of the nanodiamonds. The echo time ${\mathit{\tau }}_{\mathrm{echo}}$ was set to 400 ns, and the measurement took 7 h. The line exhibited a typical dipolar powder pattern (Pake pattern), where the two “horns” are separated by the zero-field splitting parameter $D$ and the two “shoulders” by 2  $D$ . The horns come from the crystallites with such orientation that the N-V axis is perpendicular to the magnetic field, whereas the shoulders correspond to those in which the N-V axis is along the field. The result that one of the transitions is inverted is ascribed to the optical polarization mechanism, which exclusively populates the electron spins of the ground triplet state in the NV ${}^{-}$ center into the $m_{\mathrm{S}}=\mathrm{0}$ state. To the best of our knowledge, this is the first report on the full powder EPR spectrum of optically polarized nanodiamonds observed with the conventional (i.e., not optical) detection. A similar spectrum from a large size distribution of nano-/micro-diamonds (up to ca. 1.5  $\mathrm{\mu }\mathrm{m}$ ) recently showed an enhancement of ca. 6-fold . Importantly, an optically polarized NV ${}^{-}$ signal was not seen for the “dark” nanodiamond powder before the chemical surface cleaning (see the Supplement). We assume that the visible laser light was absorbed on the particle surface.

Figure e shows the powder spectrum of the photo-excited triplet state of pentacene. In the measurement, the echo time ${\mathit{\tau }}_{\mathrm{echo}}$ was set to 2.3  $\mathrm{\mu }\mathrm{s}$ and the measurement took 5 h. The shape is also a dipolar powder pattern, where the two “horns” are separated by the zero-field splitting parameter $D$ and the two “shoulders” by 2  $D$ . The zero-field splitting parameter $D$ for pentacene is only about half of that for NV ${}^{-}$ , which explains half the powder “linewidth” of pentacene compared to NV ${}^{-}$ . In the case of pentacene, the part of the “horn” is broadened due to the finite ZFS parameter $E$ , which is zero for NV ${}^{-}$ . Figure f shows EPR spectra of the thermally populated NV ${}^{-}$ center and pentacene simulated using EasySpin, a Matlab package .

4.2. Hyperpolarized ${}^{\mathrm{13}}$ C NMR

4.2.1. NV ${}^{-}$ -containing microdiamonds

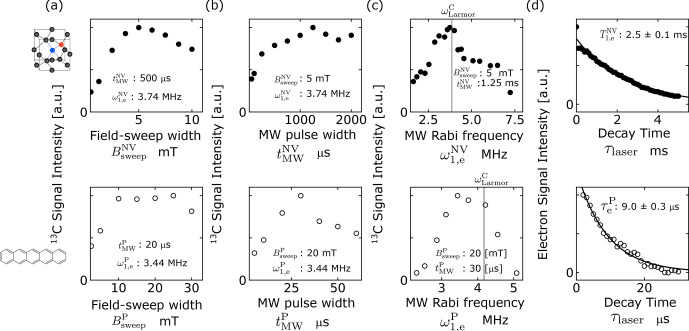
We performed hyperpolarization of the ${}^{\mathrm{13}}$ C spins in microdiamonds using the ISE pulse sequence in a magnetic field of 0.36 T, which corresponds to the position of the low-field “horn” in the spectrum of Fig. b. To find the experimental parameters that maximize the efficiency of DNP, we varied the range of the magnetic-field sweep $B_{\mathrm{sweep}}^{\mathrm{NV}}$ , the width $t_{\mathrm{MW}}^{\mathrm{NV}}$ , and the amplitude ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{NV}}$ of the microwave pulse and examined the enhanced ${}^{\mathrm{13}}$ C magnetization. As demonstrated in the upper part of Fig. a–c, the optimal conditions were found to be $B_{\mathrm{sweep}}^{\mathrm{NV}}\simeq \mathrm{5}$  mT, $t_{\mathrm{MW}}^{\mathrm{NV}}\simeq \mathrm{1250}$   $\mathrm{\mu }\mathrm{s}$ , and ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{NV}}\simeq \mathrm{3.74}$  MHz.

Figure 4.

(a–c) Optimization of the ISE pulse sequence for microdiamonds (upper row, filled circles) and pentacene-doped benzoic acid (PBA) (lower row, open circles) through adjustment of (a) the field-sweep width $B_{\mathrm{sweep}}^{\mathit{\alpha }}$ , (b) the microwave duration $t_{\mathrm{MW}}^{\mathit{\alpha }}$ , and (c) the microwave intensity ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathit{\alpha }}$ , where $\mathit{\alpha }=\mathrm{NV},\mathrm{P}$ for microdiamonds and PBA, respectively. The values of the fixed parameters are indicated in each graph. The gray lines in (c) indicate the ${}^{\mathrm{13}}$ C Larmor frequency for the magnetic field that corresponds to the low-field horn of the EPR spectrum. (d) Longitudinal relaxation (upper graph) and lifetime decay (lower graph) of the electron spins in the triplet state of NV ${}^{-}$ centers in microdiamonds and pentacene, respectively.

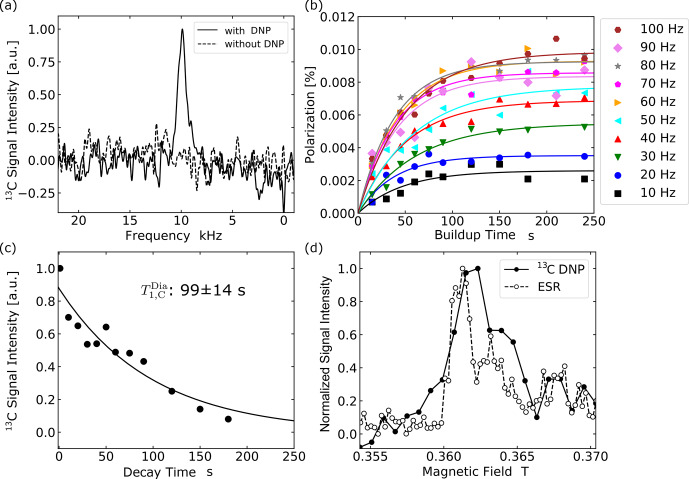
Then, adopting these parameters, we performed dynamic ${}^{\mathrm{13}}$ C polarization by repeating the ISE sequence at a rate of 100 Hz for 240 s, measured ${}^{\mathrm{13}}$ C NMR at a Larmor frequency ${\mathit{\omega }}_{\mathrm{0},\mathrm{C}}$ of 3.85 MHz, and successfully obtained a hyperpolarized ${}^{\mathrm{13}}$ C spectrum of the microdiamonds, as demonstrated in Fig. a. The result of the identical ${}^{\mathrm{13}}$ C measurement except that DNP was not performed, also plotted in Fig. a for comparison, did not show any appreciable sign of the signal above the noise level. Figure b shows buildup curves of ${}^{\mathrm{13}}$ C polarization with various ISE repetition rates $R$ ranging from 10 to 100 Hz. For $R$ of up to 60 Hz, the buildup rate and the finally attained ${}^{\mathrm{13}}$ C polarization increased with $R$ , whereas they saturated for $R>\mathrm{60}$  Hz. The maximum ${}^{\mathrm{13}}$ C polarization was 0.01 %, corresponding to 324-fold enhancement of ${}^{\mathrm{13}}$ C polarization compared to that in thermal equilibrium.

Figure 5.

Optical hyperpolarization in microdiamonds. (a) NMR spectra of ${}^{\mathrm{13}}$ C in microdiamonds averaged over four times. The solid line shows the ${}^{\mathrm{13}}$ C NMR signals with DNP and the broken line shows that without DNP. The spectrum was obtained with a laser repetition frequency of 100 Hz and 240 s of DNP buildup time. (b) Buildup curves of ${}^{\mathrm{13}}$ C polarization in microdiamonds obtained with ISE repetition rates ranging from 10 to 100 Hz. (c) The relaxation curve for the ${}^{\mathrm{13}}$ C NMR signal of microdiamonds. (d) The DNP and EPR field profile around 0.36 T.

Figure c shows the profile of ${}^{\mathrm{13}}$ C depolarization after hyperpolarization, from which the time constant $T_{\mathrm{1},\mathrm{C}}^{\mathrm{Dia}}$ of ${}^{\mathrm{13}}$ C longitudinal relaxation was determined to be $\mathrm{99}\pm \mathrm{14}$  s. Here, ${}^{\mathrm{13}}$ C relaxation is mainly caused by the P1 centers creating fluctuating local fields at the ${}^{\mathrm{13}}$ C atomic sites. Figure d compares the magnetic-field dependences of the enhanced ${}^{\mathrm{13}}$ C magnetization and of the EPR signal obtained in the microdiamond sample at around 0.36 T. The result that they showed similar profiles indicates that the underlying polarization mechanism is indeed the ISE.

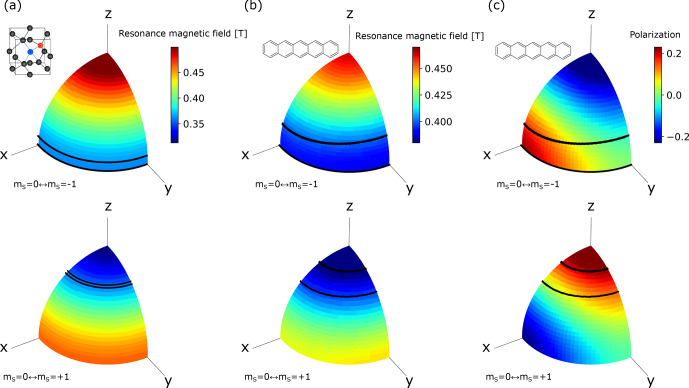
To determine the exchange probability ${\mathit{\xi }}_{\mathrm{NV}}$ appearing in Eq. () under the optimized ISE conditions, it is necessary to estimate the DNP-active spin-packet fraction ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{NV}}$ , the triplet fraction ${\mathit{\eta }}_{\mathrm{t}}^{\mathrm{NV}}$ of the NV ${}^{-}$ in the microdiamonds, and the electron polarization $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{NV}}}$ averaged over the ISE sweep time. These parameters can be extracted from the experimental powder EPR spectra shown in Fig.  and the calculated orientational dependence of the resonance magnetic field shown in Fig. . The optimized range of the magnetic-field sweep, indicated by the shaded areas in Fig. b and f, corresponds to the region between the black lines in Fig. a. From the area of this region compared to that of the whole solid angle, the active spin packet fraction ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{NV}}$ was estimated to be 0.204.

Figure 6.

Orientational dependence of the resonant magnetic field for the electron spins in the triplet state of (a) the NV ${}^{-}$ center and (b) pentacene doped in benzoic acid at a microwave frequency of 11.66 GHz. The $x$ , $y$ , and $z$ axes represent the principal axes of the ZFS tensor. (c) Orientational dependence of the polarization of the electron spins in the photo-excited triplet state of pentacene doped in benzoic acid in 0.39 T calculated from the zero-field population . The upper and lower rows correspond to the EPR transitions between the $m_S=+\mathrm{1}$ and $m_S=\mathrm{0}$ states and between the $m_S=\mathrm{0}$ and $m_S=-\mathrm{1}$ states, respectively. The regions between the black lines correspond to the orientations for which the crystallites experience polarization transfer by the ISE using the optimized field-sweep range found in the experiments demonstrated in Fig. .

Having estimated the electron polarization $P_{\mathrm{e}}^{\mathrm{NV}}$ of the NV ${}^{-}$ center between the $m_{\mathrm{S}}=\mathrm{0}$ and $m_{\mathrm{S}}=-\mathrm{1}$ states to be 16 %, we then obtained the triplet fraction ${\mathit{\eta }}_t^{\mathrm{NV}}=\mathrm{2}/(\mathrm{3}-P_{\mathrm{e}}^{\mathrm{NV}})=\mathrm{0.7}$ . From the measured longitudinal relaxation time $T_{\mathrm{1},\mathrm{e}}^{\mathrm{NV}}$ of 2.5 ms (Fig. d), we obtained the averaged electron polarization $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{NV}}}$ over the optimal pulse width $t_{\mathrm{MW}}^{\mathrm{NV}}=\mathrm{1250}$   $\mathrm{\mu }\mathrm{s}$ as 0.125.

In evaluating the exchange probability ${\mathit{\xi }}_{\mathrm{NV}}$ , it is important to use such experimental data as were taken under the rapid-diffusion regime that validates Eq. (4). Accordingly, the buildup curve with the ISE repetition rate of 10 Hz, which was rather low and not suitable for attaining the highest polarization, was adopted for the purpose of data fitting to Eq. (4). From the final polarization $P_{\mathrm{fin}}=\mathrm{0.0026}$  %, the time constant $T_{\mathrm{b}}=\mathrm{47.4}$  s of the buildup curve at the repetition rate $R$ of 10 Hz, the electron polarization averaged over the ISE sweep time $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{NV}}}\simeq \mathrm{0.125}$ , the active spin-packet fraction ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{NV}}\simeq \mathrm{0.204}$ , the triplet fraction ${\mathit{\eta }}_{\mathrm{t}}^{\mathrm{NV}}\simeq \mathrm{0.7}$ , the density of triplet electrons in the microdiamonds ${\mathit{\rho }}_{\mathrm{e}}^{\mathrm{NV}}\simeq \mathrm{8.9}\times {\mathrm{10}}^{\mathrm{17}}$  cm ${}^{-\mathrm{3}}$ , and the ${}^{\mathrm{13}}$ C spin density ${\mathit{\rho }}_{\mathrm{C}}^{\mathrm{Dia}}\simeq \mathrm{1.9}\times {\mathrm{10}}^{\mathrm{21}}$  cm ${}^{-\mathrm{3}}$ , we obtained the exchange probability ${\mathit{\xi }}_{\mathrm{NV}}$ as 0.0067.

4.2.2. Pentacene-doped benzoic acid

We also successfully hyperpolarized the ${}^{\mathrm{13}}$ C spins in PBA through direct electron-to- ${}^{\mathrm{13}}$ C polarization transfer by the ISE. Our results contrast with the previous works that relied on prior ${}^{\mathrm{1}}$ H polarization followed by ${}^{\mathrm{1}}\mathrm{H}{-}^{\mathrm{13}}\mathrm{C}$ cross-polarization . The lower parts of Fig. a–c show the dependence of the efficiency of dynamic ${}^{\mathrm{13}}$ C polarization by the ISE on the field-sweep width $B_{\mathrm{sweep}}^{\mathrm{P}}$ , the ISE duration $t_{\mathrm{MW}}^{\mathrm{P}}$ , and the microwave intensity ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{P}}$ , experimentally examined at 0.39 T, which corresponds to the EPR at the position where the low-field “horn” appears in the spectrum of Fig. e. The optimal conditions were found to be $B_{\mathrm{sweep}}^{\mathrm{P}}\simeq \mathrm{20}$  mT, $t_{\mathrm{MW}}^{\mathrm{P}}\simeq \mathrm{30}$   $\mathrm{\mu }\mathrm{s}$ , and ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{P}}\simeq \mathrm{3.44}$  MHz.

The optimal values for the field-sweep width $B_{\mathrm{sweep}}^{\mathrm{P}}$ and the microwave intensity ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{P}}$ differed from those ( $B_{\mathrm{sweep}}^{\mathrm{NV}}$ , ${\mathit{\omega }}_{\mathrm{1},\mathrm{e}}^{\mathrm{NV}}$ ) in the case of the microdiamond sample just by small factors, whereas the optimal microwave-pulse width $t_{\mathrm{MW}}^{\mathrm{P}}$ was shorter than $t_{\mathrm{MW}}^{\mathrm{NV}}$ by more than an order of magnitude. Such a striking difference can be ascribed to the sources of polarization for PBA being the electron spins in the metastable, photo-excited triplet state of pentacene, while those for the diamond sample are the electron spins in the persistent, ground triplet state. For the PBA sample, the contact time is limited by the lifetime of the photo-excited triplet state of pentacene, whereas for the microdiamond sample it is by the electron spin-lattice relaxation time $T_{\mathrm{1}\mathit{\rho }}$ in the rotating frame. As we shall discuss below, it is not those ${}^{\mathrm{13}}$ C spins adjacent to the NV ${}^{-}$ centers but those located at a moderate distance that need to receive the polarization of the electron spins of the NV ${}^{-}$ center, in order for the enhanced polarization to be eventually transported away by ${}^{\mathrm{13}}$ C spin diffusion. Since the interaction between such ${}^{\mathrm{13}}$ C spins and the NV ${}^{-}$ centers is expected to be relatively weak, direct polarization transfer by the ISE needs to be performed for a relatively longer time duration, as long as the electron magnetization is retained along the effective field in the rotating frame.

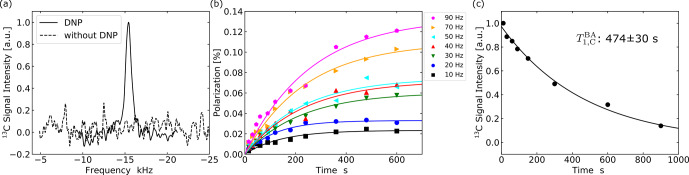
${}^{\mathrm{13}}$ C NMR measurements were performed at 4.19 MHz. An enhanced ${}^{\mathrm{13}}$ C NMR spectrum, in comparison with that obtained without performing the ISE, is demonstrated in Fig. a. Figure b shows ${}^{\mathrm{13}}$ C polarization buildup behaviors for various ISE repetition frequencies. With the highest experimentally feasible ISE repetition rate $R$ of 90 Hz, the ${}^{\mathrm{13}}$ C polarization finally reached 0.12 %, i.e., $\sim \mathrm{3600}$ times the thermal ${}^{\mathrm{13}}$ C polarization. The ${}^{\mathrm{13}}$ C longitudinal relaxation time $T_{\mathrm{1},\mathrm{C}}^{\mathrm{BA}}$ was determined to be $\mathrm{474}\pm \mathrm{30}$  s (Fig. c). We estimated the active spin-packet fraction ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{PBA}}$ and the triplet fraction ${\mathit{\eta }}_{\mathrm{t}}^{\mathrm{P}}$ of pentacene in a similar way to the case of the microdiamond sample. From comparison of the optimal magnetic-field sweep range $B_{\mathrm{sweep}}$ indicated by the shaded region in Fig. e and f with the area between the black lines in Fig. b and c, ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{PBA}}$ was estimated to be 0.565. The average electron polarization $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{P}}}$ between the two triplet sublevels over the field-sweep time was estimated using the populations over the zero-field eigenstates of the triplet state of pentacene doped in benzoic acid, which is known to be $P_x:P_y:P_z=\mathrm{0.44}:\mathrm{0.34}:\mathrm{0.22}$ . Assuming that the external magnetic field is nearly perpendicular to the $z$  axis of the principal axis system of the ZFS tensor for the relevant electron-spin packets, we calculated the populations over the triplet sublevels in the magnetic field and obtained $P_{\mathrm{e}}^{\mathrm{P}}=\mathrm{0.105}$ and ${\mathit{\eta }}_{\mathrm{t}}^{\mathrm{P}}=\mathrm{2}/(\mathrm{3}-P_{\mathrm{e}}^{\mathrm{P}})=\mathrm{0.69}$ . Then, taking account of the lifetime decay with the time constant ${\mathit{\tau }}_{\mathrm{e}}^{\mathrm{P}}=\mathrm{9}$   $\mathrm{\mu }\mathrm{s}$ (lower row of Fig. d), we determined $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{P}}}$ to be 0.03.

Figure 7.

(a)  ${}^{\mathrm{13}}$ C spectra of PBA with and without DNP. The enhanced spectrum (solid line) was obtained after performing the ISE sequence at a repetition frequency of 90 Hz for 600 s. (b) Buildup curves of ${}^{\mathrm{13}}$ C polarization in PBA for ISE repetition rates ranging from 10 to 90 Hz. Each data point represents the ${}^{\mathrm{13}}$ C polarization derived from the intensity of the NMR signal accumulated over 16 times. (c) Dependence of the ${}^{\mathrm{13}}$ C magnetization in PBA on the time interval between DNP and NMR measurement.

Using Eq. (4), we performed curve fitting of the buildup data experimentally obtained with the ISE repetition rate $R$ of 10 Hz, at which the rapid-diffusion limit is expected to be valid, and obtained $P_{\mathrm{fin}}^{\mathrm{PBA}}(R=\mathrm{10}\mathrm{Hz})=\mathrm{0.023}$  % and $T_{\mathrm{b}}^{\mathrm{PBA}}(R=\mathrm{10}\mathrm{Hz})=\mathrm{141}$  s, whence, with $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{P}}}\simeq \mathrm{0.03}$ , ${\mathit{\eta }}_{\mathrm{p}}^{\mathrm{PBA}}\simeq \mathrm{0.535}$ , ${\mathit{\eta }}_{\mathrm{t}}^{\mathrm{P}}\simeq \mathrm{0.69}$ , ${\mathit{\rho }}_{\mathrm{e}}^{\mathrm{P}}\simeq \mathrm{2.6}\times {\mathrm{10}}^{\mathrm{18}}$  cm ${}^{-\mathrm{3}}$ , and ${\mathit{\rho }}_{\mathrm{C}}^{\mathrm{PBA}}\simeq \mathrm{6.26}\times {\mathrm{10}}^{\mathrm{21}}$  cm ${}^{-\mathrm{3}}$ , we determined ${\mathit{\xi }}_{\mathrm{PBA}}$ to be 0.035.

For the microdiamond and PBA samples, the exchange probabilities were found to be ${\mathit{\xi }}_{\mathrm{PBA}}=\mathrm{0.035}$ and ${\mathit{\xi }}_{\mathrm{NV}}=\mathrm{0.0067}$ , respectively. To account for such a significant difference in the probability of the spin states being transferred between the electron and the ${}^{\mathrm{13}}$ C spins in a single shot of the ISE sequence, we again note the different types of the sources of polarization. In the case of the microdiamond sample, the relevant electron spins are in the ground triplet state, persistently causing significant local fields at the ${}^{\mathrm{13}}$ C sites in the vicinity. They would create the ${}^{\mathrm{13}}$ C spin-diffusion barriers , in which the ${}^{\mathrm{13}}$ C spins would not be able to transport their polarization to other ${}^{\mathrm{13}}$ C spins via the mutual spin flip-flop process. Unfortunately, those ${}^{\mathrm{13}}$ C spins that are most likely to receive spin polarization from the NV ${}^{-}$ centers are inside the barrier and thus are least likely to distribute the enhanced polarization away.

Nevertheless, the experimental result that the bulk enhancement of the ${}^{\mathrm{13}}$ C polarization in the microdiamonds was indeed realized indicates the presence of those ${}^{\mathrm{13}}$ C spins in the sample that are capable of both receiving the polarization from the NV ${}^{-}$ center and passing it over other ${}^{\mathrm{13}}$ C spins through spin diffusion. Such ${}^{\mathrm{13}}$ C spins ought to be located at a moderate distance from the NV ${}^{-}$ center just outside the barrier. That is, if the ${}^{\mathrm{13}}$ C spins are too close to the NV ${}^{-}$ center, the interaction with the electron spin would overwhelm the dipolar interaction among the ${}^{\mathrm{13}}$ C spins, hindering the flip-flop transitions between the ${}^{\mathrm{13}}$ C spins. The relatively long distance between such mediating ${}^{\mathrm{13}}$ C spins and the NV ${}^{-}$ centers would result in the low exchange probability ${\mathit{\xi }}_{\mathrm{NV}}$ .

Conversely, the ${}^{\mathrm{13}}$ C spins in PBA do not suffer from the local fields produced by the electron spins in the triplet state for most of the time during the buildup experiment, because of the transient nature of the excited triplet state. Indeed, the lifetime of the triplet scale, found to be ca.  $\mathrm{9}$   $\mathrm{\mu }\mathrm{s}$ (Fig. d), is 3 orders of magnitude shorter than the time interval between the ISE sequence even with its highest feasible repetition rate. For the ${}^{\mathrm{13}}$ C spins located relatively close to the pentacene molecules, the probability of receiving the polarization from the electron spins in the triplet state is expected to be relatively high. Even if they ought to temporarily feel the strong local field and are trapped inside the spin-diffusion barrier during the photo-excitation cycles, they are allowed to undergo spin diffusion for most of the time when the pentacene molecules are in the ground, diamagnetic state. Hence, the exchange probability is expected to be higher.

4.3. ${}^{\mathrm{13}}$ C spin diffusion

4.3.1. In diamond

In addition to the efficiency $\mathit{\xi }$ of direct polarization transfer from the electron spins in the triplet state to the ${}^{\mathrm{13}}$ C spins, spin diffusion among the ${}^{\mathrm{13}}$ C spins is another important factor that affects the overall enhancement of polarization. Spin diffusion is driven by the flip-flop component of homonuclear dipolar interactions, and in turn, the dipolar interaction is determined by the geometrical configuration of the relevant spins. It follows that spin diffusion is characterized by the geometrical configuration. According to Lowe and Gade , the $(\mathit{\alpha },\mathit{\beta })$ -component $(\mathit{\alpha },\mathit{\beta }=x,y,z)$ of the spin-diffusion tensor ${\mathcal{D}}_{\mathit{\alpha }\mathit{\beta }}$ is given by

$${\mathcal{D}}_{\mathit{\alpha }\mathit{\beta }}={\displaystyle \frac{\sqrt{\mathit{\pi }}}{\mathrm{2}}}\sum _i{A}_{\mathrm{0}i}{\mathit{\alpha }}_{\mathrm{0}i}{\mathit{\beta }}_{\mathrm{0}i}{\mathrm{\Delta }}_{\mathrm{0}i}^{-\frac{\mathrm{1}}{\mathrm{2}}}.$$ 7

Here, $\mathrm{0}$ in the subscripts represents one of the ${}^{\mathrm{13}}$ C sites arbitrarily chosen to be the origin of the coordinate system, and the sum is taken over all ${}^{\mathrm{13}}$ C sites. ${\mathit{\alpha }}_{ij}\left({\mathit{\beta }}_{ij}\right)$ is the $\mathit{\alpha }\left(\mathit{\beta }\right)$ component of the internuclear vector ${\mathit{r}}_{ij}$ between sites $i$ and $j$ . $A_{ij}$ and ${\mathrm{\Delta }}_{ij}$ are expressed as

$$\begin{array}{}\text{8}& {\displaystyle }{A}_{ij}=\left({\displaystyle \frac{{\mathit{\mu }}_{\mathrm{0}}}{\mathrm{4}\mathit{\pi }}}\right)\cdot \left(-{\displaystyle \frac{\mathrm{1}}{\mathrm{4}}}\right){\mathit{\gamma }}^{\mathrm{2}}\mathrm{\hslash }{r}_{ij}^{-\mathrm{3}}\left(\mathrm{1}-\mathrm{3}{\cos }^{\mathrm{2}}{\mathit{\theta }}_{ij}\right),\text{9}& {\displaystyle }{\mathrm{\Delta }}_{\mathrm{0}i}={\displaystyle \frac{\mathrm{1}}{\mathrm{2}}}\sum _{j\ne i}{\left(B_{\mathrm{0}j}-B_{ij}\right)}^{\mathrm{2}},\text{10}& {\displaystyle }{B}_{ij}=-\mathrm{2}{A}_{ij},\end{array}$$

where ${\mathit{\mu }}_{\mathrm{0}}=\mathrm{4}\mathit{\pi }\times {\mathrm{10}}^{-\mathrm{7}}$  Hm ${}^{-\mathrm{1}}$ is the vacuum permeability, $\mathit{\gamma }$ is the gyromagnetic ratio of ${}^{\mathrm{13}}$ C, and ${\mathit{\theta }}_{ij}$ is the angle between the internuclear vector ${\mathit{r}}_{ij}$ and the external static field.

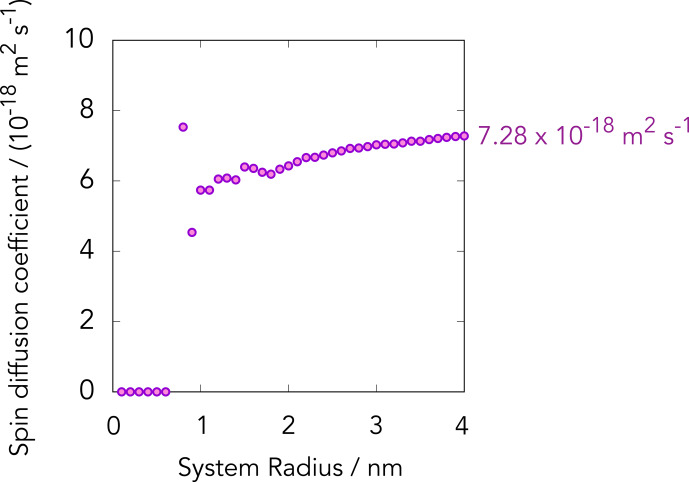
To estimate the ${}^{\mathrm{13}}$ C spin-diffusion coefficient in the diamond sample outside the diffusion barrier where the effect of the hyperfine couplings is negligibly small, we made a table of vectors representing the carbon atomic sites in diamond. We then randomly picked up the ${}^{\mathrm{13}}$ C sites assuming the natural abundance of 1 %. Using the Lowe–Gade formula, we calculated the isotropic spin-diffusion coefficient $D=({\mathcal{D}}_{xx}+{\mathcal{D}}_{yy}+{\mathcal{D}}_{zz})/\mathrm{3}$ by taking the ${}^{\mathrm{13}}$ C sites inside the spherical region with various radii into account. As shown in Fig. , the calculated value of $\mathcal{D}$ increased with the size of the region that we considered and asymptotically converged to $\mathrm{7.28}\times {\mathrm{10}}^{-\mathrm{18}}$  m ${}^{\mathrm{2}}$  s ${}^{-\mathrm{1}}$ .

Figure 8.

${}^{\mathrm{13}}$ C spin-diffusion coefficients calculated for diamond crystal using the Lowe–Gade formula (Eq. ) by taking account of naturally abundant ${}^{\mathrm{13}}$ C sites within spherical regions with various radii; 750 ${}^{\mathrm{13}}$ C sites are involved within the sphere with a radius of 4 nm.

4.3.2. In PBA

Unlike the case of diamond, where all atomic sites other than ${}^{\mathrm{13}}$ C are magnetically inert, estimation of the ${}^{\mathrm{13}}$ C spin-diffusion coefficient in PBA by the Lowe–Gade formula cannot be made in a straightforward way, because of the presence of the abundant ${}^{\mathrm{1}}$ H spins causing the considerable dipolar fields at the ${}^{\mathrm{13}}$ C sites. The effect of the ${}^{\mathrm{1}}\mathrm{H}{-}^{\mathrm{13}}\mathrm{C}$ dipolar interaction is to lift up the degeneracy in the energy levels of the ${}^{\mathrm{13}}$ C spin packets, so that the flip-flop process among the ${}^{\mathrm{13}}$ C spins tends to not conserve the energy. Thus, the ${}^{\mathrm{13}}$ C diffusion rate ought to be slower than in the case if it were not for the proton spins.

In this work, we estimated the ${}^{\mathrm{13}}$ C spin-diffusion coefficient in [carboxyl- ${}^{\mathrm{13}}$ C] benzoic acid from the experimental repetition-rate dependence of the initial buildup rate of the ${}^{\mathrm{13}}$ C polarization . With relatively low ISE repetition rates for which the rapid-diffusion condition is valid, the buildup rate is proportional to the repetition rate. As the repetition rate is increased, spin diffusion would no longer be able to transport the polarization completely during the time interval of ISE repetition, and the repetition-rate dependence of the buildup rate begins to saturate. The saturation of the initial buildup rate of ${}^{\mathrm{13}}$ C polarization was observed in the case of PBA (Fig. a). Since the initial buildup rate is independent of relaxation, one can estimate the spin-diffusion rate from the data plotted in Fig. a without having to take the effect of the former into account.

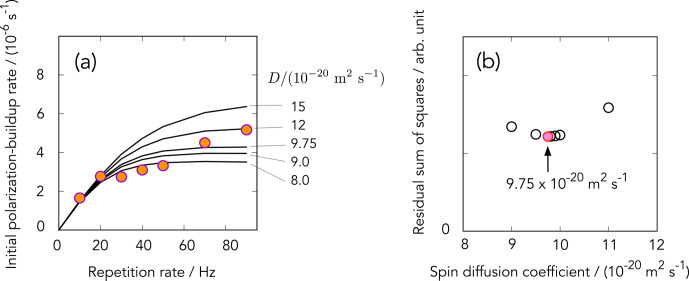
Figure 9.

(a) Dependence of the initial buildup rate as a function of the ISE repetition rate $R$ obtained for the PBA sample. The data points indicated by the circles were obtained from the slopes of the buildup curves shown in Fig. b at time zero. Solid lines represent the $R$ dependence simulated for various spin-diffusion coefficients $\mathcal{D}$ according to the model described in the text. (b) A plot of the residual sum of squares calculated for various spin-diffusion coefficients, which gave the minimum for $\mathcal{D}=\mathrm{9.75}\times {\mathrm{10}}^{-\mathrm{20}}$  m ${}^{\mathrm{2}}$  s ${}^{-\mathrm{1}}$ .

In order to reproduce the profile of the experimentally obtained initial buildup rate that increased with the repetition rate $R$ and exhibited saturation at relatively higher values of $R$ , we considered a cubic region with a side length of 10.4 nm, which includes one single pentacene molecule on average, and supposed that the position-dependent ${}^{\mathrm{13}}$ C polarization evolves in time according to the diffusion equation with a periodic boundary condition. We also assumed that the timescale of spin diffusion is much longer than the time interval $t_{\mathrm{MW}}$ of the ISE sequence, so that the point source inside the cubic region instantly creates the ${}^{\mathrm{13}}$ C polarization $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}^{\mathrm{P}}}=\mathrm{0.030}$ at each moment when the ISE sequence is implemented, with a probability ${\mathit{\xi }}_{\mathrm{PBA}}=\mathrm{0.035}$ .

The profiles of numerically simulated time evolution of the net polarization for various spin-diffusion coefficients $\mathcal{D}$ , ranging from $\mathrm{8.0}\times {\mathrm{10}}^{-\mathrm{20}}$ to $\mathrm{1.5}\times {\mathrm{10}}^{-\mathrm{19}}$  m ${}^{\mathrm{2}}$  s ${}^{-\mathrm{1}}$ , are plotted in Fig. a. We found that $\mathcal{D}=\mathrm{9.75}\times {\mathrm{10}}^{-\mathrm{20}}$  m ${}^{\mathrm{2}}$  s ${}^{-\mathrm{1}}$ is the most likely value for the ${}^{\mathrm{13}}$ C spin-diffusion coefficient that minimized the residual sum of squares in the case of our ${}^{\mathrm{13}}$ C-labeled benzoic acid sample (Fig. b).

Interestingly, the ${}^{\mathrm{13}}$ C spin-diffusion coefficient in PBA was estimated to be 2 orders of magnitude smaller than that in diamond, despite the ${}^{\mathrm{13}}$ C enrichment in the former sample. This is ascribed to the presence of the ${}^{\mathrm{1}}$ H spins that slow down the flip-flop process between the ${}^{\mathrm{13}}$ C spins in PBA and to the dense packing of the carbon atoms in the diamond crystal. From the DNP point of view, ${}^{\mathrm{13}}$ C spin diffusion should be as fast as possible. One way to make it is to continuously apply ${}^{\mathrm{1}}$ H decoupling throughout the buildup experiment . This strategy, however, is not practical because of the complexity of the hardware that realizes simultaneous application of radiofrequency and microwave irradiation and of the serious heating of the circuit. Deuteration of the sample can be an alternative way to make ${}^{\mathrm{13}}$ C spin diffusion faster in PBA.

4.4. The behavior of ${}^{\mathrm{13}}$ C polarization buildup

It is the contribution of both the direct polarization transfer from the electrons in the triplet state to the ${}^{\mathrm{13}}$ C spins and ${}^{\mathrm{13}}$ C spin diffusion that eventually leads to bulk ${}^{\mathrm{13}}$ C hyperpolarization. In reality, spin-lattice relaxation tends to drag the spin system back toward thermal equilibrium. The balance between the buildup and relaxation processes determines the profile of the ${}^{\mathrm{13}}$ C polarization-buildup curve, the finally attainable bulk ${}^{\mathrm{13}}$ C polarization $P_{\mathrm{fin}}$ , and the time required to attain $P_{\mathrm{fin}}$ .

The ${}^{\mathrm{13}}$ C longitudinal relaxation time $T_{\mathrm{1},\mathrm{C}}^{\mathrm{Dia}}$ for the microdiamond sample was $\mathrm{99}\pm \mathrm{14}$  s, whereas $T_{\mathrm{1},\mathrm{C}}^{\mathrm{BA}}=\mathrm{474}\pm \mathrm{30}$  s for the PBA sample was longer than the former by a factor of ca. 5. The presence/absence of the paramagnetic electrons in the dark state can be a factor making the difference in the relaxation time. Table  summarizes the exchange probability $\mathit{\xi }$ , ${}^{\mathrm{13}}$ C spin-diffusion coefficient $\mathcal{D}$ , and the spin-lattice relaxation time $T_{\mathrm{1},\mathrm{C}}$ . As discussed above, in PBA, the average electron polarization $\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}$ and the ${}^{\mathrm{13}}$ C spin-diffusion coefficient were found to be lower than those in microdiamonds. Nevertheless, the higher exchange probability ${\mathit{\xi }}_{\mathrm{PBA}}$ and longer longitudinal relaxation time $T_{\mathrm{1},\mathrm{C}}$ more than compensate for the lower electron polarization and the slower ${}^{\mathrm{13}}$ C spin diffusion, resulting in the higher final ${}^{\mathrm{13}}$ C polarization.

Table 3.

Comparison of parameters of the DNP experiment.

Sample	${}^{\mathrm{13}}$ C in microdiamond	${}^{\mathrm{13}}$ C in PBA
$P_{\mathrm{fin}}$ (%)	0.01	0.12
$T_{\mathrm{1},\mathrm{C}}$ (min)	1.65	7.9
$\mathit{\xi }$ (%)	0.67	3.5
${\mathit{\eta }}_{\mathrm{p}}$	0.20	0.54
${\mathit{\eta }}_{\mathrm{t}}$	0.70	0.69
$\stackrel{\mathrm{‾}}{P_{\mathrm{e}}}$ (%)	12.5	3.0
${\mathit{\rho }}_{\mathrm{n}}/{\mathit{\rho }}_{\mathrm{e}}$	2200	2400
$R$ (Hz)	100	90
$\mathcal{D}$ (m ${}^{\mathrm{2}}$  s ${}^{-\mathrm{1}}$ )	$\mathrm{7.28}\times {\mathrm{10}}^{-\mathrm{18}}$	$\mathrm{9.75}\times {\mathrm{10}}^{-\mathrm{20}}$

In order to examine the possibility that the spin polarization is leaking into the proton spin system in PBA, we implemented the ISE sequence in PBA with the parameters that we found to be optimal for polarizing the ${}^{\mathrm{13}}$ C spins and then examined whether the ${}^{\mathrm{1}}$ H magnetization was enhanced. We found that the ${}^{\mathrm{1}}$ H polarization was indeed built up to 0.16 % (not shown), higher than the final ${}^{\mathrm{13}}$ C polarization. Thus, deuteration of the sample, also suggested above regarding the acceleration of ${}^{\mathrm{13}}$ C spin diffusion, would improve the efficiency of dynamic ${}^{\mathrm{13}}$ C polarization using the electron spin in the photo-excited triplet state without being mediated by the ${}^{\mathrm{1}}$ H spins.

Since only a part of the broad EPR lines is excited in triplet DNP in the polycrystalline samples, the buildup of nuclear polarization takes place only in those crystallites that happen to be oriented in such a way that the resonant field is within the region of the adiabatic sweep. In other words, other crystallites with their resonant field outside the region of the adiabatic sweep would not experience nuclear polarization. The size of the crystallites is such that spin diffusion across the boundaries of adjacent crystallites is negligibly slow. It follows that those inert crystallites continue to be inert, and polarization speckle would develop in the sample. In this respect, the relatively compact EPR powder spectrum of pentacene compared to the broader NV ${}^{-}$ powder spectrum is an advantage for the former, as the larger number of the crystallites can be excited. We estimate that the fraction of the crystallites contributing to the DNP process is 0.21 for microdiamonds and 0.565 for PBA. Considering this, the local ${}^{\mathrm{13}}$ C polarization within the DNP-active crystallites approaches 0.05 % for microdiamonds and 0.21 % for PBA, whereas the ${}^{\mathrm{13}}$ C NMR transitions in the other inert crystallites remain unpolarized.

4.5. Summary and prospects

We have demonstrated room-temperature optical ${}^{\mathrm{13}}$ C DNP using the NV ${}^{-}$ centers and the pentacene molecules. The ${}^{\mathrm{13}}$ C polarization was successfully built up in microdiamonds to 0.01 % and in PBA to 0.12 %. The difference between these polarizations is due to a multitude of favoring/disfavoring factors.

In the diamond sample, the contact time $t_{\mathrm{MW}}$ in the ISE sequence can be set much longer than that in PBA, thanks to the persistent nature of the ground triplet state of the NV ${}^{-}$ center. However, the permanent nature of the paramagnetic NV ${}^{-}$ system turns into a disadvantage for the next, spin-diffusion step: paramagnetic electrons of the NV ${}^{-}$ centers cause a significant local field around the ${}^{\mathrm{13}}$ C spins in the vicinity, creating spin-diffusion barriers around them. Unfortunately, those ${}^{\mathrm{13}}$ C spins that are most likely to be directly polarized are inside the barrier, so that they are least likely to distribute the polarization away outside the barrier. The ${}^{\mathrm{13}}$ C spins just outside the barrier can both receive the electron polarization and undergo spin diffusion. However, their relatively long distance to the NV ${}^{-}$ center leads to the low exchange probability ${\mathit{\xi }}^{\mathrm{NV}}\simeq \mathrm{0.0067}$ . Moreover, many other paramagnetic electron defects in diamond, such as P1 centers, play the same detrimental role in the efficient spread of the nuclear polarization.

The PBA system is free from the problem of diffusion barriers due to the transient nature of the paramagnetic electrons in the triplet state, which decays to the “dark”, diamagnetic, ground state within the lifetime on the order of several microseconds. Even though the contact time is limited by the rather short lifetime, the efficiency of the direct, electron-to- ${}^{\mathrm{13}}$ C polarization transfer was found to be higher than that for the case of the microdiamond sample.

However, the effect of abundant ${}^{\mathrm{1}}$ H spins reduces both the efficiency of the direct polarization transfer and of ${}^{\mathrm{13}}$ C spin diffusion. Even though one carefully optimizes the microwave amplitude in favor of the ${}^{\mathrm{13}}$ C spins, a considerable amount of electron polarization was found to leak to the ${}^{\mathrm{1}}$ H spins.

In addition, ${}^{\mathrm{1}}\mathrm{H}{-}^{\mathrm{13}}\mathrm{C}$ dipolar broadening (see the Supplement) lifts up the degeneracy in the energy levels of the ${}^{\mathrm{13}}$ C spin packets, reducing the ${}^{\mathrm{13}}$ C spin-diffusion rate and thereby the buildup of overall ${}^{\mathrm{13}}$ C polarization. The ${}^{\mathrm{13}}$ C spin-diffusion coefficient in PBA evaluated from the $R$ dependence of the initial buildup rate in comparison with numerical simulation was found to be 2 orders of magnitude lower than the spin-diffusion coefficient for the naturally abundant ${}^{\mathrm{13}}$ C spins in diamond.

The optical bulk DNP experiments in NV ${}^{-}$ -containing diamonds reported so far all used continuous-wave laser light sources, whereas we have demonstrated a pulsed optical excitation for the first time. The optical polarization of NV ${}^{-}$ centers in microdiamonds was 16 %. The polarization of the electrons in the ground triplet state created through the pulsed photo-excitation can be lower than that obtained through continuous photo-excitation, in particular when the pulse length is shorter than the decay time of the meta-stable singlet state ${}^{\mathrm{1}}E$ to the triplet ground state ${}^{\mathrm{3}}{A}_{\mathrm{2}}$ , which is ca. 300–400 ns (see Fig. ). On the other hand, the pulsed excitation can be beneficial in preventing NV ${}^{-}$ from photo-ionization to NV ${}^{\mathrm{0}}$ ( $S=\mathrm{1}/\mathrm{2}$ ) .

How could we improve optical DNP experiments, with these results in mind? showed a smart way to strongly increase the number of excited crystallites, by choosing a low magnetic field, where the total broadening is $<\mathrm{2}D$ (a range where the Zeeman term is smaller than the ZFS term) and where a ${}^{\mathrm{13}}$ C polarization of 0.25 % (detected in a high-field NMR spectrometer) was achieved . A direct comparison of the results is practically impossible, due to differences in diamond samples (varying concentrations in ${}^{\mathrm{13}}$ C, NV ${}^{-}$ , and P1 centers) . It would be interesting to repeat DNP experiments of both samples in this low-field setup. At higher fields, a frequency-swept (in contrast to our field-swept) version combined with ultra-wide bandwidth MW chirp pulses could increase the number of excited crystallites. Also, DNP schemes using the double-quantum transition could enhance the polarization by exciting more crystallites at different orientations . From the diamond engineering side, highly pure crystals with a low concentration of paramagnetic defects (P1 centers and others) would be desirable, extending both electron and nuclear spin relaxation times. Diamonds fabricated using chemical vapor deposition (CVD) may fulfill this criterion, albeit at a very high price. We could not perform DNP experiments on our 100 nm nanodiamonds, since the sample amount was too low. The best DNP enhancement for 100 nm nanodiamonds reported in the literature is only $\mathit{ϵ}=\mathrm{3}$ (compared with the thermal signal intensity at 7 T) . The difficulty in achieving DNP in real nano-particles is directly linked to the drastic reduction of nuclear longitudinal relaxation times $T_{\mathrm{1},\mathrm{C}}$ , finally arriving at values shorter than 1 s for 5 nm nanodiamonds . Methods to eliminate paramagnetic surface defects, known as dangling bonds, as well as ways to transfer the polarization outside the diamond crystals , are yet to be experimentally demonstrated.

In this respect, pentacene-doped organic host crystals can highly compete; ${}^{\mathrm{1}}$ H spins in 150 nm size crystals of pentacene in $p$ -terphenyl were optically polarized at room temperature in water, achieving a polarization of 0.086 % . Optically hyperpolarized benzoic acid crystals doped with pentacene were dissolved and a DNP-enhanced liquid-state ${}^{\mathrm{1}}$ H NMR signal of benzoic acid was measured . These examples show impressive advantages on the material side of organic crystals with a controlled pentacene doping. However, organic crystals suffer from melting or damage when they are irradiated with high-power laser beams, whereas this is not a big problem for diamonds. The quest for new defects with ODMR signals, which has just started, will also lead to a variety of new candidates for optical triplet DNP . The field of semiconductor spintronics presents a rich source of inspiration . Hopefully, our comparative approach serves as a guideline to select or even engineer improved systems for room-temperature hyperpolarization of dilute/low- $\mathit{\gamma }$ spins.

Data availability

Experimental data are available upon request from the corresponding authors.

Author contributions

KoMi, TFS, and MN designed the experiment and KoMi carried out the experiments under the supervision of TFS, AK, MK, and MN. SO, TO, HT, ST, FTKS, DT, RI, and MS prepared nanodiamonds containing NV centers. IO, HA, and NM prepared microdiamonds containing NV centers. YW and KT performed simulations estimating diffusion coefficients. AIS estimated the electron spin concentrations of the nanodiamonds from CW EPR spectra. KoMo estimated the P1 center concentration of the microdiamonds under the supervision of IO and NM. KoMi, TFS, KT, and MN analysed and interpreted the data and KoMi, TFS, KT, and MN wrote the manuscript with input from all the authors.

Competing interests

The authors declare that they have no conflict of interest.

Special issue statement

This article is part of the special issue “Geoffrey Bodenhausen Festschrift”. It is not associated with a conference.

Review statement

This paper was edited by Konstantin Ivanov and reviewed by Jeffrey Reimer and one anonymous referee.