Abstract
Group-structured models often explain the evolution of prosocial activities in terms of selection acting at both individual and group levels. Such models do not typically consider how individuals' behaviours may have consequences beyond the boundaries of their groups. However, many behaviours affect global environmental variables, including climate change and ecosystem fragility. Against this background, we propose a simple model of multi-level selection in the presence of global externalities. In our model, group members can cooperate in a social dilemma with the potential for group-level benefits. The actions of cooperators also have global consequences, which can be positive (a global good) or negative (a global bad). We use simulations to consider scenarios in which the effects of the global externality either are evenly distributed, or have stronger influences on either the rich or the poor. We find that the global externality promotes the evolution of cooperation only if it either disproportionately benefits the poor or disproportionately reduces the payoffs of the rich. If the global externality primarily harms the poor, it undermines the evolution of prosocial behaviour. Understanding this effect is important given concerns that poorer households are more vulnerable to climate change impacts.
This article is part of the theme issue ‘Evolution and sustainability: gathering the strands for an Anthropocene synthesis’.
Keywords: cooperation, group selection, evolution
1. Introduction
Maintaining a common good within groups requires individual sacrifice, creating a temptation to free-ride on the efforts of others within a group. Sometimes, individual behaviours will also create consequences beyond group boundaries, affecting the population at large. The question we tackle in this paper is how this type of global externality affects the evolution of cooperative behaviour in a group-structured population. Will the global externality, arising from local cooperation, enhance or undermine the evolution of local cooperation? Would incentives of group members to cooperate change if defection by out-group members generates a global bad (as opposed to a global good) that undermines well-being (or reproductive fitness) of in-group members? These questions are important in the context of climate change, where local cooperation or defection can have global impacts. For instance, production and consumption in developed countries drive the accumulation of greenhouse emissions, influencing the climate. In turn, climate change affects developing countries most severely. If global externalities have an impact on individual behaviours, crowding-out pro-environmental behaviour, this would undermine the evolution of cooperation and could enhance the process of environmental degradation. Alternatively, if global externalities provide an additional incentive to cooperate, for instance, by reducing greenhouse emissions or protecting natural resources, emphasizing global impacts can help prevent the tragedy of commons. Previous studies on the tragedy of the commons have focused on how rules evolve within groups that can help prevent resource degradation [1] or how such rules and social norms emerge owing to cooperation and competition at different levels of organization [2], but have not considered global externalities. We argue that considering the global effects of local cooperation can reveal circumstances that may help us avoid the tragedy of the commons.
In this paper, we study the impact of global externalities on the evolution of prosocial behaviour in public good games. In public good games, cooperators contribute their endowments to the common pool, which are then multiplied by a positive factor and distributed equally among group members. Thus, cooperators generate local benefits. In our model, they additionally generate a global externality, which affects everyone in the (global) population, across all groups. Note that our use of the word ‘global’ throughout the paper simply refers to the population across all groups (what population biologists sometimes call the metapopulation). The model dynamics proceed in discrete time steps, each of which involves two stages in which agents' behaviours lead to the accrual of wealth in the form of payoffs. In Stage 1, group members can either cooperate or defect. Within groups, cooperators always receive lower payoffs than defectors because they bear the costs of cooperation. However, members of all-cooperators groups receive higher payoffs than of all-defectors groups because of the local benefits from cooperation. In Stage 2, global benefits are distributed based on payoffs received in Stage 1.
The global externality can be either positive (a global good) or negative (a global bad). In the first case, cooperators generate global benefits that increase payoffs of everyone in the population. In the second case, a global bad reduces everyone's payoffs. We study evolutionary dynamics of our model under three distributions of the global externality: uniform, proportional to payoffs, and inversely proportional to payoffs. We will show that the externality promotes the evolution of cooperation when the global good disproportionately benefits the poor or when the global bad falls mostly on the rich. When the externality affects everyone equally, it has no impact on the evolution of cooperation. Moreover, we show that the size of the global externality is less important than its distribution for the evolution of cooperation. The more the distribution is skewed in favour of the rich or the poor, the greater the impact the global externality has on prosocial behaviour.
Behavioural change and demand-side solutions have some potential to reduce carbon dioxide emissions [3,4]. Such solutions vary from investing in renewable energy (installing a photovoltaic system), eating more sustainably (becoming vegetarian), buying electric vehicles or cycling to work instead of using private motorized transport. All of these activities not only come at a cost to those involved, but also bring local benefits to all by reducing local pollution, and global benefits by slowing down the accumulation of global emissions. Both local and global pollution cause economic damage that reduces overall economic growth [5], even if it creates short-term benefits for polluters. As a result, local cooperation in the form of pollution prevention ensures higher income for everyone in the long run. Acts of resource conservation that allow the common pool resources to re-grow can also be seen as cooperative. This makes groups with many cooperators affluent in resources. Resource conservation (or lack thereof) also has global consequences. For example, deforestation in the Amazon results in significant species loss and degradation of indigenous peoples' incomes and health. In addition, it negatively impacts carbon emissions and the global water cycle. In scenarios where cooperators cause global harm, cooperators will still contribute to the greater economic prosperity of their group. However, this local growth may come at the cost of an increase in CO2 emissions and global temperature. Higher global temperature, in turn, results in long-term economic losses due to climate change that are distributed globally [6,7]. Our present study indicates that the evolution of prosocial behaviour is undermined if the global externality primarily harms the poor. Understanding this effect is important in the context of climate policies and rising wealth inequality (e.g. [8]). There are concerns that poorer households are more vulnerable to climate change impacts [9,10]. Thus, if the distribution of climate damages fall disproportionally onto the poor, it may undermine cooperation and push vulnerable groups into poverty in the future.
Our approach builds on the theoretical literature on group selection. Group selection models have been widely employed to study the evolution of cooperation and social institutions (e.g. [11–13]). It has been shown that larger between-group variance of a trait (i.e. prosocial behaviour) compared with the variance of that trait within groups can enhance the evolution of prosocial behaviour in structured populations. Recently, the theory of cultural group selection has achieved much attention in studies of sustainability [14–16]. Such studies have shown that resource conservation and supporting economic institutions co-evolve owing to selection pressure operating on individuals and groups. Although the idea that human cooperation has evolved (whether genetically or culturally) through group selection has raised some controversy, group selection is now widely regarded as a theoretically relevant evolutionary force, especially for cultural groups. It is beyond the scope of this paper to discuss the debate [12,13,17–22]. To date, however, the role of global externalities in the evolution of cooperation via group selection has received little attention, though notable exceptions include models of coalition-structured governance [23,25]. Nevertheless, global externalities are particularly important in understanding cooperative behaviour in modern humans. Groups often interact in the common environment, which affects selection pressures in more than one group or community at the time. For instance, empirical studies show that environmental changes affect cooperation in groups that manage shared resources [24]. In addition, actions of groups or communities can have global consequences affecting the entire population, such as in the case of climate or resources.
Our study contributes to the literature on inequality, cooperation and sustainability [24,25]. The relationship between cooperation and inequality has been showed to be U-shaped, meaning that cooperation persists at either low or high levels of inequality [26]. Other studies show that changes in the biosphere affect local inequalities, either through sudden shocks, such as natural disasters, or through more gradual environmental changes, e.g. by shifting climate patterns [25]. As a result, equity and sustainable behaviours co-evolve, affecting pathways of change in socio-ecological systems [24]. Studies have also examined the effects of intergroup inequalities on pro-environmental behaviour using common pool resource experiments [27,28]. For example, the results of Safarzynska & Sylwestrzak's [28] experiment show that when resources are unequal, members of low-endowment groups are likely to overharvest resources in expectation of donations from more affluent groups.
In this paper, we contribute to the literature on cultural group selection and sustainability by studying the impact of global externalities on the evolution of cooperation in structured populations. We use model simulations to examine this issue, which allowed us to systematically compare the effects of different distributions of global externalities on prosocial behaviour. Such formal exercises are important as empirical evidence typically comes from case studies that are not directly comparable [29]. The main message of our study is that reducing the unequal distribution of climate impacts, as well as reducing inequality more generally, promotes cooperation. Previous studies have focused on the impact of the unequal distribution of climate damages on economic growth [6], but have not yielded general conclusions regarding its impact on prosocial behaviour. We also investigated the effect of the global externality on cooperation depending on the (initial) distribution of wealth. We show that if the cost of cooperation is relatively higher for poor individuals than for rich individuals, the evolution of cooperation is undermined, regardless of the distribution of the global externality. The remainder of this paper is structured as follows. In §2, we briefly review literature on the evolution of cooperation by multi-level selection. In §3, we present our theoretical setting and derive a fundamental condition of cooperation to evolve in the presence of the global externality. In §4, we present simulation results. Section 5 concludes.
2. The evolution of cooperation by multi-level selection
Group selection provides a powerful conceptual framework to study the evolutionary mechanisms acting on different levels of organization [30–35]. In particular, cultural group selection theory has been helpful in explaining diffusion of prosocial behaviour, the evolution of different types of institutions and the emergence and persistence of sustainable social–ecological states [11,21,32,34,36,37]. Cultural group selection, as compared with group selection operating solely on genes, involves cognitive learning and cultural acquiring of social traits [12,21].
Formal models of the evolution of cooperation via cultural group selection are very diverse. In particular, they differ with respect to mechanisms of group selection and replication, as well as games underlying interactions within groups. Typically, individuals within groups engage in either a cooperative dilemma such as a public goods game [11,38,39] or a coordination game [40–42]. In coordination games, individuals can choose between different strategies and the system dynamics are characterized by multiple equilibria. For a novel group-beneficial trait to evolve two things must occur [40]: (i) it must become common in one population and (ii) it must spread from that population to others.
In public good games, individuals contribute some of their endowment to a common pool. Subsequently, all contributions are multiplied by a factor and divided among group members, so that the group's total payoff is maximized if everyone contributes. Yet, non-contributing, i.e. defection, is a dominant strategy. As a result, within groups defection spreads. However, if selection at the group level promotes groups that are better off—i.e. those with more cooperators—cooperation can evolve in the population if group selection is sufficiently strong relative to individual selection. In this context, policies that reduce the payoff disadvantage to cooperators (e.g. punishment imposed on defectors) within groups or affect the group structure so that individuals are more likely to interact with their own type can help cooperation to evolve [11,20,38,39].
A distinction can be drawn between formal group selection models in which higher-level selection emerges as a by-product of individual interactions (e.g. [13]) versus those in which there is an explicit top-down mechanism for group selection. The specific mechanism of group selection can take several possible forms, including: conflict, so that groups with more defectors are more likely to lose in conflict and be replaced by members of groups with more cooperators [11,39,43–45]; cultural transmission, where institutions of more successful groups are more likely to be imitated by other groups [40,42,46–48]; and payoff-biased migration, where individuals preferentially leave less successful groups to join groups that are more successful [41,49].
An important class of models has shown that intergroup conflict can promote the evolution of prosocial behaviour. Welfare aligns the fate of group members, making individuals cooperate to inflict force on other groups [36]. Results from experiments conducted before, during and after the 2006 Israel–Hezbollah war indicate that during wartime people are more willing to pay costs to punish defectors and to reward cooperative behaviour [50]. In addition, warfare promotes the evolution of institutions, which enabled large human groups to function without splitting up [51]. Bowles & Choi [46] propose a formal model to show how altruism and parochialism, which captures hostility toward out-group members, can co-evolve together owing to group selection, although both behaviours reduce individual payoffs. As another example, using a model of intergroup conflict, Makowsky & Smaldino [45] show how institutions that promote inequality may naturally divide a population into a ruling class of non-cooperators and an underclass of cooperators whose contributions sustain the group.
In cultural evolutionary theory, biased transmission involves the adoption of cultural variants that enhance reproductive success. Preferentially imitating behaviours that are common in one's social group has been referred to in the literature as conformist transmission [30]. Instead of copying the most common strategies, individuals can also imitate the most influential, knowledgeable or skillful behaviour. Imitating ‘the most successful’ is known as prestige-biased or success-biased transmission [52,53]. If individuals have contact with out-group members, they may imitate the behaviours of members of more successful groups. In this way, individual transmission can promote the evolution of group-beneficial norms and institutions [40,42]. Other factors that enhance the evolution of prosocial behaviour include punishment of deviant behaviours or symbolic markers that correlate with behavioural unobservable norms [21]. Studying the importance of these mechanisms for diffusion of sustainable behaviours has recently received increasing attention in the literature [37].
Finally, selective migration between groups can promote the evolution of cooperation. Boyd & Richerson [41] show that in the presence of non-random, payoff-biased migrations, the evolution of a group-beneficial trait depends on the relative strength of migration and local adaptation. Group-beneficial traits can evolve if local adaptation dominates migration. In their model, when individuals migrate from a group with a low level of prosocial behaviour to a group with a high frequency of prosocial individuals, two things happen. First, the level of prosociality decreases in the latter, while increasing in the former group as a result of changes in the relative frequencies of strategies between groups. This may reverse the direction of migration. The process will continue until both groups achieve the same average fitness. It is important to note that payoff-biased migration is not effective in promoting cooperation in group selection models that rely on cooperative dilemmas such as public good games [54]. Here, defectors always achieve higher fitness and thus diffuse within groups over time. In such models, more migration reduces the between-group variance compared with the within-group variance, weakening the strength of group selection and thereby making the evolution of cooperation less likely.
3. A simple group selection model with the global externality
The studies discussed in the previous section show the conditions under which cooperation evolves in structured populations. In particular, cooperation is favoured when there is greater variance between groups relative to variance within groups, when the cost-to-benefit ratio for cooperative behaviour is smaller, and when there are more groups relative to the number of group members. In this section, we examine how a global externality affects these previous results. In §3a, we use the Price equation to understand a general condition favouring the evolution of cooperation in the presence of a global externality that affects every member of the population. The Price equation is often used to decompose evolutionary change into effects of within- and between-group components [55]. Van Veelen [56] points out that the Price equation is often misused to perform statistics or to make predictions. We do not use the Price equation for such purposes. We instead use it to decompose evolutionary changes into within-group and between-group variances to better understand the effects of the global externality on cooperation, which we then verify with a dynamic model. In particular, in §3b, we present a set-up of the simulation model that includes additional components, e.g. group conflict and mutation, and assumes a specific distribution of a global externality. The results from the model simulations could not be studied analytically owing to the complexity of the model and the presence of stochastic components.
(a) . The Price decomposition
Consider a population in which individuals may choose to participate in a group-beneficial social activity at a personal cost. Examples of such activities may include reducing meat consumption or becoming a vegetarian, cycling to work instead of using a car, or investing in solar panels. All of these activities come at a personal cost but reduce local pollution. In addition, they may entail modest reductions to global temperature and carbon dioxide emissions if a critical mass of adopters is achieved. Formally, in our model, a population is divided into m groups, each of which contains exactly n individuals. We consider only two pure strategies: individuals are either cooperators (C) who engage in prosocial behaviour, or defectors (D) who do not. Engaging in prosocial behaviour reduces payoffs of cooperators, but brings benefits to everyone in their community. We will often refer to acquired payoffs as wealth throughout the paper—where wealth can represent any desirable material resources. Individuals change their behaviour to adopt strategies of more affluent persons, which has been referred to in the literature as success-biased social learning [53]. Formally, this is also mathematically equivalent to vertical transmission in which more successful individuals have more offspring. Within groups, the frequencies of strategies associated with above-average payoffs increase over time. Cooperators incur a cost c. The prosocial activity generates a local benefit b, which is equally distributed among group members, so that each member receives a benefit equal to , where is the frequency of cooperators in group j.
If b > 0 > c and , the scenario described heretofore is a classic cooperative dilemma, i.e. the public goods game, internal to each group. Here we extend the impact of social behaviour beyond the boundaries of a single group. In particular, each cooperator in the population also generates a global externality d. Thus, actions have social consequences not only at the individual and local levels, but also at the global level. To illustrate with an example, if d < 0, one might think about the global externality as climate damages that reduce wealth of individuals owing to some catastrophic events. If d > 0, the externality captures a global good, for instance, the engagement of social activists in climate education, which not only creates knowledge in the local community, but also contributes to global climate awareness.
The payoff to cooperators in group j is given by
| 3.1a |
while payoffs to defectors are
| 3.1b |
where a represent baseline payoffs, c is the cost of cooperation, b represents local benefits of prosocial behaviour, d is the global, population-level externality resulting from prosocial behaviour and p is the frequency of cooperators in the population. This game is a general form, but special cases involve more familiar games. For example, if d = 0, the scenario is a familiar cooperation dilemma without any consequences of group structure. In such cases, cooperation cannot evolve without some mechanism facilitating either assortment among cooperators or punishment of defectors. In equations 3.1a,b, and capture the shares of the global externality going to cooperators and defectors in group j, respectively, with .
The change in the global frequency of cooperators can be expressed as (see [57,58]:
| 3.2 |
where the global frequency of cooperators is , and the mean wealth is equal to
| 3.3 |
Using , equation (3.3) reduces to
| 3.4 |
Equation (3.2) can be re-written as
| 3.5 |
After simple transformations, using the following notation: for the between-group variance and for the within-group variance, it can be shown that (see electronic supplementary material, appendix A for derivations)
| 3.6 |
If each member of the group receives an equal share of global benefits , the last component of equation (3.6) disappears, and the equation reduces to the well-known formula (see [59]). The idea behind this formula is simple: the larger the difference between the benefits b and costs c of prosocial behaviour, the more likely cooperation is to evolve. The probability is also greater if the between-group variance is large, meaning that the groups are characterized by different compositions, or the within-group variance is small. The former intensifies the selection between groups, while the latter reduces selection within-group, which works against cooperators.
According to equation (3.6), the global externality does not affect within- or between-group selection in the case where global benefits are distributed equally within the population. The global externality promotes the evolution of cooperation only if cooperators receive on average a larger share of it ( > 0) for d > 0, or its smaller share for d < 0. Equation (3.6) presents the general condition for the evolution of prosocial behaviour in the presence of the global spillovers, which applies to any distribution of the global externality. In §3b and §4, we will compare the impact of the global externality distributed uniformly, proportionally and inversely proportionally to payoffs within the population using model simulations.
(b) . Simulation model
In this section, we conduct model simulations to examine the impact of global externality on local cooperation. The basic model set-up is the same as in §3a. The simulation model specifies additional mechanisms such as group conflict or mutation. Formally, we extend the simulation model by Bowles et al. [11] by adding the global externality. In the model, a population is subdivided into groups. Individuals preferentially adopt strategies that generate above-average payoffs. In each step, groups are matched in pairs and engage in conflict with a certain probability. A group with higher payoffs wins and repopulates a losing group. Cooperators engage in prosocial behaviour, which generates local benefits for everyone in the group, but at a cost to themselves. As a result, groups with many cooperators have higher chances of winning in conflict. In this setting, we examine the role of global externalities in the evolution of prosocial behaviour.
We consider a population divided into m groups each populated by n members. We initialize the population so that each individual is randomly assigned to be a cooperator or a defector, with equal probability. We compute payoffs in a two-stage process. In Stage 1, individuals either cooperate or defect. The initial payoff to an agent i in group j is calculated without consideration of the global externality:
| 3.7 |
where a is the baseline payoff, and b and c are local benefits and costs of cooperation, respectively, if the individual is a cooperator and 0 if they are a defector. In other words, Stage 1 payoffs are determined entirely by the individual's behaviour strategy and by the frequency of cooperators within their group.
Payoffs received in Stage 1 make individuals relatively poor or wealthy. In Stage 2, global externalities are then distributed based on these Stage 1 payoffs, so that the global externality affects the payoffs of everyone in the population. Formally, we re-calculate payoffs as
| 3.8 |
where is the share of the global externality received by individual i. We consider three distributions of the global externality as a function of the Stage 1 payoffs (): uniform, proportional to payoffs, and inversely proportional to payoffs. The share of the global externality is calculated using a modified function from Dennig et al. [6]:
| 3.9 |
where ensures that , while is the relative Stage 1 payoff of individual i. The coefficient γ captures the wealth elasticity of global benefits/damages. If γ = 0, everyone receives an equal share of global benefits, namely 1/mn. Elasticity γ < 0 implies that ‘poor’ individuals receive a larger share of global benefits, whereas if elasticity γ > 0, they receive a smaller share of it. The total share of the global externality is constrained to sum to 1, , with and .
Within groups, frequencies of cooperators change according to the replicator dynamic standard in population biology:
| 3.10 |
where captures the strength of individual selection, i.e. how fast behaviours generating the above-average payoffs diffuse in the group, and is the mean wealth of group j.
After individuals revise their strategies, with some small probability µ (the ‘mutation rate’), an individual changes their strategy at random. With probability , each group is selected for conflict, and competition takes places between randomly matched groups. A group with higher total payoffs wins and repopulates a losing group. Formally, each member of the losing group becomes a cooperator with the probability equal to the frequency of cooperators in the winning group or a defector otherwise.
To examine the impact of the global externality on cooperation, we consider seven scenarios:
-
1.
The baseline scenario, in which we assume no global externality (d = 0);
-
2.
Scenario 1—‘an equal distribution of the global good’ with a global good (d > 0) distributed equally within the population;
-
3.
Scenario 2—‘the global good favouring the rich’ with a global good (d > 0) that disproportionately benefits the rich (γ > 0);
-
4.
Scenario 3—‘the global good favouring the poor’ with a global good (d > 0) that disproportionately benefits the poor (γ < 0);
-
5.
Scenario 4—‘an equal distribution of the global bad’ with a global bad (d < 0) that is evenly distributed within the population;
-
6.
Scenario 5—‘the global bad disfavouring the rich’ with a global bad (d < 0) that disproportionately reduces payoffs of the rich (γ > 0);
-
7.
Scenario 6—‘the global bad disfavouring the poor’ with a global bad (d < 0) that disproportionately reduces payoffs of the poor (γ < 0).
4. Simulation results
In this section, we use model simulations to study the evolution of cooperation under different distributions of the global externality. Unless stated otherwise, we report the mean frequencies of cooperators over time (between 1st and 2500th time step) from 25 simulations conducted for the same initial conditions with different initial seeds.1 Table 1 summarizes the baseline values of the parameters, which we took from Bowles et al. [11] with the exception to parameters related to the global externality: γ and d. We focus on how different distributions of the global externality affect the evolution of cooperation. To study this, we assume parameter values in the baseline scenario for which cooperation evolved in Bowles et al. [11]. Our results hold qualitatively for any benefit-to-cost ratio that led to cooperation in that study; we have opted not to explore this ratio systematically.2 Model simulations were conducted using the Laboratory for Simulation Development software (https://www.labsimdev.org/).
Table 1.
Model parameters and values.
| parameter | default value (range) | |
|---|---|---|
| m | number of groups | 20 (5,50) |
| n | group size | 10 (5,30) |
| a | baseline payoffs | 10 (1,20) |
| γ | wealth elasticity | (−5,5) |
| b/c | benefit-to-cost ratio | 1.25 |
| d | global externality | d is set in model simulations so that , for p = 1; in the baseline |
| probability of conflict | 0.05 (0.05–0.25) | |
| µ | mutation | 0.01 |
| α | strength of within-group (individual) selection | 0.5 (0.5–1) |
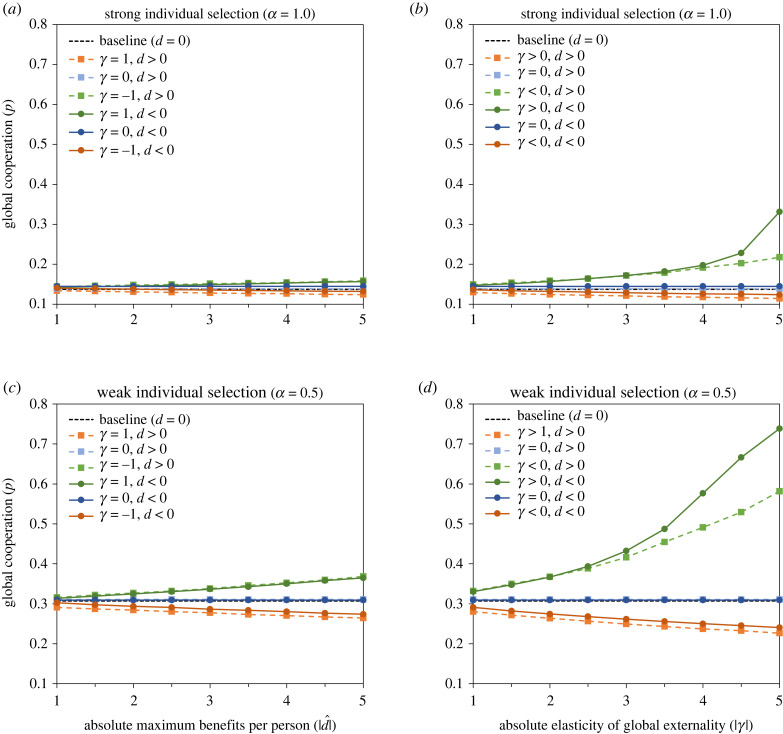
Figure 1a,b compares the mean global frequency of cooperators in model simulations characterized by different magnitudes of the global externality (d) and the wealth elasticity of the global externality (γ). Unless stated otherwise, we set d so that it satisfies a condition (for p = 1). We keep equal to the same value regardless of group size n and number of groups m, to allow for meaningful comparisons between different scenarios. Parameter can be thought of as the ‘maximum’ amount of the global externality received per person if everyone engages in prosocial behaviour (p = 1), under the assumption of equal distribution. In model simulations, everyone typically receives much less than this as the global frequency of cooperation rarely reaches 100%.
Figure 1.
The distribution of the global externality, not its size, affects cooperation in the population. In the left panels, γ = ± 1; in the right panels, = ± 2.5. Comparing the bottom row with the top row shows that cooperation is more strongly favoured when group selection is stronger relative to individual-level selection. Each data point summarizes the mean over 2500 time steps from 25 simulations conducted for the same initial conditions. (Online version in colour.)
In figure 1a, we examine the impact of different values of on the evolution of cooperation for γ = |1|, while in figure 1b, we keep constant but increase the value of elasticity γ. We assume strong within-group selection in these model simulations (α = 1). Figure 1c,d do the same for moderate selection pressure within groups (α = 0.5). The results reveal that the size of global benefits has a negligible impact on the evolution of cooperation when γ = |1| (figure 1a,c). On the other hand, increasing the skewness of the distribution (γ) can have a significant impact on global cooperation. Comparing figure 1b–d shows that an increase in γ affects cooperation more, the slower individual selection is. These results can be explained by the last component of equation (3.6). The equation shows that an increase in d promotes the evolution of cooperation only if the share of benefits received by cooperators is sufficiently high (). Thus, the distribution of the global externality is more important than its size for the evolution of global cooperation.
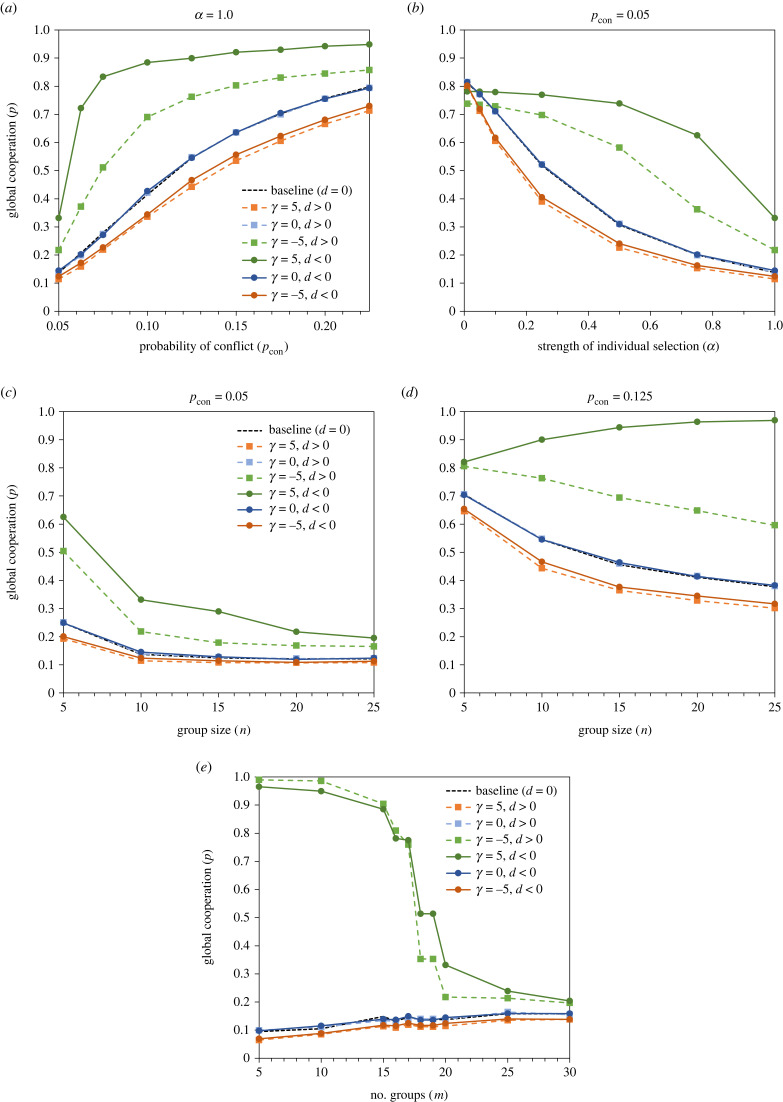
Figure 2a–f examine the impact of increasing the strength of individual (α) versus group selection (), group size (n) and number of groups (m) on the evolution of cooperation under different distributions of the global externality. In each figure, we compare the impact of the global externality in the cases where d is positive (d > 0, a global good) or negative (d < 0, a global bad) under three damage/benefit distributions γ = {−5,0,5}, which together with the baseline scenario (d = 0) result in seven possibilities.
Figure 2.
The evolution of global cooperation (p) responds to several parameters. Cooperation increases with more conflict (a), weaker individual-level selection (b) and smaller groups (c), though the effect of group size interacts with the rate of conflict (d). In all cases, cooperation is maximized when the externalities disproportionately favour the poor over the rich. (e) The effect of redistribution favouring cooperators is highly affected by the number of groups. In the figure, γ is the wealth elasticity of the global externality, α is the strength of individual selection and captures the probability of intergroup conflict. Each data point summarizes the mean over 2500 time steps from 25 simulations conducted for the same initial conditions. (Online version in colour.)
Our most important findings can be summarized as follows. First, there are no differences in the global frequencies of cooperation between the baseline model simulations and model simulations under an equal distribution of the global externality, regardless of whether the externality is positive or negative (Scenarios 1 and 4). Second, the global externality promotes the evolution of cooperation compared with the baseline model only under two conditions: (i) with the global good disproportionately favouring the poor in Scenario 3 (d > 0 and γ < 0), which implies that that ‘poor’ individuals receive a larger share of the global benefits, and (ii) in Scenario 5, in which the global bad disproportionately harms the rich (d < 0 and γ > 0). The impact of the global externality on global cooperation is larger under the second condition compared with other scenarios. Finally, if the ‘global bad’ disproportionately affects the poor (Scenario 6), as is expected with climate change impacts, the global externality reduces global cooperation. A similar effect occurs if the global externality benefits disproportionately the rich (Scenario 2).
Figure 2a replicates the findings from Bowles et al. [11] that intergroup conflict promotes cooperation. However, we show that this result is strongly influenced by the distribution of the global externality. If the global benefits of cooperation are directed back toward cooperators, overall cooperation can be increased with substantially less intergroup conflict. On the other hand, redistribution that favours the already-wealthy hurts cooperation and requires additional intergroup conflict to recover baseline levels of global cooperation.
Figure 2a,b show that these results are robust to different strengths of group versus individual selection. In general, the weaker the within-group selection (α) or the stronger the between-group selection () is, the higher cooperation becomes. This replicates the most important finding from group selection models [60,61]. In addition, our results indicate that the effect of individual and group selection depends on the distribution of the global externality: it is stronger in Scenarios 3 and 5, and weaker in Scenarios 2 and 6.
An important class of group selection models has shown that cooperation diffuses more rapidly the smaller the groups are [43,62,63] or if selection operates on more groups [13]. Figure 2c illustrates that this is also the case in our model regardless of the distribution of the global externality and its sign (positive/negative). There is one exception. If the probability of conflict is sufficiently large ( in figure 2d), the effect of group selection dominates within-group selection, offsetting the negative impact of group size on cooperation in the scenario in which the global bad disproportionately hurts the rich.3 In group selection models, an increase in the group size requires an increase in the b/c ratio for cooperation to evolve [13]. A larger b/c ratio attenuates the selection pressure against cooperators in larger groups. Similarly, the global externality for d < 0 and γ > 0 (or d > 0 and γ < 0) reduces the payoff disadvantage to cooperation within groups. Simultaneously, it increases between-group variances as the global externality affects payoffs of cooperators and defectors in different groups. Typically, the largest payoffs are received by defectors in groups dominated by cooperators, but also by cooperators in such groups.
Finally, in all model simulations, we re-adjusted parameter d so that the value of , which can be thought of as benefits per person, is the same in all model simulations. The only exception concerns figure 2e, where we examine the impact of keeping d constant despite increasing the number of groups. This implies that the size of the global externality per person declines with the number of groups. The results show that a sufficiently high global externality promotes the evolution of cooperation only in small groups in Scenarios 3 and 5. This result appears to be driven by the fact that the value of externality, d, was connected to the population size in our model, and so the per capita externality decreased with the number of groups, m. Thus, cooperation decreases when the same reward is distributed among more individuals.
(a) . Global bad generated by defectors
How would our predictions change if the global externality were generated by defectors rather than cooperators? In this case, the condition for the evolution of cooperation in equation (3.6) would become:
| 4.1 |
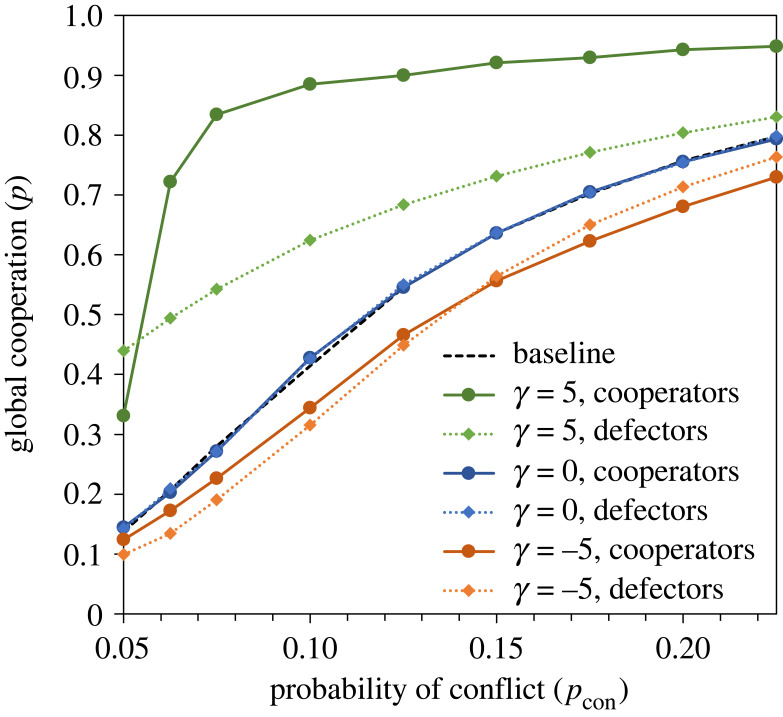
Equation (4.1) implies that the global bad (d < 0) generated by defectors promotes cooperation if . As a result, the global externality has two opposing effects on cooperation here: more defectors increase benefits to cooperation (if d > 0), but simultaneously reduce the frequency of prosocial behaviour. In the model version, where the global externality was caused by actions of cooperators, these effects enhanced each other. As a result, for each damage distribution, cooperation is greater if the global externality is generated by cooperators compared with non-cooperators, as illustrated in figure 3.
Figure 3.
The results are qualitatively similar whether the global bad is produced by cooperators (solid lines) or defectors (dotted lines). The solid lines are the same as in figure 2a, and all parameters are the same as in that figure. (Online version in colour.)
Model simulations show that other general findings from our study are qualitatively unaffected by this modification: the higher the probability of conflict, the smaller the group size, or the more groups, the higher cooperation in prosocial behaviour becomes (we do not show these results). In general, global cooperation increases if a negative externality caused by behaviour of defectors disproportionately reduces the payoffs of the rich individuals compared with the situation where the global bad affects everyone in the population equally or the poor disproportionately (figure 3).
(b) . Unequal wealth
In our model, payoffs received from the public good determine who is better off (more affluent) than others. However, people are often poor or rich for reasons that are unrelated to their behaviour in social dilemmas. In this section, we examine how our results are affected by different initial distributions of wealth. To this end, we conduct additional model simulations, where we replace the parameter a, describing baseline payoffs in equations (3.7) and (3.8), by an individual-level parameter , representing the baseline payoff for individual i in group j. We consider four scenarios: we draw the parameter for each individual i in group j from the uniform distribution , and compare the results with simulations in which parameter is drawn from the uniform distribution with much larger mean . These result in stronger and weaker selection, respectively. In particular, selection is weaker when a larger portion of total fitness comes from baseline payoffs. In addition, we run model simulations in which parameter is drawn separately for each group j from either or ).
The parameter distributed at the individual level can be interpreted as reflecting the global distribution of initial wealth, which favours some individuals over others owing to heredity or luck, i.e. being born into a wealthy family. The distribution of the parameter at the group level describes a situation in which some countries/groups are generally better off because of differences in the level of economic development. As a result, members of wealthy groups earn more, regardless of their efforts/contributions to the public good, owing to historical contingencies in economic development or having access to more abundant resources.
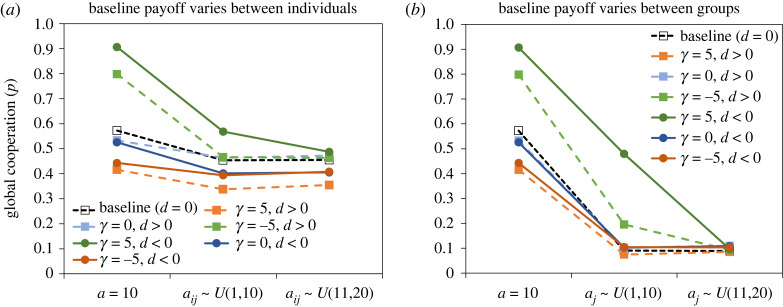
Figure 4a,b present the results from such additional model simulations in the cases where the baseline fitness is distributed at the individual or group level, respectively. Each figure panel compares the mean global frequency of cooperators between the baseline scenario, where a is constant (a = 10), with the results of model simulations conducted under strong selection, where or is drawn from , and weak selection, where or is drawn from . Other parameters are equal to their baseline values as described in table 1 with the exception of the probability of conflict, which we set to . This is motivated by the fact that for this value of the parameter, the distribution of the global externality has a strong impact on the global frequency of cooperation (figure 2a).
Figure 4.
The evolution of cooperation under different distributions of initial wealth. The leftmost data points in each panel represent the standard model above in which all agents receive a baseline payoff of a = 10. (a) Each agent independently draws a baseline payoff. (b) Baseline payoff varies between groups in which each individual has the same baseline payoff. As before, γ is the wealth elasticity of the global externality, d is the strength of global externality. Each data point summarizes the mean over 2500 time steps from 25 simulations conducted for the same initial conditions. (Online version in colour.)
The results in figure 4 indicate that increasing the mean of the distribution from which the parameter is drawn reduces the impact of the global externality on the level of cooperation, regardless of whether the parameter is distributed at the level of individuals or of groups. In the case where the parameter is drawn at the individual level from ) (strong selection), the more/less the distribution of the global good/bad is skewed towards the poor, the greater the global cooperation. Thus, our general findings are not affected by this scenario. Nevertheless, for each Scenario 1–7, the global frequency of cooperation is lower in model simulations with heterogeneous baseline payoffs compared with model simulations, where a is constant (figure 4a). The parameter distributed at the group level undermines further the impact of the global externality on cooperation. This can be explained by the fact that the parameter distributed at the individual level changes the selection pressure against cooperators within groups, which is not the case if the parameter is distributed at the group level. In electronic supplementary material, appendix B, we present additional results from the sensitivity analysis, where we draw the parameter from the normal distribution . In supplementary material, figure B1(a–c), we compare the results from model simulations with the mean equal to 5, 10 and 15, respectively. In each figure panel (a–c), we increase the variance of baseline payoffs. The results indicate that the impact of the global externality on the cooperation is weaker, the greater the variance, and the weaker the selection. However, the main result of our study regarding the impact (direction) of the global externality on cooperation is not affected; only the magnitude of the effect is affected by increasing the variance of baseline payoffs, at least for a sufficiently small variance.
5. Conclusion
Our results show that if local behaviours have consequences beyond group boundaries, the evolution of cooperation in the population can be affected via the global impacts of those behaviours. We have used a simple model of multi-level selection to derive the general condition for the evolution of prosocial behaviour in the presence of a global externality. We showed that a global externality has no effect on within-group or between-group selection when it is evenly distributed among all members of the population, regardless of the magnitude of the externality. This makes sense, since a trait that affects every member of a population equally will have no effect on relative fitness and thus cannot influence selection between individuals. However, if the global externality is distributed unequally in the population, it can have a large impact on prosocial behaviour. Cooperators evolve when the global good disproportionately benefits the poor or when the global bad falls disproportionately on the rich—in other words, when the benefits of cooperation are bestowed preferentially on those most likely to themselves be prosocial (and therefore relatively poorer than more selfish individuals). This effect is stronger the more skewed the distribution of the global externality is.
One of the most important implications of our model is that when the global bad disproportionately affects the poor, the evolution of cooperation is undermined. In general, cooperators are relatively poorer than other group members because they bear the costs of financing the public good that benefits everyone. However, groups with many cooperators are better off, i.e. everyone in such groups receives higher payoffs compared with groups dominated by defectors. If climate damage disproportionately reduces cooperators' payoffs, this prevents group selection from promoting groups with many cooperators. In this context, our results provide another argument for increasing efforts to mitigate climate change. It has been shown that when climate change primarily affects the poor, greater mitigation efforts are needed than when damages are proportional to income [6]. Our analysis reveals that under an unequal distribution of climate damages, climate change not only reduces payoffs but also undermines prosocial behaviour. Reducing the inequality that results from the unequal effects of climate change, e.g. through subsidies, could reduce the negative effect of this type of inequality on cooperation.
Our results have been shown to depend on the distribution of initial wealth. We can interpret payoff differences imposed exogenously on individuals as those caused by historical contingencies in economic development or by differences in natural resources between groups. When factors other than payoffs in social dilemmas determine individual wealth, overall cooperation may be reduced; this has also been found by other researchers (e.g. [64]). Ruttan & Borgerhoff Mulder [65], for example, showed that if the wealthy benefit more from cooperation than the poor, initial (exogenous) differences in wealth can promote cooperation via coercion. Although in our study wealth inequality undermines cooperation, it does not entirely eliminate the effect of global externalities. This is especially true under strong selection, when initial wealth is distributed among population members from a distribution with a low mean. This latter assumption may imply fairly low levels of global wealth or natural resources. This in turn suggests that the global externality is particularly important in the early stages of economic development, or that it may become more important in the future with progressing (global) resource scarcity.
Our approach provides a first step in studying the role of global externalities in the evolution of prosocial behaviour. In future studies, it will be important to examine different distributions of global benefits, such as when the distribution depends on behaviours (strategies) rather than payoffs as in our study, or to examine the distribution of global externalities at the group instead of individual level. Extending the model to more than two levels of selection or including other behaviours that have been shown to influence prosocial behaviour, such as punishment or migration, may also provide new insights into the conditions under which prosocial behaviour evolves.
Endnotes
We conducted 100 simulations for selected parameter settings with different initial seeds. The results did not differ between 25 and 100 repetitions.
Values of wealth elasticity (γ) and global externality per person () would need to be adjusted for each benefits-to-cost ratio. For example, the smaller the benefits-to-cost ratio, the lower global benefits per person are needed to achieve the same level of cooperation.
Data accessibility
The code for replication is available from the Open Science Framework repository: https://osf.io/eb3gy/ [66].
Authors' contributions
K.S.: conceptualization, formal analysis, writing—original draft; P.E.S.: conceptualization, writing—original draft.
Both authors gave final approval for publication and agreed to be held accountable for the work performed herein.
Conflict of interest declaration
We declare we have no competing interests.
Funding
This work was conducted as a part of the Evolutionary Approaches to Sustainability Working Group at the National Institute for Mathematical and Biological Synthesis, sponsored by the National Science Foundation through NSF Award no. DBI-1300426, with additional support from the University of Tennessee, Knoxville.
References
- 1.Ostrom E. 1990. Governing the commons: the evolution of institutions for collective action. Cambridge, UK: Cambridge University Press. [Google Scholar]
- 2.Levin S. 2010. Crossing scales, crossing disciplines: collective action in the Global Commons. Phil. Trans. R. Soc. B 365, 13-18. ( 10.1098/rstb.2009.0197) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 3.Creutzig F, et al. 2018. Towards demand-side solutions for mitigating climate change. Nat. Clim. Change 8, 268. ( 10.1038/s41558-018-0121-1) [DOI] [Google Scholar]
- 4.Creutzig F, et al. 2022. Demand-side solutions to climate change mitigation consistent with high levels of wellbeing. Nat. Clim. Change 12, 36-46. ( 10.1038/s41558-021-01219-y) [DOI] [Google Scholar]
- 5.Nordhaus WD. 1994. Managing the global commons: the economics of climate change. Cambridge, MA: MIT Press. [Google Scholar]
- 6.Dennig F, Budolfson MB, Fleurbaey M, Socolow RH. 2015. Inequality, climate impacts on the future poor, and carbon prices. Proc. Natl Acad. Sci. USA 112, 15 827-15 832. ( 10.1073/pnas.1513967112) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 7.Safarzyńska K, van den Bergh J. 2022. ABM-IAM: optimal climate policy under bounded rationality and multiple inequalities. Environ. Res. Lett. 17, 094022. ( 10.1088/1748-9326/ac8b25) [DOI] [Google Scholar]
- 8.Piketty T. 2014. Capital in the twenty-first century. Cambridge, MA: Belknap Press. [Google Scholar]
- 9.Cutter SL, Boruff BJ, Shirley SL. 2003. Social vulnerability to environmental hazards. Social Sci. Q. 84, 242-261. ( 10.1111/1540-6237.8402002) [DOI] [Google Scholar]
- 10.IPCC. 2022. Climate change 2022: impacts, adaptation, and vulnerability. Contribution of Working Group II to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change. Cambridge, UK: Cambridge University Press.
- 11.Bowles S, Choi JK, Hopfensitz A. 2003. The co-evolution of individual behaviours and social institutions. J. Theor. Biol. 223, 135-147. ( 10.1016/S0022-5193(03)00060-2) [DOI] [PubMed] [Google Scholar]
- 12.Henrich J. 2004. Cultural group selection. Co-evolutionary process and large-scale cooperation. J. Econ. Behav. Org. 53, 85-88. ( 10.1016/S0167-2681(03)00108-2) [DOI] [Google Scholar]
- 13.Traulsen A, Nowak MA. 2006. Evolution of cooperation by multilevel selection. Proc. Natl Acad. Sci. USA 103, 10 952-10 955. ( 10.1073/pnas.0602530103) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Safarzynska K. 2013. The coevolution of culture and environment. J. Theor. Biol. 322, 46-57. ( 10.1016/j.jtbi.2013.01.004) [DOI] [PubMed] [Google Scholar]
- 15.Kline MA, Waring TM, Salerno J. 2018. Designing cultural multilevel selection research for sustainability science. Sustain. Sci. 13, 9–19. ( 10.1007/s11625-017-0509-2) [DOI] [PMC free article] [PubMed]
- 16.Waring T, Goff SH, Smaldino PE. 2017. The coevolution of economic institutions and sustainable consumption via cultural group selection. Ecol. Econ. 131, 424-532. ( 10.1016/j.ecolecon.2016.09.022) [DOI] [Google Scholar]
- 17.Sober E, Wilson DS. 1998. Unto others: the evolution and psychology of unselfish behavior. Cambridge, MA: Harvard University Press.
- 18.Wilson D, Wilson EO. 2007. Rethinking the theoretical foundation of sociobiology. Q. Rev. Biol. 82, 327-348. ( 10.1086/522809) [DOI] [PubMed] [Google Scholar]
- 19.Lehmann L, Keller L, West S, Roze D. 2007. Group selection and kin selection: two concepts but one process. Proc. Natl Acad. Sci. USA 104, 6736-6739. ( 10.1073/pnas.0700662104) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Killingback T, Bieri J, Flatt T. 2006. Evolution in group-structured populations can resolve the tragedy of the commons. Proc. R. Soc. B 273, 1477-1481. ( 10.1098/rspb.2006.3476) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Richerson P, et al. 2016. Cultural group selection plays an essential role in explaining human cooperation: a sketch of evidence. Behav. Brain Sci. 30, E30. ( 10.1017/S0140525X1400106X) [DOI] [PubMed] [Google Scholar]
- 22.Wilson DS, Madhavan G, Gelfand MJ, Hayes SC, Atkins PW, Colwell RR. 2023. Multilevel cultural evolution: from new theory to practical applications. Proc. Natl Acad. Sci. USA 120, e2218222120. ( 10.1073/pnas.2218222120) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Vasconcelos VV, Hannam PM, Levin S, Pacheco JM. 2020. Coalition-structured governance improves cooperation to provide public goods. Scient. Rep. 10, 9194. ( 10.1038/s41598-020-65960-8) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Leach M, Reyers B, Bai X, Brondizio ES, Cook C, Díaz S, Espindola G, Scobie M. 2018. Equity and sustainability in the Anthropocene: a social–ecological systems perspective on their intertwined futures. Glob. Sustain. 1, e13. ( 10.1017/sus.2018.12) [DOI] [Google Scholar]
- 25.Hamann M, et al. 2018. Inequality and the biosphere. Annu. Rev. Environ. Resour. 43, 61-83. ( 10.1146/annurev-environ-102017-025949) [DOI] [Google Scholar]
- 26.Dayton-Johnson J, Bardhan P. 2002. Inequality and conservation on the local commons: a theoretical exercise. Econ. J. 112, 577-602. ( 10.1111/1468-0297.00731) [DOI] [Google Scholar]
- 27.Cardenas JC, Stranlund J, Willis C. 2002. Economic inequality and burden-sharing in the provision of local environmental quality. Ecol. Econ. 40, 379-395. ( 10.1016/S0921-8009(01)00285-3) [DOI] [Google Scholar]
- 28.Safarzynska K, Sylwestrzak M. In press. The impact of bridging social capital on the tragedy of the commons: experimental evidence. J. Inst. Econ. ( 10.1017/S1744137423000073) [DOI] [Google Scholar]
- 29.Smaldino PE. 2023. Modeling social behavior: mathematical and agent-based models of social dynamics and cultural evolution. Princeton, NJ: Princeton University Press. [Google Scholar]
- 30.Boyd R, Richerson PJ. 1985. Culture and the evolutionary process. Chicago, IL: University of Chicago Press. [Google Scholar]
- 31.Frank S. 1998. Foundations of social evolution. Princeton, NJ: Princeton University Press. [Google Scholar]
- 32.Wilson D. 2002. Darwin's cathedral: evolution, religion, and the nature of society. Chicago, IL: University of Chicago Press. [Google Scholar]
- 33.Henrich J. 2004. Cultural group selection. Co-evolutionary process and large-scale cooperation. J. Econ. Behav. Org. 53, 3–35. ( 10.1016/S0167-2681(03)00094-5) [DOI]
- 34.van den Bergh JCJM, Gowdy JM. 2009. A group selection perspective on economic behavior, institutions and organizations. J. Econ. Behav. Org. 72, 1-20. ( 10.1016/j.jebo.2009.04.017) [DOI] [Google Scholar]
- 35.Turchin P. 2011. Warfare and the evolution of social complexity: a multi-level-selection approach. Struct. Dyn. 4. ( 10.5070/sd943003313) [DOI] [Google Scholar]
- 36.van Veelen M, Hopfensitz A. 2007. In love and war; altruism, norm formation, and two different types of group selection. J. Theor. Biol. 249, 667-680. ( 10.1016/j.jtbi.2007.09.007) [DOI] [PubMed] [Google Scholar]
- 37.Waring MW, Kline MA, Brooks JS, Goff SH, Gowdy J, Janssen MA, Smaldino PE, Jacquet J. 2015. A multilevel evolutionary framework for sustainability analysis. Ecol. Soc. 20, 34. ( 10.5751/ES-07634-200234) [DOI] [Google Scholar]
- 38.Rand DG, Nowak MA. 2011. The evolution of antisocial punishment in optional public goods games. Nat. Commun. 2, 434. ( 10.1038/ncomms1442) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 39.Bowles S, Choi JK. 2007. The coevolution of parochial altruism and war. Science 26, 636-640. ( 10.1126/science.1144237) [DOI] [PubMed] [Google Scholar]
- 40.Boyd R, Richerson PJ. 2002. Group beneficial norms spread rapidly in a structured population. J. Theor. Biol. 215, 287-296. ( 10.1006/jtbi.2001.2515) [DOI] [PubMed] [Google Scholar]
- 41.Boyd R, Richerson PJ. 2009. Voting with your feet: payoff biased migration and the evolution of group beneficial behavior. J. Theor. Biol. 257, 331-339. ( 10.1016/j.jtbi.2008.12.007) [DOI] [PubMed] [Google Scholar]
- 42.Smaldino PE, O'Connor C. 2022. Interdisciplinarity can aid the spread of better methods between scientific communities. Collective Intell. 1, 26339137221131816. ( 10.1177/26339137221131816) [DOI] [Google Scholar]
- 43.Boyd R, Gintis H, Bowles S, Richerson PJ. 2003. The evolution of altruistic punishment. Proc. Natl Acad. Sci. USA 100, 3531-3535. ( 10.1073/pnas.0630443100) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 44.Garcia J, van den Bergh JCJM. 2011. Evolution of parochial altruism by multilevel selection. Evol. Hum. Behav. 32, 277-287. ( 10.1016/j.evolhumbehav.2010.07.007) [DOI] [Google Scholar]
- 45.Makowsky MD, Smaldino PE. 2016. The evolution of power and the divergence of cooperative norms. J. Econ. Behav. Org. 126, 75-88. ( 10.1016/j.jebo.2015.09.002) [DOI] [Google Scholar]
- 46.Bowles S, Choi JK. 2003. The evolution of love and hate. Univ. Siena Econ. Working Pap., no. 401.
- 47.Choi JK. 2008. Play locally, learn globally: the structural basis of cooperation. J. Bioecon. 3, 239-257. ( 10.1007/s10818-008-9039-4) [DOI] [Google Scholar]
- 48.Janssen MA, Rollins ND. 2012. Evolution of cooperation in asymmetric commons dilemmas. J. Econ. Behav. Org. 81, 220-229. ( 10.1016/j.jebo.2011.10.010) [DOI] [Google Scholar]
- 49.Rogers AR. 1990. Group selection by selective emigration: the effects of migration and kin structure. Am. Nat. 135, 398-413. ( 10.1086/285053) [DOI] [Google Scholar]
- 50.Gneezy A, Fessler DM. 2011. Conflict, sticks and carrots: war increases prosocial punishment and rewards. Proc. R. Soc. B 279, 219-223. ( 10.1098/rspb.2011.0805) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 51.Turchin P, Currie TE, Turner E, Gavrilets S. 2013. War, space, and the evolution of Old World complex societies. Proc. Natl Acad. Sci. USA 110, 16 384-16 389. ( 10.1073/pnas.1308825110) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Henrich J, Boyd R, Young P, McCabe K, Alberts W, Ockenfelds A, Gigerenzer G. 2001. What is the role of culture in bounded rationality. In Bounded rationality: the adaptive toolbox (eds Gigerenzer G, Selten R), pp. 344-359. Cambridge, MA: MIT Press. [Google Scholar]
- 53.Laland KN. 2004. Social learning strategies. Learn. Behav. 32, 4-14. ( 10.3758/BF03196002) [DOI] [PubMed] [Google Scholar]
- 54.Houston AI. 1993. Mobility limits cooperation. Trends Ecol. Evol. 8, 194-196. ( 10.1016/0169-5347(93)90096-8) [DOI] [PubMed] [Google Scholar]
- 55.Price GR. 1970. Selection and covariance. Nature 227, 520-521. ( 10.1038/227520a0) [DOI] [PubMed] [Google Scholar]
- 56.van Veelen M. 2005. On the use of the Price equation. J. Theor. Biol. 237, 412-426. ( 10.1016/j.jtbi.2005.04.026) [DOI] [PubMed] [Google Scholar]
- 57.van Veleen M. 2009. Group selection, kin selection, altruism and cooperation: when inclusive fitness is right and when it can be wrong. J. Theor. Biol. 259, 589-600. ( 10.1016/j.jtbi.2009.04.019) [DOI] [PubMed] [Google Scholar]
- 58.van Veleen M. 2019. The problem with the Price equation. Phil. Trans. R. Soc. B 375, 20190355. ( 10.1098/rstb.2019.0355) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 59.Bowles S, Gintis H. 2011. A cooperative species. Princeton, NJ: Princeton University Press.
- 60.Price GR. 1972. Extension of covariance selection mathematics. Ann. Hum. Genet. 35, 485-490. ( 10.1111/j.1469-1809.1957.tb01874.x) [DOI] [PubMed] [Google Scholar]
- 61.Maynard Smith J. 1976. Group selection. Q. Rev. Biol. 51, 277-283. ( 10.1086/409311) [DOI] [Google Scholar]
- 62.Santos FC, Pacheco JM. 2011. Risk of collective failure provides an escape from the tragedy of the commons. Proc. Natl Acad. Sci. USA 108, 10 421-10 425. ( 10.1073/pnas.1015648108) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 63.Kohler TA, Cockburn D, Hooper PL, Bocinsky K, Kobti Z. 2012. The coevolution of group size and leadership: an agent-based public goods model for pre-Hispanic pueblo societies. Adv. Complex Syst. 15, 1150007. ( 10.1142/S0219525911003256) [DOI] [Google Scholar]
- 64.Hauser OP, Hilbe C, Chatterjee K, Nowak MA. 2019. Social dilemmas among unequals. Nature 572, 524-527. ( 10.1038/s41586-019-1488-5) [DOI] [PubMed] [Google Scholar]
- 65.Ruttan LM, Borgerhoff Mulder M. 1999. Are East African pastoralists truly conservationists? Curr. Anthropol. 40, 621-652. ( 10.1086/300086) [DOI] [PubMed] [Google Scholar]
- 66.Safarzynska K, Smaldino PE. 2023. Reducing global inequality increases local cooperation: a simple model of group selection with a global externality. OSF Science Framework repository. (https://osf.io/eb3gy/) [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
The code for replication is available from the Open Science Framework repository: https://osf.io/eb3gy/ [66].