Abstract
The development of quantum simulators, artificial platforms where the predictions of many-body theories of correlated quantum materials can be tested in a controllable and tunable way, is one of the main challenges of condensed matter physics. Here we introduce artificial lattices made of lead halide perovskite nanocubes as a new platform to simulate and investigate the physics of correlated quantum materials. We demonstrate that optical injection of quantum confined excitons in this system realizes the two main features that ubiquitously pervade the phase diagram of many quantum materials: collective phenomena, in which long-range orders emerge from incoherent fluctuations, and the excitonic Mott transition, which has one-to-one correspondence with the insulator-to-metal transition described by the repulsive Hubbard model in a magnetic field. Our results demonstrate that time-resolved experiments provide a quantum simulator that is able to span a parameter range relevant for a broad class of phenomena, such as superconductivity and charge-density waves.
Keywords: quantum simulation, halide perovskite nanocube superlattices, superradiance, Mott transition
The paradigm of quantum simulations1,2 has been pioneered by the development of ultracold-atom systems3−6 and extended to the solid state via nano- and heterostructured1,7−9 devices and, more recently, twisted bidimensional materials.10−13 An additional promising path consists in coupling a quantum material with the photons of a cavity,14,15 which opens the possibility to optically drive and control the emergence of collective phenomena and long-range coherence. Intense efforts are currently being dedicated to the development of photonics-based platforms aimed at replicating the many-body physics of quantum correlated materials. External optical control of the microscopic parameters entering the relevant Hamiltonian, such as doping, hopping, and interaction strength, is key to tackle open problems relevant to solid-state physics.
The most fundamental properties of many correlated materials, such as copper oxides,16 are captured by the Hubbard model, which describes charges moving in a lattice and subject to an on-site Coulomb repulsion U. When U is of the order of the bandwidth (4t, t being the hopping), the Hubbard model reproduces the correlation-driven metal-to-insulator Mott transition, thus capturing the insulating nature of copper oxide parent compounds.17 When a small number of free carriers is injected (doping), the model hosts the emergence of a wealth of long-range phases,18,19 such as charge density waves and high-temperature superconductivity, characterized by the macroscopic phase locking of fundamental incoherent fluctuations. More recently, the introduction of light-excitation protocols has unveiled the possibility of inducing nonequilibrium superconducting-like states20 or enhanced charge density waves,21 whose relation with equilibrium thermodynamical phases is still not clarified. The understanding of these phenomena in real materials is still a major challenge that is driving the search for synthetic systems where to reproduce the physics of doped Mott insulators and investigate, in a controlled way, the manifestation of long-range collective phenomena in both equilibrium and nonequilibrium conditions mediated by long-range electromagnetic interactions.
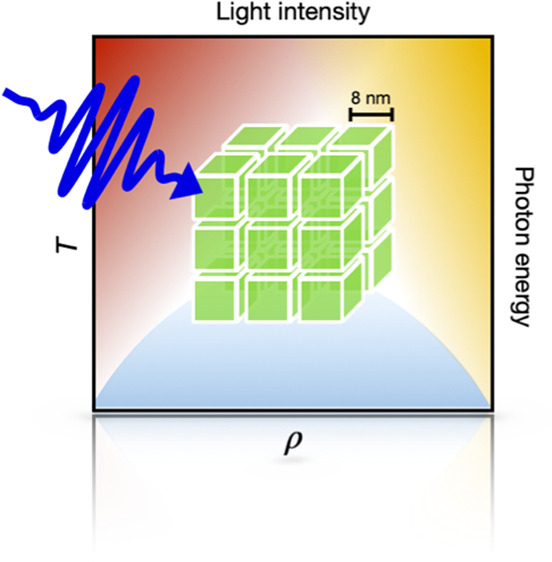
In this work, we introduce macroscopic lattices constituted by lead halide perovskite nanocubes as a new photonic platform to artificially implement the Hubbard model, which describes the local excitonic physics within each nanocube. At the same time, the long-range interactions among excitons in different nanocubes drive the emergence of collective phase-coherent states. We perform broadband time-resolved optical measurements and demonstrate the possibility of spanning different quantum phases, namely, the excitonic Mott insulating phase, the super-radiant collective state, and the metallic electron–hole liquid phase, on the same artificial solid system by continuously tuning the light excitation intensity.
Lead halide perovskite nanocubes (NC) feature appealing optoelectronic properties,22−24 characterized by great tunability and quantum confined excitons,25−28 and can self-organize into a highly ordered three-dimensional superlattice.29,30 The use of these artificial solids as a platform to simulate the many-body problems that characterize quantum correlated materials is based on the possibility of simultaneously controlling short-range correlations, which determine the Mott insulating ground state, and long-range interactions, which are responsible for the emergence of collective phases such as superconductivity or charge-density waves. To this purpose, we introduce the total Hamiltonian:
| 1 |
where 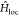 describes the local interactions
within
each single nanocube and is defined on the perovskite cubic lattice
with periodicity a, whereas
describes the local interactions
within
each single nanocube and is defined on the perovskite cubic lattice
with periodicity a, whereas 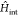 describes the interunit cell interactions
and is defined on the superlattice with periodicity Λ > a (see Figure 1).
describes the interunit cell interactions
and is defined on the superlattice with periodicity Λ > a (see Figure 1).
Figure 1.
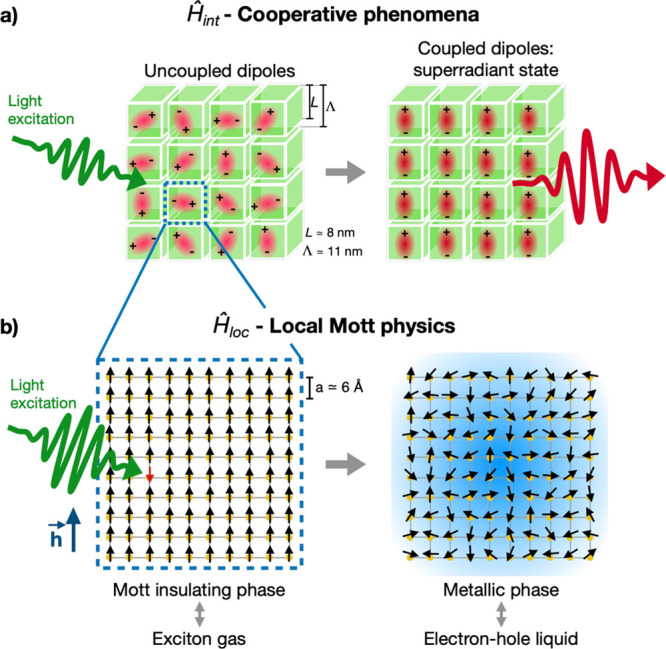
Cartoon describes how perovskite nanocube artificial solids can
be harnessed to simulate cooperative phases in the vicinity of the
Mott insulator-to-metal transition. (a) Cooperative effects, such
as super-radiance, emerge from long-range interactions 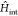 when coupled via an external light field.
(b) Local interactions
when coupled via an external light field.
(b) Local interactions 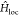 govern the photoinduced transition from
an exciton gas and an electron–hole liquid in a semiconductor,
realizing a Mott transition that can be fully mapped onto a repulsive
Hubbard model in a magnetic field h.
govern the photoinduced transition from
an exciton gas and an electron–hole liquid in a semiconductor,
realizing a Mott transition that can be fully mapped onto a repulsive
Hubbard model in a magnetic field h.
The first element to realize 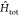 is given by the electromagnetic
field that
drives the long-range interaction among excitons in different nanocubes,
which can be expressed by31−33
is given by the electromagnetic
field that
drives the long-range interaction among excitons in different nanocubes,
which can be expressed by31−33
| 2 |
where 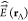 is the
electromagnetic field and
is the
electromagnetic field and 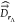 the dipole operator at the superlattice
site rΛ. If the electromagnetic
field is traced out, eq 2 can be written as an effective dipole–dipole interaction
term, whose strength is characterized by a long-range |rΛ,n – rΛ,m|–1 decay,32 |rΛ,n – rΛ,m| being
the distance between the nth and mth dipoles. The
the dipole operator at the superlattice
site rΛ. If the electromagnetic
field is traced out, eq 2 can be written as an effective dipole–dipole interaction
term, whose strength is characterized by a long-range |rΛ,n – rΛ,m|–1 decay,32 |rΛ,n – rΛ,m| being
the distance between the nth and mth dipoles. The 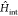 term is responsible for a rich family of
collective phenomena, broadly indicated as super-radiant.34 As sketched in Figure 1a, super-radiance occurs when the electromagnetic-field-driven
interaction leads to phase coherence of N quantum
emitters. A typical manifestation is the collective emission of radiation
(superfluorescence), which is both enhanced and faster than the emission
from individual nanocubes, with the radiative rate scaling as N2 for large N.34,35 Groundbreaking photoluminescence (PL) experiments recently reported
evidence of superfluorescence effects in halide perovskites.30,36−41 The main manifestations of this collective phenomenon are (i) the
superlinear dependence of the emission amplitude with respect to the
intensity of the exciting external field;30,36,37,39 (ii) the emergence
of a narrow red-shifted peak in the PL spectrum, which is assigned
to the cooperative emission from a subpopulation of nanocubes within
a single superlattice.30,36−39 These cooperative effects are
suppressed at high temperatures in nanocube superlattices due to thermal
noise that undermines quantum coherence.32?
term is responsible for a rich family of
collective phenomena, broadly indicated as super-radiant.34 As sketched in Figure 1a, super-radiance occurs when the electromagnetic-field-driven
interaction leads to phase coherence of N quantum
emitters. A typical manifestation is the collective emission of radiation
(superfluorescence), which is both enhanced and faster than the emission
from individual nanocubes, with the radiative rate scaling as N2 for large N.34,35 Groundbreaking photoluminescence (PL) experiments recently reported
evidence of superfluorescence effects in halide perovskites.30,36−41 The main manifestations of this collective phenomenon are (i) the
superlinear dependence of the emission amplitude with respect to the
intensity of the exciting external field;30,36,37,39 (ii) the emergence
of a narrow red-shifted peak in the PL spectrum, which is assigned
to the cooperative emission from a subpopulation of nanocubes within
a single superlattice.30,36−39 These cooperative effects are
suppressed at high temperatures in nanocube superlattices due to thermal
noise that undermines quantum coherence.32?
The second
element necessary to reproduce the physics of correlated
materials is described by 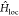 . It is well-known that short-range electronic
interactions can give rise to a transition from an exciton gas (EG)
to a liquid of weakly interacting electrons and holes (EHL), achieved
when a very large number of excitons is photoinjected in bulk and
low-dimensional semiconductors.42−46 This transition is believed to almost perfectly realize the insulator-to-metal
Mott transition, i.e., a transition driven by the weakening of the
electronic interactions without any symmetry breaking. It has been
demonstrated47,48 that the Hamiltonian describing
the EG → EHL transition in photoexcited semiconductors has
a one-to-one correspondence (see Supporting Information section S1) with the repulsive Hubbard model
. It is well-known that short-range electronic
interactions can give rise to a transition from an exciton gas (EG)
to a liquid of weakly interacting electrons and holes (EHL), achieved
when a very large number of excitons is photoinjected in bulk and
low-dimensional semiconductors.42−46 This transition is believed to almost perfectly realize the insulator-to-metal
Mott transition, i.e., a transition driven by the weakening of the
electronic interactions without any symmetry breaking. It has been
demonstrated47,48 that the Hamiltonian describing
the EG → EHL transition in photoexcited semiconductors has
a one-to-one correspondence (see Supporting Information section S1) with the repulsive Hubbard model 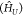 in a magnetic field, in which
the electron
spins play the role of the electron–hole excitations:48
in a magnetic field, in which
the electron
spins play the role of the electron–hole excitations:48
| 3 |
where
| 4 |
niσ is the occupation number at site i of electrons
with spin ↑ and ↓; 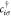 and ciσ are creation and annihilation operators for an electron of
spin σ at site i.
and ciσ are creation and annihilation operators for an electron of
spin σ at site i. 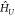 is a repulsive Hubbard model, identical
to that capturing the insulator-to-metal Mott transition in transition
metal oxides16,49 and the insulating ground state
of cuprate parent compounds.17 In this
effective description, the total number of excitons (neh) is mapped into the number of flipped spins with respect
to the ferromagnetic background, i.e., neh = ∑i⟨ni↓⟩/N (N being the total number of particles). The exciton
density is therefore controlled by the Lagrange multiplier h, which acts as an auxiliary magnetic field inducing the
effective magnetization m = 1–2neh. For large h, the auxiliary repulsive
model is fully polarized (m = 1), which corresponds
to the absence of excitons in the original model (eq S1). The sudden reduction of h, which
injects a finite number of spin excitations (m <
1), mimics the sudden photoinjection of excitons (neh > 0).
is a repulsive Hubbard model, identical
to that capturing the insulator-to-metal Mott transition in transition
metal oxides16,49 and the insulating ground state
of cuprate parent compounds.17 In this
effective description, the total number of excitons (neh) is mapped into the number of flipped spins with respect
to the ferromagnetic background, i.e., neh = ∑i⟨ni↓⟩/N (N being the total number of particles). The exciton
density is therefore controlled by the Lagrange multiplier h, which acts as an auxiliary magnetic field inducing the
effective magnetization m = 1–2neh. For large h, the auxiliary repulsive
model is fully polarized (m = 1), which corresponds
to the absence of excitons in the original model (eq S1). The sudden reduction of h, which
injects a finite number of spin excitations (m <
1), mimics the sudden photoinjection of excitons (neh > 0).
The physics generated by 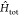 is here investigated
by broadband transient
reflectivity measurements. We performed experiments on artificial
lattices constituted by L = 8 nm CsPbBr3 nanocubes (Bohr exciton diameter ∼7 nm27) arranged
in cubic superlattices of periodicity Λ = L + l = 11 nm, where l is the thickness
of the ligand layer between two neighboring NCs. The size of each
superlattice is of the order of a few micrometers (1–10 μm).
Ultrashort light pulses are used to impulsively inject optical excitons,
whose density is controlled by the light intensity. The broadband
probe (2.1–2.5 eV photon energy) measures the femto/picosecond
time evolution of the optical properties following the impulsive excitation.
In particular, we employ a resonant pumping scheme in which the pump
photon energy (≃2.41 eV) is tuned to the exciton energy, thus
limiting the direct generation of free carriers in the conduction
band.
is here investigated
by broadband transient
reflectivity measurements. We performed experiments on artificial
lattices constituted by L = 8 nm CsPbBr3 nanocubes (Bohr exciton diameter ∼7 nm27) arranged
in cubic superlattices of periodicity Λ = L + l = 11 nm, where l is the thickness
of the ligand layer between two neighboring NCs. The size of each
superlattice is of the order of a few micrometers (1–10 μm).
Ultrashort light pulses are used to impulsively inject optical excitons,
whose density is controlled by the light intensity. The broadband
probe (2.1–2.5 eV photon energy) measures the femto/picosecond
time evolution of the optical properties following the impulsive excitation.
In particular, we employ a resonant pumping scheme in which the pump
photon energy (≃2.41 eV) is tuned to the exciton energy, thus
limiting the direct generation of free carriers in the conduction
band.
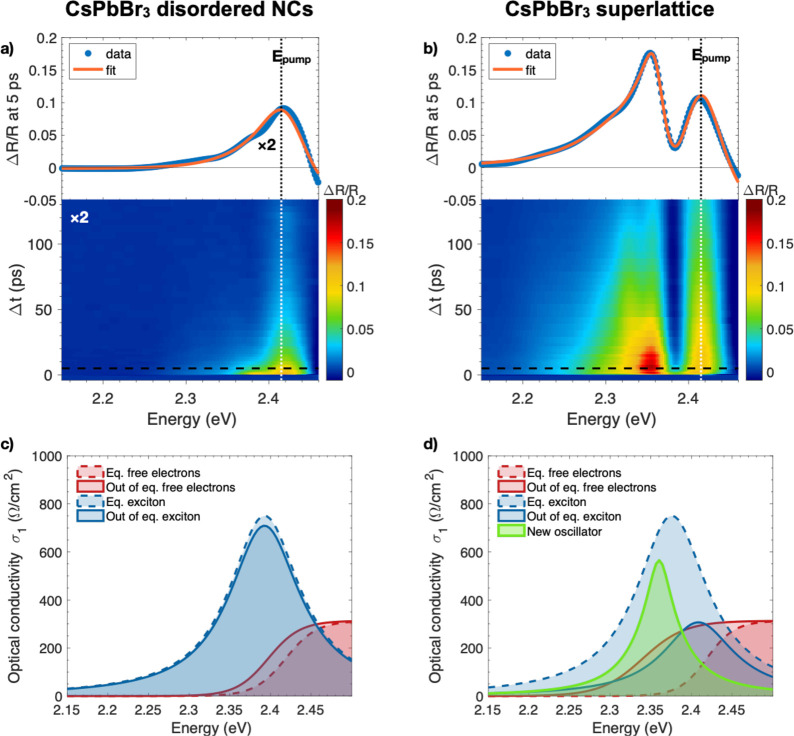
Figure 2 panels a and b display the typical data collected from pump–probe experiments (see Supporting Information section S5 for experimental details) on CsPbBr3 disordered NCs and NC superlattices, respectively. For the disordered sample (see Figure 2a), the transient reflectivity signal ΔR/R is characterized by a positive reflectivity variation of the order of 5% centered at 2.41 eV. The same experiment performed on NC superlattices (see Figure 2b) displays a larger signal amplitude, with a similar spectral response around 2.41 eV and, additionally, a more structured spectral response extending down to ≃2.20 eV probe energy. The origin of these structures in the ΔR/R signal is assessed by performing a differential fit, which consists of modifying the parameters of the model describing the equilibrium optical properties that are responsible for the observed reflectivity variation. The starting point is the equilibrium optical conductivity that is obtained from a Kramers-Krönig constrained model matching the experimental absorbance of the samples and the temperature dependent trends reported in the literature (Supporting Information S6). In the 2–2.5 eV energy range, the model is dominated by the conduction band edge absorption (red dashed line in Figure 2c) and the exciton peak (blue dashed line), modeled through a Drude-Lorentz oscillator. The outcome of the differential fitting procedure is depicted in Figure 2 panels c and d, where the solid lines represent the out-of-equilibrium components of the optical conductivity σ1 necessary to fit the experimental ΔR/R signal (see the orange solid lines in the top panels of Figure 2a,b and Supporting Information S8 for details about the robustness of the fitting procedure). In order to reproduce the measured spectral response of CsPbBr3, for both samples it is necessary to assume (i) a decrease of the excitonic spectral weight and a concomitant blue-shift of the exciton energy and (ii) an increase of in-gap free-electron states accounted for by a red-shift of the semiconducting band gap. In addition to the (i,ii) contributions observed in both samples, the feature observed in the 2.2–2.4 eV energy range solely for NC superlattices (see Figure 2b) requires an additional narrow structure (iii) that we model through a new oscillator (green line in Figure 2d) appearing in the out-of-equilibrium optical conductivity.
Figure 2.
Ultrafast transient reflectivity of CsPbBr3 disordered NCs sample (left panels) and NC superlattice sample (right panels), measured at 17 K, 230 μJ/cm2 excitation fluence and pump photon energy resonant with the excitonic line, i.e., Epump = 2.41 eV. The bottom (a) and (b) panels report the two-dimensional pump–probe maps displaying the ΔR/R signal (see color scale on the right) as a function of the delay (Δt) and probe photon energy. The top panels (a) and (b) report the ΔR/R signal (blue dots) as a function of the probe photon energy at fixed delay time, Δt = 5 ps (horizontal dashed line in the color maps). The orange solid lines represent the differential fit to the data. (c) and (d) optical conductivity (σ1) at equilibrium (dashed lines) and out-of-equilibrium at Δt = 5 ps (solid lines) obtained from experimental absorbance and fit of ΔR/R spectra. The colors represent the different contributions to the total optical conductivity: (i) main excitonic line (blue); (ii) across gap optical transitions (red); and the photoinduced peak emerging at low temperature in ordered NC superlattices (green).
The narrow additional peak (green area in Figure 2d) emerging out-of-equilibrium in NC superlattices features characteristics very similar to the superfluorescence recently observed in perovskite superlattices by means of low-temperature photoluminescence.36,37 While the detailed analysis of this new resonance is discussed in Supporting Information section S9, here we only summarize the main results that allow us to assess its cooperative origin. First, the new resonance appears as a narrower and red-shifted peak as compared to the equilibrium excitonic line. The new peak has a ≃ 40 meV line width, to be compared to the 100 meV width of the main excitonic line, and is centered around 2.36 eV, corresponding to a red-shift δ with respect to the instantaneous position of the main excitonic resonance. By comparing results obtained on nine different NC superlattice samples with the same nominal characteristics, we obtain a value of δ that varies between 40 and 80 meV. Second, a detailed fluence (F) dependence (see Figure S17b) shows peculiar behaviors of the different spectral features in ΔR/R for F < 150–200 μJ/cm2. For photon energies ℏω > 2.37 eV, i.e., far from the photoinduced additional peak, the transient reflectivity scales with a power law with exponent (0.91 ± 0.07), which corresponds to a linear behavior. In contrast, the spectral region corresponding to the additional photoinduced peak (ℏω < 2.37 eV) features a clearly superlinear fluence dependence, corresponding to a superlinear exponent (1.43 ± 0.05), in agreement with what is expected for super-radiant phenomena.30,36,39,41 It is useful to compare the present results with those obtained on disordered NCs, in which collective super-radiant phenomena should be quenched50−52 due to disorder-driven dephasing. As reported in the Supporting Information section S9, in this case, the fluence-dependence of the signal is always linear, independent of the energy region considered. Third, the reflectivity variation corresponding to the spectral feature at 2.36 eV is considerably reduced at room temperature. The temperature-dependent data (Figure S17c) report a suppression of the photoinduced peak at temperatures as high as 200 K, which is compatible with the thermally driven loss of coherence of super-radiant emitters.32
The physics of the excitonic Mott transition from
EG to EHL can
be experimentally accessed in the high photodoping limit, which is
achieved at light fluences on the order of ∼200 μJ/cm2. As shown above, in this regime the transient optical response
of NC superlattices is dominated by a decrease of the excitonic spectral
weight and the corresponding increase of in-gap free electron states,
as captured by the effective red-shift of the semiconducting gap (see Figures 2d and 3a). The transient increase of in-gap states at the expenses
of the intensity of the exciton peak demonstrates that, at high fluence,
the NC superlattices no longer support well-defined excitons but rather
delocalized electron–hole excitations. To assess the nature
of this high-excitation regime, we calculated from the differential
model (see Supporting Information S8) the
spectral weight variation associated with the direct across-gap transitions
ΔSWfree. At Δt = 5 ps we obtain ΔSWfree/SWexc ≃ 0.2, with SWexc being the spectral weight of the excitonic peak already
present in the equilibrium optical conductivity, which indicates that
approximately 20% of the initial SWexc is transferred into in-gap free electrons states. This spectral
weight transfer can be considered as the direct signature of the excitonic
Mott transition, which is ubiquitously characterized by the appearance
of new in-gap metallic states at the expenses of the correlated lower
and upper Hubbard bands, corresponding to a single localized exciton
and delocalized electron–hole excitations separated by the
binding energy Eb (see Figure 3b).48 The time-resolved dynamics contains important information about
the temporal evolution of the newly created metallic states and the
recovery of the initial excitonic gas. Since during the relaxation
the excitonic density neh decreases due
to the slow recombination across the semiconducting gap, at some time
the system will undergo the transition from the photoinduced EHL state
back to the EG insulating phase. In Figure 3c we plot the temporal evolution of the fraction
of the excitonic spectral weight that is transferred to the free carrier
states. At low fluence (∼30 μJ/cm2, green
markers in Figure 3c), the spectral weight of the photoinduced metallic states is very
limited and exponentially decays with a time scale of 130 ps (black
solid line in Figure 3c). This slow relaxation is in agreement with what expected for the
recombination of electrons and holes across the gap and with the fluorescence
time scale.36,53 At large fluences (∼230
μJ/cm2, red markers in Figure 3c), we observe additional spectral weight
variation, which exceeds that present in the low-fluence data. This
additional ΔSWfree component rapidly
relaxes with a time scale of ∼20 ps, thus allowing us to estimate
the critical number of excitations necessary for re-establishing the
insulating EG phase. Assuming that neh spontaneously decays with the time scale of 130 ps, the change in
slope of the ΔSWfree/SWexc dynamics at ∼20 ps corresponds to a threshold
value 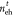 = 0.5% (see section S7). A similar conclusion is obtained by analyzing the data
on disordered NCs, which display a similar change in the slope of
the ΔSWfree/SWexc dynamics (see Figure 3c).
= 0.5% (see section S7). A similar conclusion is obtained by analyzing the data
on disordered NCs, which display a similar change in the slope of
the ΔSWfree/SWexc dynamics (see Figure 3c).
Figure 3.
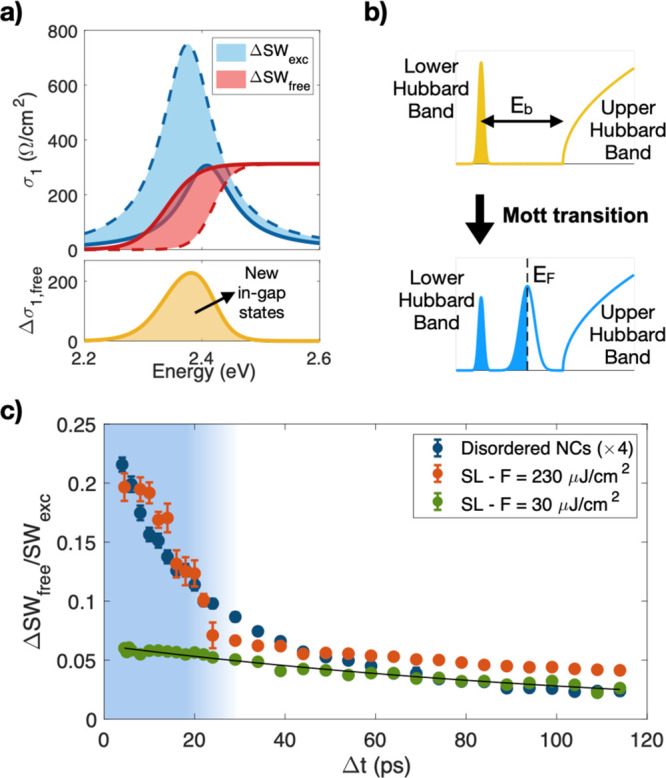
(a) Top panel: plot of the equilibrium (dashed lines) and out-of-equilibrium (solid lines) optical conductivity obtained from fitting the experimental data at Δt = 5 ps. The blue filled area represents the excitonic spectral weight decrease, which is counterbalanced by the appearance of new states below the conduction band minimum, described by a red-shift of the band edge (red filled area). Bottom panel: difference between out-of-equilibrium and equilibrium σ1,free, which represents the contribution from the conduction band states to the optical conductivity. (b) In terms of the excitonic problem, the insulating phase of the magnetized Hubbard model maps the existence of well-defined localized excitonic states (lower Hubbard band), separated from the upper Hubbard band by an energy amount Eb. When the excitonic density neh exceeds a critical value, the Mott transition to a liquid of delocalized electrons and holes takes place and is characterized by the emergence of metallic states at the Fermi level. (c) Time evolution of the spectral weight transfer from the exciton state to free carrier states, estimated as ΔSWfree/SWexc. The black line denotes an exponentially decaying function fitted to the data. The blue area highlights the region where an excess of ΔSWfree is observed for a large excitation fluence.
The experiments presented
above allow access to a region of the
zero-temperature phase diagram of 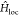 +
+  that is relevant for describing real correlated
materials.49,54 As discussed in ref (48), the parameters controlling
the electronic phases of photoexcited halide perovskites are the excitation
density neh, which is mapped into the
magnetization m through
that is relevant for describing real correlated
materials.49,54 As discussed in ref (48), the parameters controlling
the electronic phases of photoexcited halide perovskites are the excitation
density neh, which is mapped into the
magnetization m through 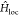 , and the binding energy Eb, which is mapped into the Coulomb repulsion U. For small excitation densities (neh < 1%) and moderately large Coulomb repulsion (U > 3.8t, with t being
proportional to the hopping parameter, following the notation of ref (48)), the phase diagram is
characterized by the boundary between an excitonic insulating gas
and a mixed state with phase separation between EG and EHL, as shown
in Figure 4.48 The resonant excitation of the excitonic line
directly modifies neh without creating
an additional effective electron–hole population, which would
require a finite temperature description. At the same time, the excitonic
energy undergoes a transient blueshift, δEb (see Figure 2 and related discussion), that is, maximum at short delays (∼5
ps) and progressively decreases as the system relaxes and returns
to the initial state. The observed blue shift is likely related to
a dynamical weakening of the exciton binding energy as a consequence
of the increased screening after the light excitation. The determined
time-dependent values of neh and δEb define a trajectory
in the phase diagram, in which the energy scales are expressed as
a function of the unknown effective hopping t. The
determination of
, and the binding energy Eb, which is mapped into the Coulomb repulsion U. For small excitation densities (neh < 1%) and moderately large Coulomb repulsion (U > 3.8t, with t being
proportional to the hopping parameter, following the notation of ref (48)), the phase diagram is
characterized by the boundary between an excitonic insulating gas
and a mixed state with phase separation between EG and EHL, as shown
in Figure 4.48 The resonant excitation of the excitonic line
directly modifies neh without creating
an additional effective electron–hole population, which would
require a finite temperature description. At the same time, the excitonic
energy undergoes a transient blueshift, δEb (see Figure 2 and related discussion), that is, maximum at short delays (∼5
ps) and progressively decreases as the system relaxes and returns
to the initial state. The observed blue shift is likely related to
a dynamical weakening of the exciton binding energy as a consequence
of the increased screening after the light excitation. The determined
time-dependent values of neh and δEb define a trajectory
in the phase diagram, in which the energy scales are expressed as
a function of the unknown effective hopping t. The
determination of 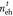 = 0.5% as the density threshold for the
instability of the EG, allows us to anchor the time-dependent trajectory
and fix the range of the U/2t values
spanned by the time-resolved experiment. Figure 4 shows the trajectory in the phase diagram
for the high-fluence experiment. At very short delays, the NC superlattices
are driven into a nonequilibrium state corresponding to U/2t ≈ 1.6 and neh = 0.6%, which is characterized by phase separation between insulating
EG and metallic EHL regions. In this regime, cooperative phenomena
start to be quenched (see Figure S17b)
due to the progressive growth of metallic domains. During the relaxation
dynamics, the system undergoes a dynamical transition back to the
EG insulating phase before the initial parameters (U/2t ≈ 2.8 and neh = 0) are recovered on longer time scales. When combined together,
our results demonstrate the possibility to explore the region of the
magnetized Hubbard model
= 0.5% as the density threshold for the
instability of the EG, allows us to anchor the time-dependent trajectory
and fix the range of the U/2t values
spanned by the time-resolved experiment. Figure 4 shows the trajectory in the phase diagram
for the high-fluence experiment. At very short delays, the NC superlattices
are driven into a nonequilibrium state corresponding to U/2t ≈ 1.6 and neh = 0.6%, which is characterized by phase separation between insulating
EG and metallic EHL regions. In this regime, cooperative phenomena
start to be quenched (see Figure S17b)
due to the progressive growth of metallic domains. During the relaxation
dynamics, the system undergoes a dynamical transition back to the
EG insulating phase before the initial parameters (U/2t ≈ 2.8 and neh = 0) are recovered on longer time scales. When combined together,
our results demonstrate the possibility to explore the region of the
magnetized Hubbard model 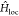 that is the most relevant to describe many-body
effects in correlated materials (U/t = 3.2–5.6). Although in the present case we access the insulator-to-metal
transition in
that is the most relevant to describe many-body
effects in correlated materials (U/t = 3.2–5.6). Although in the present case we access the insulator-to-metal
transition in 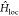 only in the presence
of a magnetic field h, the observed phenomenology
is a very general property
of correlated materials, such as iron-based superconductors, superconducting
copper oxides and fullerides, which develop superconductivity and
other long-range collective phases, e.g., charge density waves, nematicity,
antiferromagnetism, and spin density waves, when the Mott insulating
state is lightly doped.19,55
only in the presence
of a magnetic field h, the observed phenomenology
is a very general property
of correlated materials, such as iron-based superconductors, superconducting
copper oxides and fullerides, which develop superconductivity and
other long-range collective phases, e.g., charge density waves, nematicity,
antiferromagnetism, and spin density waves, when the Mott insulating
state is lightly doped.19,55
Figure 4.
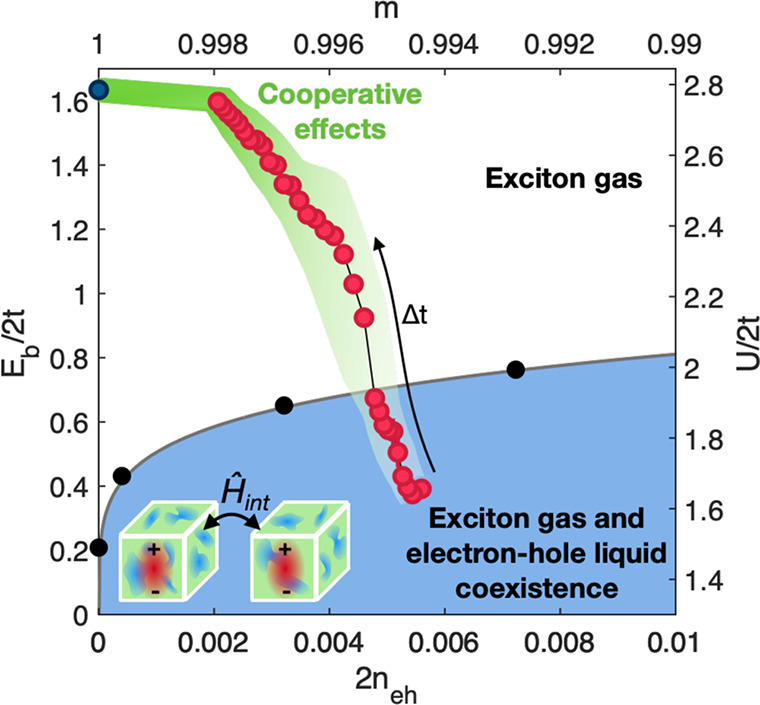
Phase diagram showing the electron gas (EG) and electron–hole liquid (EHL) regions. The electron–hole density 2neh and the exciton binding energy Eb correspond to magnetization m and on-site interaction U in the equivalent Hubbard model. The black points are taken from ref (48). The red dots are the experimental data points representing the trajectory the system follows while relaxing to the equilibrium EG phase from the photoinduced EHL phase. The plotted values are the binding energy values extracted from the time-resolved fit of the pump–probe data on NC superlattices at 230 μJ/cm2 fluence, as a function of the estimated electron–hole density at the corresponding Δt. The green shaded area represents the phase-space region compatible with the outputs of the time-resolved experiment when the error bars associated with neh and Eb are considered.
We have demonstrated here that halide perovskite NC artificial solids represent a unique platform to investigate the emergence of long-range cooperative phases in lightly doped Mott insulators. Future nonresonant experiments will allow to directly create a nonequilibrium electron–hole population at a very high effective temperature, thus providing a platform to simulate the magnetized Hubbard model at finite temperatures and with controllable disorder and lattice size. The possibility to manipulate local correlations and long-range orders on similar time (5–100 ps) and spatial (Λ ≃ 10a) scales can thus trigger the development of fully tunable synthetic quantum correlated materials. As compared to cold-atoms based or solid-state platforms, halide perovskite NC superlattices represent an easy to handle solution, which presents many interesting advantages: (i) they allow continuous tuning of the excitonic density and span collective phenomena and Mott insulating phases in a region of the phase diagram that is directly relevant for correlated quantum materials; (ii) the 1/r scaling of the effective long-range interaction drives a direct all-to-all coupling of a large number of dipoles, on the order of 106, in a spatially confined geometry; (iii) this platform can be easily integrated onto small-scale optoelectronic systems operating in the visible range; (iv) the spontaneous cavity formed by the superlattice itself40,56 can be naturally exploited to investigate long-range orders in cavity-coupled lattices.
Acknowledgments
C.G., M.C., P.F., A.M., S.M. acknowledge financial support from MIUR through the PRIN 2015 (Prot. 2015C5SEJJ001) and PRIN 2017 (Prot. 20172H2SC4_005) programs. C.G., S.P., and G.F. acknowledge support from Università Cattolica del Sacro Cuore through D.1, D.2.2 and D.3.1 grants. M.C. acknowledges financial support from MUR via PNRR MUR project PE0000023-NQSTI, PNNR National Center for HPC, Big Data, and Quantum Computing (grant No. CN00000013). S.M. acknowledges partial financial support through the grant ”Finanziamenti ponte per bandi esterni” from Università Cattolica del Sacro Cuore.
Supporting Information Available
The Supporting Information is available free of charge at https://pubs.acs.org/doi/10.1021/acs.nanolett.3c03715.
Theoretical mapping of the excitonic Mott transition; sample preparation; absorbance and photoluminescence spectra, XRD patterns and optical microscopy of disordered NCs and NCs superlattices samples; analysis of aging effects; description of the pump–probe experimental setup; details of the transient reflectivity data fitting; estimation of exciton density; discussion of the experimental evidence of cooperative effects (PDF)
The authors declare no competing financial interest.
Supplementary Material
References
- Altman E.; et al. Quantum simulators: Architectures and opportunities. PRX Quantum 2021, 2, 017003. 10.1103/PRXQuantum.2.017003. [DOI] [Google Scholar]
- Cirac J. I.; Zoller P. Goals and opportunities in quantum simulation. Nat. Phys. 2012, 8, 264–266. 10.1038/nphys2275. [DOI] [Google Scholar]
- Bloch I.; Dalibard J.; Zwerger W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885. 10.1103/RevModPhys.80.885. [DOI] [Google Scholar]
- Bloch I.; Dalibard J.; Nascimbene S. Quantum simulations with ultracold quantum gases. Nat. Phys. 2012, 8, 267–276. 10.1038/nphys2259. [DOI] [Google Scholar]
- Chien C.-C.; Peotta S.; Di Ventra M. Quantum transport in ultracold atoms. Nat. Phys. 2015, 11, 998–1004. 10.1038/nphys3531. [DOI] [Google Scholar]
- Freeney S. E.; Slot M. R.; Gardenier T. S.; Swart I.; Vanmaekelbergh D. Electronic Quantum Materials Simulated with Artificial Model Lattices. ACS Nanoscience Au 2022, 2, 198–224. 10.1021/acsnanoscienceau.1c00054. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bernevig B. A.; Hughes T. L.; Zhang S.-C. Quantum Spin Hall Effect and Topological Phase Transition in HgTe Quantum Wells. Science 2006, 314, 1757–1761. 10.1126/science.1133734. [DOI] [PubMed] [Google Scholar]
- Buluta I.; Nori F. Quantum Simulators. Science 2009, 326, 108–111. 10.1126/science.1177838. [DOI] [PubMed] [Google Scholar]
- Lagoin C.; Bhattacharya U.; Grass T.; Chhajlany R. W.; Salamon T.; Baldwin K.; Pfeiffer L.; Lewenstein M.; Holzmann M.; Dubin F. Extended Bose-Hubbard model with dipolar excitons. Nature 2022, 609, 485–489. 10.1038/s41586-022-05123-z. [DOI] [PubMed] [Google Scholar]
- Cao Y.; Fatemi V.; Demir A.; Fang S.; Tomarken S. L.; Luo J. Y.; Sanchez-Yamagishi J. D.; Watanabe K.; Taniguchi T.; Kaxiras E.; Ashoori R. C.; Jarillo-Herrero P. Correlated insulator behaviour at half-filling in magic-angle graphene superlattices. Nature 2018, 556, 80–84. 10.1038/nature26154. [DOI] [PubMed] [Google Scholar]
- Cao Y.; Fatemi V.; Fang S.; Watanabe K.; Taniguchi T.; Kaxiras E.; Jarillo-Herrero P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. 10.1038/nature26160. [DOI] [PubMed] [Google Scholar]
- Carr S.; Fang S.; Kaxiras E. Electronic-structure methods for twisted moiré layers. Nature Reviews Materials 2020, 5, 748–763. 10.1038/s41578-020-0214-0. [DOI] [Google Scholar]
- Kennes D. M.; Claassen M.; Xian L.; Georges A.; Millis A. J.; Hone J.; Dean C. R.; Basov D.; Pasupathy A. N.; Rubio A. Moiré heterostructures as a condensed-matter quantum simulator. Nat. Phys. 2021, 17, 155–163. 10.1038/s41567-020-01154-3. [DOI] [Google Scholar]
- Walther H.; Varcoe B. T.; Englert B.-G.; Becker T. Cavity quantum electrodynamics. Rep. Prog. Phys. 2006, 69, 1325. 10.1088/0034-4885/69/5/R02. [DOI] [Google Scholar]
- Haroche S.; Kleppner D. Cavity Quantum Electrodynamics. Phys. Today 1989, 42, 24–30. 10.1063/1.881201. [DOI] [Google Scholar]
- Imada M.; Fujimori A.; Tokura Y. Metal-insulator transitions. Rev. Mod. Phys. 1998, 70, 1039–1263. 10.1103/RevModPhys.70.1039. [DOI] [Google Scholar]
- Lee P. A.; Nagaosa N.; Wen X.-G. Doping a Mott insulator: Physics of high-temperature superconductivity. Rev. Mod. Phys. 2006, 78, 17–85. 10.1103/RevModPhys.78.17. [DOI] [Google Scholar]
- Sachdev S. Colloquium: Order and quantum phase transitions in the cuprate superconductors. Rev. Mod. Phys. 2003, 75, 913–932. 10.1103/RevModPhys.75.913. [DOI] [Google Scholar]
- Keimer B.; Kivelson S. A.; Norman M. R.; Uchida S.; Zaanen J. From quantum matter to high-temperature superconductivity in copper oxides. Nature 2015, 518, 179–186. 10.1038/nature14165. [DOI] [PubMed] [Google Scholar]
- Cavalleri A. Photo-induced superconductivity. Contemporary Physics 2018, 59, 31–46. 10.1080/00107514.2017.1406623. [DOI] [Google Scholar]
- Wandel S.; et al. Enhanced charge density wave coherence in a light-quenched, high-temperature superconductor. Science 2022, 376, 860–864. 10.1126/science.abd7213. [DOI] [PubMed] [Google Scholar]
- Fu Y.; Zhu H.; Chen J.; Hautzinger M. P.; Zhu X.-Y.; Jin S. Metal halide perovskite nanostructures for optoelectronic applications and the study of physical properties. Nature Reviews Materials 2019, 4, 169–188. 10.1038/s41578-019-0080-9. [DOI] [Google Scholar]
- Green M. A.; Ho-Baillie A.; Snaith H. J. The emergence of perovskite solar cells. Nat. Photonics 2014, 8, 506–514. 10.1038/nphoton.2014.134. [DOI] [Google Scholar]
- He C.; Liu X. The rise of halide perovskite semiconductors. Light: Science & Applications 2023, 12, 15. 10.1038/s41377-022-01010-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Shamsi J.; Urban A. S.; Imran M.; De Trizio L.; Manna L. Metal halide perovskite nanocrystals: synthesis, post-synthesis modifications, and their optical properties. Chem. Rev. 2019, 119, 3296–3348. 10.1021/acs.chemrev.8b00644. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Dey A.; Ye J.; De A.; Debroye E.; Ha S. K.; Bladt E.; Kshirsagar A. S.; Wang Z.; Yin J.; Wang Y.; et al. State of the art and prospects for halide perovskite nanocrystals. ACS Nano 2021, 15, 10775–10981. 10.1021/acsnano.0c08903. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Protesescu L.; Yakunin S.; Bodnarchuk M. I.; Krieg F.; Caputo R.; Hendon C. H.; Yang R. X.; Walsh A.; Kovalenko M. V. Nanocrystals of cesium lead halide perovskites (CsPbX3, X= Cl, Br, and I): novel optoelectronic materials showing bright emission with wide color gamut. Nano Lett. 2015, 15, 3692–3696. 10.1021/nl5048779. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sercel P. C.; Lyons J. L.; Bernstein N.; Efros A. L. Quasicubic model for metal halide perovskite nanocrystals. J. Chem. Phys. 2019, 151, 234106. 10.1063/1.5127528. [DOI] [PubMed] [Google Scholar]
- Brennan M. C.; Toso S.; Pavlovetc I. M.; Zhukovskyi M.; Marras S.; Kuno M.; Manna L.; Baranov D. Superlattices are greener on the other side: how light transforms self-assembled mixed halide perovskite nanocrystals. ACS Energy Letters 2020, 5, 1465–1473. 10.1021/acsenergylett.0c00630. [DOI] [Google Scholar]
- Cherniukh I.; Rainò G.; Stöferle T.; Burian M.; Travesset A.; Naumenko D.; Amenitsch H.; Erni R.; Mahrt R. F.; Bodnarchuk M. I.; Kovalenko M. V. Perovskite-type superlattices from lead halide perovskite nanocubes. Nature 2021, 593, 535–542. 10.1038/s41586-021-03492-5. [DOI] [PubMed] [Google Scholar]
- Mazza G.; Georges A. Superradiant Quantum Materials. Phys. Rev. Lett. 2019, 122, 017401. 10.1103/PhysRevLett.122.017401. [DOI] [PubMed] [Google Scholar]
- Mattiotti F.; Kuno M.; Borgonovi F.; Jankó B.; Celardo G. L. Thermal Decoherence of Superradiance in Lead Halide Perovskite Nanocrystal Superlattices. Nano Lett. 2020, 20, 7382–7388. 10.1021/acs.nanolett.0c02784. [DOI] [PubMed] [Google Scholar]
- Li J.; Schamriß L.; Eckstein M. Effective theory of lattice electrons strongly coupled to quantum electromagnetic fields. Phys. Rev. B 2022, 105, 165121. 10.1103/PhysRevB.105.165121. [DOI] [Google Scholar]
- Cong K.; Zhang Q.; Wang Y.; Noe G. T.; Belyanin A.; Kono J. Dicke superradiance in solids. Journal of the Optical Society of America B 2016, 33, C80–C101. 10.1364/JOSAB.33.000C80. [DOI] [Google Scholar]
- Dicke R. H. Coherence in spontaneous radiation processes. Phys. Rev. 1954, 93, 99. 10.1103/PhysRev.93.99. [DOI] [Google Scholar]
- Rainò G.; Becker M. A.; Bodnarchuk M. I.; Mahrt R. F.; Kovalenko M. V.; Stöferle T. Superfluorescence from lead halide perovskite quantum dot superlattices. Nature 2018, 563, 671–675. 10.1038/s41586-018-0683-0. [DOI] [PubMed] [Google Scholar]
- Findik G.; Biliroglu M.; Seyitliyev D.; Mendes J.; Barrette A.; Ardekani H.; Lei L.; Dong Q.; So F.; Gundogdu K. High-temperature superfluorescence in methyl ammonium lead iodide. Nat. Photonics 2021, 15, 676–680. 10.1038/s41566-021-00830-x. [DOI] [Google Scholar]
- Pashaei Adl H.; Gorji S.; Muñoz-Matutano G.; Gualdrón-Reyes A. F.; Suárez I.; Chirvony V. S.; Mora-Seró I.; Martínez-Pastor J. P. Superradiance Emission and Its Thermal Decoherence in Lead Halide Perovskites Superlattices. Advanced Optical Materials 2023, 11, 2202497. 10.1002/adom.202202497. [DOI] [Google Scholar]
- Krieg F.; Sercel P. C.; Burian M.; Andrusiv H.; Bodnarchuk M. I.; Stöferle T.; Mahrt R. F.; Naumenko D.; Amenitsch H.; Rainò G.; Kovalenko M. V. Monodisperse long-chain sulfobetaine-capped CsPbBr3 nanocrystals and their superfluorescent assemblies. ACS Central Science 2021, 7, 135–144. 10.1021/acscentsci.0c01153. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zhou C.; Zhong Y.; Dong H.; Zheng W.; Tan J.; Jie Q.; Pan A.; Zhang L.; Xie W. Cooperative excitonic quantum ensemble in perovskite-assembly superlattice microcavities. Nat. Commun. 2020, 11, 329. 10.1038/s41467-019-14078-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Biliroglu M.; Findik G.; Mendes J.; Seyitliyev D.; Lei L.; Dong Q.; Mehta Y.; Temnov V. V.; So F.; Gundogdu K. Room-temperature superfluorescence in hybrid perovskites and its origins. Nat. Photonics 2022, 16, 324–329. 10.1038/s41566-022-00974-4. [DOI] [Google Scholar]
- Palmieri T.; Baldini E.; Steinhoff A.; Akrap A.; Kollár M.; Horváth E.; Forró L.; Jahnke F.; Chergui M. Mahan excitons in room-temperature methylammonium lead bromide perovskites. Nat. Commun. 2020, 11, 1–8. 10.1038/s41467-020-14683-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Chernikov A.; Ruppert C.; Hill H. M.; Rigosi A. F.; Heinz T. F. Population inversion and giant bandgap renormalization in atomically thin WS2 layers. Nat. Photonics 2015, 9, 466–470. 10.1038/nphoton.2015.104. [DOI] [Google Scholar]
- Bataller A. W.; Younts R. A.; Rustagi A.; Yu Y.; Ardekani H.; Kemper A.; Cao L.; Gundogdu K. Dense electron–hole plasma formation and ultralong charge lifetime in monolayer MoS2 via material tuning. Nano Lett. 2019, 19, 1104–1111. 10.1021/acs.nanolett.8b04408. [DOI] [PubMed] [Google Scholar]
- Schlaus A. P.; Spencer M. S.; Miyata K.; Liu F.; Wang X.; Datta I.; Lipson M.; Pan A.; Zhu X.-Y. How lasing happens in CsPbBr3 perovskite nanowires. Nat. Commun. 2019, 10, 1–8. 10.1038/s41467-018-07972-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Yu Y.; Bataller A. W.; Younts R.; Yu Y.; Li G.; Puretzky A. A.; Geohegan D. B.; Gundogdu K.; Cao L. Room-temperature electron–hole liquid in monolayer MoS2. ACS Nano 2019, 13, 10351–10358. 10.1021/acsnano.9b04124. [DOI] [PubMed] [Google Scholar]
- Brinkman W. F.; Rice T. M. Electron-Hole Liquids in Semiconductors. Phys. Rev. B 1973, 7, 1508–1523. 10.1103/PhysRevB.7.1508. [DOI] [Google Scholar]
- Guerci D.; Capone M.; Fabrizio M. Exciton Mott transition revisited. Physical Review Materials 2019, 3, 054605. 10.1103/PhysRevMaterials.3.054605. [DOI] [Google Scholar]
- Georges A.; Kotliar G.; Krauth W.; Rozenberg M. J. Dynamical mean-field theory of strongly correlated fermion systems and the limit of infinite dimensions. Rev. Mod. Phys. 1996, 68, 13–125. 10.1103/RevModPhys.68.13. [DOI] [Google Scholar]
- Masson S. J.; Ferrier-Barbut I.; Orozco L. A.; Browaeys A.; Asenjo-Garcia A. Many-Body Signatures of Collective Decay in Atomic Chains. Phys. Rev. Lett. 2020, 125, 263601. 10.1103/PhysRevLett.125.263601. [DOI] [PubMed] [Google Scholar]
- Masson S. J.; Asenjo-Garcia A. Universality of Dicke superradiance in arrays of quantum emitters. Nat. Commun. 2022, 13, 2285. 10.1038/s41467-022-29805-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sierra E.; Masson S. J.; Asenjo-Garcia A. Dicke Superradiance in Ordered Lattices: dimensionality Matters. Physical Review Research 2022, 4, 023207. 10.1103/PhysRevResearch.4.023207. [DOI] [Google Scholar]
- Diroll B. T.; Zhou H.; Schaller R. D. Low-temperature absorption, photoluminescence, and lifetime of CsPbX3 (X= Cl, Br, I) nanocrystals. Adv. Funct. Mater. 2018, 28, 1800945. 10.1002/adfm.201800945. [DOI] [Google Scholar]
- Kotliar G.; Vollhardt D. Strongly Correlated Materials: Insights From Dynamical Mean-Field Theory. Phys. Today 2004, 57, 53–59. 10.1063/1.1712502. [DOI] [Google Scholar]
- Keimer B.; Moore J. E. The physics of quantum materials. Nat. Phys. 2017, 13, 1045–1055. 10.1038/nphys4302. [DOI] [Google Scholar]
- Zhou B.; Dong H.; Jiang M.; Zheng W.; Sun L.; Zhao B.; Tang B.; Pan A.; Zhang L. Single-mode lasing and 3D confinement from perovskite micro-cubic cavity. Journal of Materials Chemistry C 2018, 6, 11740–11748. 10.1039/C8TC03543A. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.