Energetics of muscle contraction: further trials

Abstract

Knowledge accumulated in the field of energetics of muscle contraction has been reviewed in this article. Active muscle converts chemical energy into heat and work. Therefore, measurements of heat production and mechanical work provide the framework for understanding the process of energy conversion in contraction. In the 1970s, precise comparison between energy output and the associated chemical reactions was performed. It has been found that the two do not match in several situations, resulting in an energy balance discrepancy. More recently, efforts in resolving these discrepancies in the energy balance have been made involving chemical analysis, phosphorus nuclear magnetic resonance spectroscopy, and microcalorimetry. Through reviewing the evidence from these studies, the energy balance discrepancy developed early during isometric contraction has become well understood on a quantitative basis. In this situation energy balance is established when we take into account the binding of Ca to sarcoplasmic proteins such as troponin and parvalbumin, and also the shift of cross-bridge states. On the other hand, the energy balance discrepancy observed during rapid shortening still remains to be clarified. The problem may be related to the essential mechanism of cross-bridge action.

Introduction

The study of energy exchanges in muscle has a long history, and the present day concept of the subject has progressed only gradually. However, the subject has progressed rapidly in recent years owing to the progress in muscle research and to the introduction of modern technology. This review has been written in view of our own trials made in this fruitful period. The description is not intended to be comprehensive. An interested reader should consult with an excellent and comprehensive monograph written by Woledge, Curtin and Homsher [1].

Active muscle produces energy in the form of work and heat, which are derived from chemical reactions. To understand fully the process of energy conversion during contraction, it is absolutely necessary to identify what these reactions are and how much their enthalpy changes (heat + work). Energy balance studies, in which energy output and the associated chemical reactions are precisely compared, have been one of the most important developments in this field of study. The following are the key steps that led to the energy balance studies.

In the 1920s it was found that the initial processes proceed and give rise to the work and the initial heat without the need for oxygen during contraction. Oxygen is used largely after contraction is over, producing the aerobic recovery heat. In the following decade phosphocreatine (PCr) was discovered, and it has been recognized that PCr splitting mostly contributes to the initial processes. By the early 1960s it was confirmed that the sarcoplasmic reticulum (SR), the physiological relaxing factor, exerts its effect by actively removing Ca ions from the actomyosin system. The energy output can therefore be divided between the actomyosin system and the SR.

In 1971 it was realized that PCr splitting cannot explain the whole of the initial energy output during isometric contraction (energy balance discrepancy) [2]. Furthermore, there develops another completely different type of energy balance discrepancy during rapid shortening. Dislocations of Ca ions and associated binding to proteins, and the changes of actomyosin states are candidate contributors in order to resolve the energy balance discrepancies during the initial processes of contraction.

This article is divided into two parts. An interested reader may read part II first. Reviews on these topics have been written by various authors [3–7].

Part I. Era of heat

Development of muscle energetics

It was recognized at one time that lactic acid was formed as the result of muscular activity, and was disposed of in the presence of oxygen [e.g., 8]. A new standpoint was reached, as A. V. Hill wrote in 1932 [9, 10], when Eggleton and Eggleton described phosphagen (phosphocreatine), a labile form of organic phosphates in muscle [11]. In the early 1930s, Lundsgaard showed that muscles treated with iodoacetic acid (IAA) can contract without any lactic acid formation, but only with the breakdown of phosphagen [12]. Lundsgaard’s hypothesis was that phosphagen is the substance directly supplying the energy for contraction, while lactic acid formation provides the energy for its resynthesis. By the early 1960s it had been suggested that adenosine triphosphate (ATP) is the immediate energy source for muscle contraction on various experimental grounds. However, attempts to demonstrate changes in the concentration of ATP during a single contraction were unsuccessful. It was suggested that this difficulty was due to a rapid regeneration of ATP from PCr catalyzed by the enzyme creatine phosphotransferase (creatine kinase). Cain, Infante and Davies [13] and Cain and Davies [14] clearly showed that ATP is split in muscle treated with fluorodinitrobenzene (FDNB), an inhibitor of creatine kinase (CK). This was later confirmed by Mommaerts and Wallner in the contraction cycle of frog muscles [15].

Principles of muscle energetics

Thermodynamic Principles

A rigorous treatment of the thermodynamics that is relevant to biology has been given by Wilkie [16]. According to his considerations some of the principles of thermodynamics concerning the energetics of muscle contraction, which are relevant to the following discussions, will be described here. Most significant of his considerations is perhaps that of efficiency, which will be described below (“Mechanical and thermodynamic efficiencies”). Living processes have several properties that greatly simplify the applications of thermodynamic reasoning. All their chemical reactions take place in solution, i.e., at constant pressure and at constant volume. More importantly, chemical processes proceed at almost uniform temperature. It should be stressed here that, in the biological system, heat cannot be converted to work by any means, and work can easily be converted to heat by friction, viscosity and so on. On the other hand, the free energy (maximum capacity to perform work) in principle can be freely interconverted from one form to another. In practice, however, the interconversion is never complete. The degree to which free energy is degraded into heat therefore measures the inefficiency of energy conversion.

Consider 1 mol of reaction taking place in a large volume so that changes in concentrations are negligible. When no external work is performed, the amount of the heat of reaction, or enthalpy change (ΔH), is equal to the difference between the energy content of the products and that of the reactants. ΔH = energy content of products − energy content of reactants (per mol). ΔH can be a positive number (endothermic) or a negative number (exothermic). Thus,

$$\text{Heat}\text{evolved}=-\mathrm{\Delta }H$$ 1

when external work is performed by the system, then according to the first law of thermodynamics (conservation of energy), the heat evolved must be less by an amount equivalent to the external work performed:

$$\text{Heat}\text{evolved}=(-\mathrm{\Delta }H)-\text{external}\text{work}\text{performed}$$ 2

The change in the reactants is the same as before, so ΔH is unchanged. The actual amount of work that can be performed should have a limit. Therefore, reactants and products have a maximum work, or free energy content, then ΔG = free energy of products − free energy of reactants (per mol). Therefore,

$$\text{Maximum}\text{work}=-\mathrm{\Delta }G.$$ 3

Introducing entropy change (ΔS)1 to Eq. (2), one obtains:

$$\text{Heat}\text{produced}=T(-\mathrm{\Delta }S)+(-\mathrm{\Delta }G-\text{work}\text{performed}),$$ 4

where ΔS = entropy of products − entropy of reactants (per mol), and T is absolute temperature. We should realize that heat arises from two sources; the first is a reversible movement of heat and the second an irreversible waste of free energy.

In case the problem is to find out the amounts of the chemical processes and their time-course, one is concerned with ΔH, the heat of reaction. From Eq. (2):

$$\text{Heat}\text{produced}+\text{work}\text{performed}=n(-\mathrm{\Delta }H),$$ 5

where n is the number of moles of reaction that has taken place. If several reactions proceed simultaneously, the total enthalpy change, (heat + work), is the sum of the enthalpy changes due to the individual reactions:

$$\text{Heat}\text{produced}+\text{work}\text{performed}=\sum n_i(-\mathrm{\Delta }{H}_i),$$ 6

where n _i is the number of moles of the reaction i that have proceeded, and ΔH _i is its heat of reaction per mol. Equation (6) makes it possible to draw up a balance sheet either for the whole process, or for any part of it (“Discovery of energy balance discrepancy”).

Chemical reactions supplying energy in muscle

Fast skeletal muscles consume ATP, producing ADP and inorganic phosphate (P_i), faster than it is regenerated by glycogenolysis and oxidation of glycogen. In muscle cells the reaction (Lohmann reaction) catalyzed by CK is close to equilibrium:

$$PCr+ADP\leftrightarrow Cr+ATP$$ 7

The equilibrium is strongly towards the right in the above equilibria; therefore, the concentration of ATP is essentially unchanged, and only a fall in PCr concentration is observed. The adenylate kinase or myokinase reaction (2ADP ↔ AMP + ATP) is also close to equilibrium. The concentrations of ATP, ADP, P_i, PCr, creatine (Cr) and AMP can be calculated by using the equilibrium constants [12, 17–19]. During contraction under physiological conditions, the concentration of ATP scarcely changes, and a fall in PCr concentration and a rise in P_i and Cr concentrations are observed. These have been confirmed in frog muscles by chemical analysis [20], by biopsy studies in human muscles [21] and by phosphorus nuclear magnetic resonance (³¹P NMR) spectroscopic studies in human and frog muscles [22]. In fatigued muscles, when the PCr concentration has fallen to a low level, the ATP concentration falls and that of ADP actually rises. When such a stage is reached, the level of AMP rises and is broken down by AMP deaminase to inosine monophosphate (IMP) and NH₃. In the 1960s it was confirmed that a FDNB–treated frog muscles, due to the inhibition of creatine kinase (CK), do not split PCr, but do use ATP during contraction [14, 15]. The amount of ATP present in muscle cells was found to be just enough for less than ten brief contractions. More recently, mice completely deficient in CK (CK^−/−) have been bred [23], which would be useful in assessing the importance of CK-catalyzed reactions [24].

Glycogenolysis. ADP and P_i are resynthesized to ATP at several points in the metabolic chain. During glycogenolysis, glycogen that is abundantly present in muscle cells is converted to ATP and lactate (Embden-Meyerhof pathway). Glycogenolysis can be blocked by IAA.

Oxidation of glycogen. This reaction produces 12 times more ATP (36 ATP per glycogen unit) than does glycogenolysis. Glycogen is finally converted to ATP, CO₂ and H₂O. The enzymes for this mechanism, the citric acid or tricarboxylic acid (TCA) cycle and the cytochrome chain, are confined to the mitochondria. In frog skeletal muscle, oxidative recovery is slow and full recovery takes about 1 h at low temperature [21, 25, 26] and in fish white fibers it is similarly slow even at 12 °C which is the animal’s normal physiological temperature [27]. In mammalian muscles, however, oxidative recovery is much faster [28].

Thermopiles

Studies on heat production by muscle have been performed mainly with thermopiles. The principal design and the actual use of the thermopiles have been given by Hill [10] and by Woledge et al. [1]. A thermocouple consisting of different materials produces electromotive force (emf) when placed where a temperature gradient exists. As an example, a thermocouple consisting of constantan and chromel produces 57.7 μV/ °C temperature difference between hot and cold junctions at 0 °C [10]. The thermopile for muscle consists of many couples connected in series. Junctions between the two kinds of metals are so arranged that the hot junctions are placed close to the muscle and cold junctions at the metal frame at a constant temperature. The change in temperature observed during muscle activity depends on both the heat capacity of the muscle and that of the thermopile. Therefore, in order to avoid a loss in emf, the heat capacity of the thermopile, including the thermocouples themselves and the film and other materials used for electrical insulation, must be as small as possible.

Several types of thermopiles have been constructed. Hill-Downing thermopiles are constructed from thermocouples made by welding or brazing two different kinds of thin wires and flattening them. The construction of this type of thermopile is simple but difficult. Galvanometers that were traditionally used for amplification of their emf are no longer available commercially. Electroplated thermopiles are made, for instance, by electroplating silver onto sections of a thin constantan wire. The method of constructing this type of thermopile was established [30], and they have been constructed in many laboratories [31–35]. Amplification of emf can be made using FET-input chopper amplifiers, owing to the thermopile’s high electrical resistance. Metal-frame thermopiles have been constructed by vacuum-depositing materials onto the film [36]. This type of thermopile has been used for frog single muscle fibers [37] and extensively in fish fiber bundles [e.g., 27] and mammalian skeletal and cardiac muscles [38, 39]. In integrating thermopiles a thin silver plate is placed in contact with the muscle and a thermocouple through the insulation [40]. An advantage of this type of thermopile is to avoid uncertainties such as uneven distribution of temperature along the muscle length.

The thermopile output (emf) must be corrected for heat loss and, if high time resolution is required, for thermopile lag [1, 10]. Output of a thermopile would be smaller than the real value by an amount of heat lost via the materials constructing the thermopile (time constant may be as long as 30 s). Thermopile lag is due to the time required for the muscle to heat the hot junctions of the thermopile. A calibration of thermopile output can be made by the Peltier method developed by Kretzschmar and Wilkie [41, 42]. This method is to utilize Peltier heating produced in hot junctions of the thermopile by passing current through it. By recording the thermopile output when the added heat capacity at the hot junctions is known, it is possible to precisely determine both sensitivity (Seebeck coefficient, microvolt output/degree temperature difference/thermocouple) and heat capacity of the thermopile.

Muscle heat measurements

Resting heat production

Resting heat production forms a baseline for active heat production. The resting heat rate of frog muscle at 20 ^°C is close to 2.4 mcal (10.0 mJ) g⁻¹ min⁻¹ [43],2 and is in agreement with measurements of oxygen consumption which fall close to 0.5 mm³ g⁻¹ min⁻¹ at 20 ^°C [44]. In different tissues from muscle, an oxygen consumption of 0.47 mm³ g⁻¹ min⁻¹ at 20 °C has been reported for frog sciatic nerve [10].

It has been shown that the rate of heat production of the resting frog sartorius muscle rises when the muscle is placed in a hypertonic solution (Yamada effect3) [45]. A similar increase in heat rate has been known to occur when the K⁺ concentration was raised (Solandt effect) [43, 46] and when the muscle was stretched (Feng effect) [47–49]. Sub-contracture concentration of caffeine is also known to stimulate the metabolism of the muscle [51, 52]. The hypertonicity response (Yamada effect) is similar to the Solandt effect in several respects, and both of them differ from the Feng effect (Table 1). Yamada suggested that deformations produced by hypertonicity in the SR system might trigger Ca release and the increase in oxidative metabolism [45]. More recently, Chaura et al. have shown that calcium-induced calcium release (CICR) is enhanced by exposure to hypertonic solutions [53]. They also showed, from close examination of structural changes, that ryanodine receptor (RyR)-Ca release channels can be liberated from their control by dihydropyridine receptor (DHPR)-voltage sensors in hypertonic solutions, thereby enhancing SR Ca release.

Table 1.

Comparison between Yamada, Solandt and Feng effect

	Yamada effect	Solandt effect	Feng effect
Stimulus	Hypertonicity of the medium	Depolarization of membraneb,c	Stretchinga,f
Threshold	Less than 2× normal osmolality	About −65 mVb,c	To 1.2 × normal lengthf
Maximal heat rate	30 – 50 mcal.g−1. min−1 (125 − 209  mJ. g−1. min−1)	40 mcal.g−1. min−1 c (167 mJ.g−1. min−1)	10 − 14 mcal.g−1. min−1 f (42 − 58 mJ.g−1. min−1)
Effect of anaerobic condition	Reduced to 1/10	Reduced to 1/10c	Reduced to 1/2a
Effect of procaineg (<10 mM)	Substantially reduced	Suppressed completelye	Potentiatedf
Effect of ouabainh (10−1 M)	Not affected	Not affected	–
In K2SO4 (isotonic)	Remains substantial	–	Remains normald,f

Yamada [41]. Headings are added on top of each column

^aFeng [47]; Euler [48]

^bSolandt [46]

^cHill and Howarth [43]

^dHowarth JV, unpublished

^eNovotný et al. [50]

^fClinch [49]

^gBlocks voltage-gated Na channels

^hInhibits the Na/K ATPase (pump) and consequently increases intracellular Ca

It is not clear why Ca triggers metabolism without producing force. Stewart et al. have reported a specific state of myosin with a very low ATP turnover rate in skeletal muscle [54]. Cooke has proposed a possibility that phosphorylation of the myosin regulatory light chain by Ca may transform myosin heads from the specific low-turnover state into the normal relaxed state, increasing thermogenesis markedly [55]. It is of interest that skeletal muscle thermogenesis may play prominent roles in whole body metabolic rate and, therefore, provide new approaches to the treatment of obesity or high blood sugar levels. More recently, sarcolipin (Slp), a newly identified regulator of the SR Ca-ATPase (Serca) pump, has been shown to be necessary for muscle-mediated thermogenesis and control of whole-body energy metabolism [56]. It is suggested that Slp interacts with Serca in the presence of Ca, leaked from RyR, and thereby promotes uncoupling of the Serca pump, leading to heat production.

Initial heat production

Heat production in isometric tetanus

Maintenance heat

In 1920, Hill and Hartree raised the serious question of what happens when a muscle is stimulated and develops elastic potential energy (i.e., force produced), and then the muscle becomes relaxed [57]. By constructing a thermopile and a galvanometer system they succeeded in resolving four phases of heat production associated with isometric tetanic contractions of frog muscles at 0 °C (Fig. 1). The four phases are: (a) an initial rapid heat production associated with the development of the mechanical response; (b) a heat production during the maintenance of contraction (heat alone is being liberated); (c) an evolution of heat during the stages of relaxation (relaxation heat); and (d) a slow production of heat in the presence of oxygen (recovery heat). These characteristic features have been observed continually with improved methods [e.g., 58, 59].

Fig. 1.

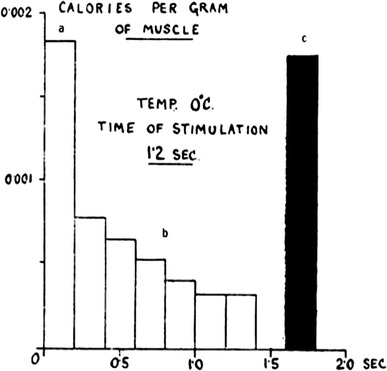
Results of analysis of galvanometer deflections obtained by stimulating frog sartorius muscles for 1.2 s (90 Hz) at 0 °C. The results show the rate of heat production in blocks of 0.2 s. These represent the first three phases, viz the heat production associated respectively with a the development, b the maintenance, and c the disappearance of the mechanical response. The recovery heat is not seen because it is very slow at low temperature. Heat production given in cal/g muscle. Hill and Hartree [57]. Although the analysis involved large corrections, the results are remarkably similar to those in later studies [59, 60]

An influential way to examine the initial heat production (i.e., phase b of heat in Hill and Hartree [57]) has been developed by Aubert, who produced a thesis after staying in Hill’s laboratory. According to Aubert [60], the heat produced during maintenance of contraction in tetanus can be described as the sum of two terms: a time-dependent heat rate, h _a, the labile maintenance heat, and a time-independent heat rate, h _b, the stable maintenance heat. Thus, the time course of the heat rate, h _t, during maintained contraction in an isometric tetanus is given by the following equation,

$$h_t=h_a(e^{-\mathit{\alpha }t})+h_b,$$ 8

where t is time (s) and α is a rate constant (s⁻¹). Integration of Eq. (8) from the beginning of the tetanus to a time t gives the total heat production H _t as Eq. (9):

$$H_t=(h_a/\mathit{\alpha })(1-e^{-\mathit{\alpha }t})+h_{b}t$$ 9

According to Hill and Hartree, during development of tension in an isometric contraction, both heat and mechanical potential energy are being produced by the muscle because the muscle performs work against external compliance; during the maintenance of tension, heat alone is being liberated; next a considerable evolution of heat occurs, which is derived from the mechanical potential energy lost in relaxation [57]. The records shown in Fig. 1 include the heat of relaxation (the bar in black), and it can be conceived that the most rapid heat production seen at the very beginning may almost correspond to the amount of the potential energy thus produced. The labile maintenance heat is so termed because it is much reduced in the second tetanus separated by a short time interval from the first in the two isometric tetanic contractions. It recovers gradually as the separation between the tetani increases [60, 61].

In energy balance studies (“Energy balance studies”) the rate of heat and work production in isometric tetanic contractions is well accounted for by ATP splitting (i.e., PCr decline) except for the first several seconds [2, 31, 59]. Woledge et al. (1985) summarize, from various published sources, the dependence of isometric heat production on muscle length [1]. The results show that at lengths longer than the optimal length the stable maintenance heat rate declines linearly to reach a value of about 28 % at the no-overlap length. These results also show that the rate of stable maintenance heat production is mainly due to ATP splitting associated with actomyosin ATPase activity and that the remaining 28 % is possibly to do with Serca. On the other hand, the labile maintenance heat seems more or less independent over quite a wide range of lengths, although it is reduced at very long lengths. According to these results Woledge et al. (1985) suggest that the labile maintenance heat may be associated with the binding of Ca to parvalbumin (Pvalb) (“Unexplained heat in isometric tetanus” and “Ca binding to parvalbumins”) [1].

Shortening heat

In 1938, Hill published an influential paper on the heat of shortening [58]. The design of those experiments in frog muscle at 0 °C was to measure heat in isometric tetanic contractions, followed by shortening in a controlled manner. In this way he observed an extra heat production associated with the shortening. The shortening heat was proportional to the extent of shortening, and was the same whenever the release occurred. The shortening heat rate was proportional to the speed of isotonic shortening with various loads, while the total amount of heat remained the same according to the distance shortened. Even when more work was done, the total heat of shortening was the same. Thus, it seemed that when a muscle shortens it produces extra energy in two terms, (1) heat of shortening, the rate being av, where a is a constant and v is shortening velocity, and (2) mechanical work, the rate being Pv, where P is force during shortening. It was also found that the rate of the extra energy produced, (P + a)v, was linearly related to the load P, or more exactly (P ₀ − P), where P ₀ is isometric force. From these results the following equation emerged:

$$(P+a)v=b(P_0-P).$$ 10

Therefore,

$$(P+a)(v+b)=(P_0+a)b=\text{const,}$$ 11

where a is the shortening heat coefficient, v the velocity of shortening, P the force produced during shortening, and P ₀ the maximum isometric tension.

Equation 2 is a hyperbola relating force (load) and velocity of shortening, and can also be derived from mechanical recordings. For many years after it was presented in Hill’s 1938 paper, the so-called characteristic equation was taken by everyone to be of fundamental significance. Note that these results were obtained on the ground that the shortening heat coefficient, a, was independent of load as was noted above. However, a is actually dependent on load, as was later confirmed by Hill himself [62]. Note that other types of equations can describe the relation between force and velocity just as well [1].

Starting in the late 1970s, shortening heat was extensively studied. (1) Irving, Woledge and Yamada looked for the effect of previous shortening in experiments with repeated shortening, each in a separate tetanus [63]. With a minimum interval of 5 s, there was no effect of the previous shortening. (2) Irving and Woledge showed that, when there were two shortenings in single tetanus, the shortening heat in the second shortening was reduced, its recovery being about half complete with an interval of 0.3 s [64]. The work produced in the second contraction was also reduced. (3) Irving and Woledge also showed that the shortening heat and work were non-linearly related to the distance shortened [65]. This contradicts the earlier results of Hill [58], who showed that the shortening heat was linearly related to the extent of shortening in isotonic releases. Irving and Woledge [65] studied a series of shortenings ending at the same final sarcomere length. The shortening heat was nonlinearly related to the distance shortened and greater with smaller distance shortened. In Hill’s experiments [58] the sarcomere length was not precisely controlled and also account was not taken of the heat absorption during length change in unstimulated muscle, which must be substantial in sartorius muscles (see below) and most probably takes place in stimulated muscle also [35, 66–68]. (4) Finally, the shortening heat depends linearly on sarcomere length as isometric tension does at sarcomere lengths greater than 2.2 μm [35, 66–68]. The last results fit in well with the independent force generator idea of cross-bridges and show that shortening heat originates from the cyclic interaction of cross-bridges [69, 70]. When unstimulated muscle is allowed to shorten, heat is absorbed. This heat effect is smaller in more extensible muscles such as semitendinosus, but is still significant. The prominent thermoelastic heat absorption seen when stretched muscles are released is discussed elsewhere (“Thermoelasticity of resting muscle”).

Almost in parallel with the studies on shortening heat described above, measurements of ATP utilization during shortening (energy balance studies) were performed, yielding informative results. Therefore, the mechanism of producing the shortening heat will be discussed associated with the results of energy balance studies (“Unexplained heat developed during shortening”).

Stretching active muscle

The stretch of active muscle occurs frequently in life such as during the descent of stairs. When tetanically contracting muscle is stretched, the muscle develops large tension during the stretch, and after the end of the stretch when stimulation continues, it produces more active tension compared to that without stretch. This excess tension after the stretch increases as the sarcomere length increases from 2.5 to 3 μm, whereas the active tension under isometric conditions decreases [71, 72]. Curtin and Woledge studied the heat plus work (h + w) produced and compared this with the extent of ATP splitting by stretching tetanically contracting muscle [73]. The excess (h + w) during stretch coincided well with the work done on the muscle, while during the interval (~1 s) shortly after the stretch the rate of heat production was significantly greater than in the control condition. The amount of ATP split was not different from the control. Linari, Woledge and Curtin confirmed the above results using single fibers [37]. The excess heat observed shortly after stretching the active muscle may be caused by the delayed dissipation of some of the work that had been done on the muscle during the stretch. No evidence has been found that the energy introduced into the muscle was absorbed in a chemical process such as ATP synthesis [73–77]. It could be that the mechanical properties of cross-bridges are changed by the stress on the thick filament and remain altered for some time [72, 78].

Heat production in twitches

Activation heat

Activation heat was defined as the most rapidly produced component of the initial heat production in twitch contractions of frog skeletal muscle [79]. It may thus be related closely to the initial rapid component, the labile heat, in tetanic contractions [60]. Later, the activation heat was redefined owing to the results by Smith [80] and Homsher et al. [81] as a component that remains after muscles are stretched to a non-overlap length. Both of these authors agree that the activation heat thus observed is 26–30 % of isometric heat at rest length [0.6–1.0 mcal (2.5 − 4.2 mJ) per gram muscle]. Homsher et al. showed that the time course of the activation heat can be resolved into two phases of fast and slow [81]. The time constant of the fast phase is 35 ms and is temperature insensitive, while that of the slow phase is temperature sensitive (Q ₁₀, 2.8). It can, therefore, be deduced that the two phases of the activation heat reflect different processes. The fast phase of the activation heat most probably reflects the release from the SR and/or the subsequent binding of Ca to the Ca-specific sites of troponin (Tn). On the other hand, the slow phase of the activation heat probably reflects the transport of Ca to the SR with the associated utilization of ATP.

Yamada, Mashima and Ebashi measured the heat of binding of Ca to Tn calorimetrically, and showed that 0.93 − 1.77 mcal (3.9 − 7.4 mJ) per gram muscle would be produced associated with a complete activation of contraction of frog muscles (“Ca binding to troponin”) [82]. This fits in well with the activation heat determined as above [0.6–1.0 mcal (2.5–4.2 mJ) per gram muscle]. The release of Ca from the SR and calsequestrin may be taken to be thermally neutral [83].

The Fenn effect

In 1923, Fenn published a series of papers on experiments performed in collaboration with A. V. Hill [84, 85]. As he noted, his findings (now known as the Fenn effect) were related to a historical debate on whether a fixed amount of potential energy is set up when a muscle is stimulated, or whether, when the muscle does work, an extra energy is liberated as required. Figure 2 shows one of Fenn’s original figures [84]. Carlson, Hardy and Wilkie [86] reported results of similar studies to Fenn’s. In view of the error in calibrating procedures then available to Fenn [87], both results are in good agreement [86]. These results show that the energy output is greatest for a moderately heavy load. It can be seen that the energy liberated is not a fixed amount as the then prevailing viscoelastic model predicted, but varies according to the load that the muscle encounters, and thus the work done. The Fenn effect has been reexamined under various mechanical and other conditions [see 1, 88]. From the energetics point of view, muscles perform more like automobile engines controlled by accelerators, the control of which may be made completely automatic in the near future. The molecular mechanism of this control in muscle is not known. Linari et al. have reported a novel finding that, although control of muscle contraction is mediated by Ca-dependent structural change in the thin filament, thick-filament stress caused by heavy load triggers changes in the thick filament structure and unlocks more myosin motors [78]. This concept of the thick filament as a regulatory mechanosensor may provide an explanation for the above-mentioned characteristics of contraction.

Fig. 2.

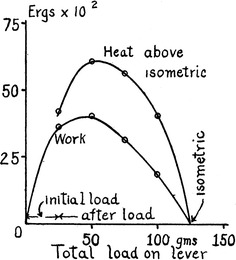
Variations of work and heat (ordinate) in units of 100 ergs (10⁻⁵ J) in isotonic contractions of sartorius muscle of the frog against variations in load (abscissa). The heat represents only the heat in excess of the isometric. Fenn [84]

Recovery heat production

We all know from our everyday experience that muscles have to recover after exercise. In frog muscle it can take almost 1 h for PCr to recover to its original level before the stimulation, in oxygen at 0 °C [26, 89]. During this period of recovery from contraction the rate of heat production is known to be increased (recovery heat) [10].

Anaerobic recovery heat

A.V. Hill described a historical note on the development of the idea concerning the non-oxidative character of the initial process of muscle contraction [10]. Later, D. K. Hill showed that the oxygen consumption occurs entirely after activity [25]. Earlier, Hill and Hartree had already noticed signs of anaerobic recovery heat production in muscles devoid of oxygen [57]. These results are all related to the development of the present view of the energy supply in muscle contraction as described earlier; the discovery of the roles played by PCr and ATP.

There is an intensive series of studies on the topic as described by Hill [10]. The anaerobic recovery heat is probably related to the synthesis of ATP by anaerobic glycolysis leading to lactic acid formation. The anaerobic recovery heat is much smaller (10–20 %) than the oxidative recovery heat and, therefore, difficult to measure accurately. The presence of the early negative phase, and also the phase seen even in the IAA-treated muscles, might arise from these difficulties, as was discussed by Woledge et al. [1]. Yamada, Kikuchi and Sugi studied the regulation of glycogenolysis in frog skeletal muscle, in which oxidative recovery was inhibited by NaCN, using ³¹P NMR at 10 °C [90]. The results have shown that the onset of glycogenolysis is regulated by the P_i concentration.

Oxidative recovery heat

The oxidative recovery heat is much greater than the anaerobic counterpart as has been described above. The time course of oxygen consumption is the same as that of oxidative recovery heat [25]. When muscles on a thermopile were heated for a brief period of time by passing an alternating electric current of high frequency, the emf of the thermopile is suddenly increased and then falls exponentially as heat is lost by conduction. On the other hand, the fall is slower when muscles have been stimulated to contract than when they have been heated electrically. The total areas under these curves, from the start to the end (i.e., the base line), give the total heat produced in the muscles. When both curves (stimulated and heated) are made to match to the same maximal value at the start, the ratio of areas of these curves, i.e., the ratio (recovery heat)/(initial heat), is known as the recovery ratio, a convenient measure of the amount of oxidative recovery heat. The recovery ratio varies between 1.2 and 1.5 according to the experimental conditions [1].

Efficiency and fatigue

Mechanical and thermodynamic efficiencies

The efficiency with which a process such as muscle contraction is carried out is important as well as of interest in life sciences. A.V. Hill defined the ratio of the work done to the total energy used, i.e., w/(h + w), as the mechanical efficiency associated with the muscle contraction [91]. This was a term derived from engineering, not from physical chemistry [10]. The value of mechanical efficiency has been reported to be 0.33–0.45 for the initial processes in maximal conditions [91]. However, the idea of mechanical efficiency has been derived from an analogy with the heat engine. Biological systems operate at constant as well as uniform temperature so that heat cannot be converted to work. Therefore, the above analogy cannot be appropriate. Wilkie has made a rigorous treatment of the subject and has shown that, in the case of muscle contraction (“Thermodynamic principles”) [16]:

$$\text{Efficiency}=(\text{external}\text{work}\text{performed})/(\text{free}\text{energy}\text{used}),$$ 12

since for any chemical process:

$$\text{Free}\text{energy}\text{used}=-\mathrm{\Delta }G=(\mathrm{\Delta }G/\mathrm{\Delta }H)(-\mathrm{\Delta }H).$$ 13

Therefore, by assuming a single process to occur:

$$\text{Efficiency}=(\mathrm{\Delta }H/\mathrm{\Delta }G)w/(h+w).$$ 14

Note that neither ΔH nor ΔG need be known, only their ratio. Wilkie noted that, in the discussions of efficiency in muscle contraction to the date of his writing in 1960, the factor ΔH/ΔG is overlooked or implicitly assumed to be unity [16]. It is not possible to estimate the genuine efficiency from measurements of heat and work. According to Wilkie, considering the total energy conversion in muscle as the oxidation of glycogen, in which ΔH/ΔG is known to be about unity, the genuine or thermodynamic efficiency for the complete cycle of contraction and recovery may be estimated to be 0.2 by taking w/(h + w) = 0.2 in this situation [16, 91]. It is more difficult to estimate the thermodynamic efficiency for the initial processes associated with the contraction per se. Wilkie has shown that it must be greater than 0.2 at least [16]. Kushmerick and Davies have shown that the thermodynamic efficiency of muscle is very high and can be nearly 100 % in muscle shortening at a constant velocity [92]. Woledge et al. discuss that the thermodynamic efficiency calculated from the extent of ATP splitting, assuming ATP splitting to be the only process providing the free energy, can be very high during the initial process of contraction [1]. See also Barclay, Woledge and Curtin [93].

Fatigue

Muscles, especially fast muscles, show a progressive decline in performance, which is noted as fatigue. Subsequently, performance recovers during a period of rest. It is noteworthy that fatigue in skeletal muscles is still an unsolved problem. Many factors may affect the progress of fatigue to a certain extent [94]. However, for the present interest, only changes in the level of metabolites will be treated here. The amount of metabolites that support contractile activities in muscle is limited, as has been described above (“Chemical reactions supplying energy in muscle”). In the previous Section, the mechanism of recovery from contraction has been treated. Dawson et al. studied fatigue in anaerobic frog muscles, poisoned with KCN, using phosphorus nuclear magnetic resonance (NMR) spectroscopy at 4 °C [89]. They attempted to find the relationship between the force development on stimulation and the metabolite levels. The fact that various patterns of stimulation revealed the same relationship between the force development and levels of metabolites indicated that changes in activation processes, if any, are not responsible for the force decline by itself, although activation may somehow be linked to the changes in metabolites. Studies by Dawson et al. have shown that force development is not related in a simple way to levels of substrates for contraction, i.e., PCr and ATP [89]. The force development also depended on the levels of ADP, H⁺ and P_i, suggesting the product inhibition mechanism and also an effect on the activation of contraction. Free energy change for the hydrolysis of ATP declines only slightly during fatigue. However, the free energy changes of ATP hydrolysis may be related to the slowing of mechanical relaxation during fatigue [95].

Nakamura and Yamada studied the effect of intracellular pH, which can be controlled by CO₂ concentration equilibrated to the circulating fluid, on the tetanic force produced by frog muscles at 4 °C by using ³¹P NMR [96]. CO₂ was increased in the range from 5 to 40 % and, correspondingly, pH_i was changed in the range from 7.20 to 6.57. The levels of P_i were also measured. Initially, the increase in CO₂ affected pH_i, but not the level of P_i; therefore, the effect of pH_i per se can be studied. Subsequently, by applying a series of tetanic stimulations force declined until a steady state was reached in elevated CO₂. Thus, the effect of pH_i per se, as well as the effect of P_i at that particular pH_i, was able to be evaluated separately. The results have shown that the effect of P_i is more marked in reducing the force production than that of pH_i per se. Force was suppressed linearly with an increase in P_i up to 30 mmol (l fiber water)⁻¹, while an interaction between the effect of P_i and pH_i was indicated. Involvement of the acidic form of P_i, i.e., H₂PO₄ ⁻, was indicated as already reported by others [97–99].

In conclusion, fatigue is a complex process involving both the contractile mechanism (cross-bridges) and activation of contraction [94]. However, fatigue is mostly caused by an increase in myoplasmic P_i level, suppressing the rate of cross-bridge turnover by way of product inhibition. Elevated P_i may also affect the activation processes.

Thermoelastic properties of muscle

Thermoelasticity of contracting muscle

When an active muscle is quickly released, the tension falls rapidly, accompanied by rapid liberation of heat (thermoelastic heat or normal elasticity) [100]. The amount of heat liberated (ΔQ) is in proportion to both the muscle length (l ₀, the standard or rest length) and the change in tension (ΔP), i.e., ΔQ = R × l _o × ΔP. The thermoelastic heat:tension ratio, R, has negative values in active muscles, in contrast to the positive values in resting muscles (thermokinetic or rubber-like elasticity). The values of R reported for active muscles range from −0.0038 to −0.018 [100–102].

Woledge studied the effect of a small quick release ranging from 0.3 to 0.9 mm (minimum length change ~1 %), allowing the muscle to redevelop tension spontaneously [101]. Since this procedure necessarily involves shortening, an allowance was made for the heat of shortening. Because a fall of tension is recovered as the tension is redeveloped, no mechanical potential energy is lost by the muscle to appear as heat. In this way, he has shown that a rise of tension in active muscle is accompanied by an absorption of heat similar to the production of heat known to accompany the fall of tension. Gilbert and Matsumoto reexamined the thermoelastic effect in active muscles by applying very small stretches or releases (~0.5 %) during the plateau of the isometric tetanus [102]. Production of the thermoelastic heat on small releases is apparently separated from the shortening heat produced during the tension recovery. Small stretches are accompanied by a transient absorption of heat, followed by a production of heat as the energy of the stretch is dissipated. The slope of the line for the pooled results corresponds to R = −0.0084.

Kometani and Yamada studied the thermoelasticity in rigor muscles using chemically skinned frog muscles [103]. All values of the thermoelastic heat:tension ratio, under various conditions of the presence or absence of Ca and lowering pH, were within the range reported for active muscles. These results have shown that the thermoelasticity in active muscles should originate from the thermoelasticity of myofilaments or cross-bridges and not from a detachment or stepping of cross-bridges as suggested by Huxley and Simmons [104]. However, in IAA treated frog muscles in rigor, the values for R reported are much smaller than in active muscles [60]. The reason why the difference in the thermoelasticity between the rigor muscles prepared from the living and the skinned preparations arises is unknown.

Thermoelasticity of resting muscle

Unstimulated muscles are highly extensible and the elasticity is known to be rubber-like, i.e., entropic, in nature [100]. Feng studied thermoelastic properties of unstimulated sartorius muscle of the frog [105]. He found that the thermoelastic property depends on the initial length of muscle. At moderate lengths the thermoelasticity is rubber-like, while at long lengths it becomes normal.

A third filament system, formed by the giant protein connectin/titin, has been discovered, in addition to thick and thin filaments [106, 107]. Connectin/titin is an approximately 3-MDa filamentous protein of striated muscle sarcomere. Single connectin/titin molecules extend from Z discs to M lines and are longer than 1 μm. The connectin/titin filament contributes to muscle assembly, positioning the thick filament in the sarcomere, and is also involved in forming the ultrastructure of the A band. In the I band connectin/titin filament is extensible and therefore is likely to account for the elasticity of myofibrils [108]. It has been suggested that, from measurements of the force required to stretch a single molecule of connectin/titin, a fraction of the molecule behaves as an entropic (rubber-like) spring in the sarcomere of muscle [109]. Wang et al. reported the resting tension-sarcomere length relation of skinned rabbit muscle fibers [110]. According to their study, tension produced by a stretch increases exponentially until a sarcomere length of 3.8-3.9 μm is reached.

Kometani and Yamada [35], Homsher, Irving and Lebacq [67], and Yamada and Kometani [68] studied the dependence of shortening heat on sarcomere length in semitendinosus muscle, which is more extensible than sartorius muscle. They found that unstimulated muscles showed prominent thermoelastic heat absorption (rubber-like elasticity) when released by 0.2–0.3 μm at sarcomere lengths longer than 2.5 μm. The amount of heat absorbed was greatest at the sarcomere length of around 3 μm and became less at longer lengths. This may be related to the observation by Feng described above [105]. The fact that the thermoelastic heat absorption at long sarcomere lengths was substantially reduced in fiber bundles indicated that the elasticity responsible for the thermoelastic effect is mainly present outside muscle cells in whole muscle [68].

Part II. Era of energy balance

Energy balance studies

Discovery of energy balance discrepancy

The energy liberated in muscle contraction must come from chemical processes. The output of physical energy, heat plus work (h + w), should be accounted for by the concurrent chemical changes. Following the first law of thermodynamics

$$h+w=n_1(-\mathrm{\Delta }{H}_1)+n_2(-\mathrm{\Delta }{H}_2)\text{etc,}$$ 15

where the n are the numbers of moles of the various chemical processes, and the ΔH are their molar enthalpy changes. Gilbert et al. were the first to test deliberately if such a balance between the output of physical energy and the description of the chemical events can be shown to hold. In testing the balance between the two, it is essential to measure heat [2]. It is also essential that both types of measurements, i.e., physical and chemical, be carried out simultaneously in one laboratory. Gilbert et al. [2] also employed the so-called hammer apparatus (Kretzschmar and Wilkie [111]), which was designed to cool the muscle (and thus stop chemical reactions) more rapidly than rapid immersion into liquid nitrogen or freon. The key feature about the hammers that produced rapid freezing was that the muscle was flattened to be very thin during freezing.

As a prerequisite for energy balance comparisons, Wilkie (1968) compared the heat plus work with the amount of PCr split, using the integrating thermopile described above (“Thermopiles”) [40]. In a variety of different types of contraction of IAA-treated frog muscles in N₂, the relation between heat plus work and PCr splitting was always the same; the so-called in vivo ΔH was −11 kcal/mol (−46 kJ/mol). In retrospect, this value might have included heat of all the reactions other than ATP utilization directly involved in force development, except for the recovery metabolism. Note that in all the subsequent energy balance studies the ΔH value of −8.1 kcal/mol (−34 kJ/mol) for PCr splitting, including buffer reactions, determined directly by calorimetry have been used (ΔH for ATP splitting, −48 kJ/mol; ΔH for creatine kinase reaction, +14 kJ/mol) [4, 112].

The results of Gilbert et al. have been summarized in Fig. 3 [2]. In this figure, in order to compare physical and chemical events, the heat plus work (h + w) measured in mcal/mmol total Cr, is plotted in terms of chemical units using a conversion factor of 11 kcal per mol of PCr split. They found that, during the first few seconds of isometric contraction, there is heat production, amounting to 10 mcal/g (41.8 mJ/g) without corresponding PCr break-down.4 Thereafter, the hydrolysis of PCr corresponds very well with the steady rate of heat production. The gap slowly diminishes later as PCr is split without corresponding heat production. The heat production in short tetanic contractions cannot be accounted for by splitting of PCr or ATP. This means simply that some other reactions than PCr splitting occur during the early period in tetanus. In a tetanus of 2-s duration, most of the (h + w) comes from other processes. The unexplained heat plus work most probably amounts to 10 mcal/g (41.8 mJ/g).

Fig. 3.

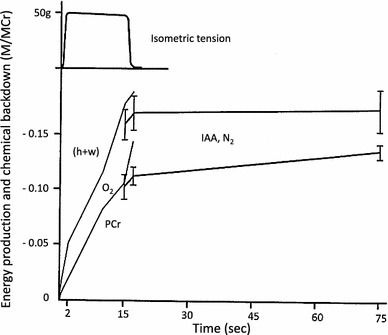
Heat and work produced, and break-down of PCr during and following a 15-s tetanus at 0 °C. The uppermost graph represents the tension during a typical 15-s isometric tetanus. The physical (h + w) and chemical (PCr) changes are shown in a dimensionless ratio, mol/mol creatine (M/MCr, Cr = total creatine in muscle). The heat plus work (h + w) is plotted in equivalent chemical units (M/MCr) using a conversion factor of 11 kcal/mol (46 kJ/mol) (see text). Muscles (light lines) used for studying changes during contractions were placed in O₂, while muscles (heavy lines with ± 1 S.E. bars) used for studying changes during the recovery period had been treated with IAA and were placed in N₂ in order to avoid complications arising from the recovery process. Gilbert et al. [2]. Printing errors are corrected and labels added

Gilbert et al. discuss some processes which could be responsible for the unexplained (h + w) [2]. Movement of calcium and subsequent binding to proteins are expected during the activation of muscle. The unexplained heat is equivalent to 25 kcal/mol (105 kJ/mol) of moved calcium, assuming transfer of 0.4 μmol/g calcium from one site within the sarcoplasm to another (Winegrad [113]), or to 140 kcal/mol (586 kJ/mol) of calcium bound to troponin, assuming 0.07 μmol/g of calcium to activate troponin (Ebashi, Endo & Ohtsuki [114]). As will be discussed later, these values are too large to be accounted for by calcium binding to troponin (“Ca binding to troponin”) [82]. Gilbert et al. also noted a likely connection between the labile heat, Winegrad’s calcium movements and the unexplained enthalpy [2]. They also suggested a possibility that the unexplained enthalpy reflects conformational changes of the contractile proteins themselves associated with the contractile activation.

Gilbert et al. discussed their finding of a delayed PCr splitting, which is significant and amounts to 0.35 μmol/g [2]. They also noted that, for the delayed PCr splitting, both favorable and contradictory results had been reported previously. Using a time resolved ³¹P NMR, Yamada and Tanokura [115] and Kawano et al. [26] studied contraction and recovery of frog skeletal muscles at 5 °C. The amount of PCr split coincided well with the appearance of P_i except for the initial few minutes following relaxation. However, during the early recovery period ΔPCr was smaller than ΔP_i. The difference was 0.35 mol/kg, which is the same as that reported by Gilbert et al. [2]. The delayed PCr splitting will be dealt with below in relation to the possible sources that explain the unexplained enthalpy (“Possible causes of energy balance discrepancy”). Phillips et al. studied the recovery time course of phosphate metabolites using ³¹P NMR and compared this with recovery heat in rat soleus muscle at 20 °C [116]. Quite unexpectedly, they found unexplained heat during the entire course of contraction and recovery.

Chemical energy balance. On the grounds that the recovery oxygen consumption (ΔO₂) should provide a valid measure of the net initial energy utilization for contraction (Kushmerick and Paul [117]), Kushmerick and Paul [118] compared ΔO₂ with the initial chemical changes (Δ ~ P) in frog muscles at 0 °C. A constant factor (Δ ~ P/ΔO₂) of 4.3 was obtained for the range of tetanic durations, while the expected value for the factor should be 6.5 for the oxidation of glucosyl units from glycogen. They discuss that there might be some unknown sources of chemical energy present during contraction. DeFuria and Kushmerick obtained similar results on ~P/lactate ratio in anaerobic frog muscle [119]. Paul presented evidence that favors this interpretation [120]. It is difficult, however, to quantify the extent of the discrepancy due to uncertainties in some steps.

Possible causes of energy balance discrepancy

We may think of two possibilities from the evidences so far discussed. First of these possibilities is the binding of Ca ions to proteins such as troponin and the associated conformational changes of the contractile proteins themselves [2]. The other possibility is the changes of the cross-bridge states, i.e., the idea of an incomplete cross-bridge cycle [121]. These possibilities will be discussed below.

Unexplained heat in isometric tetanus

The unexplained heat in isometric contraction has been reported by many authors as will be described below. Gilbert et al. have shown that the discrepancy amounts to about 0.04 μmol/μmol total creatine (M/MCr), which can be converted to more conventional unit, i.e., 10 mcal/g (41.8 mJ/g) [2]. Woledge et al. have shown a numerical example of an energy balance comparison, the unexplained energy being 169 mJ/g dry weight of muscle [1] (33.8 mJ/g muscle; muscle dry weight is about 0.2 of the wet weight).

Because the unexplained heat in isometric tetani develops early during contractions, and recovers gradually after the muscles relax from the contraction, it is conceivable that the unexplained energy arises from the processes related to the Ca movements associated with the contractile activation. Because the labile maintenance heat in tetanic contractions, like the activation heat in twitches, seems to be associated with the Ca movements on activation, the phenomenological relations between the two have been investigated extensively. The following results have been reported. (1) Curtin and Woledge compared the energy balance in two successive isometric tetani [61]. The unexplained heat was reduced to 39 % of that in the first tetanus separated by 3 s. The labile heat was also reduced to 35 % of that in the first tetanus, while the stable heat was 83 % of that in the first tetanus. Because the labile heat has been ascribed to the activation process involving Ca, they discuss the possibility that both the labile heat and the unexplained heat may arise from the events closely associated with activation. (2) The amount of unexplained energy is similar to that of the labile maintenance heat production [31, 59]. (3) Curtin and Woledge [59] and Homsher et al. [31] studied the time course of the unexplained heat and showed that its rate of evolution, which was most rapid during the first second of contraction, fell progressively and finished at around 10 s. Although the magnitude of the unexplained heat was similar to the labile heat, the time course of evolution of the unexplained heat was slower than that of the labile heat. (4) Curtin and Woledge have also shown that the unexplained heat is dependent on muscle length, but that a significant amount of the unexplained heat remains at l _max, where the actin-myosin interaction should be negligible [33]. The results have also indicated that at both l ₀ and at l _max the unexplained heat is almost equal to the labile heat. In conclusion, definitely there are similarities between the unexplained energy and the labile maintenance heat, but there are differences that indicate that the two arise, at least partly, from different processes.

A hypothesis has been put forward that the unexplained energy and the labile heat produced during an isometric tetanus comes mostly from the binding of Ca ions to Pvalb [1]. Frog skeletal muscles are known to contain sarcoplasmic water-soluble Ca-binding proteins, Pvalb, in large quantities. During tetanic contractions, unlike in twitches, a large quantity of Ca is released so that Ca-binding sites of Tn and Pvalb may become fully saturated [122–124]. Enthalpy changes associated with the Ca binding to Pvalb of frog muscles have been reported [125, 126]. In both studies it has been shown that the binding of Ca to Pvalb, in exchange for Mg, is exothermic, producing 27 − 35 kJ/mol site Ca bound, which is independent of temperature (“Ca binding to parvalbumins”) [127]. As the concentration of the binding site is 0.8 μmol per gram muscle (two sites in a molecule), the heat produced in muscle would be about 25 mJ/g muscle [1, 125, 128]. This value is not enough to account for all of the unexplained energy (30 − 40 mJ/g; 7.2 − 9.6 mcal/g) [1]. As has been discussed above there is a possibility that other processes are involved.

Kitano studied the effect of lowering pH_i on heat production in tetanic contraction by increasing CO₂ in frog skeletal muscle at 4 °C [129]. The results showed that as pH_i was decreased the labile heat was not altered, nor was the time course of its repriming from the previous contractile activation separated by a varying period of time. This partly agrees with the observation that little pH change exists for Mg–Ca exchange of Pvalb [125]. However, Ogawa and Tanokura have estimated that as many as half or more of the binding sites of Pvalb are occupied by Ca even in resting muscle [130]. Lännergren, Elzinga and Stienen have also shown that although binding of Ca to Pvalb is responsible for part of the labile heat production, about 70 % of labile heat cannot be accounted for by this process in Xenopus muscle [131].

Gilbert et al. who first reported the energy balance discrepancy during isometric tetani, have shown that during contraction there is a certain amount of heat production without PCr break-down, while subsequently there is PCr break-down without heat production (see “Discovery of energy balance discrepancy”) [2]. A shift in cross-bridge states during isometric contraction has been introduced to explain the energy balance discrepancy during rapid shortening [65, 121, 132]. A similar mechanism would be able to explain the cause of the unexplained heat in isometric tetanic contractions (see below). Kawano et al. have reported the results of a time-resolved ³¹P NMR study on isometric tetani of frog muscle at 4 °C (“Phosphorus NMR studies”) [26]. The results showed a post-contractile splitting of PCr, the amount of which coincided with the measured amount of myosin S1 in the sarcomere, 0.28 μmol/g [114] and also the results of Gilbert et al. [2] described above (“Discovery of energy balance discrepancy”). The results can be explained by P_i being released and ADP remaining bound for a time after relaxation from contraction. This is particularly because the release of ADP from myosin is markedly temperature dependent and is very slow at a low temperature such as at 5 °C [133].

Kodama and Woledge showed that the dissociation of ADP from myosin is endothermic, ΔH being +60 ~ + 90 kJ mol⁻¹ (“Myosin and actomyosin ATPase”) [134]. Further, ADP released will be rapidly converted to ATP by the CK reaction, the operation of which is also endothermic, ΔH being +14 kJ mol⁻¹ (“Discovery of energy balance discrepancy”) [112]. According to Kodama [135] the process from ADP dissociation to ATP splitting (steps 4 +1 +2 in the scheme (16) in “Myosin and actomyosin ATPase”) in the myosin ATPase catalytic cycle is endothermic. The enthalpy change can be obtained from the reaction heats for intermediate steps of myosin ATPase reaction listed in Kodama [135] to be +70 kJ mol⁻¹ at 4 °C, including that of the CK reaction described above. Allowing for 0.28 μmol/g of myosin S1 in the sarcomere [114], the heat absorbed should be 20 mJ/g (4.7 mcal/g). The value thus expected corresponds to at least part of the value of energy balance discrepancy in isometric contraction, 10 mcal/g (41.8 mJ/g) [2]. The excess heat produced early during tetanus caused by the incomplete actomyosin cycle will thus be absorbed slowly due to the release of ADP after the contraction is over. Similarly, the heat of Ca binding to Pvalb will be absorbed as Ca is taken up by SR, the process of which is likely to be thermally neutral [83]. Curtin and Woledge have shown that about half of the unexplained energy observed at full-overlap length remains at l _max, where nearly all actin-myosin interaction is prevented (see above) [33]. Thus, half of the energy balance discrepancy in isometric tetanus must be related to the cross-bridges. The remaining half observed at no-overlap length may thus be caused by the binding of Ca to Pvalb and other Ca-binding proteins.

In summary, Gilbert et al. have shown that, allowing for 11 kcal (46 kJ) physical energy per mol of PCr split, during the first few seconds of isometric contraction there is heat production, amounting to 10 mcal/g (41.8 mJ/g), without corresponding break-down of PCr [2]. The energy balance discrepancy in isometric contraction has since been confirmed and its characteristics have been examined. It can be concluded that both heat of binding of Ca to Pvalb and the development of incomplete actomyosin cycle contribute almost equally to the energy balance discrepancy in isometric contraction.

Unexplained heat developed during shortening

Since Hill has shown that when a muscle is allowed to shorten the rate of energy liberation increases (“Shortening heat”) [58], it has been assumed that the rate of energy liberation is proportional to the rate of cross-bridge turnover [136]. However, direct measurements did not support this simple interpretation. Early experiments showed that there is extra PCr splitting associated with the performance of mechanical work by a shortening muscle, but there is no component associated with shortening per se [13, 86, 137]. Kushmerick, Larson and Davies [138] and Rall et al. [139] have shown that, by directly comparing between rapidly shortening and isometric contractions, during shortening there is more heat produced than can be accounted for by PCr splitting.

Homsher, Irving and Wallner reported an important study on energy balance during rapid shortening of frog muscle at 0 °C [132]. The shortening was induced at near-maximum velocity starting after 2 s of isometric tetanus, where the unexplained heat observed in isometric tetanus becomes negligibly small. The results are summarized in Fig. 4. As is seen in the figure, less than half of the heat plus work during the rapid shortening period could be accounted for by simultaneous PCr splitting. The unexplained heat was 6.5 mJ/g. On the other hand, during the post-shortening isometric period immediately following such rapid shortening, the observed heat plus work was less by a similar amount (6.2 mJ/g) than that expected from the simultaneous PCr splitting. Thus, up to a half of the extra energy liberated during rapid shortening is not associated with a simultaneous increase in PCr splitting. This energy balance discrepancy is rapidly reversed during the post-shortening isometric period so that in this period there is the energy balance discrepancy in the opposite direction. It should be noted here that the energy balance discrepancy seen during rapid shortening is different in nature from that seen during isometric contraction. The unexplained heat during isometric contraction is confined to the first few seconds of the tetanus and is not reversed during contraction.

Fig. 4.

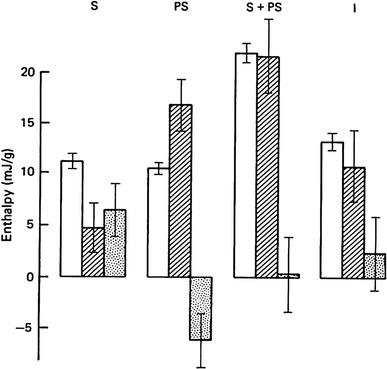
Enthalpy production (mJ/g) during rapid shortening. Unshaded, observed enthalpy (h + w); diagonal shading, explained enthalpy; dots, unexplained enthalpy (observed − explained). Columns from left to right show results for the rapid shortening period (S), post-shortening period (PS), the sum of these two (S + PS) and the period (I) of an isometric tetanus (of duration equal to that of S plus PS). Mean ± S.E. of mean. Homsher, Irving and Wallner [132]

Homsher et al. have also reported the results of a similar study as above but with the shortening at one-half maximum velocity [140]. The results have shown that a large amount of heat plus work was produced during the shortening period, and quite surprisingly this was accounted for by simultaneous PCr splitting. This is in sharp contrast to that obtained from the similar study performed at a shortening at V _max. To account for the energy balance discrepancy in muscles shortening at a high velocity, as well as the reversal of the energy balance discrepancy during the post-shortening period, models using the idea of incomplete cross-bridge cycles have been proposed [65, 121, 132]. Kodama and Yamada proposed a model based on an accumulation of an intermediate of actomyosin ATPase, M.ATP state, during shortening [121]. Irving and Woledge [65] and Homsher et al. [132] proposed a model with two different intermediate states between isometric and isotonic conditions. These models seemed plausible in explaining the energy balance discrepancy produced during the rapid shortening period, and its subsequent reversal during the post-shortening period. However, none of these models were able to explain the non-linear behavior of the energy balance discrepancy produced during the shortening period with the velocity of shortening.

Rall et al. proposed that, during rapid shortening, cross-bridge detachment from actin may occur before product dissociation, resulting in temporal dissociation of energy liberation and ATP hydrolysis [139]. Homsher suggested that, during rapid shortening, some cross-bridges would be forcedly detached from the thin filament after releasing P_i so that the cross-bridge with bound ADP would be accumulated; upon the cessation of shortening, dissociation of ADP would rapidly take place, followed by a burst of ATP cleavage [7]. Kawano et al. proposed from their ³¹P NMR study that immediately after the isometric tetanus is over, cross-bridges are in a state of bound ADP, and as ADP is released slowly, ATP is cleaved at a low temperature (“Phosphorus NMR studies”) [26]. Ohno and Kodama have shown that: (1) the turnover of ATP is negligibly small during rapid shortening of myofibrils and that (2) there is a transient burst of ATP hydrolysis after the rapid shortening ceases. From this and other evidence they have proposed that a cross-bridge with bound ADP and P_i could change over many actin monomers dissipating a fraction of the stored energy step by step [141, 142]. The conformational distortion thus induced pushes the actin filament. The delayed ATP hydrolysis would proceed without heat production. These are the most probable explanations of what occurs.

Phosphorus NMR studies

In the previous Section, the energy balance studies involving newly developed ultra-rapid freezing followed by extraction and chemical analysis have been described. Following the results of the chemical studies, Wilkie expressed serious doubt about the then current description of the situation [22]. The hydrolysis of PCr was insufficient to account for the concurrent heat plus work production, under conditions in which the only known net chemical reaction proceeding during contraction was thought to be the hydrolysis of PCr [1, 2]. This serious doubt described above led him to the then newly available non-destructive measurement of phosphorus compounds in intact muscles using ³¹P NMR.

In the 1970s NMR emerged as a novel method of studying the metabolism and anatomical structure of intact biological systems. ³¹P is one of the sensitive nuclei for NMR, and is the naturally occurring isotope of phosphorus. Wilkie was the first to study ³¹P NMR on biological samples in a well oxygenated physiological condition [22]. With colleagues he established a way to follow phosphorus compounds in living muscle during rest, contraction and recovery (see Figs. 5a, 6a). Figure 6a shows the time course of recovery of metabolites in frog sartorius muscles from contractions (25 s tetani). Both PCr and Pi recover with a half-time of approximately 10 min at 4 °C. The level of ATP did not change appreciably throughout.

Fig. 5.

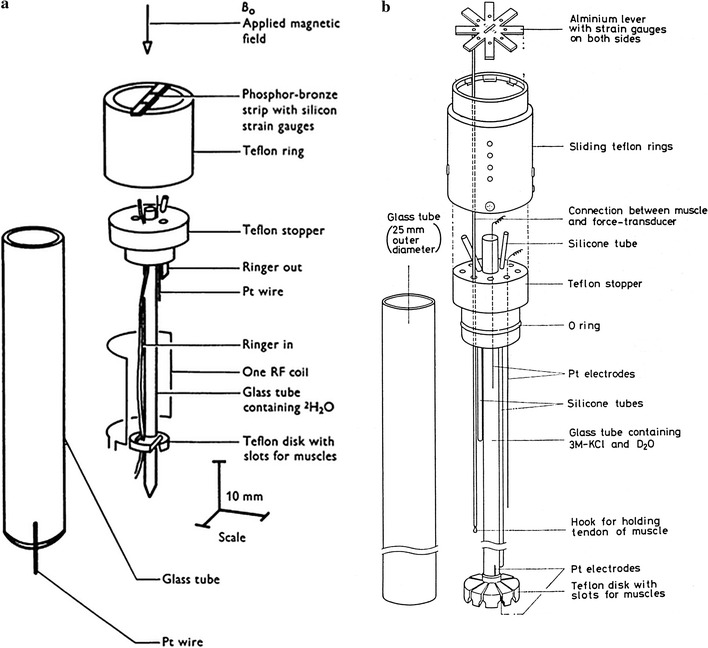
Design of experimental chambers for muscles. a Experimental chamber (7.5 mm diameter) for ³¹P NMR studies of contracting frog muscles. A single pair of frog sartorius muscles can be held in a vertical position parallel to the central glass tube by the support system constructed of Teflon, glass and epoxy resin. By these arrangements muscles can be stimulated, force produced can be recorded and the muscles can be superfused with oxygenated Ringer solution. Dawson et al. [22]. b Chamber of 25 mm diameter for ³¹P NMR studies, in which 8 pairs of semitendinosus muscles of bullfrogs can be held. The use of many muscles (average 4.4 g) greatly improved the signal intensity as is seen from the results shown in Fig. 6. Yamada and Tanokura [115]

Fig. 6.

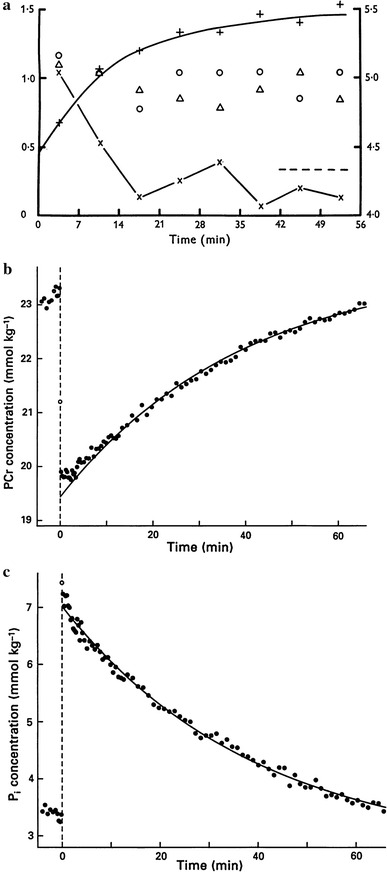
Time course of changes of metabolites after tetanic contractions of frog muscles studied by ³¹P NMR. a Recovery of frog muscles from contractions studied by using the chamber apparatus for ³¹P NMR shown in Fig. 5a. Four frog sartorius muscles were repeatedly stimulated for 25 s every 56 min and spectra accumulated into eight bins of 7 min each. The graph shows how PCr (+), P_i (×) and sugar P (Δ) varied. The ordinates show the resonance peak areas as multiples of the mean area for the β ATP peak. The right-hand scale applies to PCr. The exponential curve drawn through the PCr points has a T _1/2 of 9.1 min. Dawson, Gadian and Wilkie [22]. b The changes of PCr concentrations associated with 10-s isometric tetanus using chamber apparatus shown in Fig. 5b with sixteen dorsal heads of bullfrog semitendinosus muscles at 4 °C. Note that the time resolution is greatly improved compared to the results in a. The curve is the same exponential for P_i in c. Kawano, Tanokura and Yamada [26]. c Changes in P_i concentrations in the same experiments and muscle preparations as in b. The curve shows a single exponential fitted to the time course of the recovery of P_i (τ 37.5 min; T _1/2 26 min). Kawano, Tanokura and Yamada [26]

One of the major advantages of ³¹P NMR in muscle studies is that it provides a means to follow time courses of metabolite changes in the same muscle preparations. On the other hand a major disadvantage of NMR is its inherent lack of sensitivity. A simple way to improve the signal-to-noise ratio of ³¹P NMR is to increase the amount of samples. Another way of improving the sensitivity is to develop a mini-probe for small samples [143]. Yamada and Tanokura [115] and Kawano et al. [26] used many muscles (semitendinosus) of toad or bullfrog (Fig. 5b). They studied contraction and recovery of these muscles, which were kept in well-oxygenated conditions, with a time resolution of 16 s at 4 °C (Fig. 6b and c). Their results are the most quantitative study of metabolite changes in contraction and recovery of skeletal muscles using ³¹P NMR. Muscles were stimulated for various durations in separate experiments. Figure 6b and c shows the time course of the changes of P_i and PCr during recovery of 10 s isometric tetanic contractions. Figure 7 shows ΔP_i (●) and (ΔP_i + ΔPCr, ○), both of which are averages over three separate experiments for each, plotted against the duration of contractions. The inset of Fig. 7 shows (ΔP_i + ΔPCr) in an enlarged scale. The differences between ΔP_i and –ΔPCr, i.e., (ΔP_i + ΔPCr, ○) are 0.35 mmol.kg⁻¹ for 2, 5 and 10 s, and are less for 0.2- and 0.5-s contractions.

Fig. 7.

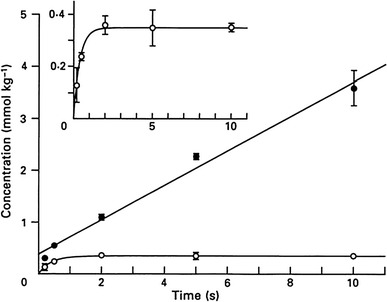
Inorganic phosphate released (ΔP_i), and the difference between ΔP_i and −ΔPCr, plotted against the duration of contraction. Vertical bars represent ±1 S.D. The inset shows the difference between ΔP_i and −ΔPCr (ΔPi + ΔPCr) in an enlarged scale. Kawano et al. [26]

The essential feature of their results may be summarized as follows. (1) The increase of Pi with the duration of stimulation is biphasic; consisting of an early burst of 0.38 mol/kg, which is complete within 0.2–0.5 s, being followed by a slower steady-state increase (Fig. 7). The steady-state rate is 0.33 mol/kg/s, which is in agreement with the results of previous studies using chemical analysis [31, 59, 117, 144]. (2) −ΔPCr coincided well with ΔPi except for the initial few minutes following relaxation, when −ΔPCr is smaller than ΔPi. The difference is 0.35 mol/kg. This value is comparable to the number of myosin heads (cross-bridges) in muscle (0.28 mmol/kg) [114] and also to the delayed splitting of PCr [2] (“Discovery of energy balance discrepancy”). From these, the results during the few minutes following relaxation can be explained by P_i being released and ADP remaining bound for a time after relaxation from contraction. This is particularly because the release of ADP from myosin extracted from rabbit muscle is markedly temperature dependent and is slow at a low temperature such as at 5 °C [133]. It has been shown that the rate constant of ADP dissociation from myosin-ADP complex derived from frog muscle is approximately 0.5 s⁻¹ at 0–5 °C [145], though the value might be less in intact muscle. Therefore, by assuming that a shift in actomyosin state occurs during contraction and is reversed by ATP utilization after the contraction is over, the unexplained energy discussed above may be accounted for at least partly (“Unexplained heat in isometric tetanus”).

The in vivo studies of living contracting muscle using NMR by Dawson et al. [22] provided a completely independent confirmation of the validity of the methods of chemical analysis. The greatest problem of the low sensitivity, and the consequent poor time resolution, of NMR was only overcome by Kawano et al. [26] in showing the post-contractile splitting of PCr described above (see “Unexplained heat in isometric tetanus”).

Calorimetric studies on muscle proteins

Troponin and troponin C

Ca binding to troponin

Troponin (Tn) is a complex of three proteins including troponin C (TnC) that binds Ca very strongly with binding constants in the range 10⁵ to 10⁹ M⁻¹. The binding of Ca to TnC initiates a series of events that transform actin into an activator of the myosin ATPase [146]. Proteins that bind Ca with high affinity possess characteristic domains which were first identified by Kretzinger and Nockolds in carp Pvalb [147]. They consist of a common structural motif of helix–loop–helix (HLH) or EF-hand. TnC has four Ca-binding sites in a molecule. Two sites in the C-terminal region (denoted as sites III and IV) show higher affinity for Ca among these four sites and also bind Mg (Ca–Mg sites). The affinity for Mg is lower than that for Ca; however, as the intracellular Mg concentration is much higher than Ca, these sites are occupied by Mg in resting muscle. When Ca concentration rises it will displace Mg from these sites but the process is slow because off-rate for Mg is slow. For this reason such sites will not be effective in triggering contraction in response to the rise in Ca concentration. The other two N-terminal sites (denoted as I and II) have lower affinity for Ca but do not bind Mg. It is generally agreed that sites I and II should be involved in the activation of contraction.

In energy balance studies described above, the energy output during muscle contraction was compared with the chemical changes associated with the contraction. In doing this, enthalpy changes (ΔH) of the chemical reactions must be known, and enthalpy changes can only be obtained by calorimetric measurements. In the energy balance studies by Gilbert et al., a rapid heat production appeared during the first few seconds of contraction, which amounts to 10 mcal/g (41.8 mJ/g) muscle and cannot be accounted for by PCr break-down [2]. They discuss that, in view of the amount of Ca, 0.07 μmol/g, needed to activate contraction (Ebashi et al. [114]), the unexplained enthalpy would be accounted for if the ΔH were 140 kcal/mol (586 kJ/mol) of Ca bound to Tn [2].

Yamada, Mashima and Ebashi have performed microcalorimetric measurements of Ca binding to Tn [82, 148]. Calorimetry was made using a type of rotation microcalorimeter, principal design of which is the Tian-Calvet’s conduction microcalorimeter [149]. Tn that was extracted from rabbit skeletal muscle was saturated with four Ca. Therefore, in order to remove bound Ca, Tn was passed through a column of Dowex-A1 chelating resin. By this procedure the level of Ca bound was reduced to less than 0.1 mol per mol of Tn. Ca was added to Ca-free Tn in several steps in the presence of 1 mM Mg at pH 7 at 10 °C. Enthalpy changes associated with the binding of Ca to Tn were estimated to be −39 kJ (−9.3 kcal) per mol Ca bound to the high-affinity sites and −74 kJ (−17.7 kcal) for the low-affinity sites. About 1 μmol Ca per gram myofibrils or, assuming 0.1 g myofibrils per gram muscle, 0.1 μmol Ca per gram muscle, is involved in the activation of contraction [150]. Thus, in view of the enthalpy change of −9.3 to −17.7 kcal (−39 to −74 kJ) per mol Ca bound, the heat production of 0.93 to 1.77 mcal (3.9 to 7.4 mJ) per gram muscle would be observed in the complete activation of contraction. The activation heat observed in muscle is 0.6 to 1.0 mcal (2.5 to 4.2 mJ) per gram muscle [80, 81]. Thus, the activation heat can be explained by the heat of Ca binding to Tn associated with the activation of contraction. This is by assuming that the initial rapid production of heat in isometric tetanus is related to the activation heat produced in twitch contraction. Proton exchanges of Tn on Ca binding and the heat associated with subsequent interaction with a buffer was negligibly small. However, the ultra-centrifugal sedimentation pattern is known to be altered by the level of Ca bound to Tn, so that at low Ca concentration Tn molecules have a tendency to associate with each other reversibly [151, 152]. A possibility exists therefore that, assuming a negative enthalpy change for the association of Tn molecules, dissociation of Tn molecules on Ca binding would absorb some heat.

Ca binding to troponin C (TnC)

Yamada and Kometani further performed the enthalpy titration of TnC, obtained from rabbit skeletal muscle, with Ca [153]. The microcalorimetric titration system had been improved so that titrations with Ca in a number of smaller steps were possible [134]. The effect of protons released on Ca binding to TnC and the subsequent interaction with the buffer produced only a negligible amount of heat. Sedimentation velocity measurements showed that, unlike Tn, TnC molecules do not aggregate when Ca is removed, in agreement with Murray and Kay [154].

It can be assumed that TnC has two high-affinity or Ca–Mg sites (III and IV), and two low-affinity Ca-specific sites (I and II) [155]. The results of enthalpy titrations of TnC with Ca have shown that the two high-affinity sites (III and IV) are distinct from each other when Mg is not present (Fig. 8). Note that, in Fig. 8, although heat is plotted against Ca added, the affinity of TnC for Ca in these sites is so strong that the Ca added almost corresponds to the Ca bound. The association constants for Ca of these sites are different by two orders of magnitude [153], in contrast to the results of binding studies (Potter et al. [156]). The results also indicated that, in a Mg-free condition, the binding of Ca to the site of highest affinity caused a prominent hydrophobic effect, i.e., large negative heat capacity changes,5 while Ca binding to the site having secondary-high affinity showed large positive heat capacity changes [Fig. 9a(a)]. The distinction between the two high-affinity sites in terms of both affinity for Ca and heat capacity changes on Ca binding disappeared in the presence of 1 mM Mg [Fig. 9a(b)].

Fig. 8.

Microcalorimetric titrations of TnC with Ca. Total heat produced per mol TnC against the concentration ratio of Ca (or Mg) added to TnC at pH7 and 10 °C. a Titration of Mg-free TnC with Ca; b in the presence of 0.2 mM Mg; c 1 mM Mg; d the titration with Mg. Yamada and Kometani [153]

Fig. 9.

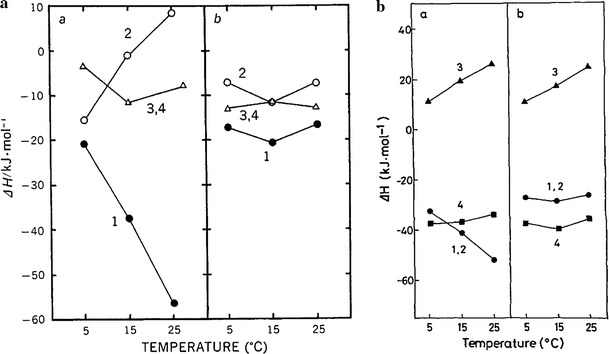
Dependence of enthalpy changes associated with Ca binding on temperature. a Rabbit TnC. a Mg-free TnC and b TnC in the presence of 1 mM Mg. Numbers attached indicate the particular site involved; 1 one of the Ca–Mg sites for which Ca binds first; 2 another Ca–Mg site for which Ca binds next; 3 and 4 Ca-specific sites. Yamada and Kometani [153]. b Bullfrog TnC. Symbols are the same as in a, except that the Mg concentration is 5 mM. Imaizumi and Tanokura [164]

TnC has multiple Ca binding sites with different affinities as well as enthalpies. Therefore, in order to determine these parameters from the results of calorimetric titrations, it was necessary to solve multiple non-linear equations with multiple parameters by employing iterative procedures. These processes might have introduced uncertainties into the interpretation of the results [153]. However, the main results were simple and clear. The robust results are: (1) the two high-affinity Ca–Mg sites in the C-terminal region are clearly distinct in terms of both affinities for Ca and the associated thermodynamic changes, and (2) the second step of Ca binding to the site having secondary-high affinity produces very little heat or even absorbs it. The large positive heat capacity changes associated with the Ca binding to the secondary-high affinity site can be analyzed further to indicate that hydrophobic residues become exposed to water and the molecular structure becomes less ordered. The characteristic behavior observed in the second step of Ca titration disappears in the presence of Mg, indicating that the site involved should correspond to one of the two Ca–Mg sites in rabbit skeletal muscle TnC. Ca binding to the two low-affinity Ca-specific sites (I and II) shows a moderate hydrophobic effect and is not affected by Mg (Fig. 9a(b)).

Potter, Hsu and Pownall have reported a calorimetric study of Ca binding to TnC, showing different results from those of Yamada and Kometani [153] in that all the four Ca sites have the same enthalpy of Ca binding [157]. The difference may be caused by the use of EDTA to remove Ca from TnC in Potter et al. [157].

Cardiac troponin C. It has been reported that cardiac TnC (cTnC) has two sites with high affinity and one site with low affinity for Ca [158]. Kometani and Yamada reported the results of enthalpy titrations of cTnC with Ca and Mg [159]. The results have shown that cTnC differs from skeletal TnC in the characteristics seen in the absence of Mg²⁺. In skeletal TnC it has been shown that, in the absence of Mg²⁺ the two high-affinity Ca sites are different from each other in their affinity for Ca and also in thermodynamic characteristics, whereas no such distinction between the two high-affinity Ca-binding sites is present in cTnC, both in the absence and presence of Mg²⁺. Thus, the two high-affinity Ca-sites in cTnC are identical in both affinity and enthalpy of binding for Ca.

Studies on peptide analogs

A common structural motif among Ca binding proteins, including TnC, Pvalb and calmodulin (CaM), is the EF hand or helix-loop-helix motif, where about 30 amino acids form each Ca binding site. A peptide analog of the helix-loop-helix Ca binding unit, similar to rabbit skeletal muscle TnC site III, has been synthesized [160]. This peptide forms a symmetric dimer in the presence of Ca [161]. Moreover, Shaw et al. have shown that the most stable is the heterodimer formed by the synthetic TnC site III and site VI, which is similar to the C-terminal domain of TnC [162]. Based on these studies they conclude that (1) Ca binding to the C-terminal region of TnC occurs sequentially, and (2) Ca affinity and the association of the two Ca binding domains are tightly linked. Hydrophobic interactions due to the formation of two distinct hydrophobic pockets at the interface of the two-site domain are not only responsible for the dimerization but also for the strong Ca affinity of the C-terminal region of TnC. These results on the synthetic peptide analogs of TnC correspond very well with the results by direct calorimetry of Ca binding by TnC [153].

Frog skeletal muscle TnC

Subsequent to the microcalorimetric enthalpy titration studies of rabbit muscle TnC with Ca, similar studies have been carried out on bullfrog skeletal muscle TnC [163, 164]. The essential features of the results of the enthalpy titration of the frog muscle TnC by Ca are quite similar to those of the rabbit muscle TnC except that, in frog muscle TnC, the characteristic positive enthalpy changes appear associated with the third Ca binding (one of the low-affinity sites I and II). Unlike the rabbit muscle TnC, this characteristic positive enthalpy change is not affected by Mg, implying that the site involved is one of the Ca-specific sites (Fig. 9b).

The characteristic Ca binding site, showing large positive heat capacity changes on Ca binding, should bear specific functionally important roles. The fact that this specific Ca binding site is associated with one of the Ca-specific low-affinity, or regulatory, sites in frog skeletal muscle TnC, instead of one of the Ca–Mg high-affinity sites as in rabbit skeletal TnC, is puzzling as well as interesting (see below). It should be noted here that the endothermic nature of Ca binding to the characteristic site has also been found associated with all four Ca binding sites of CaM bearing functional roles, while it is distinctly different from those of the CaM-trifluoperazine (TFP) complex and Pvalbs (“Ca binding to calmodulin”) [125, 165–167].

The characteristic Ca binding sites of TnC, which show anomalous positive enthalpy changes as well as positive heat capacity changes, should have distinct roles associated with the regulation of contraction. In frog (and possibly in avian) muscle this type of characteristic Ca binding site may be directly used for a regulatory purpose, while in rabbit (and possibly in other mammalian) muscle this characteristic site is used for establishing the structural basis, on which the regulatory process takes place.

Parvalbumin and calmodulin

Ca binding to parvalbumins

Parvalbumins (Pvalbs) are present in large quantities, mostly in white muscles of lower vertebrates. They have two Ca-binding sites per molecule, which also bind Mg competitively (Ca–Mg sites). Their primary structures are homologous to those of TnC and CaM [168]. They are classified into two genetically different categories. They have been considered to have a role as a soluble relaxing factor [122, 123, 169, 170]. A hypothesis has been put forward that the unexplained energy and the labile component of heat produced during an isometric tetanus originate partly from the binding of Ca ions to Pvalb (“Unexplained heat in isometric tetanus”). Results of enthalpy titrations of frog and toad Pvalbs have been reported by Tanokura and Yamada [125], Smith and Woledge [126], Tanokura, Imaizumi and Yamada [171] and Tanokura and Yamada [127]. According to Tanokura and Yamada, enthalpy changes (ΔH) of Ca binding are −34.6 (isotype PA1, bullfrog) and −26.7 kJ/mol site (PA2) in the presence of 5 mM Mg at 25 °C and pH 7.0 [125]. Smith and Woledge also reported enthalpy changes of −33 kJ/mol site (isotype 1Vb, frog) in 1 mM Mg at 12 °C and pH 8.0 [126]. The binding of Ca to Pvalbs is exothermic at various temperatures in both the presence and absence of Mg [127]. The heat capacity changes are always negative, a characteristic of ligand binding, but different from other Ca-binding proteins such as TnC [“Ca binding to troponin C (TnC)”].

Ca binding to calmodulin

Calmodulin (CaM) is one of the Ca-binding proteins and plays important roles in many intracellular Ca-dependent functions [172]. Enthalpy titrations of CaM with Ca by microcalorimetry have been reported using bovine brain [165, 166] and wheat germ CaM [173]. The binding of both Ca and Mg to CaM are endothermic. It has been confirmed that all four metal binding sites are Ca–Mg sites, Ca displacing Mg. When Ca was introduced to metal-free CaM, heat capacity changes were negative but much smaller than those of skeletal and cardiac troponin C. These results may indicate that the changes in molecular structure caused by Ca binding are different from that in troponin C, but that the end results are similar to those with troponin C. In this context, it is of great interest that, in the presence of TFP, one of the inhibitors of CaM, Ca binding to the CaM-TFP complex becomes exothermic [167, 174].

Myosin and actomyosin ATPase

Pioneering calorimetric studies on the myosin ATPase mechanism have been reported by Yamada, Shimizu and Suga [175]. They showed that when ADP was added to heavy meromyosin (HMM, a fractional part of myosin that is obtained by treating with trypsin and possesses all the ATPase properties of myosin), a rapid heat production was observed. Because ADP binds strongly to HMM this heat should come from the reversal of the reaction in which ADP dissociates from myosin (M) (reaction 4 in the scheme below). When ATP was used instead of ADP, a similar heat was produced as for ADP, and this was followed by a slow heat production, the rate of which coincided with that known for the release of P_i from the M.ADP.P_i complex. These results show that reaction 3 below is exothermic and that the initial rapid heat must come from reactions 1 and 2 together.

Kodama and Woledge [134], Kodama, Watson and Woledge [176] and Kodama and Woledge [177] studied the problem further with improved methods excluding heat of mixing and with much precision and confirmed that the reaction 4 in the scheme is indeed endothermic, ΔH being +60 ~ + 90 kJ mol⁻¹. They also studied the effect of introducing ATP to HMM or S1, essentially confirming the results of Yamada et al. [175]. The time course of the heat production showed a small rapid phase (27 kJ mol⁻¹), followed by a large slower phase of heat production (125 kJ mol⁻¹) [177]. The rate of the slow heat production was exponential and was in agreement with the rate of dissociation of P_i.

$$\text{M}+\text{ATP}\stackrel{1}{⟶}\text{M}.\text{ATP}\stackrel{2}{⟶}\text{M}.\text{ADP}.{\text{P}}_i\stackrel{3}{⟶}\text{M}.\text{ADP}+{\text{P}}_i\stackrel{4}{⟶}\text{M}+\text{ADP}+{\text{P}}_{\text{i}}$$ 16

The amount of the initial phase of rapid heat production increased when non-hydrolysable ATP analogs (AMP.PNP and ATPγS) were used instead of ATP, to 90 to 110 kJ mol⁻¹, respectively. Because this initial rapid phase of heat should come from reactions 1 and 2 together when ATP was the substrate, the heat of reaction 2 (i.e., splitting of ATP on myosin ATPase site) should be endothermic, ΔH being +51 kJ mol⁻¹. This is in sharp contrast to the enthalpy of ATP hydrolysis in solution, which is known to be exothermic as expected (−20 kJ mol⁻¹).

The strong binding of ATP to myosin (reaction 1) is driven by a large negative enthalpy change. Therefore, applying the second law of thermodynamics, entropy changes should be negative. On the other hand, the step, in which ATP is hydrolyzed by the myosin active site, should be driven by a positive entropy change. When P_i is released (reaction 3) the entropy change becomes negative again. These results lead to an interesting possibility that the increase of entropy associated with the ATP splitting on myosin might correspond to a loss of water molecules from the protein surface, and thereby a breakdown of ordered water structure [135]. Because the magnitudes of hydrophobic contributions are not known it is difficult to deduce this from thermodynamic data [178]. On the other hand, a recent study of the hydration of myosin S1 using dielectric spectroscopy confirmed that when S1 changes from the S1.ADP state into the S1.ADP.P_i state (ADP release followed by ATP binding and splitting) water molecules are actually released [179]. Relatively little calorimetric work has been done on the reactions involving the actomyosin system. Actomyosin hydrolyzes ATP much more rapidly than myosin and this factor is likely to be the major difficulty. Therefore, these are not dealt with further here.

Hindsight and foresight

Since the pioneering study of Hill and Hartree, heat production of muscle has drawn much attention [57]. Although heat studies set a framework for all studies, one of the characteristics of heat production is its lack of specificity. For this very reason, for more than three decades following the middle of the 1960s much research was devoted to overcoming this difficulty. In other words, muscle produces heat and work from chemical reactions. Therefore, an attempt has been made to answer questions about what these reactions are and, more recently, how much of the enthalpy (heat + work) does each reaction produce. Earlier attempts have revealed that the energy output can be divided into the anaerobic reactions that occur during contraction, giving rise to the work and the initial heat, and the aerobic reactions that occur largely after contraction providing recovery heat. It has been recognized that PCr splitting is a major contributor to the initial processes. It has also been recognized that the control of contraction requires a considerable amount of energy expenditure, involving Ca release and reuptake by SR.

The attempt to answer the question of identifying all the chemical reactions involved in contraction is the energy balance study, which requires precise measurements of both energy output and of chemical change. It has been established through these studies that PCr splitting cannot explain the whole of the initial heat. The energy balance discrepancy observed in isometric contraction may well be explained by additional reactions to PCr spitting. These reactions are Ca binding to Tn and Pvalb, as well as a shift of actomyosin states during contraction (an incomplete cycle of actomyosin ATPase). On the other hand, the energy balance discrepancy observed in rapid shortening waits for further studies.

Meanwhile, one of the aims of A. V. Hill’s effort was to record accurate time courses of the production of heat during contraction of muscle under various mechanical conditions [1]. In this context, the complex time course of shortening heat production, for example, observed during the force redevelopment after a small quick release of contracting muscle by Gilbert and Matsumoto seems to be of great interest [102]. Further, energy balance discrepancies in rapidly shortening muscle open a broad field of research including in vitro studies.

On the other hand, we now see unexpected novel findings continue to appear and enlighten the field.

Compliance with ethical standards

Conflict of interest

The author declares that he has no conflict of interest.