Abstract
We introduce the refractiveindex.info database, a comprehensive open-source repository containing optical constants for a wide array of materials, and describe in detail the underlying dataset. This collection, derived from a meticulous compilation of data sourced from peer-reviewed publications, manufacturers’ datasheets, and authoritative texts, aims to advance research in optics and photonics. The data is stored using a YAML-based format, ensuring integrity, consistency, and ease of access. Each record is accompanied by detailed metadata, facilitating a comprehensive understanding and efficient utilization of the data. In this descriptor, we outline the data curation protocols and the file format used for data records, and briefly demonstrate how the data can be organized in a user-friendly fashion akin to the books in a traditional library.
Subject terms: Optical materials and structures, Materials for optics
Background & summary
The complex index of refraction is essential for understanding the optical properties of materials due to its close relation with the relative permittivity εr ( in the case of non-magnetic materials1). In this context, n is the phase velocity ratio of light in a vacuum to that in the material, representing refraction. The extinction coefficient k measures absorption. The absorption coefficient α can be expressed as , with λ representing the light’s wavelength.
Accurately determining both n and k, and their chromatic dispersion, is crucial in science, engineering, and 3D artistic rendering. The intricate design of optical instruments, particularly those minimizing aberrations, depends heavily on understanding the n(λ) function of transparent optical materials2. Cross-disciplinary efforts to characterize optical material properties have resulted in a wealth of datasets and analytical formulas, enhancing our grasp and utilization of optical constants.
Initiatives to amalgamate scattered information on optical constants resulted in seminal works like Palik’s comprehensive compilation3, offering structured and uniformly formatted data. Though monumental, the rapid advancement of technology and research methodologies signposted the need for a more dynamic, easily accessible repository of n and k values. This need intensified with the emergence of high-peak-power lasers, highlighting the critical role of the nonlinear refractive index, n2, in decoding material behavior under intense light. n2 characterizes the refractive index’s variation with the optical field intensity I, expressed as n = n0 + n2I, where n0 is the refractive index at zero intensity.
The refractiveindex.info database emerged in response, offering a systematically organized, dynamic repository of optical constants. Since its inception in 2008, continuous enhancements have established it as a reliable resource, with the YAML-based file format ensuring data integrity and ease of access. The integration of the n2 database in 2023 reaffirms our dedication to addressing modern challenges in optics and photonics.
The subsequent sections delve into the methods employed for collecting the data included in refractiveindex.info, detailing the file format used for storing data records and our approach to verifying dataset integrity. We then highlight the application aspects of this dataset, illustrated with an example of an interactive data browser, and conclude with a statement on data availability.
Methods
Data source clarification
It is pivotal to underscore that our work does not generate original experimental data. Instead, we create a comprehensive data repository, systematizing and cataloging existing optical constants published by others. This approach ensures a diverse and rich collection of verified data is readily accessible for various applications in a standardized format. As a result, this section does not describe experimental procedures but focuses on the methodologies employed in data collection and formatting.
Data collection
Data on the optical properties of materials is aggregated from a variety of publicly accessible and credible sources, ensuring a wide range of optical constants is represented. The systematic collection process involves categorizing the data based on the type of material, its properties, and the source of information. The entire process is mostly manual due to the large variety of data sources and presentation methods. Below, we detail the steps typically undertaken to identify sources, extract data, and convert it into a unified format used in the data records.
Identification of data sources
Existing data collections: The initial sources for literature containing data on optical constants were published collections, such as those included in the Handbook of Optics4. The publications referenced therein constituted our initial list of data sources.
Cross-references from the initial list of data sources: In the next iteration, we scan for references to other publications in the sources identified in the previous step (usually, scientific papers reporting new measurements describe previous works on similar topics).
Search engines: We perform a broader search for publications on optical properties of materials using tools like Google Scholar to find newer references and those that may have been missed in earlier steps.
Glass makers’ catalogs: We checked the websites of glass manufacturers for catalogs of glasses they produce. These catalogs are often available in Zemax AGF format, suitable for automatic data extraction.
User input: At the present maturity level of the project, most new data sources are recommended by users. Researchers often provide us with data in a format ready-to-include in the refractiveindex.info dataset as soon as the data are first published.
-
2.
Data extraction
Dispersion formula: When the linear refractive index as a function of wavelength is given as a dispersion formula, the coefficients are manually transferred to the data record. Adjustments are made if necessary to fit the formula to one of the standard forms documented in the following section.
Tabulated numerical data: In cases where optical constants are presented in tabulated numerical form, the data are transferred to the data record with minimal changes required by the used format (e.g., using micrometer as the unit of wavelength and expressing internal absorption by extinction coefficient k). This process is simplified if data is in a standard table format (e.g., CSV or Microsoft Excel) in supplementary materials or directly provided by authors. For older publications, typically available as bitmap-based PDF files, text recognition options in PDF reading software prove helpful.
Models: If a reference presents a model for calculating optical constants, a Python script is developed to generate a tabulated data record compatible with the required format. This script is then made publicly available to refractiveindex.info users on GitHub.
Graphical-only data: Occasionally, authors choose to include only graphical data in publications without numerical data. In such cases, we first attempt to contact the authors for the original data. If this is not feasible (in the case of older publications) or if there is no response, semi-automatic digitization of the plots is sometimes performed (e.g., using Engauge Digitizer software); however, this is considered a last resort due to its time-consuming and less accurate nature and is typically used only when no alternative data is available.
Glass catalogs: Glass makers’ catalogs in AGF format are automatically converted into refractiveindex.info data records using a dedicated Python script.
Data formatting and updates
All collected data are converted into a standardized format stored in human-readable YAML files, as detailed in the following section. This approach ensures consistency and facilitates easy access and manipulation by both users and computer programs.
Errors in converting original data to standardized data records occasionally occur and are typically reported by users. All efforts are made to implement necessary corrections promptly via regular updates of the dataset.
Data Records
The dataset is available at Figshare5.
YAML-based file format for data records
The data records employ YAML-based file format (https://yaml.org), ensuring the data is both easily readable and maintainable. Each YAML file encompasses data related to a specific material, evaluated under defined conditions and reported in a particular publication, with a primary focus on the refractive index and extinction coefficient.
As an illustrative example, consider a data record for SiO2 in which the refractive index n is expressed through a dispersion formula. The associated YAML file is located at data-nk/main/SiO2/Malitson.yml and is organized as follows:
REFERENCES: “1) I. H. Malitson, J. Opt. Soc. Am. 55, 1205-1209 (1965)...
COMMENTS: “Fused silica, 20 °C”
DATA:– type: formula 1wavelength_range: 0.21 6.7coefficients: 0 0.6961663 0.0684043 0.4079426...SPECS:n_is_absolute: falsewavelength_is_vacuum: false
temperature: 20 °C
Every YAML data file primarily consists of two mandatory fields: REFERENCES and DATA. REFERENCES cite the source of the data, while DATA provides the values of the optical constants. There are also two optional fields, COMMENTS and SPECS, offering additional context and structured information respectively.
DATA
In the case of a data record for linear optical constants, the DATA field can be specified as a dispersion formula, identified by a formula number, or as tabulated data sets of n(λ) and/or k(λ). For dispersion formulas, the ‘wavelength_range’ entry indicates the applicable wavelength range for the data, always expressed in micrometers. Each dispersion formula in the database is numerically identified and described as follows:
| 1 |
| 2 |
| 3 |
| 4 |
| 5 |
| 6 |
| 7 |
| 8 |
| 9 |
In another example, found at data-nk/main/Si/Aspnes.yml, n(λ) and k(λ) for Silicon (Si) are provided in tabulated numerical form:
DATA:- type: tabulated nkdata: |0.2066 1.010 2.9090.2101 1.083 2.982...
Here, the first column corresponds to the wavelength in micrometers, while the others represent the unitless refractive index n and extinction coefficient k. Alternatively, n and k can be outlined separately in two two-column entries. A data entry might also combine a dispersion formula for n with numerical data for k, as illustrated in the SCHOTT N-BK7 glass dataset at data-nk/glass/schott/N-BK7.yml:
DATA:- type: formula 2wavelength_range: 0.3 2.5coefficients: 0 1.03961212 0.00600069867 0.231792344...- type: tabulated kdata: |0.300 2.8607E-060.310 1.3679E-060.320 6.6608E-07...
In n2 datasets, the data are always presented numerically. An example can be found at data-n2/main/SiO2/Flom.yml. n2 is expressed in m2/W:
DATA:- type: tabulated n2data: |0.772 2.07e-201.030 2.23e-201.550 2.42e-20SPECS:n2_method: Z-scanpulse_duration: 280e-15 140e-15 97e-15
It is noteworthy that for n2, information on the measurement method and pulse duration is essential for comparing data from different sources. This information is specified in the optional SPECS field.
COMMENTS and SPECS
The COMMENTS field can incorporate additional information to provide context to the data, while the SPECS field presents structured data in key-value pairs, giving machine-interpretable insights into specific measurement conditions or additional properties.
In the initial example, the SPECS entries clarify that the refractive index is not absolute and is measured relative to air, that the wavelength is gauged under atmospheric conditions rather than in vacuum, and that the data is applicable at a temperature of 20 °C. In the preceding example concerning n2, the SPECS delineate the use of the Z-scan measurement method, specifying pulse durations of 280 fs, 140 fs, and 97 fs for the corresponding data points. Furthermore, the SPECS section is adaptable to encapsulate an enhanced depth of information regarding the material, as illustrated in the forthcoming example for SCHOTT N-BK7 glass:
SPECS:...thermal_dispersion:- type: “Schott formula”coefficients: 1.86e-06 1.31e-08 −1.37e-11 4.34e-07 6.27e-10 0.17nd: 1.5168Vd: 64.17glass_code: 517642.251glass_status: standard...acid_resistance: 1.0alkali_resistance: 2.3phosphate_resistance: 2.3
Inclusion of additional information on optical glasses makes the data records suitable for use in optical design software.
Notes on data records
Adhering strictly to the syntactical rules of YAML is paramount. This adherence includes the mandatory use of spaces for indentation (tabs are prohibited) and the application of UTF-8 encoding without BOM, ensuring consistency and readability across diverse data records. While not a stringent requirement, a uniform format for similar entries, particularly references, is encouraged to optimize the user experience and enhance data interpretability.
Unless explicitly defined, non-prefixed SI units (e.g., watts, meters, seconds) are the default to ensure unambiguous and standardized data representation and interpretation. For instance, n2 is expressed in units of m2/W. However, an exception exists for the wavelength, which is always specified in micrometers to comply with a general practice accepted in optical design and, in particular, to allow the direct use of published dispersion formulas that traditionally assume wavelength expressed in micrometers.
Technical Validation
Since the described dataset5 represents an extensive array of data compiled by the work of thousands of researchers over more than a century, we cannot verify the accuracy of every individual record. Instead, we rely on the peer-review process employed by publishers of scientific and technical journals, and on the experience and reputation of optical material manufacturers publishing their material properties. However, we make every effort to ensure the correctness of the data extraction and conversion process, as well as the consistency of the information included in the dataset. In particular, the following steps are typically involved in the process of adding a new data record.
Verification and documentation of data attribution: We trace the origin of the data to the first publication where it appeared and include the corresponding information in the data record file, ensuring users can validate the origins of the data. If the original data were re-analyzed, or a combination of data from multiple sources was used in a later publication (for example, to produce a dispersion formula valid in an extended wavelength range), this fact is also documented in the REFERENCES field of the data record file.
- Verification of accuracy of data extraction: Several tests are used to ensure the absence of errors in the conversion process:
- Manual number-by-number comparison: This test is routinely performed when the data is represented by a relatively small amount of numerical values, e.g., coefficients of a dispersion formula.
- Plot comparison: We use Python scripts that automatically read YAML data record files and plot the included optical constants as a function of wavelength. Comparing these plots with those included in the original publications is a robust tool for spotting inconsistencies.
- Looking for data abnormalities: A deviation of a data point from the general trend in the data record is usually an indication of a data extraction error. All such abnormalities are manually compared against the original data, and necessary corrections are made.
- Cross-entry comparison: Plotting data entries for the same material from different sources on the same plot may help reveal a systematic error in a particular data record if a corresponding curve deviates from a general trend. An example of a Python-based user interface allowing for easy data comparison is given in the following section.
Testing of data file adherence to the YAML standard: This is typically performed by verifying the as-expected operation of several scripts used to access the data and relying on standardized YAML processing libraries. Error or warning messages generated by these scripts indicate a problem in the data record file that must be identified and corrected.
User feedback: This is our strongest defense line in assuring the integrity of the data records. The users report errors via the GitHub page of the refractiveindex.info project or by directly contacting the maintainer. Each report is analyzed, and necessary corrections are promptly implemented.
Usage Notes
The described dataset5 is a collection of human-readable YAML files, meticulously organized for user convenience. While these files can be directly individually accessed for specific optical constants of materials, organizing them in a logically-structured way and employing computer programs for data retrieval and analysis unleashes the dataset’s comprehensive utility. The YAML format, a standardized data serialization language, ensures that the data files are easily parsable with libraries such as PyYAML for Python and libyaml for C/C++.
The refractiveindex.info database is build upon the dataset described in the previous sections and include additions aimed at simplifying the access and navigation through the data. The main addition is a descriptor defining a hierarchical structure akin to a library. In this setup, each data record is akin to a "page," housed within a "book," and all such books are systematically arranged on different "shelves." This logical structure is defined and maintained through YAML-based catalog files, that categorize each data record following the ‘library’ analogy and indicate the relative path of the corresponding data records.
The library is defined by two catalog files: catalog-nk.yml for linear optical properties and catalog-n2.yml for nonlinear properties. Below is an excerpt from the catalog-n2.yml file, exemplifying the integration of HTML typesetting and showcasing optional entries like ‘DIVIDER’ and ‘info’. The ‘DIVIDER’ is employed to separate distinct groups of books or pages clearly, while ‘info’ links to an HTML file furnishing extra details about a particular shelf, book, or page.
- SHELF: mainname: “MAIN - simple inorganic materials”content:- DIVIDER: “Al - Aluminates, Aluminium garnets”- BOOK: BeAl2O4name: “BeAl2O4 (Beryllium aluminate, chrysoberyl)”info: “main/BeAl2O4.html”content:- PAGE: Adairname: “Adair 1989”data: “main/BeAl2O4/Adair.yml”...- BOOK: MgAl2O4name: “MgAl2O4 (Magnesium aluminate, spinel)”info: “main/MgAl2O4.html”content:- PAGE: Flomname: “Flom et al. 2015”data: “main/MgAl2O4/Flom.yml”- PAGE: Adairname: “Adair et al. 1989”data: “main/MgAl2O4/Adair.yml”...
In this schema:
‘SHELF’ represents a specific category or collection of materials.
‘BOOK’ denotes a particular material.
‘PAGE’ entries detail individual data records associated with a material.
‘DIVIDER’ assists in visually and logically separating related materials or data records, enhancing the navigation experience.
The ‘info’ entry specifies paths to additional HTML-based information, enabling users to access in-depth insights. Each ‘PAGE’ entry is linked with a ‘data’ field, pointing to the exact location of the data record’s YAML file within the dataset. The ‘name’ entry provides a “long” name for a shelf, book, or page in HTML typesetting. Paths to the data files for the linear (nk) and nonlinear (n2) subsets of the database, as well as to the HTML files with additional information (info), are relative to the data-nk, data-n2, and info directories, respectively, all located in the database’s root directory.
It’s important to note that users can create alternative ‘catalog’ files tailored to their specific needs. For instance, a customized catalog can contain only a subset of the dataset that is relevant for a particular application or study.
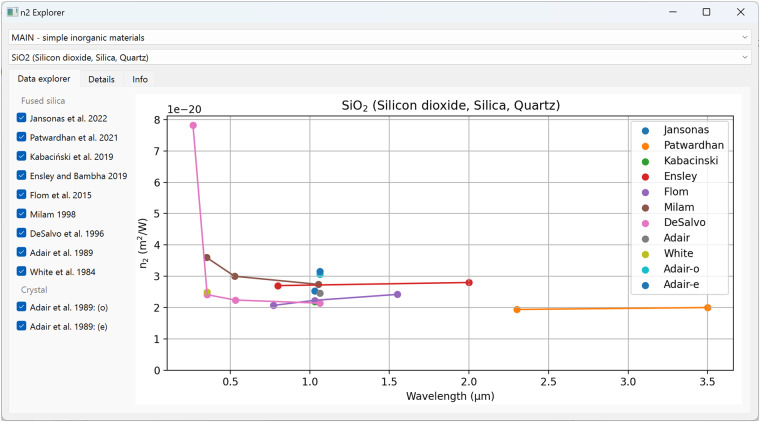
To aid users in navigating the data, two Python scripts, nkexplorer.py and n2explorer.py, are housed in the tools folder in the root directory of the refractiveindex.info database. These utilities, boasting a QT-based graphical interface, facilitate the location and comparison of data from a variety of sources. Figure 1 displays the n2explorer.py interface, illustrating the visual comparison of n2 data for SiO2 sourced from multiple publications.
Fig. 1.
The n2explorer.py script’s graphical user interface facilitates visual navigation through the n2 data. The nkexplorer.py script provides a similar interface for exploring linear optical constants.
Furthermore, users have the option to access the database through the RefractiveIndex.INFO website (https://refractiveindex.info). This online platform facilitates the browsing of data records and computations of various optical properties linked with n and k constants, such as Abbe numbers, reflectance, and Brewster’s angle.
A variety of third-party scripts and web applications that harness the refractiveindex.info dataset can be found, notably on GitHub. These tools offer users alternative avenues to efficiently access and employ the data.
Acknowledgements
The development of the n2 segment of the refractiveindex.info database was facilitated by grants from the US Department of Energy Accelerator Stewardship Program. Originally a part of the Office of High Energy Physics (HEP), this program is now under the purview of the Accelerator Research & Development and Production (ARDAP) office. The author extends heartfelt appreciation to the multitude of contributors from the scientific and engineering realms. Their contributions, spanning data submissions, error reporting, and invaluable insights, have been instrumental in the refinement and expansion of the database. The collective engagement of this dedicated community has been pivotal in elevating the database to its current stature of utility and comprehensiveness.
Author contributions
M.N.P. conceptualized, created, and has maintained the refractiveindex.info database throughout the years, ensuring its continuous update and improvement to meet the evolving needs of the optics and photonics community. The compilation, verification, and organization of the data, as well as the development and maintenance of the associated tools included with the database, were all carried out by M.N.P. The invaluable feedback and data contributions from the user community have played a crucial role in enhancing the comprehensiveness and accuracy of the database.
Code availability
The dataset described here, which represents the core of the refractiveindex.info database, is available at Figshare5. It presently (as of December 2023) contains 3135 data records on 605 materials in the part of the dataset corresponding to linear optical properties (nk), and 193 records on 89 materials in the part corresponding to nonlinear optical properties (n2).
The code that underpins the refractiveindex.info database is made accessible under the Creative Commons Zero (CC0) license (https://creativecommons.org/publicdomain/zero/1.0). This license facilitates the unrestricted use, distribution, and modification of the code, making it widely accessible for various applications. The entire codebase, including detailed documentation, is publicly available on the refractiveindex.info-database GitHub project (https://github.com/polyanskiy/refractiveindex.info-database). This repository is regularly updated, ensuring it evolves to meet the ongoing needs of both the scientific and engineering sectors. For additional utility, users can explore the refractiveindex.info-scripts project on GitHub (https://github.com/polyanskiy/refractiveindex.info-scripts), which offers scripts for deriving optical constants from established models and tools for converting Zemax glass catalogs to the dataset’s YAML format.
It is essential to note that the data encapsulated within the refractiveindex.info dataset is meticulously curated from publicly available sources. This includes peer-reviewed journals, authoritative books, and manufacturer datasheets, ensuring that the dataset is not only expansive but also anchored in reliability and veracity. Each data record within the dataset explicitly cites the source, offering users a pathway to delve deeper into the original data and its context. All journal papers from which data are presently used in the refractiveindex.info dataset, excluding those without a DOI identifier, are included in the following reference list6–471.
By integrating a comprehensive data collection, adopting a standard-based data file format, ensuring ongoing updates, and maintaining open access, the refractiveindex.info emerges as an essential tool for researchers, engineers, and students delving into the complex world of optical constants and material properties.
Competing interests
The author declares no competing interests. However, for complete transparency, it is noted that the author is the owner of the RefractiveIndex.INFO website, which utilizes the refractiveindex.info database to provide optical constants. The website generates modest revenue from advertisements, which primarily covers maintenance expenses including web hosting, domain registration, and email services.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- 1.Born, M. & Wolf, E. Principles of optics (Cambridge University Press, Cambridge, 1999).
- 2.Smith, W. J. Modern Optical Engineering (McGraw-Hill, New York, 2007).
- 3.Palik, E. D. (ed.) Handbook of Optical Constants of Solids (Academic Press, San Diego, 1998).
- 4.Bass M, et al., editors. Handbook of Optics, Volume IV: Optical Properties of Materials, Nonlinear Optics, Quantum Optics. New York: McGraw-Hill; 2009. [Google Scholar]
- 5.Polyanskiy MN. 2024. refractiveindex.info data set. Figshare. [DOI]
- 6.Adachi S. Optical dispersion relations for GaP, GaAs, GaSb, InP, InAs, InSb, AlxGa1-xAs, and In1-xGaxAsyP1-y. Journal of Applied Physics. 1989;66:6030–6040. doi: 10.1063/1.343580. [DOI] [Google Scholar]
- 7.Adachi S. Optical dispersion relations for AlSb from E=0 to 6.0 eV. Journal of Applied Physics. 1990;67:6427–6431. doi: 10.1063/1.345115. [DOI] [Google Scholar]
- 8.Adachi S, Taguchi T. Optical properties of ZnSe. Physical Review B. 1991;43:9569–9577. doi: 10.1103/physrevb.43.9569. [DOI] [PubMed] [Google Scholar]
- 9.Adachi S, Kimura T, Suzuki N. Optical properties of CdTe: Experiment and modeling. Journal of Applied Physics. 1993;74:3435–3441. doi: 10.1063/1.354543. [DOI] [Google Scholar]
- 10.Adair R, Chase LL, Payne SA. Nonlinear refractive-index measurements of glasses using three-wave frequency mixing. Journal of the Optical Society of America B. 1987;4:875. doi: 10.1364/josab.4.000875. [DOI] [Google Scholar]
- 11.Adair R, Chase LL, Payne SA. Nonlinear refractive index of optical crystals. Physical Review B. 1989;39:3337–3350. doi: 10.1103/physrevb.39.3337. [DOI] [PubMed] [Google Scholar]
- 12.Afsar MN, Hasted JB. Measurements of the optical constants of liquid H2O and D2O between 6 and 450 cm−1. Journal of the Optical Society of America. 1977;67:902. doi: 10.1364/josa.67.000902. [DOI] [Google Scholar]
- 13.Aftenieva, O. et al. Lasing by template-assisted self-assembled quantum dots. Advanced Optical Materials11, 10.1002/adom.202202226 (2023).
- 14.Aftenieva O, et al. Directional amplified photoluminescence through large-area perovskite-based metasurfaces. ACS Nano. 2023;17:2399–2410. doi: 10.1021/acsnano.2c09482. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Aguilar O, de Castro S, Godoy MPF, Rebello Sousa Dias M. Optoelectronic characterization of Zn1-xCdxO thin films as an alternative to photonic crystals in organic solar cells. Optical Materials Express. 2019;9:3638. doi: 10.1364/ome.9.003638. [DOI] [Google Scholar]
- 16.Harasaki Akiko, Kato Kiyoshi. New data on the nonlinear optical constant, phase-matching, and optical damage of AgGaS2. Japanese Journal of Applied Physics. 1997;36:700. doi: 10.1143/jjap.36.700. [DOI] [Google Scholar]
- 17.Al-Kuhaili M. Optical properties of hafnium oxide thin films and their application in energy-efficient windows. Optical Materials. 2004;27:383–387. doi: 10.1016/j.optmat.2004.04.014. [DOI] [Google Scholar]
- 18.Althoff R, Hertz J. Measurement of the optical constants of Na and K in the range of wavelength from 2.5 to 10 μ. Infrared Physics. 1967;7:11–16. doi: 10.1016/0020-0891(67)90025-5. [DOI] [Google Scholar]
- 19.Amotchkina T, Trubetskov M, Hahner D, Pervak V. Characterization of e-beam evaporated Ge, YbF3, ZnS, and LaF3 thin films for laser-oriented coatings. Applied Optics. 2019;59:A40. doi: 10.1364/ao.59.000a40. [DOI] [PubMed] [Google Scholar]
- 20.Arakawa ET, Williams MW, Inagaki T. Optical properties of arc-evaporated carbon films between 0.6 and 3.8 eV. Journal of Applied Physics. 1977;48:3176–3177. doi: 10.1063/1.324057. [DOI] [Google Scholar]
- 21.Arakawa ET, Williams MW, Ashley JC, Painter LR. The optical properties of kapton: Measurement and applications. Journal of Applied Physics. 1981;52:3579–3582. doi: 10.1063/1.329140. [DOI] [Google Scholar]
- 22.Arndt DP, et al. Multiple determination of the optical constants of thin-film coating materials. Applied Optics. 1984;23:3571. doi: 10.1364/ao.23.003571. [DOI] [PubMed] [Google Scholar]
- 23.Arosa Y, de la Fuente R. Refractive index spectroscopy and material dispersion in fused silica glass. Optics Letters. 2020;45:4268. doi: 10.1364/ol.395510. [DOI] [PubMed] [Google Scholar]
- 24.Aspnes DE, Studna AA. Dielectric functions and optical parameters of Si, Ge, GaP, GaAs, GaSb, InP, InAs, and InSb from 1.5 to 6.0 eV. Physical Review B. 1983;27:985–1009. doi: 10.1103/physrevb.27.985. [DOI] [Google Scholar]
- 25.Aspnes DE, Kelso SM, Logan RA, Bhat R. Optical properties of AlxGa1-xAs. Journal of Applied Physics. 1986;60:754–767. doi: 10.1063/1.337426. [DOI] [Google Scholar]
- 26.Bååk T. Silicon oxynitride; a material for GRIN optics. Applied Optics. 1982;21:1069. doi: 10.1364/ao.21.001069. [DOI] [PubMed] [Google Scholar]
- 27.Börzsönyi A, Heiner Z, Kalashnikov MP, Kovács AP, Osvay K. Dispersion measurement of inert gases and gas mixtures at 800 nm. Applied Optics. 2008;47:4856. doi: 10.1364/ao.47.004856. [DOI] [PubMed] [Google Scholar]
- 28.Babar S, Weaver JH. Optical constants of cu, ag, and au revisited. Applied Optics. 2015;54:477. doi: 10.1364/ao.54.000477. [DOI] [Google Scholar]
- 29.Ball JM, et al. Optical properties and limiting photocurrent of thin-film perovskite solar cells. Energy & Environmental Science. 2015;8:602–609. doi: 10.1039/c4ee03224a. [DOI] [Google Scholar]
- 30.Barker AS, Ilegems M. Infrared lattice vibrations and free-electron dispersion in GaN. Physical Review B. 1973;7:743–750. doi: 10.1103/physrevb.7.743. [DOI] [Google Scholar]
- 31.Barnes NP, Piltch MS. Temperature-dependent Sellmeier coefficients and nonlinear optics average power limit for germanium. Journal of the Optical Society of America. 1979;69:178. doi: 10.1364/josa.69.000178. [DOI] [Google Scholar]
- 32.Barnes NP, Gettemy DJ. Temperature variation of the refractive indices of yttrium lithium fluoride. Journal of the Optical Society of America. 1980;70:1244. doi: 10.1364/josa.70.001244. [DOI] [Google Scholar]
- 33.Bassarab VV, Shalygin VA, Shakhmin AA, Sokolov VS, Kropotov GI. Spectroscopy of a borosilicate crown glass in the wavelength range of 0.2 μm–15 cm. Journal of Optics. 2023;25:065401. doi: 10.1088/2040-8986/accaf9. [DOI] [Google Scholar]
- 34.Beadie G, Brindza M, Flynn RA, Rosenberg A, Shirk JS. Refractive index measurements of poly(methyl methacrylate) (PMMA) from 0.4—1.6 μm. Applied Optics. 2015;54:F139. doi: 10.1364/ao.54.00f139. [DOI] [PubMed] [Google Scholar]
- 35.Beaini R, Baloukas B, Loquai S, Klemberg-Sapieha J, Martinu L. Thermochromic VO2-based smart radiator devices with ultralow refractive index cavities for increased performance. Solar Energy Materials and Solar Cells. 2020;205:110260. doi: 10.1016/j.solmat.2019.110260. [DOI] [Google Scholar]
- 36.Beal AR, Hughes HP. Kramers-Kronig analysis of the reflectivity spectra of 2H-MoS2, 2H-MoSe2 and 2H-MoTe2. Journal of Physics C: Solid State Physics. 1979;12:881–890. doi: 10.1088/0022-3719/12/5/017. [DOI] [Google Scholar]
- 37.Beliaev, L. Y., Shkondin, E., Lavrinenko, A. V. & Takayama, O. Thickness-dependent optical properties of aluminum nitride films for mid-infrared wavelengths. Journal of Vacuum Science & Technology A: Vacuum, Surfaces, and Films39, 10.1116/6.0000884 (2021).
- 38.Beliaev LY, Shkondin E, Lavrinenko AV, Takayama O. Optical, structural and composition properties of silicon nitride films deposited by reactive radio-frequency sputtering, low pressure and plasma-enhanced chemical vapor deposition. Thin Solid Films. 2022;763:139568. doi: 10.1016/j.tsf.2022.139568. [DOI] [Google Scholar]
- 39.Beliaev, L. Y., Shkondin, E., Lavrinenko, A. V. & Takayama, O. Erratum: “Thickness-dependent optical properties of aluminum nitride films for mid-infrared wavelengths” [J. Vac. Sci. Technol. A 39, 043408 (2021)]. Journal of Vacuum Science & Technology A40, 10.1116/6.0001574 (2022).
- 40.Beliaev LY, Shkondin E, Lavrinenko AV, Takayama O. Optical properties of plasmonic titanium nitride thin films from ultraviolet to mid-infrared wavelengths deposited by pulsed-DC sputtering, thermal and plasma-enhanced atomic layer deposition. Optical Materials. 2023;143:114237. doi: 10.1016/j.optmat.2023.114237. [DOI] [Google Scholar]
- 41.Belosludtsev A, et al. Correlation between stoichiometry and properties of scandium oxide films prepared by reactive magnetron sputtering. Applied Surface Science. 2018;427:312–318. doi: 10.1016/j.apsusc.2017.08.068. [DOI] [Google Scholar]
- 42.Bertie JE, Lan Z, Jones RN, Apelblat Y. Infrared intensities of liquids XVIII: Accurate optical constants and molar absorption coefficients between 6500 and 800 cm−1 of dichloromethane at 25 °C, from spectra recorded in several laboratories. Applied Spectroscopy. 1995;49:840–851. doi: 10.1366/0003702953964435. [DOI] [Google Scholar]
- 43.Bhar GC. Refractive index interpolation in phase-matching. Applied Optics. 1976;15:305. doi: 10.1364/ao.15.0305_1. [DOI] [PubMed] [Google Scholar]
- 44.Bhar GC, Ghosh G. Temperature-dependent Sellmeier coefficients and coherence lengths for some chalcopyrite crystals. Journal of the Optical Society of America. 1979;69:730. doi: 10.1364/josa.69.000730. [DOI] [Google Scholar]
- 45.Bideau-Mehu A, Guern Y, Abjean R, Johannin-Gilles A. Interferometric determination of the refractive index of carbon dioxide in the ultraviolet region. Optics Communications. 1973;9:432–434. doi: 10.1016/0030-4018(73)90289-7. [DOI] [Google Scholar]
- 46.Bideau-Mehu A, Guern Y, Abjean R, Johannin-Gilles A. Measurement of refractive indices of neon, argon, krypton and xenon in the 253.7—140.4 nm wavelength range. dispersion relations and estimated oscillator strengths of the resonance lines. Journal of Quantitative Spectroscopy and Radiative Transfer. 1981;25:395–402. doi: 10.1016/0022-4073(81)90057-1. [DOI] [Google Scholar]
- 47.Bieniewski TM, Czyzak SJ. Refractive indexes of single hexagonal ZnS and CdS crystals. Journal of the Optical Society of America. 1963;53:496. doi: 10.1364/josa.53.000496. [DOI] [Google Scholar]
- 48.Birkhoff RD, Painter LR, Heller JM. Optical and dielectric functions of liquid glycerol from gas photoionization measurements. The Journal of Chemical Physics. 1978;69:4185–4188. doi: 10.1063/1.437098. [DOI] [Google Scholar]
- 49.Bliss ES, Speck DR, Simmons WW. Direct interferometric measurements of the nonlinear refractive index coefficient n2 in laser materials. Applied Physics Letters. 1974;25:728–730. doi: 10.1063/1.1655378. [DOI] [Google Scholar]
- 50.Boidin R, Halenkovič T, Nazabal V, Beneš L, Němec P. Pulsed laser deposited alumina thin films. Ceramics International. 2016;42:1177–1182. doi: 10.1016/j.ceramint.2015.09.048. [DOI] [Google Scholar]
- 51.Bond WL. Measurement of the refractive indices of several crystals. Journal of Applied Physics. 1965;36:1674–1677. doi: 10.1063/1.1703106. [DOI] [Google Scholar]
- 52.Bond WL, Boyd GD, Carter HL. Refractive indices of HgS (cinnabar) between 0.62 and 11 μ. Journal of Applied Physics. 1967;38:4090–4091. doi: 10.1063/1.1709079. [DOI] [Google Scholar]
- 53.Boyd GD, Buehler E, Storz FG. Linear and nonlinear optical properties of ZnGeP2 and CdSe. Applied Physics Letters. 1971;18:301–304. doi: 10.1063/1.1653673. [DOI] [Google Scholar]
- 54.Boyd G, Kasper H, McFee J. Linear and nonlinear optical properties of AgGaS2, CuGaS2, and CuInS2, and theory of the wedge technique for the measurement of nonlinear coefficients. IEEE Journal of Quantum Electronics. 1971;7:563–573. doi: 10.1109/jqe.1971.1076588. [DOI] [Google Scholar]
- 55.Boyd G, Buehler E, Storz F, Wernick J. Linear and nonlinear optical properties of ternary AIIBIVC2V chalcopyrite semiconductors. IEEE Journal of Quantum Electronics. 1972;8:419–426. doi: 10.1109/jqe.1972.1076982. [DOI] [Google Scholar]
- 56.Boyd G, Kasper H, McFee J, Storz F. Linear and nonlinear optical properties of some ternary selenides. IEEE Journal of Quantum Electronics. 1972;8:900–908. doi: 10.1109/jqe.1972.1076900. [DOI] [Google Scholar]
- 57.Brannon JH, Lankard JR, Baise AI, Burns F, Kaufman J. Excimer laser etching of polyimide. Journal of Applied Physics. 1985;58:2036–2043. doi: 10.1063/1.336012. [DOI] [Google Scholar]
- 58.Brasse Y, et al. Magnetic and electric resonances in particle-to-film-coupled functional nanostructures. ACS Applied Materials & Interfaces. 2018;10:3133–3141. doi: 10.1021/acsami.7b16941. [DOI] [PubMed] [Google Scholar]
- 59.Bright T, Watjen J, Zhang Z, Muratore C, Voevodin A. Optical properties of HfO2 thin films deposited by magnetron sputtering: From the visible to the far-infrared. Thin Solid Films. 2012;520:6793–6802. doi: 10.1016/j.tsf.2012.07.037. [DOI] [Google Scholar]
- 60.Bright, T. J. et al. Infrared optical properties of amorphous and nanocrystalline Ta2O5 thin films. Journal of Applied Physics114, 10.1063/1.4819325 (2013).
- 61.Brimhall N, et al. Measured optical constants of copper from 10 nm to 35 nm. Optics Express. 2009;17:23873. doi: 10.1364/oe.17.023873. [DOI] [PubMed] [Google Scholar]
- 62.Bristow, A. D., Rotenberg, N. & van Driel, H. M. Two-photon absorption and kerr coefficients of silicon for 850–2200nm. Applied Physics Letters90, 10.1063/1.2737359 (2007).
- 63.Bucciarelli A, et al. A comparative study of the refractive index of silk protein thin films towards biomaterial based optical devices. Optical Materials. 2018;78:407–414. doi: 10.1016/j.optmat.2018.02.058. [DOI] [Google Scholar]
- 64.Burnett, J. H., Kaplan, S. G., Stover, E. & Phenis, A. Refractive index measurements of Ge. In LeVan, P. D., Sood, A. K., Wijewarnasuriya, P. & D’Souza, A. I. (eds.) Infrared Sensors, Devices, and Applications VI, 10.1117/12.2237978 (SPIE, 2016).
- 65.Caldwell RS, Fan HY. Optical properties of tellurium and selenium. Physical Review. 1959;114:664–675. doi: 10.1103/physrev.114.664. [DOI] [Google Scholar]
- 66.Callcott TA, Arakawa ET. Ultraviolet optical properties of Li. Journal of the Optical Society of America. 1974;64:839. doi: 10.1364/josa.64.000839. [DOI] [Google Scholar]
- 67.Carvajal JJ, et al. Structural and optical properties of RbTiOPO4:Nb crystals. Journal of Physics: Condensed Matter. 2007;19:116214. doi: 10.1088/0953-8984/19/11/116214. [DOI] [Google Scholar]
- 68.Chandler-Horowitz, D. & Amirtharaj, P. M. High-accuracy, midinfrared (450 cm−1 ≤ ω ≤ 4000 cm−1) refractive index values of silicon. Journal of Applied Physics97, 10.1063/1.1923612 (2005).
- 69.Chemnitz, M. et al. Hybrid soliton dynamics in liquid-core fibres. Nature Communications8, 10.1038/s41467-017-00033-5 (2017). [DOI] [PMC free article] [PubMed]
- 70.Chen L, Lynch DW. The optical properties of AuAl2 and PtAl2. physica status solidi (b) 1988;148:387–394. doi: 10.1002/pssb.2221480136. [DOI] [Google Scholar]
- 71.Chen C, et al. New nonlinear-optical crystal: LiB3O5. Journal of the Optical Society of America B. 1989;6:616. doi: 10.1364/josab.6.000616. [DOI] [Google Scholar]
- 72.Chen C-W, et al. Optical properties of organometal halide perovskite thin films and general device structure design rules for perovskite single and tandem solar cells. Journal of Materials Chemistry A. 2015;3:9152–9159. doi: 10.1039/c4ta05237d. [DOI] [Google Scholar]
- 73.Cheng F, et al. Epitaxial growth of atomically smooth aluminum on silicon and its intrinsic optical properties. ACS Nano. 2016;10:9852–9860. doi: 10.1021/acsnano.6b05556. [DOI] [PubMed] [Google Scholar]
- 74.Chengchao W, Xingcan L, Jianyu T, Linhua L. Experimental measurement of optical constant of biodiesel by double optical pathlength transmission method. Laser & Optoelectronics Progress. 2015;52:051206. doi: 10.3788/lop52.051206. [DOI] [Google Scholar]
- 75.Choy MM, Byer RL. Accurate second-order susceptibility measurements of visible and infrared nonlinear crystals. Physical Review B. 1976;14:1693–1706. doi: 10.1103/physrevb.14.1693. [DOI] [Google Scholar]
- 76.Ciddor PE. Refractive index of air: new equations for the visible and near infrared. Applied Optics. 1996;35:1566. doi: 10.1364/ao.35.001566. [DOI] [PubMed] [Google Scholar]
- 77.Ciesielski A, Skowronski L, Trzcinski M, Szoplik T. Controlling the optical parameters of self-assembled silver films with wetting layers and annealing. Applied Surface Science. 2017;421:349–356. doi: 10.1016/j.apsusc.2017.01.039. [DOI] [Google Scholar]
- 78.Ciesielski A, Skowronski L, Pacuski W, Szoplik T. Permittivity of ge, te and se thin films in the 200–1500 nm spectral range. predicting the segregation effects in silver. Materials Science in Semiconductor Processing. 2018;81:64–67. doi: 10.1016/j.mssp.2018.03.003. [DOI] [Google Scholar]
- 79.Ciesielski A, et al. Evidence of germanium segregation in gold thin films. Surface Science. 2018;674:73–78. doi: 10.1016/j.susc.2018.03.020. [DOI] [Google Scholar]
- 80.Connolly, J., diBenedetto, B. & Donadio, R. Specifications of raytran material. In Fischer, R. E. (ed.) Contemporary Optical Systems and Components Specifications, 10.1117/12.957359 (SPIE, 1979).
- 81.Coulter JK, Hass G, Ramsey JB. Optical constants and reflectance and transmittance of evaporated rhodium films in the visible. Journal of the Optical Society of America. 1973;63:1149. doi: 10.1364/josa.63.001149. [DOI] [Google Scholar]
- 82.Cunningham, P. D. et al. Broadband terahertz characterization of the refractive index and absorption of some important polymeric and organic electro-optic materials. Journal of Applied Physics109, 043505–043505–5, 10.1063/1.3549120 (2011).
- 83.Cuthbertson C, Cuthbertson M. On the refraction and dispersion of neon. Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character. 1910;83:149–151. doi: 10.1098/rspa.1910.0001. [DOI] [Google Scholar]
- 84.Cuthbertson C, Cuthbertson M. The refraction and dispersion of argon, and redeterminations of the dispersion of helium, neon, krypton, and xenon. Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character. 1910;84:13–15. doi: 10.1098/rspa.1910.0052. [DOI] [Google Scholar]
- 85.Cuthbertson C, Cuthbertson M. On the refraction and dispersion of the halogens, halogen acids, ozone, steam, oxides of nitrogen and ammonia. Philosophical Transactions of the Royal Society of London. Series A, Containing Papers of a Mathematical or Physical Character. 1914;213:1–26. doi: 10.1098/rsta.1914.0001. [DOI] [Google Scholar]
- 86.Cuthbertson C, Cuthbertson M. The refraction and dispersion of neon and helium. Proceedings of the Royal Society of London. Series A, Containing Papers of a Mathematical and Physical Character. 1932;135:40–47. doi: 10.1098/rspa.1932.0019. [DOI] [Google Scholar]
- 87.Daimon M, Masumura A. High-accuracy measurements of the refractive index and its temperature coefficient of calcium fluoride in a wide wavelength range from 138 to 2326 nm. Applied Optics. 2002;41:5275. doi: 10.1364/ao.41.005275. [DOI] [PubMed] [Google Scholar]
- 88.Daimon M, Masumura A. Measurement of the refractive index of distilled water from the near-infrared region to the ultraviolet region. Applied Optics. 2007;46:3811. doi: 10.1364/ao.46.003811. [DOI] [PubMed] [Google Scholar]
- 89.Dalzell WH, Sarofim AF. Optical constants of soot and their application to heat-flux calculations. Journal of Heat Transfer. 1969;91:100–104. doi: 10.1115/1.3580063. [DOI] [Google Scholar]
- 90.Das S, Bhar GC, Gangopadhyay S, Ghosh C. Linear and nonlinear optical properties of ZnGeP2 crystal for infrared laser device applications: revisited. Applied Optics. 2003;42:4335. doi: 10.1364/ao.42.004335. [DOI] [PubMed] [Google Scholar]
- 91.David M, et al. Structure and mid-infrared optical properties of spin-coated polyethylene films developed for integrated photonics applications. Optical Materials Express. 2022;12:2168. doi: 10.1364/ome.458667. [DOI] [Google Scholar]
- 92.DeBell AG, et al. Cryogenic refractive indices and temperature coefficients of cadmium telluride from 6 μm to 22 μm. Applied Optics. 1979;18:3114. doi: 10.1364/ao.18.003114. [DOI] [PubMed] [Google Scholar]
- 93.DeSalvo R, Said A, Hagan D, Van Stryland E, Sheik-Bahae M. Infrared to ultraviolet measurements of two-photon absorption and n2 in wide bandgap solids. IEEE Journal of Quantum Electronics. 1996;32:1324–1333. doi: 10.1109/3.511545. [DOI] [Google Scholar]
- 94.DeVore JR. Refractive indices of rutile and sphalerite. Journal of the Optical Society of America. 1951;41:416. doi: 10.1364/josa.41.000416. [DOI] [Google Scholar]
- 95.Debenham M. Refractive indices of zinc sulfide in the 0.405–13-μm wavelength range. Applied Optics. 1984;23:2238. doi: 10.1364/ao.23.002238. [DOI] [PubMed] [Google Scholar]
- 96.Djurišić AB, Li EH. Optical properties of graphite. Journal of Applied Physics. 1999;85:7404–7410. doi: 10.1063/1.369370. [DOI] [Google Scholar]
- 97.Djurišić A, Li E, Rakić D, Majewski M. Modeling the optical properties of AlSb, GaSb, and InSb. Applied Physics A: Materials Science & Processing. 2000;70:29–32. doi: 10.1007/s003390050006. [DOI] [Google Scholar]
- 98.Dodge MJ. Refractive properties of magnesium fluoride. Applied Optics. 1984;23:1980. doi: 10.1364/ao.23.001980. [DOI] [PubMed] [Google Scholar]
- 99.Dore P, et al. Infrared properties of chemical-vapor deposition polycrystalline diamond windows. Applied Optics. 1998;37:5731. doi: 10.1364/ao.37.005731. [DOI] [PubMed] [Google Scholar]
- 100.Edwards DF, Ochoa E. Infrared refractive index of silicon. Applied Optics. 1980;19:4130. doi: 10.1364/ao.19.004130. [DOI] [PubMed] [Google Scholar]
- 101.Eimerl D, Davis L, Velsko S, Graham EK, Zalkin A. Optical, mechanical, and thermal properties of barium borate. Journal of Applied Physics. 1987;62:1968–1983. doi: 10.1063/1.339536. [DOI] [Google Scholar]
- 102.El-Kashef H. The necessary requirements imposed on polar dielectric laser dye solvents. Physica B: Condensed Matter. 2000;279:295–301. doi: 10.1016/s0921-4526(99)00856-x. [DOI] [Google Scholar]
- 103.Ensley TR, Bambha NK. Ultrafast nonlinear refraction measurements of infrared transmitting materials in the mid-wave infrared. Optics Express. 2019;27:37940. doi: 10.1364/oe.380702. [DOI] [PubMed] [Google Scholar]
- 104.Ermolaev, G. A., Yakubovsky, D. I., Stebunov, Y. V., Arsenin, A. V. & Volkov, V. S. Spectral ellipsometry of monolayer transition metal dichalcogenides: Analysis of excitonic peaks in dispersion. Journal of Vacuum Science & Technology B, Nanotechnology and Microelectronics: Materials, Processing, Measurement, and Phenomena38, 10.1116/1.5122683 (2019).
- 105.Ermolaev, G. A. et al. Broadband optical properties of monolayer and bulk MoS2. npj 2D Materials and Applications4, 10.1038/s41699-020-0155-x (2020).
- 106.Ermolaev, G. A. et al. Express determination of thickness and dielectric function of single-walled carbon nanotube films. Applied Physics Letters116, 10.1063/5.0012933 (2020).
- 107.Ermolaev GA, et al. Broadband optical properties of atomically thin PtS2 and PtSe2. Nanomaterials. 2021;11:3269. doi: 10.3390/nano11123269. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 108.Ermolaev GA, et al. Broadband optical constants and nonlinear properties of SnS2 and SnSe2. Nanomaterials. 2021;12:141. doi: 10.3390/nano12010141. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 109.Ermolaev, G. A. et al. Giant optical anisotropy in transition metal dichalcogenides for next-generation photonics. Nature Communications12, 10.1038/s41467-021-21139-x (2021). [DOI] [PMC free article] [PubMed]
- 110.Ermolaev, G. A. et al. Spectroscopic ellipsometry of large area monolayer WS2 and WSe2 films. In AIP Conference Proceedings, 10.1063/5.0054947 (AIP Publishing, 2021).
- 111.Ermolaev, G. et al. Topological phase singularities in atomically thin high-refractive-index materials. Nature Communications13, 10.1038/s41467-022-29716-4 (2022). [DOI] [PMC free article] [PubMed]
- 112.Ermolaev G, et al. Giant and tunable excitonic optical anisotropy in single-crystal halide perovskites. Nano Letters. 2023;23:2570–2577. doi: 10.1021/acs.nanolett.2c04792. [DOI] [PubMed] [Google Scholar]
- 113.Ermolaev, G. A. et al. Anisotropic optical properties of monolayer aligned single-walled carbon nanotubes. physica status solidi (RRL) – Rapid Research Letters 2300199, 10.1002/pssr.202300199 (2023).
- 114.Ermolov, A., Mak, K. F., Frosz, M. H., Travers, J. C. & Russell, P. S. J. Supercontinuum generation in the vacuum ultraviolet through dispersive-wave and soliton-plasma interaction in a noble-gas-filled hollow-core photonic crystal fiber. Physical Review A92, 10.1103/physreva.92.033821 (2015).
- 115.Ewbank MD, et al. The temperature dependence of optical and mechanical properties of Tl3AsSe3. Journal of Applied Physics. 1980;51:3848–3852. doi: 10.1063/1.328128. [DOI] [Google Scholar]
- 116.Fang S, Liu H, Huang L, Ye N. Growth and optical properties of nonlinear LuAl3(BO3)4 crystals. Optics Express. 2013;21:16415. doi: 10.1364/oe.21.016415. [DOI] [PubMed] [Google Scholar]
- 117.Fang M, et al. Layer-dependent dielectric permittivity of topological insulator Bi2Se3 thin films. Applied Surface Science. 2020;509:144822. doi: 10.1016/j.apsusc.2019.144822. [DOI] [Google Scholar]
- 118.Feldman A, Horowitz D. Refractive index of cuprous chloride*. Journal of the Optical Society of America. 1969;59:1406. doi: 10.1364/josa.59.001406. [DOI] [Google Scholar]
- 119.Fern RE, Onton A. Refractive index of AlAs. Journal of Applied Physics. 1971;42:3499–3500. doi: 10.1063/1.1660760. [DOI] [Google Scholar]
- 120.Fernández-Perea M, et al. Determination of optical constants of scandium films in the 20–1000 eV range. Journal of the Optical Society of America A. 2006;23:2880. doi: 10.1364/josaa.23.002880. [DOI] [PubMed] [Google Scholar]
- 121.Fernández-Perea M, et al. Optical constants of electron-beam evaporated boron films in the 6.8–900 eV photon energy range. Journal of the Optical Society of America A. 2007;24:3800. doi: 10.1364/josaa.24.003800. [DOI] [PubMed] [Google Scholar]
- 122.Fernández-Perea M, et al. Optical constants of Yb films in the 23–1700 eV range. Journal of the Optical Society of America A. 2007;24:3691. doi: 10.1364/josaa.24.003691. [DOI] [PubMed] [Google Scholar]
- 123.Fernández-Perea, M. et al. Transmittance and optical constants of Pr films in the 4–1600ev spectral range. Journal of Applied Physics103, 10.1063/1.2939269 (2008).
- 124.Fernández-Perea, M. et al. Transmittance and optical constants of Eu films from 8.3 to 1400 eV. Journal of Applied Physics104, 10.1063/1.2982391 (2008).
- 125.Fernández-Perea, M. et al. Transmittance and optical constants of Ce films in the 6–1200ev spectral range. Journal of Applied Physics103, 10.1063/1.2901137 (2008).
- 126.Fernández-Perea, M. et al. Optical constants of evaporation-deposited silicon monoxide films in the 7.1–800 eV photon energy range. Journal of Applied Physics105, 10.1063/1.3123768 (2009).
- 127.Fernández-Perea, M. et al. Transmittance and optical constants of Ho films in the 3–1340 eV spectral range. Journal of Applied Physics109, 10.1063/1.3556451 (2011). [DOI] [PubMed]
- 128.Ferrera, M., Magnozzi, M., Bisio, F. & Canepa, M. Temperature-dependent permittivity of silver and implications for thermoplasmonics. Physical Review Materials3, 10.1103/physrevmaterials.3.105201 (2019).
- 129.Ferrini R, Patrini M, Franchi S. Optical functions from 0.02 to 6 eV of AlxGa1-xSb/GaSb epitaxial layers. Journal of Applied Physics. 1998;84:4517–4524. doi: 10.1063/1.368677. [DOI] [Google Scholar]
- 130.Fischer MP, et al. Coherent field transients below 15 THz from phase-matched difference frequency generation in 4H-SiC. Optics Letters. 2017;42:2687. doi: 10.1364/ol.42.002687. [DOI] [PubMed] [Google Scholar]
- 131.Fleming JW. Dispersion in GeO2-SiO2 glasses. Applied Optics. 1984;23:4486. doi: 10.1364/ao.23.004486. [DOI] [PubMed] [Google Scholar]
- 132.Flom SR, Beadie G, Bayya SS, Shaw B, Auxier JM. Ultrafast z-scan measurements of nonlinear optical constants of window materials at 772, 1030, and 1550 nm. Applied Optics. 2015;54:F123. doi: 10.1364/ao.54.00f123. [DOI] [PubMed] [Google Scholar]
- 133.Franta D, Nečas D, Ohlídal I. Universal dispersion model for characterization of optical thin films over a wide spectral range: application to hafnia. Applied Optics. 2015;54:9108. doi: 10.1364/ao.54.009108. [DOI] [PubMed] [Google Scholar]
- 134.French, R. H. et al. Optical properties of materials for concentrator photovoltaic systems. In 2009 34th IEEE Photovoltaic Specialists Conference (PVSC), 10.1109/pvsc.2009.5411657 (IEEE, 2009).
- 135.Frisenda R, et al. Characterization of highly crystalline lead iodide nanosheets prepared by room-temperature solution processing. Nanotechnology. 2017;28:455703. doi: 10.1088/1361-6528/aa8e5c. [DOI] [PubMed] [Google Scholar]
- 136.Fujii Y, Sakudo T. Dielectric and optical properties of KTaO3. Journal of the Physical Society of Japan. 1976;41:888–893. doi: 10.1143/jpsj.41.888. [DOI] [Google Scholar]
- 137.Gan F. Optical properties of fluoride glasses: a review. Journal of Non-Crystalline Solids. 1995;184:9–20. doi: 10.1016/0022-3093(94)00592-3. [DOI] [Google Scholar]
- 138.Gant P, et al. Optical contrast and refractive index of natural van der waals heterostructure nanosheets of franckeite. Beilstein Journal of Nanotechnology. 2017;8:2357–2362. doi: 10.3762/bjnano.8.235. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 139.Gao L, Lemarchand F, Lequime M. Comparison of different dispersion models for single layer optical thin film index determination. Thin Solid Films. 2011;520:501–509. doi: 10.1016/j.tsf.2011.07.028. [DOI] [Google Scholar]
- 140.Gao L, Lemarchand F, Lequime M. Exploitation of multiple incidences spectrometric measurements for thin film reverse engineering. Optics Express. 2012;20:15734. doi: 10.1364/oe.20.015734. [DOI] [PubMed] [Google Scholar]
- 141.Gao, L., Lemarchand, F. & Lequime, M. Refractive index determination of SiO2 layer in the UV/Vis/NIR range: spectrophotometric reverse engineering on single and bi-layer designs. Journal of the European Optical Society: Rapid Publications8, 10.2971/jeos.2013.13010 (2013).
- 142.García-Cortés, S. et al. Transmittance and optical constants of Lu films in the 3–1800 eV spectral range. Journal of Applied Physics108, 10.1063/1.3481062 (2010).
- 143.Geints, Y. E. et al. Kerr-driven nonlinear refractive index of air at 800 and 400 nm measured through femtosecond laser pulse filamentation. Applied Physics Letters99, 10.1063/1.3657774 (2011).
- 144.Ghosal S, Ebert JL, Self SA. The infrared refractive indices of CHBr3, CCl4 and CS2. Infrared Physics. 1993;34:621–628. doi: 10.1016/0020-0891(93)90120-v. [DOI] [Google Scholar]
- 145.Ghosh G. Dispersion-equation coefficients for the refractive index and birefringence of calcite and quartz crystals. Optics Communications. 1999;163:95–102. doi: 10.1016/s0030-4018(99)00091-7. [DOI] [Google Scholar]
- 146.Giannios P, et al. Complex refractive index of normal and malignant human colorectal tissue in the visible and near-infrared. Journal of Biophotonics. 2016;10:303–310. doi: 10.1002/jbio.201600001. [DOI] [PubMed] [Google Scholar]
- 147.Giannios, P. et al. Visible to near-infrared refractive properties of freshly-excised human-liver tissues: marking hepatic malignancies. Scientific Reports6, 10.1038/srep27910 (2016). [DOI] [PMC free article] [PubMed]
- 148.Gomez MS, Guerra JM, Vilches F. Weighted nonlinear regression analysis of a Sellmeier expansion: comparison of several nonlinear fits of CdS dispersion. Applied Optics. 1985;24:1147. doi: 10.1364/ao.24.001147. [DOI] [PubMed] [Google Scholar]
- 149.Grace E, Butcher A, Monroe J, Nikkel JA. Index of refraction, Rayleigh scattering length, and Sellmeier coefficients in solid and liquid argon and xenon. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment. 2017;867:204–208. doi: 10.1016/j.nima.2017.06.031. [DOI] [Google Scholar]
- 150.Green MA, Keevers MJ. Optical properties of intrinsic silicon at 300 K. Progress in Photovoltaics: Research and Applications. 1995;3:189–192. doi: 10.1002/pip.4670030303. [DOI] [Google Scholar]
- 151.Green MA. Self-consistent optical parameters of intrinsic silicon at 300 K including temperature coefficients. Solar Energy Materials and Solar Cells. 2008;92:1305–1310. doi: 10.1016/j.solmat.2008.06.009. [DOI] [Google Scholar]
- 152.Griesmann U, Burnett JH. Refractivity of nitrogen gas in the vacuum ultraviolet. Optics Letters. 1999;24:1699. doi: 10.1364/ol.24.001699. [DOI] [PubMed] [Google Scholar]
- 153.Grudinin DV, et al. Hexagonal boron nitride nanophotonics: a record-breaking material for the ultraviolet and visible spectral ranges. Materials Horizons. 2023;10:2427–2435. doi: 10.1039/d3mh00215b. [DOI] [PubMed] [Google Scholar]
- 154.Gu H, et al. Layer-dependent dielectric and optical properties of centimeter-scale 2D WSe2: evolution from a single layer to few layers. Nanoscale. 2019;11:22762–22771. doi: 10.1039/c9nr04270a. [DOI] [PubMed] [Google Scholar]
- 155.Guo Z, et al. Complete dielectric tensor and giant optical anisotropy in quasi-one-dimensional ZrTe5. ACS Materials Letters. 2021;3:525–534. doi: 10.1021/acsmaterialslett.1c00026. [DOI] [Google Scholar]
- 156.Guo Z, Gu H, Fang M, Ye L, Liu S. Giant in-plane optical and electronic anisotropy of tellurene: a quantitative exploration. Nanoscale. 2022;14:12238–12246. doi: 10.1039/d2nr03226k. [DOI] [PubMed] [Google Scholar]
- 157.Guo Z, Gu H, Yu Y, Wei Z, Liu S. Broadband and incident-angle-modulation near-infrared polarizers based on optically anisotropic SnSe. Nanomaterials. 2022;13:134. doi: 10.3390/nano13010134. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 158.Gupta V, et al. Mechanotunable surface lattice resonances in the visible optical range by soft lithography templates and directed self-assembly. ACS Applied Materials & Interfaces. 2019;11:28189–28196. doi: 10.1021/acsami.9b08871. [DOI] [PubMed] [Google Scholar]
- 159.Gupta, V. et al. Advanced colloidal sensors enabled by an out-of-plane lattice resonance. Advanced Photonics Research3, 10.1002/adpr.202200152 (2022).
- 160.Hagemann H-J, Gudat W, Kunz C. Optical constants from the far infrared to the x-ray region: Mg, Al, Cu, Ag, Au, Bi, C, and Al2O3. Journal of the Optical Society of America. 1975;65:742. doi: 10.1364/josa.65.000742. [DOI] [Google Scholar]
- 161.Hale GM, Querry MR. Optical constants of water in the 200-nm to 200-μm wavelength region. Applied Optics. 1973;12:555. doi: 10.1364/ao.12.000555. [DOI] [PubMed] [Google Scholar]
- 162.Hanson F, Dick D. Blue parametric generation from temperature-tuned LiB3O5. Optics Letters. 1991;16:205. doi: 10.1364/ol.16.000205. [DOI] [PubMed] [Google Scholar]
- 163.Hartnett T, Bernstein S, Maguire E, Tustison R. Optical properties of ALON (aluminum oxynitride) Infrared Physics & Technology. 1998;39:203–211. doi: 10.1016/s1350-4495(98)00007-3. [DOI] [Google Scholar]
- 164.Hass G, Salzberg CD. Optical properties of silicon monoxide in the wavelength region from 0.24 to 14.0 microns. Journal of the Optical Society of America. 1954;44:181. doi: 10.1364/josa.44.000181. [DOI] [Google Scholar]
- 165.Hass G, Jacobus GF, Hunter WR. Optical properties of evaporated iridium in the vacuum ultraviolet from 500 Å to 2000 Å. Journal of the Optical Society of America. 1967;57:758. doi: 10.1364/josa.57.000758. [DOI] [Google Scholar]
- 166.Heitmann W, Ritter E. Production and properties of vacuum evaporated films of thorium fluoride. Applied Optics. 1968;7:307. doi: 10.1364/ao.7.000307. [DOI] [PubMed] [Google Scholar]
- 167.Herguedas N, Carretero E. Optical properties in mid-infrared range of silicon oxide thin films with different stoichiometries. Nanomaterials. 2023;13:2749. doi: 10.3390/nano13202749. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 168.Horcholle B, et al. Growth and study of Tb3+ doped Nb2O5 thin films by radiofrequency magnetron sputtering: Photoluminescence properties. Applied Surface Science. 2022;597:153711. doi: 10.1016/j.apsusc.2022.153711. [DOI] [Google Scholar]
- 169.Hrabovský J, Kučera M, Paloušová L, Bi L, Veis M. Optical characterization of Y3Al5O12 and Lu3Al5O12 single crystals. Optical Materials Express. 2021;11:1218. doi: 10.1364/ome.417670. [DOI] [Google Scholar]
- 170.Hsu, C. et al. Thickness-dependent refractive index of 1L, 2L, and 3L MoS2, MoSe2, WS2, and WSe2. Advanced Optical Materials7, 10.1002/adom.201900239 (2019).
- 171.Hulme KF, Jones O, Davies PH, Hobden MV. Synthetic proustite (Ag3AsS3): A new crystal for optical mixing. Applied Physics Letters. 1967;10:133–135. doi: 10.1063/1.1754880. [DOI] [Google Scholar]
- 172.Hurlbut WC, Lee Y-S, Vodopyanov KL, Kuo PS, Fejer MM. Multiphoton absorption and nonlinear refraction of GaAs in the mid-infrared. Optics Letters. 2007;32:668. doi: 10.1364/ol.32.000668. [DOI] [PubMed] [Google Scholar]
- 173.Iezzi, B. et al. Electrohydrodynamic jet printing of 1D photonic crystals: Part II–optical design and reflectance characteristics. Advanced Materials Technologies5, 10.1002/admt.202000431 (2020).
- 174.Inagaki T, Hamm RN, Arakawa ET, Painter LR. Optical and dielectric properties of DNA in the extreme ultraviolet. The Journal of Chemical Physics. 1974;61:4246–4250. doi: 10.1063/1.1681724. [DOI] [Google Scholar]
- 175.Inagaki T, Arakawa ET, Birkhoff RD, Williams MW. Optical properties of liquid Na between 0.6 and 3.8 eV. Physical Review B. 1976;13:5610–5612. doi: 10.1103/physrevb.13.5610. [DOI] [Google Scholar]
- 176.Inagaki T, Emerson LC, Arakawa ET, Williams MW. Optical properties of solid Na and Li between 0.6 and 3.8 eV. Physical Review B. 1976;13:2305–2313. doi: 10.1103/physrevb.13.2305. [DOI] [Google Scholar]
- 177.Inagaki T, Arakawa ET, Williams MW. Optical properties of liquid mercury. Physical Review B. 1981;23:5246–5262. doi: 10.1103/physrevb.23.5246. [DOI] [Google Scholar]
- 178.Ishteev A, et al. Investigation of structural and optical properties of MAPbBr3 monocrystals under fast electron irradiation. Journal of Materials Chemistry C. 2022;10:5821–5828. doi: 10.1039/d2tc00128d. [DOI] [Google Scholar]
- 179.Islam, K. M. et al. In-plane and out-of-plane optical properties of monolayer, few-layer, and thin-film MoS2 from 190 to 1700 nm and their application in photonic device design. Advanced Photonics Research2, 10.1002/adpr.202000180 (2021).
- 180.Ives HE, Briggs HB. The optical constants of potassium. Journal of the Optical Society of America. 1936;26:238. doi: 10.1364/josa.26.000238. [DOI] [Google Scholar]
- 181.Ives HE, Briggs HB. Optical constants of rubidium and cesium. Journal of the Optical Society of America. 1937;27:395. doi: 10.1364/josa.27.000395. [DOI] [Google Scholar]
- 182.Ives HE, Briggs HB. The optical constants of sodium. Journal of the Optical Society of America. 1937;27:181. doi: 10.1364/josa.27.000181. [DOI] [Google Scholar]
- 183.Jansonas G, Budriūnas R, Vengris M, Varanavičius A. Interferometric measurements of nonlinear refractive index in the infrared spectral range. Optics Express. 2022;30:30507. doi: 10.1364/oe.458850. [DOI] [PubMed] [Google Scholar]
- 184.Jöbsis, H. J. et al. Recombination and localization: Unfolding the pathways behind conductivity losses in Cs2AgBiBr6 thin films. Applied Physics Letters119, 10.1063/5.0061899 (2021).
- 185.Jellison G. Optical functions of silicon determined by two-channel polarization modulation ellipsometry. Optical Materials. 1992;1:41–47. doi: 10.1016/0925-3467(92)90015-f. [DOI] [Google Scholar]
- 186.Jellison G. Optical functions of GaAs, GaP, and Ge determined by two-channel polarization modulation ellipsometry. Optical Materials. 1992;1:151–160. doi: 10.1016/0925-3467(92)90022-f. [DOI] [Google Scholar]
- 187.Jellison G, Haynes T, Burke H. Optical functions of silicon-germanium alloys determined using spectroscopic ellipsometry. Optical Materials. 1993;2:105–117. doi: 10.1016/0925-3467(93)90035-y. [DOI] [Google Scholar]
- 188.Jellison, G. E. et al. Refractive index of sodium iodide. Journal of Applied Physics111, 10.1063/1.3689746 (2012).
- 189.Jiang, Y., Pillai, S. & Green, M. A. Realistic silver optical constants for plasmonics. Scientific Reports6, 10.1038/srep30605 (2016). [DOI] [PMC free article] [PubMed]
- 190.Johnson PB, Christy RW. Optical constants of the noble metals. Physical Review B. 1972;6:4370–4379. doi: 10.1103/physrevb.6.4370. [DOI] [Google Scholar]
- 191.Johnson P, Christy R. Optical constants of transition metals: Ti, V, Cr, Mn, Fe, Co, Ni, and Pd. Physical Review B. 1974;9:5056–5070. doi: 10.1103/physrevb.9.5056. [DOI] [Google Scholar]
- 192.Joseph S, Sarkar S, Joseph J. Grating-coupled surface plasmon-polariton sensing at a flat metal–analyte interface in a hybrid-configuration. ACS Applied Materials & Interfaces. 2020;12:46519–46529. doi: 10.1021/acsami.0c12525. [DOI] [PubMed] [Google Scholar]
- 193.Joseph, S., Sarkar, S., Khan, S. & Joseph, J. Exploring the optical bound state in the continuum in a dielectric grating coupled plasmonic hybrid system. Advanced Optical Materials9, 10.1002/adom.202001895 (2021).
- 194.Jung G-H, Yoo S, Park Q-H. Measuring the optical permittivity of two-dimensional materials without a priori knowledge of electronic transitions. Nanophotonics. 2018;8:263–270. doi: 10.1515/nanoph-2018-0120. [DOI] [Google Scholar]
- 195.Křen P. Comment on “Precision refractive index measurements of air, N2, O2, Ar, and CO2 with a frequency comb”. Applied Optics. 2011;50:6484. doi: 10.1364/ao.50.006484. [DOI] [PubMed] [Google Scholar]
- 196.König TAF, et al. Electrically tunable plasmonic behavior of nanocube–polymer nanomaterials induced by a redox-active electrochromic polymer. ACS Nano. 2014;8:6182–6192. doi: 10.1021/nn501601e. [DOI] [PubMed] [Google Scholar]
- 197.Kabaciński P, Kardaś TM, Stepanenko Y, Radzewicz C. Nonlinear refractive index measurement by SPM-induced phase regression. Optics Express. 2019;27:11018. doi: 10.1364/oe.27.011018. [DOI] [PubMed] [Google Scholar]
- 198.Kabelka VI, Piskarskas AS, Stabinis AY, Sher RL. Group matching of interacting light pulses in nonlinear crystals. Soviet Journal of Quantum Electronics. 1975;5:255–256. doi: 10.1070/qe1975v005n02abeh010943. [DOI] [Google Scholar]
- 199.Kachare AH, Spitzer WG, Fredrickson JE. Refractive index of ion-implanted GaAs. Journal of Applied Physics. 1976;47:4209–4212. doi: 10.1063/1.323292. [DOI] [Google Scholar]
- 200.Kaiser W, Spitzer WG, Kaiser RH, Howarth LE. Infrared properties of CaF2, SrF2, and BaF2. Physical Review. 1962;127:1950–1954. doi: 10.1103/physrev.127.1950. [DOI] [Google Scholar]
- 201.Kaminskii AA, et al. Mechanical and optical properties of Lu2O3 host-ceramics for Ln3+ lasants. Laser Physics Letters. 2007;5:300–303. doi: 10.1002/lapl.200710128. [DOI] [Google Scholar]
- 202.Kato K. High-power difference-frequency generation at 4.4–5.7 μm in LiIO3. IEEE Journal of Quantum Electronics. 1985;21:119–120. doi: 10.1109/jqe.1985.1072617. [DOI] [Google Scholar]
- 203.Kato K, Takaoka E. Sellmeier and thermo-optic dispersion formulas for KTP. Applied Optics. 2002;41:5040. doi: 10.1364/ao.41.005040. [DOI] [PubMed] [Google Scholar]
- 204.Kato K, Umemura N. Sellmeier equations for GaS and GaSe and their applications to the nonlinear optics in GaSxSe1-x. Optics Letters. 2011;36:746. doi: 10.1364/ol.36.000746. [DOI] [PubMed] [Google Scholar]
- 205.Kato K, Tanno F, Umemura N. Sellmeier and thermo-optic dispersion formulas for GaSe (revisited) Applied Optics. 2013;52:2325. doi: 10.1364/ao.52.002325. [DOI] [PubMed] [Google Scholar]
- 206.Kato K, Petrov V, Umemura N. Phase-matching properties of yellow color HgGa2S4 for shg and sfg in the 0.944–10.5910 μm range. Applied Optics. 2016;55:3145. doi: 10.1364/ao.55.003145. [DOI] [PubMed] [Google Scholar]
- 207.Kato K, Umemura N, Petrov V. Sellmeier and thermo-optic dispersion formulas for CdGa2S4 and their application to the nonlinear optics of Hg1-xCdxGa2S4. Optics Communications. 2017;386:49–52. doi: 10.1016/j.optcom.2016.10.054. [DOI] [Google Scholar]
- 208.Kato K, et al. Phase-matching properties of LiGaS2 in the 1.025–10.5910 μm spectral range. Optics Letters. 2017;42:4363. doi: 10.1364/ol.42.004363. [DOI] [PubMed] [Google Scholar]
- 209.Kato K, Miyata K, Badikov VV, Petrov V. Phase-matching properties of BaGa2GeSe6 for three-wave interactions in the 0.778–10.5910 μm spectral range. Applied Optics. 2018;57:7440. doi: 10.1364/ao.57.007440. [DOI] [PubMed] [Google Scholar]
- 210.Kato K, Badikov VV, Miyata K, Petrov V. Refined Sellmeier equations for BaGa4S7. Applied Optics. 2021;60:6600. doi: 10.1364/ao.430424. [DOI] [PubMed] [Google Scholar]
- 211.Kato K, Miyata K, Petrov V. Refined Sellmeier equations for AgGaSe2 up to 18 μm. Applied Optics. 2021;60:805. doi: 10.1364/ao.401828. [DOI] [PubMed] [Google Scholar]
- 212.Kato K, Banerjee S, Umemura N. Phase-matching properties of AgGa0.86In0.14S2 for three-wave interactions in the 0.615–10.5910 μm spectral range. Optical Materials Express. 2021;11:2800. doi: 10.1364/ome.428688. [DOI] [Google Scholar]
- 213.Kawashima T, Yoshikawa H, Adachi S, Fuke S, Ohtsuka K. Optical properties of hexagonal GaN. Journal of Applied Physics. 1997;82:3528–3535. doi: 10.1063/1.365671. [DOI] [Google Scholar]
- 214.Kawka PA, Buckius RO. Optical properties of polyimide films in the infrared. International Journal of Thermophysics. 2001;22:517–534. doi: 10.1023/a:1010797620483. [DOI] [Google Scholar]
- 215.Kedenburg S, Vieweg M, Gissibl T, Giessen H. Linear refractive index and absorption measurements of nonlinear optical liquids in the visible and near-infrared spectral region. Optical Materials Express. 2012;2:1588. doi: 10.1364/ome.2.001588. [DOI] [Google Scholar]
- 216.Kerl K, Varchmin H. Refractive index dispersion (RID) of some liquids in the UV/VIS between 20 °C and 60 °C. Journal of Molecular Structure. 1995;349:257–260. doi: 10.1016/0022-2860(95)08758-n. [DOI] [Google Scholar]
- 217.Kerremans, R. et al. The optical constants of solution-processed semiconductors—new challenges with perovskites and non-fullerene acceptors. Advanced Optical Materials8, 10.1002/adom.202000319 (2020).
- 218.Kim YD, et al. Optical properties of zinc-blende CdSe and ZnxCd1-xSe films grown on GaAs. Physical Review B. 1994;49:7262–7270. doi: 10.1103/physrevb.49.7262. [DOI] [PubMed] [Google Scholar]
- 219.Kischkat J, et al. Mid-infrared optical properties of thin films of aluminum oxide, titanium dioxide, silicon dioxide, aluminum nitride, and silicon nitride. Applied Optics. 2012;51:6789. doi: 10.1364/ao.51.006789. [DOI] [PubMed] [Google Scholar]
- 220.Kitamura R, Pilon L, Jonasz M. Optical constants of silica glass from extreme ultraviolet to far infrared at near room temperature. Applied Optics. 2007;46:8118. doi: 10.1364/ao.46.008118. [DOI] [PubMed] [Google Scholar]
- 221.Kiyoshi Kato KK, Hiromichi Shirahata HS. Nonlinear ir generation in AgGaS2. Japanese Journal of Applied Physics. 1996;35:4645. doi: 10.1143/jjap.35.4645. [DOI] [Google Scholar]
- 222.Klein CA. Room-temperature dispersion equations for cubic zinc sulfide. Applied Optics. 1986;25:1873. doi: 10.1364/ao.25.001873. [DOI] [PubMed] [Google Scholar]
- 223.Kofman V, He J, Loes ten Kate I, Linnartz H. The refractive index of amorphous and crystalline water ice in the UV–vis. The Astrophysical Journal. 2019;875:131. doi: 10.3847/1538-4357/ab0d89. [DOI] [Google Scholar]
- 224.Kozma IZ, Krok P, Riedle E. Direct measurement of the group-velocity mismatch and derivation of the refractive-index dispersion for a variety of solvents in the ultraviolet. Journal of the Optical Society of America B. 2005;22:1479. doi: 10.1364/josab.22.001479. [DOI] [Google Scholar]
- 225.Krauter P, et al. Optical phantoms with adjustable subdiffusive scattering parameters. Journal of Biomedical Optics. 2015;20:105008. doi: 10.1117/1.jbo.20.10.105008. [DOI] [PubMed] [Google Scholar]
- 226.Kulikova DP, et al. Optical properties of tungsten trioxide, palladium, and platinum thin films for functional nanostructures engineering. Optics Express. 2020;28:32049. doi: 10.1364/oe.405403. [DOI] [PubMed] [Google Scholar]
- 227.Kumar, A. et al. Linear and nonlinear optical properties of BiFeO3. Applied Physics Letters92, 10.1063/1.2901168 (2008).
- 228.Laiho R, Lakkisto M. Investigation of the refractive indices of LaF3, CeF3, PrF3 and NdF3. Philosophical Magazine B. 1983;48:203–207. doi: 10.1080/13642818308226470. [DOI] [Google Scholar]
- 229.Lajaunie, L., Boucher, F., Dessapt, R. & Moreau, P. Strong anisotropic influence of local-field effects on the dielectric response of α-MoO3. Physical Review B88, 10.1103/physrevb.88.115141 (2013).
- 230.Lane DW. The optical properties and laser irradiation of some common glasses. Journal of Physics D: Applied Physics. 1990;23:1727–1734. doi: 10.1088/0022-3727/23/12/037. [DOI] [Google Scholar]
- 231.Larruquert JI, Méndez JA, Aznárez JA. Far-ultraviolet reflectance measurements and optical constants of unoxidized aluminum films. Applied Optics. 1995;34:4892. doi: 10.1364/ao.34.004892. [DOI] [PubMed] [Google Scholar]
- 232.Larruquert JI, Méndez JA, Aznárez JA. Optical constants of aluminum films in the extreme ultraviolet interval of 82–77 nm. Applied Optics. 1996;35:5692. doi: 10.1364/ao.35.005692. [DOI] [PubMed] [Google Scholar]
- 233.Larruquert JI, Aznárez JA, Méndez JA, Calvo-Angós J. Optical properties of ytterbium films in the far and the extreme ultraviolet. Applied Optics. 2003;42:4566. doi: 10.1364/ao.42.004566. [DOI] [PubMed] [Google Scholar]
- 234.Larruquert JI, et al. Optical properties of scandium films in the far and the extreme ultraviolet. Applied Optics. 2004;43:3271. doi: 10.1364/ao.43.003271. [DOI] [PubMed] [Google Scholar]
- 235.Larruquert JI, et al. Transmittance and optical constants of erbium films in the 325–1580 eV spectral range. Applied Optics. 2011;50:2211. doi: 10.1364/ao.50.002211. [DOI] [PubMed] [Google Scholar]
- 236.Larruquert JI, et al. Self-consistent optical constants of SiC thin films. Journal of the Optical Society of America A. 2011;28:2340. doi: 10.1364/josaa.28.002340. [DOI] [PubMed] [Google Scholar]
- 237.Larruquert JI, et al. Self-consistent optical constants of sputter-deposited B4C thin films. Journal of the Optical Society of America A. 2011;29:117. doi: 10.1364/josaa.29.000117. [DOI] [PubMed] [Google Scholar]
- 238.Larruquert JI, Rodríguez-de Marcos LV, Méndez JA, Martin PJ, Bendavid A. High reflectance ta-C coatings in the extreme ultraviolet. Optics Express. 2013;21:27537. doi: 10.1364/oe.21.027537. [DOI] [PubMed] [Google Scholar]
- 239.Larsén T. Beitrag zur dispersion der edelgase. Zeitschrift für Physik. 1934;88:389–394. doi: 10.1007/bf01343498. [DOI] [Google Scholar]
- 240.Le TN, Pelouard J-L, Charra F, Vassant S. Determination of the far-infrared dielectric function of a thin InGaAs layer using a detuned Salisbury screen. Optical Materials Express. 2022;12:2711. doi: 10.1364/ome.455445. [DOI] [Google Scholar]
- 241.Lee, S., Jeong, T., Jung, S. & Yee, K. Refractive index dispersion of hexagonal boron nitride in the visible and near-infrared. physica status solidi (b)256, 10.1002/pssb.201800417 (2018).
- 242.Leguy AMA, et al. Reversible hydration of CH3NH3PbI3 in films, single crystals, and solar cells. Chemistry of Materials. 2015;27:3397–3407. doi: 10.1021/acs.chemmater.5b00660. [DOI] [Google Scholar]
- 243.Leite TR, Zschiedrich L, Kizilkaya O, McPeak KM. Resonant plasmonic–biomolecular chiral interactions in the far-ultraviolet: Enantiomeric discrimination of sub-10 nm amino acid films. Nano Letters. 2022;22:7343–7350. doi: 10.1021/acs.nanolett.2c01724. [DOI] [PubMed] [Google Scholar]
- 244.Leonard P. Refractive indices, verdet constants, and polarizabilities of the inert gases. Atomic Data and Nuclear Data Tables. 1974;14:21–37. doi: 10.1016/s0092-640x(74)80028-8. [DOI] [Google Scholar]
- 245.Li HH. Refractive index of alkali halides and its wavelength and temperature derivatives. Journal of Physical and Chemical Reference Data. 1976;5:329–528. doi: 10.1063/1.555536. [DOI] [Google Scholar]
- 246.Li HH. Refractive index of alkaline earth halides and its wavelength and temperature derivatives. Journal of Physical and Chemical Reference Data. 1980;9:161–290. doi: 10.1063/1.555616. [DOI] [Google Scholar]
- 247.Li HH. Refractive index of silicon and germanium and its wavelength and temperature derivatives. Journal of Physical and Chemical Reference Data. 1980;9:561–658. doi: 10.1063/1.555624. [DOI] [Google Scholar]
- 248.Li HH. Refractive index of ZnS, ZnSe, and ZnTe and its wavelength and temperature derivatives. Journal of Physical and Chemical Reference Data. 1984;13:103–150. doi: 10.1063/1.555705. [DOI] [Google Scholar]
- 249.Li J, Wen C-H, Gauza S, Lu R, Wu S-T. Refractive indices of liquid crystals for display applications. Journal of Display Technology. 2005;1:51–61. doi: 10.1109/jdt.2005.853357. [DOI] [Google Scholar]
- 250.Lin M, Sverdlov B, Strite S, Morkoç H, Drakin A. Refractive indices of wurtzite and zincblende GaN. Electronics Letters. 1993;29:1759. doi: 10.1049/el:19931172. [DOI] [Google Scholar]
- 251.Lin, Q. et al. Dispersion of silicon nonlinearities in the near infrared region. Applied Physics Letters91, 10.1063/1.2750523 (2007).
- 252.Lisitsa MP, Gudymenko LF, Malinko VN, Terekhova SF. Dispersion of the refractive indices and birefringence of CdSxSe1-x single crystals. physica status solidi (b) 1969;31:389–399. doi: 10.1002/pssb.19690310146. [DOI] [Google Scholar]
- 253.Logothetidis S, Petalas J, Cardona M, Moustakas TD. Optical properties and temperature dependence of the interband transitions of cubic and hexagonal GaN. Physical Review B. 1994;50:18017–18029. doi: 10.1103/physrevb.50.18017. [DOI] [PubMed] [Google Scholar]
- 254.Loiko P, Major A. Dispersive properties of alexandrite and beryllium hexaaluminate crystals. Optical Materials Express. 2016;6:2177. doi: 10.1364/ome.6.002177. [DOI] [Google Scholar]
- 255.Loiko P, et al. Sellmeier equations, group velocity dispersion, and thermo-optic dispersion formulas for CaLnAlO4 (Ln = Y, Gd) laser host crystals. Optics Letters. 2017;42:2275. doi: 10.1364/ol.42.002275. [DOI] [PubMed] [Google Scholar]
- 256.Lomheim TS, DeShazer LG. Optical-absorption intensities of trivalent neodymium in the uniaxial crystal yttrium orthovanadate. Journal of Applied Physics. 1978;49:5517–5522. doi: 10.1063/1.324471. [DOI] [Google Scholar]
- 257.Loria S. Über die dispersion des lichtes in gasförmigen kohlenwasserstoffen. Annalen der Physik. 1909;334:605–622. doi: 10.1002/andp.19093340809. [DOI] [Google Scholar]
- 258.Lorimor OG, Spitzer WG. Infrared refractive index and absorption of InAs and CdTe. Journal of Applied Physics. 1965;36:1841–1844. doi: 10.1063/1.1714362. [DOI] [Google Scholar]
- 259.Luke K, Okawachi Y, Lamont MRE, Gaeta AL, Lipson M. Broadband mid-infrared frequency comb generation in a Si3N4 microresonator. Optics Letters. 2015;40:4823. doi: 10.1364/ol.40.004823. [DOI] [PubMed] [Google Scholar]
- 260.Magnozzi M, Ferrera M, Mattera L, Canepa M, Bisio F. Plasmonics of au nanoparticles in a hot thermodynamic bath. Nanoscale. 2019;11:1140–1146. doi: 10.1039/c8nr09038f. [DOI] [PubMed] [Google Scholar]
- 261.Malitson IH. Refraction and dispersion of synthetic sapphire. Journal of the Optical Society of America. 1962;52:1377. doi: 10.1364/josa.52.001377. [DOI] [Google Scholar]
- 262.Malitson IH. A redetermination of some optical properties of calcium fluoride. Applied Optics. 1963;2:1103. doi: 10.1364/ao.2.001103. [DOI] [Google Scholar]
- 263.Malitson IH. Refractive properties of barium fluoride. Journal of the Optical Society of America. 1964;54:628. doi: 10.1364/josa.54.000628. [DOI] [Google Scholar]
- 264.Malitson IH. Interspecimen comparison of the refractive index of fused silica. Journal of the Optical Society of America. 1965;55:1205. doi: 10.1364/josa.55.001205. [DOI] [Google Scholar]
- 265.Mansfield CR, Peck ER. Dispersion of helium. Journal of the Optical Society of America. 1969;59:199. doi: 10.1364/josa.59.000199. [DOI] [Google Scholar]
- 266.Marcos, L. R.-d. et al. Optical constants of SrF2 thin films in the 25–780-eV spectral range. Journal of Applied Physics113, 10.1063/1.4800099 (2013).
- 267.Marcos LR-d, et al. Transmittance and optical constants of Ca films in the 4–1000 eV spectral range. Applied Optics. 2015;54:1910. doi: 10.1364/ao.54.001910. [DOI] [PubMed] [Google Scholar]
- 268.Marple DTF. Refractive index of ZnSe, ZnTe, and CdTe. Journal of Applied Physics. 1964;35:539–542. doi: 10.1063/1.1713411. [DOI] [Google Scholar]
- 269.Martonchik JV, Orton GS. Optical constants of liquid and solid methane. Applied Optics. 1994;33:8306. doi: 10.1364/ao.33.008306. [DOI] [PubMed] [Google Scholar]
- 270.Mathar RJ. Refractive index of humid air in the infrared: model fits. Journal of Optics A: Pure and Applied Optics. 2007;9:470–476. doi: 10.1088/1464-4258/9/5/008. [DOI] [Google Scholar]
- 271.Mathewson AG, Myers HP. Absolute values of the optical constants of some pure metals. Physica Scripta. 1971;4:291–292. doi: 10.1088/0031-8949/4/6/009. [DOI] [Google Scholar]
- 272.Mavrona E, et al. Refractive index measurement of IP-S and IP-Dip photoresists at THz frequencies and validation via 3D photonic metamaterials made by direct laser writing. Optical Materials Express. 2023;13:3355. doi: 10.1364/ome.500287. [DOI] [Google Scholar]
- 273.McPeak KM, et al. Plasmonic films can easily be better: Rules and recipes. ACS Photonics. 2015;2:326–333. doi: 10.1021/ph5004237. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 274.Medenbach O, Dettmar D, Shannon RD, Fischer RX, Yen WM. Refractive index and optical dispersion of rare earth oxides using a small-prism technique. Journal of Optics A: Pure and Applied Optics. 2001;3:174–177. doi: 10.1088/1464-4258/3/3/303. [DOI] [Google Scholar]
- 275.Meretska, M. L. et al. Measurements of the magneto-optical properties of thin-film EuS at room temperature in the visible spectrum. Applied Physics Letters120, 10.1063/5.0090533 (2022).
- 276.Milam D, Weber MJ, Glass AJ. Nonlinear refractive index of fluoride crystals. Applied Physics Letters. 1977;31:822–825. doi: 10.1063/1.89561. [DOI] [Google Scholar]
- 277.Milam D. Review and assessment of measured values of the nonlinear refractive-index coefficient of fused silica. Applied Optics. 1998;37:546. doi: 10.1364/ao.37.000546. [DOI] [PubMed] [Google Scholar]
- 278.Miller S, et al. Polarization-dependent nonlinear refractive index of BiB3O6. Optical Materials. 2008;30:1469–1472. doi: 10.1016/j.optmat.2007.11.015. [DOI] [Google Scholar]
- 279.Moerland RJ, Hoogenboom JP. Subnanometer-accuracy optical distance ruler based on fluorescence quenching by transparent conductors. Optica. 2016;3:112. doi: 10.1364/optica.3.000112. [DOI] [Google Scholar]
- 280.Monin J, Boutry GA. Optical and photoelectric properties of alkali metals. Physical Review B. 1974;9:1309–1327. doi: 10.1103/physrevb.9.1309. [DOI] [Google Scholar]
- 281.Moutzouris K, Hloupis G, Stavrakas I, Triantis D, Chou M-H. Temperature-dependent visible to near-infrared optical properties of 8 mol% Mg-doped lithium tantalate. Optical Materials Express. 2011;1:458. doi: 10.1364/ome.1.000458. [DOI] [Google Scholar]
- 282.Moutzouris K, Stavrakas I, Triantis D, Enculescu M. Temperature-dependent refractive index of potassium acid phthalate (KAP) in the visible and near-infrared. Optical Materials. 2011;33:812–816. doi: 10.1016/j.optmat.2010.12.021. [DOI] [Google Scholar]
- 283.Moutzouris K, et al. Refractive, dispersive and thermo-optic properties of twelve organic solvents in the visible and near-infrared. Applied Physics B. 2013;116:617–622. doi: 10.1007/s00340-013-5744-3. [DOI] [Google Scholar]
- 284.Munkhbat B, Wróbel P, Antosiewicz TJ, Shegai TO. Optical constants of several multilayer transition metal dichalcogenides measured by spectroscopic ellipsometry in the 300–1700 nm range: High index, anisotropy, and hyperbolicity. ACS Photonics. 2022;9:2398–2407. doi: 10.1021/acsphotonics.2c00433. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 285.Myers TL, et al. Accurate measurement of the optical constants n and k for a series of 57 inorganic and organic liquids for optical modeling and detection. Applied Spectroscopy. 2017;72:535–550. doi: 10.1177/0003702817742848. [DOI] [PubMed] [Google Scholar]
- 286.Nibbering ETJ, Grillon G, Franco MA, Prade BS, Mysyrowicz A. Determination of the inertial contribution to the nonlinear refractive index of air, N2, and O2 by use of unfocused high-intensity femtosecond laser pulses. Journal of the Optical Society of America B. 1997;14:650. doi: 10.1364/josab.14.000650. [DOI] [Google Scholar]
- 287.Nigara Y. Measurement of the optical constants of yttrium oxide. Japanese Journal of Applied Physics. 1968;7:404. doi: 10.1143/jjap.7.404. [DOI] [Google Scholar]
- 288.Ninomiya S, Adachi S. Optical properties of wurtzite CdS. Journal of Applied Physics. 1995;78:1183–1190. doi: 10.1063/1.360355. [DOI] [Google Scholar]
- 289.Ninomiya S, Adachi S. Optical properties of cubic and hexagonal CdSe. Journal of Applied Physics. 1995;78:4681–4689. doi: 10.1063/1.359815. [DOI] [Google Scholar]
- 290.Nunley, T. N. et al. Optical constants of germanium and thermally grown germanium dioxide from 0.5 to 6.6 eV via a multisample ellipsometry investigation. Journal of Vacuum Science & Technology B, Nanotechnology and Microelectronics: Materials, Processing, Measurement, and Phenomena34, 10.1116/1.4963075 (2016).
- 291.Nyakuchena M, Juntunen C, Shea P, Sung Y. Refractive index dispersion measurement in the short-wave infrared range using synthetic phase microscopy. Physical Chemistry Chemical Physics. 2023;25:23141–23149. doi: 10.1039/d3cp03158f. [DOI] [PubMed] [Google Scholar]
- 292.Oguntoye IO, et al. Continuously tunable optical modulation using vanadium dioxide huygens metasurfaces. ACS Applied Materials & Interfaces. 2023;15:41141–41150. doi: 10.1021/acsami.3c08493. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 293.Old JG, Gentili KL, Peck ER. Dispersion of carbon dioxide. Journal of the Optical Society of America. 1971;61:89. doi: 10.1364/josa.61.000089. [DOI] [Google Scholar]
- 294.Olmon, R. L. et al. Optical dielectric function of gold. Physical Review B86, 10.1103/physrevb.86.235147 (2012).
- 295.Ordal MA, Bell RJ, Alexander RW, Long LL, Querry MR. Optical properties of fourteen metals in the infrared and far infrared: Al, Co, Cu, Au, Fe, Pb, Mo, Ni, Pd, Pt, Ag, Ti, V, and W. Applied Optics. 1985;24:4493. doi: 10.1364/ao.24.004493. [DOI] [PubMed] [Google Scholar]
- 296.Ordal MA, Bell RJ, Alexander RW, Long LL, Querry MR. Optical properties of Au, Ni, and Pb at submillimeter wavelengths. Applied Optics. 1987;26:744. doi: 10.1364/ao.26.000744. [DOI] [PubMed] [Google Scholar]
- 297.Ordal MA, Bell RJ, Alexander RW, Newquist LA, Querry MR. Optical properties of Al, Fe, Ti, Ta, W, and Mo at submillimeter wavelengths. Applied Optics. 1988;27:1203. doi: 10.1364/ao.27.001203. [DOI] [PubMed] [Google Scholar]
- 298.Otanicar TP, Phelan PE, Golden JS. Optical properties of liquids for direct absorption solar thermal energy systems. Solar Energy. 2009;83:969–977. doi: 10.1016/j.solener.2008.12.009. [DOI] [Google Scholar]
- 299.Owyoung A. Ellipse rotation studies in laser host materials. IEEE Journal of Quantum Electronics. 1973;9:1064–1069. doi: 10.1109/jqe.1973.1077417. [DOI] [Google Scholar]
- 300.Ozaki S, Adachi S. Spectroscopic ellipsometry and thermoreflectance of GaAs. Journal of Applied Physics. 1995;78:3380–3386. doi: 10.1063/1.359966. [DOI] [Google Scholar]
- 301.Palm KJ, Murray JB, Narayan TC, Munday JN. Dynamic optical properties of metal hydrides. ACS Photonics. 2018;5:4677–4686. doi: 10.1021/acsphotonics.8b01243. [DOI] [Google Scholar]
- 302.Panah MEA, et al. Highly doped inp as a low loss plasmonic material for mid-IR region. Optics Express. 2016;24:29077. doi: 10.1364/oe.24.029077. [DOI] [PubMed] [Google Scholar]
- 303.Papatryfonos, K. et al. Refractive indices of mbe-grown AlxGa(1-x)As ternary alloys in the transparent wavelength region. AIP Advances11, 10.1063/5.0039631 (2021).
- 304.Parsons DF, Coleman PD. Far infrared optical constants of gallium phosphide. Applied Optics. 1971;10:1683. doi: 10.1364/ao.10.1683_1. [DOI] [PubMed] [Google Scholar]
- 305.Pastrňák, J. & Roskovcová, L. Refraction index measurements on AlN single crystals. physica status solidi (b)14, 10.1002/pssb.19660140127 (1966).
- 306.Patwardhan GN, Ginsberg JS, Chen CY, Jadidi MM, Gaeta AL. Nonlinear refractive index of solids in mid-infrared. Optics Letters. 2021;46:1824. doi: 10.1364/ol.421469. [DOI] [PubMed] [Google Scholar]
- 307.Peck ER, Fisher DJ. Dispersion of argon. Journal of the Optical Society of America. 1964;54:1362. doi: 10.1364/josa.54.001362. [DOI] [Google Scholar]
- 308.Peck ER, Khanna BN. Dispersion of nitrogen. Journal of the Optical Society of America. 1966;56:1059. doi: 10.1364/josa.56.001059. [DOI] [Google Scholar]
- 309.Peck ER, Reeder K. Dispersion of air. Journal of the Optical Society of America. 1972;62:958. doi: 10.1364/josa.62.000958. [DOI] [Google Scholar]
- 310.Peck ER, Huang S. Refractivity and dispersion of hydrogen in the visible and near infrared. Journal of the Optical Society of America. 1977;67:1550. doi: 10.1364/josa.67.001550. [DOI] [Google Scholar]
- 311.Pennington DM, Henesian MA, Hellwarth RW. Nonlinear index of air at 1.053 μm. Physical Review A. 1989;39:3003–3009. doi: 10.1103/physreva.39.3003. [DOI] [PubMed] [Google Scholar]
- 312.Perner, L. W. et al. Simultaneous measurement of mid-infrared refractive indices in thin-film heterostructures: Methodology and results for GaAs/AlGaAs. Physical Review Research5, 10.1103/physrevresearch.5.033048 (2023).
- 313.Perotto, G. et al. The optical properties of regenerated silk fibroin films obtained from different sources. Applied Physics Letters111, 10.1063/1.4998950 (2017).
- 314.Pestryakov EV, et al. Physical properties of BeAl6O10 single crystals. Journal of Applied Physics. 1997;82:3661–3666. doi: 10.1063/1.365728. [DOI] [Google Scholar]
- 315.Peter F. Über brechungsindizes und absorptionskonstanten des diamanten zwischen 644 und 226 mμ. Zeitschrift für Physik. 1923;15:358–368. doi: 10.1007/bf01330487. [DOI] [Google Scholar]
- 316.Pettit GD, Turner WJ. Refractive index of InP. Journal of Applied Physics. 1965;36:2081–2081. doi: 10.1063/1.1714410. [DOI] [Google Scholar]
- 317.Pflüger J, Fink J, Weber W, Bohnen KP, Crecelius G. Dielectric properties of TiCx, TiNx, VCx, and VNx from 1.5 to 40 eV determined by electron-energy-loss spectroscopy. Physical Review B. 1984;30:1155–1163. doi: 10.1103/physrevb.30.1155. [DOI] [PubMed] [Google Scholar]
- 318.Philipp HR. Optical properties of silicon nitride. Journal of The Electrochemical Society. 1973;120:295. doi: 10.1149/1.2403440. [DOI] [Google Scholar]
- 319.Philipp HR, Cole HS, Liu YS, Sitnik TA. Optical absorption of some polymers in the region 240–170 nm. Applied Physics Letters. 1986;48:192–194. doi: 10.1063/1.96940. [DOI] [Google Scholar]
- 320.Phillip HR, Taft EA. Kramers-Kronig analysis of reflectance data for diamond. Physical Review. 1964;136:A1445–A1448. doi: 10.1103/physrev.136.a1445. [DOI] [Google Scholar]
- 321.Phillips LJ, et al. Dispersion relation data for methylammonium lead triiodide perovskite deposited on a (100) silicon wafer using a two-step vapour-phase reaction process. Data in Brief. 2015;5:926–928. doi: 10.1016/j.dib.2015.10.026. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 322.Pierce DT, Spicer WE. Electronic structure of amorphous Si from photoemission and optical studies. Physical Review B. 1972;5:3017–3029. doi: 10.1103/physrevb.5.3017. [DOI] [Google Scholar]
- 323.Pigeon JJ, Tochitsky SY, Welch EC, Joshi C. Measurements of the nonlinear refractive index of air, N2, and O2 at 10 μm using four-wave mixing. Optics Letters. 2016;41:3924. doi: 10.1364/ol.41.003924. [DOI] [PubMed] [Google Scholar]
- 324.Pigeon JJ, Matteo DA, Tochitsky SY, Ben-Zvi I, Joshi C. Measurements of the nonlinear refractive index of AgGaSe2, GaSe, and ZnSe at 10 μm. Journal of the Optical Society of America B. 2020;37:2076. doi: 10.1364/josab.395844. [DOI] [Google Scholar]
- 325.Polyanskiy MN, et al. Single-shot measurement of the nonlinear refractive index of air at 9.2 μm with a picosecond terawatt CO2 laser. Optics Letters. 2021;46:2067. doi: 10.1364/ol.423800. [DOI] [PubMed] [Google Scholar]
- 326.Polyanskiy MN, et al. Post-compression of long-wave infrared 2 picosecond sub-terawatt pulses in bulk materials. Optics Express. 2021;29:31714. doi: 10.1364/oe.434238. [DOI] [PubMed] [Google Scholar]
- 327.Radhakrishnan, T. The dispersion, briefringence and optical activity of quartz. Proceedings of the Indian Academy of Sciences - Section A25, 10.1007/bf03171408 (1947).
- 328.Radhakrishnan, T. Further studies on the temperature variation of the refractive index of crystals. Proceedings of the Indian Academy of Sciences - Section A33, 10.1007/bf03172255 (1951).
- 329.Rakić AD. Algorithm for the determination of intrinsic optical constants of metal films: application to aluminum. Applied Optics. 1995;34:4755. doi: 10.1364/ao.34.004755. [DOI] [PubMed] [Google Scholar]
- 330.Rakić AD, Majewski ML. Modeling the optical dielectric function of GaAs and AlAs: Extension of Adachi’s model. Journal of Applied Physics. 1996;80:5909–5914. doi: 10.1063/1.363586. [DOI] [Google Scholar]
- 331.Rakić AD, Djurišić AB, Elazar JM, Majewski ML. Optical properties of metallic films for vertical-cavity optoelectronic devices. Applied Optics. 1998;37:5271. doi: 10.1364/ao.37.005271. [DOI] [PubMed] [Google Scholar]
- 332.Rasigni M, Rasigni G. Optical constants of lithium deposits as determined from the Kramers-Kronig analysis. Journal of the Optical Society of America. 1977;67:54. doi: 10.1364/josa.67.000054. [DOI] [Google Scholar]
- 333.Ratzsch S, Kley E-B, Tünnermann A, Szeghalmi A. Influence of the oxygen plasma parameters on the atomic layer deposition of titanium dioxide. Nanotechnology. 2014;26:024003. doi: 10.1088/0957-4484/26/2/024003. [DOI] [PubMed] [Google Scholar]
- 334.Rheims J, Köser J, Wriedt T. Refractive-index measurements in the near-IR using an Abbe refractometer. Measurement Science and Technology. 1997;8:601–605. doi: 10.1088/0957-0233/8/6/003. [DOI] [Google Scholar]
- 335.Rioux D, et al. An analytic model for the dielectric function of Au, Ag, and their alloys. Advanced Optical Materials. 2013;2:176–182. doi: 10.1002/adom.201300457. [DOI] [Google Scholar]
- 336.Rodney WS. Optical properties of cesium iodide. Journal of the Optical Society of America. 1955;45:987. doi: 10.1364/josa.45.000987. [DOI] [Google Scholar]
- 337.Rodney WS, Malitson IH. Refraction and dispersion of thallium bromide iodide. Journal of the Optical Society of America. 1956;46:956. doi: 10.1364/josa.46.000956. [DOI] [Google Scholar]
- 338.Rodney WS, Malitson IH, King TA. Refractive index of arsenic trisulfide. Journal of the Optical Society of America. 1958;48:633. doi: 10.1364/josa.48.000633. [DOI] [Google Scholar]
- 339.Rodríguez-de Marcos, L. et al. Transmittance and optical constants of Sr films in the 6–1220 eV spectral range. Journal of Applied Physics111, 10.1063/1.4729487 (2012).
- 340.Rodríguez-de Marcos LV, Larruquert JI, Méndez JA, Aznárez JA. Self-consistent optical constants of SiO2 and Ta2O5 films. Optical Materials Express. 2016;6:3622. doi: 10.1364/ome.6.003622. [DOI] [Google Scholar]
- 341.Rodríguez-de Marcos LV, Larruquert JI, Méndez JA, Aznárez JA. Self-consistent optical constants of MgF2, LaF3, and CeF3 films. Optical Materials Express. 2017;7:989. doi: 10.1364/ome.7.000989. [DOI] [Google Scholar]
- 342.Rollefson R, Havens R. Index of refraction of methane in the infra-red and the dipole moment of the CH bond. Physical Review. 1940;57:710–717. doi: 10.1103/physrev.57.710. [DOI] [Google Scholar]
- 343.Rosenblatt, G., Simkhovich, B., Bartal, G. & Orenstein, M. Nonmodal plasmonics: Controlling the forced optical response of nanostructures. Physical Review X10, 10.1103/physrevx.10.011071 (2020).
- 344.Rosker M, Cheng K, Tang C. Practical urea optical parametric oscillator for tunable generation throughout the visible and near-infrared. IEEE Journal of Quantum Electronics. 1985;21:1600–1606. doi: 10.1109/jqe.1985.1072557. [DOI] [Google Scholar]
- 345.Rowe, D. J., Smith, D. & Wilkinson, J. S. Complex refractive index spectra of whole blood and aqueous solutions of anticoagulants, analgesics and buffers in the mid-infrared. Scientific Reports7, 10.1038/s41598-017-07842-0 (2017). [DOI] [PMC free article] [PubMed]
- 346.Rowe, P. M., Fergoda, M. & Neshyba, S. Temperature-dependent optical properties of liquid water from 240 to 298 K. Journal of Geophysical Research: Atmospheres125, 10.1029/2020jd032624 (2020).
- 347.Rubin M. Optical properties of soda lime silica glasses. Solar Energy Materials. 1985;12:275–288. doi: 10.1016/0165-1633(85)90052-8. [DOI] [Google Scholar]
- 348.Sahin S, Nahar NK, Sertel K. Dielectric properties of low-loss polymers for mmw and THz applications. Journal of Infrared, Millimeter, and Terahertz Waves. 2019;40:557–573. doi: 10.1007/s10762-019-00584-2. [DOI] [Google Scholar]
- 349.Salzberg CD, Villa JJ. Infrared refractive indexes of silicon germanium and modified selenium glass. Journal of the Optical Society of America. 1957;47:244. doi: 10.1364/josa.47.000244. [DOI] [Google Scholar]
- 350.Sani E, Dell’Oro A. Optical constants of ethylene glycol over an extremely wide spectral range. Optical Materials. 2014;37:36–41. doi: 10.1016/j.optmat.2014.04.035. [DOI] [Google Scholar]
- 351.Sani E, Dell’Oro A. Corrigendum to “Optical constants of ethylene glycol over an extremely wide spectral range [opt. mater. 37 (2014) 36–41] Optical Materials. 2015;48:281. doi: 10.1016/j.optmat.2015.06.039. [DOI] [Google Scholar]
- 352.Sani E, Dell’Oro A. Spectral optical constants of ethanol and isopropanol from ultraviolet to far infrared. Optical Materials. 2016;60:137–141. doi: 10.1016/j.optmat.2016.06.041. [DOI] [Google Scholar]
- 353.Sarkar S, et al. Hybridized guided-mode resonances via colloidal plasmonic self-assembled grating. ACS Applied Materials & Interfaces. 2019;11:13752–13760. doi: 10.1021/acsami.8b20535. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 354.Sarkar, S. et al. Enhanced figure of merit via hybridized guided-mode resonances in 2d-metallic photonic crystal slabs. Advanced Optical Materials10, 10.1002/adom.202200954 (2022).
- 355.Sasaki T, Mori Y, Yoshimura M. Progress in the growth of a CsLiB6O10 crystal and its application to ultraviolet light generation. Optical Materials. 2003;23:343–351. doi: 10.1016/s0925-3467(02)00316-6. [DOI] [Google Scholar]
- 356.Sato K, Adachi S. Optical properties of ZnTe. Journal of Applied Physics. 1993;73:926–931. doi: 10.1063/1.353305. [DOI] [Google Scholar]
- 357.Schinke C, Hinken D, Schmidt J, Bothe K, Brendel R. Modeling the spectral luminescence emission of silicon solar cells and wafers. IEEE Journal of Photovoltaics. 2013;3:1038–1052. doi: 10.1109/jphotov.2013.2263985. [DOI] [Google Scholar]
- 358.Schinke, C. et al. Uncertainty analysis for the coefficient of band-to-band absorption of crystalline silicon. AIP Advances5, 10.1063/1.4923379 (2015).
- 359.Schmitt P, et al. Optical, structural, and functional properties of highly reflective and stable iridium mirror coatings for infrared applications. Optical Materials Express. 2022;12:545. doi: 10.1364/ome.447306. [DOI] [Google Scholar]
- 360.Schnabel, V., Spolenak, R., Doebeli, M. & Galinski, H. Structural color sensors with thermal memory: Measuring functional properties of Ti-based nitrides by eye. Advanced Optical Materials6, 10.1002/adom.201800656 (2018).
- 361.Schneider F, Draheim J, Kamberger R, Wallrabe U. Process and material properties of polydimethylsiloxane (PDMS) for optical MEMS. Sensors and Actuators A: Physical. 2009;151:95–99. doi: 10.1016/j.sna.2009.01.026. [DOI] [Google Scholar]
- 362.Schnepf MJ, et al. Nanorattles with tailored electric field enhancement. Nanoscale. 2017;9:9376–9385. doi: 10.1039/c7nr02952g. [DOI] [PubMed] [Google Scholar]
- 363.Schröter H. Über die brechungsindizes einiger schwermetallhalogenide im sichtbaren und die berechnung von interpolationsformeln für den dispersionsverlauf. Zeitschrift für Physik. 1931;67:24–36. doi: 10.1007/bf01391040. [DOI] [Google Scholar]
- 364.Schubert M, et al. Optical constants of GaxIn1-xP lattice matched to GaAs. Journal of Applied Physics. 1995;77:3416–3419. doi: 10.1063/1.358632. [DOI] [Google Scholar]
- 365.Seçkin S, Singh P, Jaiswal A, König TAF. Super-radiant sers enhancement by plasmonic particle gratings. ACS Applied Materials & Interfaces. 2023;15:43124–43134. doi: 10.1021/acsami.3c07532. [DOI] [PubMed] [Google Scholar]
- 366.Selivanov A, Denisov I, Kuleshov N, Yumashev K. Nonlinear refractive properties of Yb3+-doped KY(WO4)2 and YVO4 laser crystals. Applied Physics B. 2006;83:61–65. doi: 10.1007/s00340-005-2098-5. [DOI] [Google Scholar]
- 367.Shaffer PTB. Refractive index, dispersion, and birefringence of silicon carbide polytypes. Applied Optics. 1971;10:1034. doi: 10.1364/ao.10.001034. [DOI] [PubMed] [Google Scholar]
- 368.Shaw M, Hooker C, Wilson D. Measurement of the nonlinear refractive index of air and other gases at 248 nm. Optics Communications. 1993;103:153–160. doi: 10.1016/0030-4018(93)90657-q. [DOI] [Google Scholar]
- 369.Sheik-Bahae M, Hutchings D, Hagan D, Van Stryland E. Dispersion of bound electron nonlinear refraction in solids. IEEE Journal of Quantum Electronics. 1991;27:1296–1309. doi: 10.1109/3.89946. [DOI] [Google Scholar]
- 370.Shimoji Y, Fay AT, Chang RSF, Djeu N. Direct measurement of the nonlinear refractive index of air. Journal of the Optical Society of America B. 1989;6:1994. doi: 10.1364/josab.6.001994. [DOI] [Google Scholar]
- 371.Shkondin E, Repän T, Takayama O, Lavrinenko AV. High aspect ratio titanium nitride trench structures as plasmonic biosensor. Optical Materials Express. 2017;7:4171. doi: 10.1364/ome.7.004171. [DOI] [Google Scholar]
- 372.Shkondin E, et al. Large-scale high aspect ratio Al-doped ZnO nanopillars arrays as anisotropic metamaterials. Optical Materials Express. 2017;7:1606. doi: 10.1364/ome.7.001606. [DOI] [Google Scholar]
- 373.Shunji Ozaki SO, Sadao Adachi SA. Optical constants of cubic ZnS. Japanese Journal of Applied Physics. 1993;32:5008. doi: 10.1143/jjap.32.5008. [DOI] [Google Scholar]
- 374.Siefke T, et al. Materials pushing the application limits of wire grid polarizers further into the deep ultraviolet spectral range. Advanced Optical Materials. 2016;4:1780–1786. doi: 10.1002/adom.201600250. [DOI] [Google Scholar]
- 375.Šik J, Hora J, Humlíček J. Optical functions of silicon at high temperatures. Journal of Applied Physics. 1998;84:6291–6298. doi: 10.1063/1.368951. [DOI] [Google Scholar]
- 376.Simon M, et al. Refractive indices of photorefractive bismuth titanate, barium-calcium titanate, bismuth germanium oxide, and lead germanate. physica status solidi (a) 1997;159:559–562. doi: 10.1002/1521-396X(199702)159:2<559::AID-PSSA559>3.0.CO;2-0. [DOI] [Google Scholar]
- 377.Singh S, Potopowicz JR, Van Uitert LG, Wemple SH. Nonlinear optical properties of hexagonal silicon carbide. Applied Physics Letters. 1971;19:53–56. doi: 10.1063/1.1653819. [DOI] [Google Scholar]
- 378.Singh S, Remeika JP, Potopowicz JR. Nonlinear optical properties of ferroelectric lead titanate. Applied Physics Letters. 1972;20:135–137. doi: 10.1063/1.1654078. [DOI] [Google Scholar]
- 379.Sinnock AC, Smith BL. Refractive indices of the condensed inert gases. Physical Review. 1969;181:1297–1307. doi: 10.1103/physrev.181.1297. [DOI] [Google Scholar]
- 380.Skauli T, et al. Improved dispersion relations for gaas and applications to nonlinear optics. Journal of Applied Physics. 2003;94:6447–6455. doi: 10.1063/1.1621740. [DOI] [Google Scholar]
- 381.Smith NV. Optical constants of sodium and potassium from 0.5 to 4.0 eV by split-beam ellipsometry. Physical Review. 1969;183:634–644. doi: 10.1103/physrev.183.634. [DOI] [Google Scholar]
- 382.Smith NV. Optical constants of rubidium and cesium from 0.5 to 4.0 eV. Physical Review B. 1970;2:2840–2848. doi: 10.1103/physrevb.2.2840. [DOI] [Google Scholar]
- 383.Smith DR, Loewenstein EV. Optical constants of far infrared materials 3: plastics. Applied Optics. 1975;14:1335. doi: 10.1364/ao.14.001335. [DOI] [PubMed] [Google Scholar]
- 384.Smith PL, Huber MCE, Parkinson WH. Refractivities of H2, He, O2, CO, and Kr for 168 ≤ λ ≤ 288 nm. Physical Review A. 1976;13:1422–1434. doi: 10.1103/physreva.13.1422. [DOI] [Google Scholar]
- 385.Smith FW. Optical constants of a hydrogenated amorphous carbon film. Journal of Applied Physics. 1984;55:764–771. doi: 10.1063/1.333135. [DOI] [Google Scholar]
- 386.Sneep M, Ubachs W. Direct measurement of the Rayleigh scattering cross section in various gases. Journal of Quantitative Spectroscopy and Radiative Transfer. 2005;92:293–310. doi: 10.1016/j.jqsrt.2004.07.025. [DOI] [Google Scholar]
- 387.Song, B. et al. Layer-dependent dielectric function of wafer-scale 2D MoS2. Advanced Optical Materials7, 10.1002/adom.201801250 (2018).
- 388.Song B, et al. Broadband optical properties of graphene and hopg investigated by spectroscopic Mueller matrix ellipsometry. Applied Surface Science. 2018;439:1079–1087. doi: 10.1016/j.apsusc.2018.01.051. [DOI] [Google Scholar]
- 389.Song B, et al. Determination of dielectric functions and exciton oscillator strength of two-dimensional hybrid perovskites. ACS Materials Letters. 2020;3:148–159. doi: 10.1021/acsmaterialslett.0c00505. [DOI] [Google Scholar]
- 390.Song B, et al. Giant gate-tunability of complex refractive index in semiconducting carbon nanotubes. ACS Photonics. 2020;7:2896–2905. doi: 10.1021/acsphotonics.0c01220. [DOI] [Google Scholar]
- 391.Stahrenberg, K. et al. Optical properties of copper and silver in the energy range 2.5–9.0 eV. Physical Review B64, 10.1103/physrevb.64.115111 (2001).
- 392.Stefaniuk, T. et al. Optical, electronic, and structural properties of ScAlMgO4. Physical Review B107, 10.1103/physrevb.107.085205 (2023).
- 393.Stelling, C. et al. Plasmonic nanomeshes: their ambivalent role as transparent electrodes in organic solar cells. Scientific Reports7, 10.1038/srep42530 (2017). [DOI] [PMC free article] [PubMed]
- 394.Stephens R, Malitson I. Index of refraction of magnesium oxide. Journal of Research of the National Bureau of Standards. 1952;49:249. doi: 10.6028/jres.049.025. [DOI] [Google Scholar]
- 395.Supansomboon S, Maaroof A, Cortie MB. “purple glory”: The optical properties and technology of AuAl2 coatings. Gold Bulletin. 2008;41:296–304. doi: 10.1007/bf03214887. [DOI] [Google Scholar]
- 396.Sutherland JC, Arakawa ET. Optical properties of potassium for photons of energy 396 to 969 eV. Journal of the Optical Society of America. 1968;58:1080. doi: 10.1364/josa.58.001080. [DOI] [Google Scholar]
- 397.Suzuki N, Sawai K, Adachi S. Optical properties of PbSe. Journal of Applied Physics. 1995;77:1249–1255. doi: 10.1063/1.358926. [DOI] [Google Scholar]
- 398.Svechnikov M, et al. Optical constants of sputtered beryllium thin films determined from photoabsorption measurements in the spectral range 20.4–250 eV. Journal of Synchrotron Radiation. 2020;27:75–82. doi: 10.1107/s1600577519014188. [DOI] [PubMed] [Google Scholar]
- 399.Sytchkova A, Belosludtsev A, Volosevičienė L, Juškėnas R, Simniškis R. Optical, structural and electrical properties of sputtered ultrathin chromium films. Optical Materials. 2021;121:111530. doi: 10.1016/j.optmat.2021.111530. [DOI] [Google Scholar]
- 400.Takaoka E, Kato K. Thermo-optic dispersion formula for AgGaS2. Applied Optics. 1999;38:4577. doi: 10.1364/ao.38.004577. [DOI] [PubMed] [Google Scholar]
- 401.Tamošauskas G, Beresnevičius G, Gadonas D, Dubietis A. Transmittance and phase matching of BBO crystal in the 3–5 µm range and its application for the characterization of mid-infrared laser pulses. Optical Materials Express. 2018;8:1410. doi: 10.1364/ome.8.001410. [DOI] [Google Scholar]
- 402.Tan C. Determination of refractive index of silica glass for infrared wavelengths by IR spectroscopy. Journal of Non-Crystalline Solids. 1998;223:158–163. doi: 10.1016/s0022-3093(97)00438-9. [DOI] [Google Scholar]
- 403.Tatian B. Fitting refractive-index data with the Sellmeier dispersion formula. Applied Optics. 1984;23:4477. doi: 10.1364/ao.23.004477. [DOI] [PubMed] [Google Scholar]
- 404.Taylor A, et al. Comparative determination of atomic boron and carrier concentration in highly boron doped nano-crystalline diamond. Diamond and Related Materials. 2023;135:109837. doi: 10.1016/j.diamond.2023.109837. [DOI] [Google Scholar]
- 405.Tikuišis KK, et al. Optical and magneto-optical properties of permalloy thin films in 0.7–6.4 eV photon energy range. Materials & Design. 2017;114:31–39. doi: 10.1016/j.matdes.2016.10.036. [DOI] [Google Scholar]
- 406.Tikuišis, K. K. et al. Dielectric function of epitaxial quasi-freestanding monolayer graphene on Si-face 6H-SiC in a broad spectral range. Physical Review Materials7, 10.1103/physrevmaterials.7.044201 (2023).
- 407.Tilton LW, Plyler EK, Stephens RE. Refractive index of silver chloride for visible and infra-red radiant energy. Journal of the Optical Society of America. 1950;40:540. doi: 10.1364/josa.40.000540. [DOI] [Google Scholar]
- 408.Tkachenko V, et al. Nematic liquid crystal optical dispersion in the visible-near infrared range. Molecular Crystals and Liquid Crystals. 2006;454:263/[665]–271/[673]. doi: 10.1080/15421400600655816. [DOI] [Google Scholar]
- 409.Treharne RE, et al. Optical design and fabrication of fully sputtered CdTe/CdS solar cells. Journal of Physics: Conference Series. 2011;286:012038. doi: 10.1088/1742-6596/286/1/012038. [DOI] [Google Scholar]
- 410.Tsuda S, Yamaguchi S, Kanamori Y, Yugami H. Spectral and angular shaping of infrared radiation in a polymer resonator with molecular vibrational modes. Optics Express. 2018;26:6899. doi: 10.1364/oe.26.006899. [DOI] [PubMed] [Google Scholar]
- 411.Uchida N. Optical properties of single-crystal paratellurite (TeO2) Physical Review B. 1971;4:3736–3745. doi: 10.1103/physrevb.4.3736. [DOI] [Google Scholar]
- 412.Umegaki S, Tanaka S-I, Uchiyama T, Yabumoto S. Refractive indices of lithium iodate between 0.4 and 2.2 μ. Optics Communications. 1971;3:244–245. doi: 10.1016/0030-4018(71)90013-7. [DOI] [Google Scholar]
- 413.Valentine J, et al. Three-dimensional optical metamaterial with a negative refractive index. Nature. 2008;455:376–379. doi: 10.1038/nature07247. [DOI] [PubMed] [Google Scholar]
- 414.Vidal-Dasilva, M. et al. Transmittance and optical constants of Tm films in the 2.75–1600 eV spectral range. Journal of Applied Physics105, 10.1063/1.3129507 (2009).
- 415.Vidal-Dasilva, M., Aquila, A. L., Gullikson, E. M., Salmassi, F. & Larruquert, J. I. Optical constants of magnetron-sputtered magnesium films in the 25–1300 eV energy range. Journal of Applied Physics108, 10.1063/1.3481457 (2010).
- 416.Vogt MR, et al. Measurement of the optical constants of soda-lime glasses in dependence of iron content and modeling of iron-related power losses in crystalline si solar cell modules. IEEE Journal of Photovoltaics. 2016;6:111–118. doi: 10.1109/jphotov.2015.2498043. [DOI] [Google Scholar]
- 417.Vogt MR, et al. Optical constants of UV transparent EVA and the impact on the PV module output power under realistic irradiation. Energy Procedia. 2016;92:523–530. doi: 10.1016/j.egypro.2016.07.136. [DOI] [Google Scholar]
- 418.Vos, M. F. J., Macco, B., Thissen, N. F. W., Bol, A. A. & Kessels, W. M. M. E. Atomic layer deposition of molybdenum oxide from (NtBu)2(NMe2)2Mo and O2 plasma. Journal of Vacuum Science & Technology A: Vacuum, Surfaces, and Films34, 10.1116/1.4930161 (2015).
- 419.Vukovic D, Woolsey GA, Scelsi GB. Refractivities of SF6 and SOF2 at wavelengths of 632.99 and 1300 nm. Journal of Physics D: Applied Physics. 1996;29:634–637. doi: 10.1088/0022-3727/29/3/023. [DOI] [Google Scholar]
- 420.Vuye G, et al. Temperature dependence of the dielectric function of silicon using in situ spectroscopic ellipsometry. Thin Solid Films. 1993;233:166–170. doi: 10.1016/0040-6090(93)90082-z. [DOI] [Google Scholar]
- 421.Wahlstrand, J. K., Cheng, Y.-H. & Milchberg, H. M. Absolute measurement of the transient optical nonlinearity in N2, O2, N2O, and Ar. Physical Review A85, 10.1103/physreva.85.043820 (2012).
- 422.Walling J, Peterson O, Jenssen H, Morris R, O’Dell E. Tunable alexandrite lasers. IEEE Journal of Quantum Electronics. 1980;16:1302–1315. doi: 10.1109/jqe.1980.1070430. [DOI] [Google Scholar]
- 423.Wang S, et al. 4H-SiC: a new nonlinear material for midinfrared lasers. Laser & Photonics Reviews. 2013;7:831–838. doi: 10.1002/lpor.201300068. [DOI] [Google Scholar]
- 424.Wang, Y. et al. Measurement of absorption spectrum of deuterium oxide (D2O) and its application to signal enhancement in multiphoton microscopy at the 1700-nm window. Applied Physics Letters108, 10.1063/1.4939970 (2016).
- 425.Wang K, et al. Order-of-magnitude multiphoton signal enhancement based on characterization of absorption spectra of immersion oils at the 1700-nm window. Optics Express. 2017;25:5909. doi: 10.1364/oe.25.005909. [DOI] [PubMed] [Google Scholar]
- 426.Wang CC, Tan JY, Jing CY, Liu LH. Temperature-dependent optical constants of liquid isopropanol, n-butanol, and n-decane. Applied Optics. 2018;57:3003. doi: 10.1364/ao.57.003003. [DOI] [PubMed] [Google Scholar]
- 427.Warren SG. Optical constants of ice from the ultraviolet to the microwave. Applied Optics. 1984;23:1206. doi: 10.1364/ao.23.001206. [DOI] [PubMed] [Google Scholar]
- 428.Warren, S. G. & Brandt, R. E. Optical constants of ice from the ultraviolet to the microwave: A revised compilation. Journal of Geophysical Research: Atmospheres113, 10.1029/2007jd009744 (2008).
- 429.Weaver JH, Lynch DW, Olson CG. Optical properties of niobium from 0.1 to 36.4 eV. Physical Review B. 1973;7:4311–4318. doi: 10.1103/physrevb.7.4311. [DOI] [Google Scholar]
- 430.Weaver JH, Olson CG, Lynch DW. Optical properties of crystalline tungsten. Physical Review B. 1975;12:1293–1297. doi: 10.1103/physrevb.12.1293. [DOI] [Google Scholar]
- 431.Weaver JH, Olson CG, Lynch DW. Optical investigation of the electronic structure of bulk Rh and Ir. Physical Review B. 1977;15:4115–4118. doi: 10.1103/physrevb.15.4115. [DOI] [Google Scholar]
- 432.Weber, J. W., Calado, V. E. & van de Sanden, M. C. M. Optical constants of graphene measured by spectroscopic ellipsometry. Applied Physics Letters97, 10.1063/1.3475393 (2010).
- 433.Weiting F, Yixun Y. Temperature effects on the refractive index of lead telluride and zinc selenide. Infrared Physics. 1990;30:371–373. doi: 10.1016/0020-0891(90)90055-z. [DOI] [Google Scholar]
- 434.Werner WSM, Glantschnig K, Ambrosch-Draxl C. Optical constants and inelastic electron-scattering data for 17 elemental metals. Journal of Physical and Chemical Reference Data. 2009;38:1013–1092. doi: 10.1063/1.3243762. [DOI] [Google Scholar]
- 435.Werner K, et al. Ultrafast mid-infrared high harmonic and supercontinuum generation with n2 characterization in zinc selenide. Optics Express. 2019;27:2867. doi: 10.1364/oe.27.002867. [DOI] [PubMed] [Google Scholar]
- 436.Wettling W, Windscheif J. Elastic constants and refractive index of boron phosphide. Solid State Communications. 1984;50:33–34. doi: 10.1016/0038-1098(84)90053-x. [DOI] [Google Scholar]
- 437.Whang US, Arakawa ET, Callcott TA. Optical properties of Cs for photons of energy 36–96 eV. Journal of the Optical Society of America. 1971;61:740. doi: 10.1364/josa.61.000740. [DOI] [Google Scholar]
- 438.Whang US, Arakawa ET, Callcott TA. Optical properties of K between 4 and 10.7 eV and comparison with Na, Rb, and Cs. Physical Review B. 1972;6:2109–2118. doi: 10.1103/physrevb.6.2109. [DOI] [Google Scholar]
- 439.Whang US, Arakawa ET, Callcott TA. Optical properties of Rb between 3.3 and 10.5 eV. Physical Review B. 1972;5:2118–2124. doi: 10.1103/physrevb.5.2118. [DOI] [Google Scholar]
- 440.White WT, Smith WL, Milam D. Direct measurement of the nonlinear refractive-index coefficient γ at 355 nm in fused silica and in BK-10 glass. Optics Letters. 1984;9:10. doi: 10.1364/ol.9.000010. [DOI] [PubMed] [Google Scholar]
- 441.Williams PA, et al. Optical, thermo-optic, electro-optic, and photoelastic properties of bismuth germanate (Bi4Ge3O12) Applied Optics. 1996;35:3562. doi: 10.1364/ao.35.003562. [DOI] [PubMed] [Google Scholar]
- 442.Windt DL, et al. Optical constants for thin films of Ti, Zr, Nb, Mo, Ru, Rh, Pd, Ag, Hf, Ta, W, Re, Ir, Os, Pt, and Au from 24 Å to 1216 Å. Applied Optics. 1988;27:246. doi: 10.1364/ao.27.000246. [DOI] [PubMed] [Google Scholar]
- 443.Wood DL, Nassau K. Refractive index of cubic zirconia stabilized with yttria. Applied Optics. 1982;21:2978. doi: 10.1364/ao.21.002978. [DOI] [PubMed] [Google Scholar]
- 444.Wood DL, Nassau K, Kometani TY, Nash DL. Optical properties of cubic hafnia stabilized with yttria. Applied Optics. 1990;29:604. doi: 10.1364/ao.29.000604. [DOI] [PubMed] [Google Scholar]
- 445.Woods BW, Payne SA, Marion JE, Hughes RS, Davis LE. Thermomechanical and thermo-optical properties of the LiCaAlF. Journal of the Optical Society of America B. 1991;8:970. doi: 10.1364/josab.8.000970. [DOI] [Google Scholar]
- 446.Wu S-T. Refractive index dispersions of liquid crystals. Optical Engineering. 1993;32:1775. doi: 10.1117/12.143988. [DOI] [Google Scholar]
- 447.Wu Y, et al. Intrinsic optical properties and enhanced plasmonic response of epitaxial silver. Advanced Materials. 2014;26:6106–6110. doi: 10.1002/adma.201401474. [DOI] [PubMed] [Google Scholar]
- 448.Yakubovsky DI, Arsenin AV, Stebunov YV, Fedyanin DY, Volkov VS. Optical constants and structural properties of thin gold films. Optics Express. 2017;25:25574. doi: 10.1364/oe.25.025574. [DOI] [PubMed] [Google Scholar]
- 449.Yakubovsky, D. I. et al. Ultrathin and ultrasmooth gold films on monolayer MoS2. Advanced Materials Interfaces6, 10.1002/admi.201900196 (2019).
- 450.Yamaguchi S, Hanyu T. Optical properties of potassium. Journal of the Physical Society of Japan. 1971;31:1431–1441. doi: 10.1143/jpsj.31.1431. [DOI] [Google Scholar]
- 451.Yamaguchi S, Hanyu T. The optical properties of Rb. Journal of the Physical Society of Japan. 1973;35:1371–1377. doi: 10.1143/jpsj.35.1371. [DOI] [Google Scholar]
- 452.Yang, H. U. et al. Optical dielectric function of silver. Physical Review B91, 10.1103/physrevb.91.235137 (2015).
- 453.Yao C, Shen W, Hu X, Hu C. Optical properties of large-size and damage-free polished Lu2O3 single crystal covering the ultraviolet-visible-and near-infrared (UV–VIS–NIR) spectral region. Journal of Alloys and Compounds. 2022;897:162726. doi: 10.1016/j.jallcom.2021.162726. [DOI] [Google Scholar]
- 454.Yim C, et al. Investigation of the optical properties of MoS2 thin films using spectroscopic ellipsometry. Applied Physics Letters. 2014;104:103114. doi: 10.1063/1.4868108. [DOI] [Google Scholar]
- 455.Zahedpour S, Wahlstrand JK, Milchberg HM. Measurement of the nonlinear refractive index of air constituents at mid-infrared wavelengths. Optics Letters. 2015;40:5794. doi: 10.1364/ol.40.005794. [DOI] [PubMed] [Google Scholar]
- 456.Zelmon DE, Small DL, Jundt D. Infrared corrected Sellmeier coefficients for congruently grown lithium niobate and 5 mol% magnesium oxide-doped lithium niobate. Journal of the Optical Society of America B. 1997;14:3319. doi: 10.1364/josab.14.003319. [DOI] [Google Scholar]
- 457.Zelmon DE, Small DL, Page R. Refractive-index measurements of undoped yttrium aluminum garnet from 04 to 50 μm. Applied Optics. 1998;37:4933. doi: 10.1364/ao.37.004933. [DOI] [PubMed] [Google Scholar]
- 458.Zelmon DE, Hanning EA, Schunemann PG. Refractive-index measurements and Sellmeier coefficients for zinc germanium phosphide from 2 to 9 μm with implications for phase matching in optical frequency-conversion devices. Journal of the Optical Society of America B. 2001;18:1307. doi: 10.1364/josab.18.001307. [DOI] [Google Scholar]
- 459.Zelmon DE, Bayya SS, Sanghera JS, Aggarwal ID. Dispersion of barium gallogermanate glass. Applied Optics. 2002;41:1366. doi: 10.1364/ao.41.001366. [DOI] [PubMed] [Google Scholar]
- 460.Zemel JN, Jensen JD, Schoolar RB. Electrical and optical properties of epitaxial films of PbS, PbSe, PbTe, and SnTe. Physical Review. 1965;140:A330–A342. doi: 10.1103/physrev.140.a330. [DOI] [Google Scholar]
- 461.Zernike F. Refractive indices of ammonium dihydrogen phosphate and potassium dihydrogen phosphate between 2000 Å and 15 μ. Journal of the Optical Society of America. 1964;54:1215. doi: 10.1364/josa.54.001215. [DOI] [Google Scholar]
- 462.Zhang ZM, Lefever-Button G, Powell FR. Infrared refractive index and extinction coefficient of polyimide films. International Journal of Thermophysics. 1998;19:905–916. doi: 10.1023/a:1022655309574. [DOI] [Google Scholar]
- 463.Zhang D, Kong Y, Zhang J-Y. Optical parametric properties of 532-nm-pumped beta-barium-borate near the infrared absorption edge. Optics Communications. 2000;184:485–491. doi: 10.1016/s0030-4018(00)00968-8. [DOI] [Google Scholar]
- 464.Zhang J, Lu ZH, Wang LJ. Precision refractive index measurements of air, N2, O2, Ar, and CO2 with a frequency comb. Applied Optics. 2008;47:3143. doi: 10.1364/ao.47.003143. [DOI] [PubMed] [Google Scholar]
- 465.Zhang, H. et al. Measuring the refractive index of highly crystalline monolayer MoS2 with high confidence. Scientific Reports5, 10.1038/srep08440 (2015). [DOI] [PMC free article] [PubMed]
- 466.Zhang X, Qiu J, Li X, Zhao J, Liu L. Complex refractive indices measurements of polymers in visible and near-infrared bands. Applied Optics. 2020;59:2337. doi: 10.1364/ao.383831. [DOI] [PubMed] [Google Scholar]
- 467.Zhang X, Qiu J, Zhao J, Li X, Liu L. Complex refractive indices measurements of polymers in infrared bands. Journal of Quantitative Spectroscopy and Radiative Transfer. 2020;252:107063. doi: 10.1016/j.jqsrt.2020.107063. [DOI] [Google Scholar]
- 468.Zhang, X. et al. Optimizing the design of the vapor-deposited CsPbCl3-based optoelectronic devices via simulations and experiments. Advanced Functional Materials 2310945, 10.1002/adfm.202310945 (2023).
- 469.Zheng Q, Wang X, Thompson D. Temperature-dependent optical properties of monocrystalline CaF2, BaF2, and MgF2. Optical Materials Express. 2023;13:2380. doi: 10.1364/ome.496246. [DOI] [Google Scholar]
- 470.Zhukovsky, S. V. et al. Experimental demonstration of effective medium approximation breakdown in deeply subwavelength all-dielectric multilayers. Physical Review Letters115, 10.1103/physrevlett.115.177402 (2015). [DOI] [PubMed]
- 471.Zollner S, Lin C, Schönherr E, Böhringer A, Cardona M. The dielectric function of AlSb from 1.4 to 5.8 eV determined by spectroscopic ellipsometry. Journal of Applied Physics. 1989;66:383–387. doi: 10.1063/1.343888. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Citations
- Polyanskiy MN. 2024. refractiveindex.info data set. Figshare. [DOI]
Data Availability Statement
The dataset described here, which represents the core of the refractiveindex.info database, is available at Figshare5. It presently (as of December 2023) contains 3135 data records on 605 materials in the part of the dataset corresponding to linear optical properties (nk), and 193 records on 89 materials in the part corresponding to nonlinear optical properties (n2).
The code that underpins the refractiveindex.info database is made accessible under the Creative Commons Zero (CC0) license (https://creativecommons.org/publicdomain/zero/1.0). This license facilitates the unrestricted use, distribution, and modification of the code, making it widely accessible for various applications. The entire codebase, including detailed documentation, is publicly available on the refractiveindex.info-database GitHub project (https://github.com/polyanskiy/refractiveindex.info-database). This repository is regularly updated, ensuring it evolves to meet the ongoing needs of both the scientific and engineering sectors. For additional utility, users can explore the refractiveindex.info-scripts project on GitHub (https://github.com/polyanskiy/refractiveindex.info-scripts), which offers scripts for deriving optical constants from established models and tools for converting Zemax glass catalogs to the dataset’s YAML format.
It is essential to note that the data encapsulated within the refractiveindex.info dataset is meticulously curated from publicly available sources. This includes peer-reviewed journals, authoritative books, and manufacturer datasheets, ensuring that the dataset is not only expansive but also anchored in reliability and veracity. Each data record within the dataset explicitly cites the source, offering users a pathway to delve deeper into the original data and its context. All journal papers from which data are presently used in the refractiveindex.info dataset, excluding those without a DOI identifier, are included in the following reference list6–471.
By integrating a comprehensive data collection, adopting a standard-based data file format, ensuring ongoing updates, and maintaining open access, the refractiveindex.info emerges as an essential tool for researchers, engineers, and students delving into the complex world of optical constants and material properties.