Carbene Complexes of Plutonium: Structure, Bonding, and Divergent Reactivity to Lanthanide Analogs

Abstract

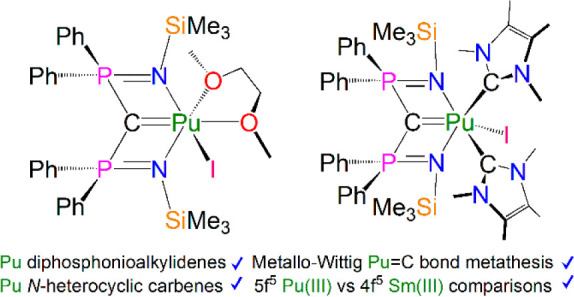
Organoplutonium chemistry was established in 1965, yet structurally authenticated plutonium–carbon bonds remain rare being limited to π-bonded carbocycle and σ-bonded isonitrile and hydrocarbyl derivatives. Thus, plutonium-carbenes, including alkylidenes and N-heterocyclic carbenes (NHCs), are unknown. Here, we report the preparation and characterization of the diphosphoniomethanide-plutonium complex [Pu(BIPM^TMSH)(I)(μ-I)]₂ (1Pu, BIPM^TMSH = (Me₃SiNPPh₂)₂CH) and the diphosphonioalkylidene-plutonium complexes [Pu(BIPM^TMS)(I)(DME)] (2Pu, BIPM^TMS = (Me₃SiNPPh₂)₂C) and [Pu(BIPM^TMS)(I)(I^Me4)₂] (3Pu, I^Me4 = C(NMeCMe)₂), thus disclosing non-actinyl transneptunium multiple bonds and transneptunium NHC complexes. These Pu–C double and dative bonds, along with cerium, praseodymium, samarium, uranium, and neptunium congeners, enable lanthanide–actinide and actinide–actinide comparisons between metals with similar ionic radii and isoelectronic 4f⁵ vs 5f⁵ electron-counts within conserved ligand fields over 12 complexes. Quantum chemical calculations reveal that the orbital-energy and spatial-overlap terms increase from uranium to neptunium; however, on moving to plutonium the orbital-energy matching improves but the spatial overlap decreases. The bonding picture that emerges is more complex than the traditional picture of the bonding of lanthanides being ionic and early actinides being more covalent but becoming more ionic left to right. Multiconfigurational calculations on 2M and 3M (M = Pu, Sm) account for the considerably more complex UV/vis/NIR spectra for 5f⁵2Pu and 3Pu compared to 4f⁵2Sm and 3Sm. Supporting the presence of Pu=C double bonds in 2Pu and 3Pu, 2Pu exhibits metallo-Wittig bond metathesis involving the highest atomic number element to date, reacting with benzaldehyde to produce the alkene PhC(H)=C(PPh₂NSiMe₃)₂ (4) and “PuOI”. In contrast, 2Ce and 2Pr do not react with benzaldehyde to produce 4.

Introduction

Plutonium (Pu) is the heaviest terrestrial element to occur naturally, though it does so in such small quantities that it is usually regarded as a synthetic element.¹ While Pu can be viewed as one of the most complex elements in the periodic table and in need of being better understood, investigations into its fundamental properties have been curtailed due to a requirement for specialist handling facilities that satisfy the safety, security, and stewardship challenges associated with this element.² Historically, these restrictions have been worked around by using synthetic surrogates, for example, using lanthanide ions with similar ionic radii or f-electron count or by studying uranium (U) as a close neighbor. However, as synthetic actinide chemistry has developed,³⁻⁷ it has become clear that there is no accurate surrogate for working with Pu itself given that its substantial relativistic effects cannot be simulated by another element.⁸ Adding further complication to researching Pu is the fact that there is not any metal with the same ionic radius and f-electron count with which to make accurate comparisons. Also, the greater effective nuclear charge at Pu compared to U means their redox chemistries are often significantly diverged.^1,2,5

Where nonaqueous Pu chemistry is concerned,⁸⁻¹⁰ while the area has developed steadily, it is particularly poorly developed with respect to structurally validated complexes. While the first organo-Pu complex, [Pu(C₅H₅)₃], was first reported in 1965,¹¹ this compound was only structurally authenticated, as [(η⁵-C₅H₅)₂Pu(μ-η¹:η⁵-C₅H₅)]_n, in 2018,¹² although [Pu{η⁵-C₅H₃(SiMe₃)₂}₃] ¹³ and [K(2.2.2-cryptand)][Pu{η⁵-C₅H₃(SiMe₃)₂}₃] ¹³ were reported in 2017. Subsequently, [K(2.2.2-cryptand)][(η⁵-C₅H₄SiMe₃)₃Pu(η¹-C₅H₄SiMe₃)],¹⁴ [(η⁵-C₅Me₅)₂PuI(THF)],¹⁵ [(η⁵-C₅H₅)₃Pu(CNCy)],¹⁶ [{η⁵-P(CMeCMe)₂}₂Pu(μ-η⁶-CH₂C₆H₅)₂K],¹⁷ and [{Pu(η⁵-C₅H₄SiMe₃)₃}₂(4,4′-bipy)]¹⁸ emerged between 2020 and 2023. Following the report of the structure of uranocene in 1969,¹⁹ [Pu(η⁸-C₈H₈)₂] appeared in 1970,²⁰ though it was not structurally characterized until 2020.¹⁴ However, substituted and reduced derivatives including [Pu{η⁸-C₈H₆(SiMe₃)₂}₂] ²¹ and [K(2.2.2-cryptand)][Pu(η⁸-C₈H₈)₂] ¹⁴ were reported in 2017 and 2020, respectively, with partial X-ray diffraction data reported for [K(L)][Pu(η⁸-C₈H₈)₂] (L = diglyme or (THF)₂)²² in 1974. The only structurally characterized Pu-arene complex is [{C₆H₄-1,4-(C₆H₄-2-NDipp)₂}PuI(THF)] (Dipp = 2,6-diisopropylphenyl)²³ reported in 2023. Though there are prior reports of Pu-alkyls being prepared, for example, [Pu{CH(SiMe₃)₂}₃],²⁴ the only structurally authenticated Pu–C σ-bonds are in the aforementioned η¹-ligated complexes [{η⁵-P(CMeCMe)₂}₂Pu(μ-η⁶-CH₂C₆H₅)₂K],¹⁷ [K(2.2.2-cryptand)][(η⁵-C₅H₄SiMe₃)₃Pu(η¹-C₅H₄SiMe₃)],¹⁴ and [(η⁵-C₅H₅)₂Pu(μ-η¹:η⁵-C₅H₅)]_n ¹² and the isonitrile complex [(η⁵-C₅H₅)₃Pu(CNCy)].¹⁶ It is hence the case that unambiguously structurally authenticated organo-Pu complexes that provide metal–ligand bond metrics all date from 2017 onward, which stands in contrast to the analogous U and neptunium (Np) organometallics whose structural chemistry spans six and four decades, respectively.⁵⁻¹⁰

The preceding survey emphasizes the dominance of multihapto π-bonded ligands in organo-Pu chemistry.¹⁰ There are only four formally σ-bonded complexes to date,^12,14,16,17 and each of those is derived from potentially η^n>1-ligands. It hence follows that there are no Pu–C multiple (alkylidene, Fischer carbene) or dative N-heterocyclic carbene (NHC) bonds, despite the mature nature of carbene chemistry generally. Indeed, where Pu-multiple bonding is concerned more widely, the literature is dominated by plutonyl, PuO₂ⁿ⁺, which until recently was mirrored in Np chemistry that was dominated by neptunyl, NpO₂ⁿ⁺, and a neptunyl-like bis(imido),²⁵ until reports of a Np-mono(oxo)²⁶ and diphosphonioalkylidene and NHC complexes of Np ²⁷ emerged in 2022. Given that uranium phosphonio-, arsonio-, diphosphonio-, and phosphino-silyl-alkylidene and allenylidene chemistry has grown over four decades,^{3−7,28−46} and that we recently showed that the disphosphoniomethanide {(Me₃SiNPPh₂)₂CH}^1– ((BIPM^TMSH)^1–), diphosphonioalkylidene {(Me₃SiNPPh₂)₂C}^2– ((BIPM^TMS)^2–), and NHC C(NMeCMe)₂ (I^Me4) ligands were effective at producing rare Np-methanides and the first transuranium-carbon double and Np–C dative bonds,²⁷ we turned our attention to establishing Pu congeners.

Here, we report the preparation of a diphosphoniomethanide-Pu complex along with two diphosphonioalkylidene-Pu complexes, one as a 1,2-dimethoxyethane adduct and the other as a bis(I^Me4) adduct, thus disclosing the first non-actinyl transneptunium multiple bonds and a transneptunium NHC complex. These Pu=C double and Pu–C dative bonds, realized after six decades of organo-Pu endeavor, along with new (Pr and Sm) and previously reported (U, Np, and Ce) diphosphonioalkyldiene complexes provide an opportunity to make lanthanide–actinide and actinide–actinide comparisons between metals with similar ionic radii and isoelectronic 4f⁵ vs 5f⁵ electron-counts within a conserved ligand field over 12 complexes. From this, we elucidate the variance of f- and d-orbital contributions to these M=C double bonds, permitting insight into orbital energy vs spatial overlap components that define the covalency of these M=C bonds, and also to probe the relative magnitudes of interelectronic repulsion (IER), spin–orbit coupling (SOC), and crystal field (CF) effects.

Results and Discussion

Synthesis and Reactivity

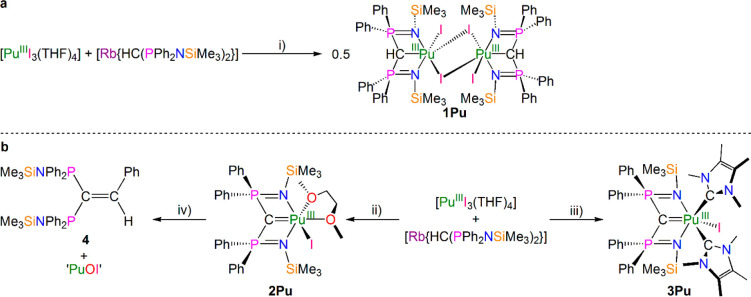
Our prior work preparing Np-carbenes (diphosphonioalkylidenes and NHCs) emphasized the importance of prechoregraphed, multistep “one-pot” reactions,²⁷ a consequence of the small scale of reactions mandated by stewardship of our transuranium stocks, and also the radiological restrictions associated with working in this study with ²³⁹Pu. That work also demonstrated the necessity of avoiding occluded LiCl and the tendency of transuranium ions to increasingly favor the trivalent state as the 5f-block is traversed left to right, a situation even more pronounced for Pu compared to Np.^1,2,5,8−10 Hence, we selected [PuI₃(THF)₄] ^15,47 and [Rb(BIPM^TMSH)] ⁴⁸ as the most eligible starting materials from which to construct Pu-carbene linkages.

In our initial attempt to prepare a Pu=C double bond directly, [PuI₃(THF)₄] was treated with 1 equiv of [Rb(BIPM^TMSH)] in THF, with subsequent DME and solid benzyl potassium addition, Scheme 1a. Following multiple filtrations to remove pale amorphous solids, recrystallization from benzene afforded growth conditions for a small quantity of teal crystals of [Pu(BIPM^TMSH)(I)(μ-I)]₂·2Benzene (1Pu·2Benz) (11% crystalline yield). During our work preparing Np-carbene complexes, we found that use of [Rb(BIPM^TMSH)] that has been previously exposed to the transuranium glovebox He-atmosphere often resulted in the isolation of products containing (BIPM^TMSH)^1– diphosphoniomethanide even when the reaction conditions had been designed to effect full deprotonation and produce (BIPM^TMS)^2– diphosphonioalkylidene. Since other reactions with air- and moisture-sensitive reagents proceeded as expected in the transuranium glovebox (with acceptable levels of O₂ and H₂O in the atmosphere, and solvents verified by [Ti(Cp)₂(μ-Cl)]₂ and sodium benzophenone ketyl tests, respectively), we speculated that the [Rb(BIPM^TMSH)] reagent was being affected by residual solvent vapors in the transuranium glovebox atmosphere; safety constraints imposed by the negative-pressure glovebox mode of operation prevented the atmosphere from being effectively purged of residual solvent vapors.

Scheme 1. Reactions Producing the Organoplutonium Complexes 1Pu-3Pu and the Wittig Alkene Product 4.

(a) Reaction producing 1Pu. Reagents and conditions: (i) THF, DME and benzyl potassium, DME/toluene, several filtration steps, benzene recrystallization (-RbI). (b) Reactions producing 2Pu, 3Pu, and 4. Reagents and conditions: (ii) THF, DME and benzyl potassium, DME/toluene recrystallization (-KI); (iii) THF, DME and benzyl potassium, DME and I^Me4, THF/DME recrystallization (-KI); (iv) C₆D₆, PhCHO (-DME). The different outcomes between (i) and (ii) emphasize the importance of using fresh reagents to synthesize transuranium complexes in a small-scale regime.

To avoid the formation of diphosphoniomethanide products, flame-sealed tubes of fresh [Rb(BIPM^TMSH)] were introduced to the glovebox then opened and used immediately to minimize glovebox solvent/atmospheric exposure. Gratifyingly, this strategy was effective. Treatment of [PuI₃(THF)₄] in THF with portion-wise addition of 1 equiv of [Rb(BIPM^TMSH)], followed by 1 equiv of benzyl potassium and DME afforded, after workup and recrystallization from a toluene/DME solution stored at −35 °C, green crystals of [Pu(BIPM^TMS)(I)(DME)]·0.5Toluene (2Pu·0.5Tol) in 60% yield, Scheme 1b. This successful and consistently reproducible isolation, and yield, of the intended product with doubly deprotonated (BIPM^TMS)^2– diphosphonioalkylidene serves to highlight the nonroutine synthetic challenges and practical constraints associated with small-scale transuranium reactions.

Having established a reliable methodology, and seeking to expand the range of Pu-BIPM^TMS complexes, we targeted an NHC adduct. Accordingly, we prepared 2Puin situ and then treated it with 1.75 equiv of I^Me4;⁴⁹ the use of slightly less than two equivalents of I^Me4 was in anticipation of the formation of 2Pu not being quantitative and also to ensure reproducibility by avoiding the formation of imidazolium byproducts. After workup and recrystallization from warm THF solution, green-yellow blocks [Pu(BIPM^TMS)(I)(I^Me4)₂]·0.5Toluene (3Pu·0.5Tol) were isolated in 28% yield, Scheme 1b.

In our prior report of Np-carbene complexes,²⁷ we showed that [Np(BIPM^TMS)(I)(DME)] (2Np) exhibits metallo-Wittig reactivity with PhCHO, affording the alkene PhC(H)=C(PPh₂NSiMe₃)₂ (4),⁵⁰ as evidenced by ¹H and ³¹P NMR data, and “NpOI”. We were interested to establish whether the Pu=C bond is also capable of effecting metallo-Wittig chemistry and so treated 2Pu with PhCHO. Indeed, metallo-Wittig reactivity of 2Pu was found, Scheme 1b, with the characteristic ¹H and ³¹P NMR spectroscopic data for 4 being observed (Figures S20–S22). This reactivity is consistent with a complex formally containing a Pu=C bond, with multiple bond metathesis presumably producing “PuOI”, though this was not confirmed due to the radiological nature of the work. To benchmark the metallo-Wittig reactivity of trivalent 2Pu (and 2Np), we examined the reactivity of the trivalent complexes [M(BIPM^TMS)(I)(DME)] (M = Ce, 2Ce;²⁷ M = Pr, 2Pr, see below) with PhCHO. Under the same reaction conditions as for 2Pu, 2Ce and 2Pr do not produce the alkene 4. Instead, ³¹P NMR spectroscopy (Figures S39 and S40) reveals a complex mixture of products that is consistent with the formation of previously observed methanide species.⁵¹ The production of methanides in the reactions of 2Ce and 2Pr with PhCHO is consistent with prior work with the closely related trivalent complex [Y(BIPM^TMS)(I)(THF)₂] that readily engages in C–H activation reactions with a range of ketones.⁵² However, a tetravalent Ce-BIPM^TMS complex,⁵³ which contains a covalent Ce=C double bond corroborated by a chemical shift anisotropy NMR spectroscopy study,⁵⁴ does engage in metallo-Wittig chemistry.⁵³ These observations illustrate an experimental divergence between the reactivity of trivalent Ln=C double bonds⁵² and Ce(IV)– and actinide–carbon double bonds which all execute metallo-Wittig chemistry with ketones and aldehydes, including PhCHO.^{27,33−35,50,55} This hints at macrolevel differences in reactivity that might relate to differing covalency between lanthanide(III)–carbon and lanthanide(IV)– and actinide(III/IV/V/VI)–carbon multiple bonds in this instance.

We previously reported the Ce and Np analogs of 2Pu and 3Pu, namely, 2Ce, 3Ce, 2Np, and 3Np,²⁷ and for this study extended that to include the synthesis of the Pr and Sm congeners 2Pr, 3Pr, 2Sm, and 3Sm (see Supporting Information for further details of all complexes); the 4f¹ Ce(III), 4f² Pr(III), and 5f⁴ Np(III) complexes provide similarly sized lanthanide and actinide analogs for bond length comparisons to 2Pu and 3Pu, and the 4f⁵2Sm and 3Sm complexes provide isoelectronic comparisons to 5f⁵2Pu and 3Pu, respectively. As part of the Pr and Sm work we also prepared the methanide derivatives [M(BIPM^TMSH)(I)₂(THF)] (1M.THF) and [M(BIPM^TMSH)(I)₂(I^Me4)] (1M.IMe4) (M = Pr, Sm); however, since it was not practicable to prepare the Pu-congeners of these complexes, the methanide complexes are not specifically discussed in detail (see Supporting Information for further details). They do, however, provide validation of the formulations of 2M and 3M (M = Pr, Sm). Hence, within the context of the previously reported Np and Ce analogs of 2M and 3M, alongside computational modeling of the U-congeners, this presents an opportunity to evaluate 2M and 3M as a function of actinide and lanthanide identity.

Solid-State Structures

The solid-state structures of 1Pu-3Pu, 2Pr, 2Sm, 3Pr, and 3Sm were determined, and selected metrical values and comparisons are summarized in Table 1 along with the corresponding values for 2Np, 3Np, 2Ce, and 3Ce. Further crystallographic data including those of 1M.THF and 1M.IMe4 can be found in the Supporting Information (Tables S1–S4).

Table 1. Selected Solid-State Metrical and Key IR M=C Vibrational Data for 1Pu, 2M, and 3M (M = Np, Pu, Ce, Pr, Sm).

entrya (CN6 r, Å)	M–CBIPM (Å)	ΔM/Pub (Å)	M–CIMe4 (Å)	ΔM/Pub (Å)	M–N (Å)	ΔM/Pub (Å)	M–I (Å)	ΔM/Pub (Å)	M–O (Å)	ΔM/Pub (Å)	M=Cc (cm–1)	M=Cd (cm–1)
1Pu	2.732(4)				2.361(3)		3.0249(3)				f	f
(1.00)					2.351(3)		3.1568(3)				f	f
							3.2173(3)
2Npe	2.425(7)	0.003			2.414(6)	0.012	3.1065(5)	0.0016	2.524(5)	–0.018	508	f
(1.01)					2.431(6)	0.014			2.636(5)	–0.005	448	f
2Pu	2.422(6)				2.402(5)		3.1049(5)		2.542(4)		487	487
(1.00)					2.413(5)				2.641(4)		446	445
2Cee	2.477(2)	0.055			2.456(2)	0.054	3.1752(2)	0.0703	2.570(2)	0.028	463	464
(1.01)					2.459(2)	0.046			2.676(2)	0.035	441	441
2Pr	2.448(5)	0.026			2.416(4)	0.014	3.1333(5)	0.0284	2.533(5)	–0.009	470	475
(0.99)					2.422(4)	0.009			2.612(4)	–0.029	442	438
2Sm	2.381(4)	–0.041			2.387(3)	–0.015	3.0919(3)	–0.0130	2.472(3)	–0.070	463	448
(0.958)					2.396(3)	–0.017			2.548(3)	–0.093	422	415
3Npe	2.490(6)	0.013	2.677(5)	0.014	2.485(4)	0.007	3.1571(4)	0.0067			473	f
(1.01)			2.751(6)	0.012	2.492(5)	0.003					428	f
3Pu	2.477(4)		2.663(5)		2.478(4)		3.1504(4)				472	471
(1.00)			2.739(4)		2.489(4)						426	424
3Cee	2.519(2)	0.042	2.737(3)	0.074	2.494(2)	0.016	3.2054(2)	0.0550			474	469
(1.01)			2.806(2)	0.067	2.510(2)	0.021					430	433
3Pr	2.492(3)	0.015	2.723(3)	0.060	2.475(2)	–0.003	3.1796(2)	0.0292			473	467
(0.99)			2.784(3)	0.045	2.498(2)	0.009					428	423
3Sm	2.444(3)	–0.033	2.667(3)	0.004	2.448(3)	–0.030	3.1329(2)	–0.0175			469	464
(0.958)			2.738(3)	–0.001	2.462(3)	–0.027					412	411

^aThe metal radii in parentheses are the revised ionic radii reported by Shannon for the metal ions with a coordination number of 6.⁶⁰

^bComparison of the respective lanthanide or Np bond length to the Pu metric; positive Δ values indicate shorter bonds in the Pu complex, whereas negative Δ values indicate that the bond is shorter in the lanthanide or Np complex.

^cKey computed M=C vibrations.

^dKey experimentally observed IR bands assigned as M=C vibrations.

^eData for the Np and Ce complexes were reported previously.²⁷

^fData not available or applicable. There is no comparison of the 1Pu metrics because it has no isostructural lanthanide or Np analog.

Complex 1Pu, Figure 1, crystallizes as a halide-bridged dimer; each Pu(III) ion is chelated by a (BIPM^TMSH)^1– ligand, bound in a tridentate manner, and is further coordinated by one terminal iodide and two bridging iodides. Each Pu(III) ion is thus six-coordinate, adopting a distorted octahedral geometry. The P–C_BIPMH–P angle of 135.8(2)° is typical of the “open book”⁵¹ conformation of (BIPM^TMSH)^1–. The Pu–C_BIPMH distance in 1Pu of 2.732(4) Å is long compared to the sum of the single bond covalent radii of Pu and C (2.47 Å)⁵⁶ but can be compared to the few examples of crystallographically authenticated formal Pu–C σ-bonds that include [K(2.2.2-cryptand)][(η⁵-C₅H₄SiMe₃)₃Pu(η¹-C₅H₄SiMe₃)] (2.740(5) Å),¹⁴ [(η⁵-C₅H₅)₂Pu(μ-η¹:η⁵-C₅H₅)]_n (2.830(12) and 2.888(12) Å),¹² [(η⁵-C₅H₅)₃Pu(CNCy)] (2.58(3) Å),¹⁶ and [{η⁵-P(CMeCMe)₂}₂Pu(μ-η⁶-CH₂C₆H₅)₂K] (2.542(19) and 2.614(19) Å).¹⁷ The iodide-bridged dimeric structure of 1Pu does not have any isostructural trivalent f-element (BIPM^TMSH)^1– complexes for direct comparison, but for dimeric trivalent actinide complexes with (BIPM^TMSH)^1– we previously reported [(BIPM^TMSH)Np(Cl)(μ-Cl)₃Np{(μ-Cl)Li(DME)(OEt₂)}(BIPM^TMSH)],²⁷ which exhibits Np–C_BIPMH distances of 2.831(4) and 2.838(4) Å. That those Np–C_BIPMH distances are ∼0.1 Å longer than the Pu–C_BIPMH distances in 1Pu likely reflects the seven- and six-coordinate natures of the Np and Pu ions in those complexes, respectively. That notion is supported by the Np–C_BIPMH distance of 2.753(7) Å in monomeric, six-coordinate [Np(BIPM^TMSH)(I)₂(I^Me4)] (1Np.IMe4),²⁷ which by the 3σ-criterion is statistically indistinguishable to the Pu–C_(BIPMH) distance in dimeric six-coordinate 1Pu.¹ The terminal Pu–I distance in 1Pu of 3.0249(3) Å is consistent with other Pu(III)–I bonds and can be compared to [(η⁵-C₅Me₅)₂PuI(THF)] (3.0353(7) Å),¹⁵ [PuI₃(THF)₄] (3.0712(6)–3.1295(6) Å),^15,57 [PuI₃(tachMe₃)₂] (tach = 1,3,5=trimethyl-1,3,5-triazacyclohexane, 3.1200(14)–3.1314(12) Å),⁵⁸ [PuI₃(9S₃)(NCMe)₂] (9S₃ = 1,4,7-trithiacyclononane, 3.0530(6)–3.1275(7) Å),⁵⁹ [PuI₃{N(CH₂C₄H₃N₂)₃}(NCMe)] (3.0982(6)–3.1839(6) Å),⁵⁹ and [{C₆H₄-1,4-(C₆H₄-2-NDipp)₂}PuI(THF)] (3.0276(7) Å),²³ while the bridging Pu–I distances of 3.1568(3) and 3.2173(3) Å have no precedent for direct comparison. The Pu–N distances in 1Pu are 2.351(3) and 2.361(3) Å, substantially shorter than the seven-coordinate Np–N distances in [(BIPM^TMSH)Np(Cl)(μ-Cl)₃Np{(μ-Cl)Li(DME)(OEt₂)}(BIPM^TMSH)] (2.451(3)–2.473(4) Å) and 1M.IMe4 (M = Np, 2.423(6), 2.458(6); M = Ce, 2.448(6), 2.493(6); M = Pr, 2.430(9), 2.476(9) Å),²⁷ but closer to the Pr–N distances in 1Pr.THF (2.376(3), 2.412(3) Å); this suggests that the Pu–N distances in 1Pu are short owing to the six-coordinate nature of the Pu ions combined with the fact two of the three coordinated iodides per Pu center are bridging.

Figure 1.

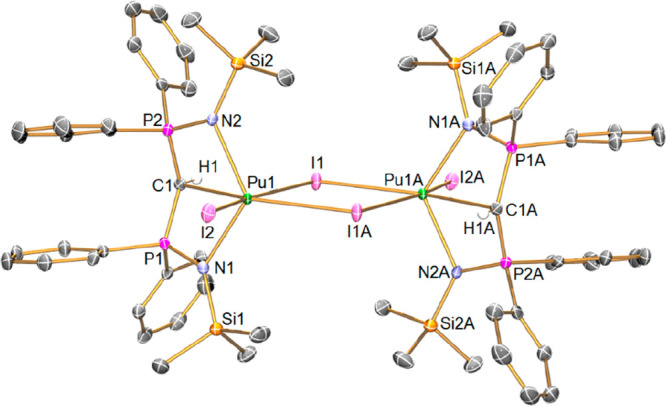
Solid-state molecular structure of 1Pu at 150 K. Displacement ellipsoids are set at 40% probability, and non-methanide H atoms and lattice solvent are omitted for clarity.

Complex 2Pu, Figure 2, is isomorphous to 2Np, and is monomeric. The Pu(III) ion adopts a distorted octahedral geometry, being chelated by a (BIPM^TMS)^2– ligand, bound in a tridentate manner, and further coordinated by one terminal iodide and the two oxygen atoms of a chelating DME ligand. The C_BIPM center is effectively trans to one of DME O atoms, with the iodide being trans to the other DME O atom; thus the stronger donors are trans to the weakest donors in the molecule. This also places the iodide cis to the C_BIPM center.⁴² The carbene center in 2Pu adopts a planar (∑∠ = 359.7(4)°) T-shaped geometry with a P–C–P angle of 170.8(4)°, which is statistically indistinguishable from the corresponding angle of 170.4(5)° in 2Np(27) and close to the values of 167.6(4)° for 2Pr and 165.2(2)° for 2Sm. The Pu=C_BIPM distance in 2Pu of 2.422(6) Å is the shortest Pu–C distance of any type to date, the previous being a Pu–CH₂ bond length of 2.542(19) Å in [{η⁵-P(CMeCMe)₂}₂Pu(μ-η⁶-CH₂C₆H₅)₂K],¹⁷ and there are no other Pu=C double bonds reported for comparison. However, by the 3σ-criterion the Pu=C distance in 2Pu is indistinguishable to the Np=C_BIPM distance of 2.425(7) Å in 2Np.²⁷ The six-coordinate ionic radii of Ce(III) and Pr(III) are 1.01 and 0.99 Å,⁶⁰ and hence they are good structural comparison points to Pu(III) (1.00 Å).⁶⁰ We note that the Pr=C_BIPM distance in 2Pr (2.448(2) Å) is indistinguishable to the analogous distance in 2Pu by the 3σ-criterion, but the corresponding 2Ce (2.477(2) Å) distance is clearly longer than would be predicted based on the ionic radii data. The difference for 2Pu vs 2Sm is essentially accounted for by the ionic radii of Pu(III) vs Sm(III) (0.958 Å).⁶⁰ The two P–C_alkylidene distances in 2Pu (1.649(6) and 1.646(6) Å) are statistically the same as those in 2Np, 2Ce, and 2Pr, suggesting that differences in the M=C_BIPM distances may reflect a stronger, more covalent M=C_BIPM double bond interaction in 2Pu compared to 2Ce. The Pu–N distances in 2Pu (2.402(5) and 2.413(5) Å) are indistinguishable from the Np–N distances in 2Np,²⁷ and, as with the M=C_BIPM distances, are indistinguishable from the Pr–N distances in 2Pr, though they are clearly shorter than the Ce–N distances in 2Ce; for the latter this is by more than can be accounted for by ionic radius difference alone. The Pu–I distance of 3.1065(5) Å in 2Pu is unremarkable compared to other Pu(III)–I distances (see above),^15,57−59 though we note that it is indistinguishable from the corresponding Np–I distance in 2Np, yet distinctly shorter than the Ce–I and Pr–I distances in 2Ce an 2Pr. Lastly, the Pu–O distances in 2Pu are indistinguishable or only just distinguishable from the Np–O and Pr–O distances in 2Np and 2Pr, respectively, but clearly shorter than the Ce–O distances in 2Ce.

Figure 2.

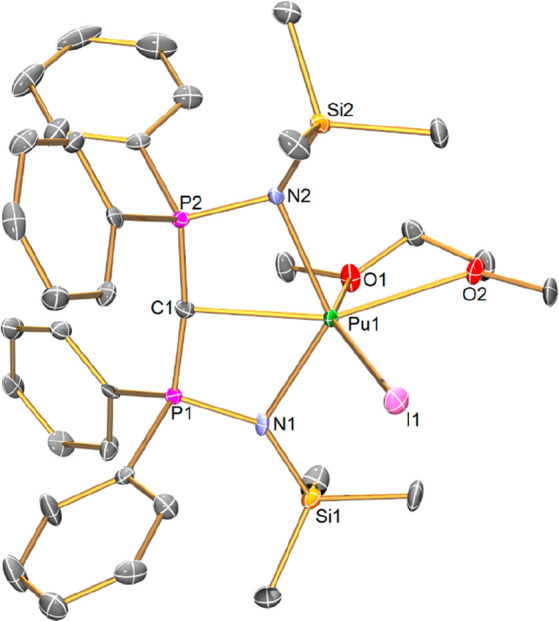
Solid-state molecular structure of 2Pu at 100 K. Displacement ellipsoids are set at 40% probability, and H atoms and lattice solvent are omitted for clarity.

Complex 3Pu, Figure 3, is isomorphous to 3Np and is monomeric. The Pu(III) ion in 3Pu adopts an irregular six-coordinate geometry, being chelated by a (BIPM^TMS)^2– ligand, bound in a tridentate manner, one terminal iodide and two I^Me4 carbene ligands. The (BIPM^TMS)^2– ligand adopts a more “open-book”⁵¹ carbene geometry than its planar conformation in 2Pu, which manifests in the carbene center being pyramidalized (∑∠ = 322.77(19)°) and exhibiting a more bent P–C–P angle of 137.4(3)°. This results in a longer Pu=C_BIPM distance in 3Pu of 2.477(4) Å compared to the corresponding distance in 2Pu; however by the 3σ-criterion it is within the statistical uncertainty of the corresponding Np=C_BIPM distance in 3Np.²⁷ Analogously to the trends found for 2Pu, the Pu=C_BIPM distance is statistically indistinguishable from the corresponding Pr=C_BIPM distance of 2.492(3) Å in 3Pr, but shorter than the Ce=C_BIPM distance of 2.519(2) Å and shorter than anticipated from ionic radii differences alone. The Pu=C_BIPM distances in 2Pu and 3Pu differ by ∼0.05 Å, whereas the corresponding difference for 2Np and 3Np is ∼0.07 Å. The two Pu–C_NHC distances in 3Pu (2.663(5) and 2.739(4) Å) are unprecedented examples of Pu–C_NHC bonds and hence have no other examples to compare to, nonetheless those distances are within the statistical 3σ-variance of the corresponding Np–C_NHC values in 3Np (2.677(5) and 2.751(6) Å).²⁷ However, noting that the 3M–NHC distances come in pairs of one short and one long, the Pu–C_NHC distances in 3Pu are like-for-like clearly shorter than the corresponding Ce–C_NHC (2.737(3) and 2.806(2) Å) and Pr–C_NHC (2.723(3) and 2.784(3) Å) metrics in 3Ce and 3Pr. Interestingly, the Pu–N_NHC distances are statistically indistinguishable from the corresponding Sm–C_NHC distances in 3Sm (2.667(3) and 2.738(3) Å), despite the smaller size of Sm(III) compared to Pu(III),⁶⁰ and when these data are taken together they suggest that the Pu–C_NHC bonds may be more covalent than the M–C_NHC (M = Ce, Pr, Sm) bonds. The Pu–N distances in 3Pu (2.478(4) and 2.489(4) Å) are longer (∼0.07 Å) than the corresponding Pu–N distances in 2Pu, likely reflecting the replacement of DME O-donor atoms with the much more strongly donating C_NHC centers. There is little statistically meaningful variance of the Pu–N distances compared to the corresponding Np–N, Ce–N, and Pr–N bonds, and indeed the Sm–N bonds are only marginally shorter from a 3σ-criterion perspective. The Pu–I distance in 3Pu is 3.1504(4) Å, which is ∼0.05 Å longer than the corresponding Pu–I distance in 2Pu, again likely reflecting the replacement of DME O-donors with strongly donating C_NHC donors. Consistent with the above trends, the Pu–I distance in 3Pu is indistinguishable to the corresponding Np–I distance in 3Np ²⁷ but shorter than the Ce–I and Pr–I distances in 3Ce and 3Pr than ionic radii considerations would predict. In passing, we note that the Ce–I bonds differ by ∼0.03–0.04 Å between 2Ce and 3Ce; nonetheless the Pu–I and Np–I distances consistently vary by ∼0.05 Å.

Figure 3.

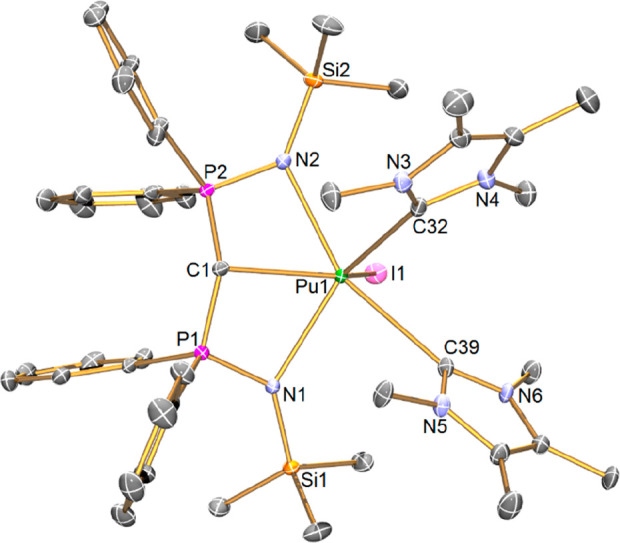
Solid-state molecular structure of 3Pu at 100 K. Displacement ellipsoids are set at 40% probability, H atoms and lattice solvent are omitted for clarity.

Overall, while it is clear that the metrical data for Np and Pu are often quite similar, for a given 2M or 3M comparison, the bonds to Pu are often shorter than would be anticipated for lanthanide congeners based on their respective ionic radii. These data suggest that simple ionic models that adjust solely for ionic radii are not appropriate on their own. While more data are certainly needed, this highlights a limitation associated with using ionic radii to make transuranium actinide–ligand bond distance predictions.

Spectroscopic Analysis

The ¹H NMR spectra of 1Pu-3Pu (Figures S12–S19) span the ranges −7 to 8, −1 to 8, and −2 to 8 ppm, respectively. These ranges are surprisingly similar, and for 2Pu and 3Pu are notably narrower chemical shift ranges compared to the chemical shift ranges of 2Np and 3Np (∼25 ppm, which also in appearance exhibit qualitatively broader resonances)²⁷ reflecting the 5f⁵ nature of Pu(III) compared to 5f⁴ Np(III). The ³¹P{¹H} chemical shifts of 1Pu-3Pu are −83.4, −128.4, and −141.9 ppm, respectively, and while the value for 1Pu is similar to 1Np.IMe4 (δ_31P −54.6 ppm),²⁷ the values for 2Pu and 3Pu are rather different to 2Np and 3Np (δ_31P −789 and −740 ppm, respectively),²⁷ reflecting the different 5fⁿ counts of Np and Pu. However, we note that conversion of (BIPM^TMSH)^1– to (BIPM^TMS)^2– results in shifts to lower frequencies for both Np- and Pu-BIPM^TMS complexes.

The Evans method effective magnetic moments of 1Pu-3Pu were determined (Table S5), yielding values of 3.65, 1.35, and 0.90 μ_B per molecule, respectively, which, noting that 1Pu is dinuclear (1.83 μ_B per Pu ion), are in fair agreement with the Evans method effective magnetic moments of mononuclear [PuI₃(THF)₄] (1.17 μ_B) and [(η⁵-C₅Me₅)₂PuI(THF)] (0.97 μ_B).¹⁵ Those values are consistent with the fact that the predicted μ_eff value for ⁶H_5/2 f-element ions is 0.85 μ_B, although magnetic moments of ∼1.4 μ_B are typically found because the ground state is not well-isolated from low-lying paramagnetic states, and the resulting mixing increases the observed effective magnetic moments. We note in passing that the effective magnetic moments are smallest for 3Pu and [(η⁵-C₅Me₅)₂PuI(THF)],¹⁵ which might be predicted to have some of the strongest CFs, but the effective magnetic moments of more complexes need to be obtained to determine whether this constitutes a real trend.

Analytical frequency calculations on geometry optimized models of 2M and 3M (M = Np, Pu, Ce, Pr, Sm), Table 1, reveal for each series two principal vibrations that correspond to M=C stretches. The first, at higher energy, is M=C bond contraction with simultaneous M–N bond lengthening and vice versa, whereas the second, lower energy, stretch is isolated M=C contraction and lengthening without any other significant vibrations in the molecule. The computed values are in good agreement with the experimentally observed IR spectra of 2Pu and 3Pu (Figures S41 and S42), providing confidence in the DFT calculations. For the 3M series the energies of the stretches are very close to each other, likely reflecting the pyramidalized nature of the alkylidene centers, and hence the stretches are less responsive to the nature of the metal. However, larger differences are found for the 2M series, likely reflecting the optimal bonding scenario, and here the differences are significant enough that they can be ordered as Pu > Pr > Ce ∼ Sm. This is in agreement with the bond length data above, and also the bonding analysis that is revealed by the DFT analysis (see below). Unfortunately, experimental IR data for previously reported 2Np and 3Np were not obtained, though being part of respective series there can be confidence in the DFT data for those two complexes. Interestingly the calculated vibrational data for 2Np and 3Np suggest that the Np=C bonds are stronger than the corresponding Pu=C bonds, which is supported by the DFT analysis (see below). Lastly, the IR data suggest that the Pu=C bond in 2Pu is stronger than the analogous bond in 3Pu, in-line with the structural data, binding modes, and DFT bonding analysis.

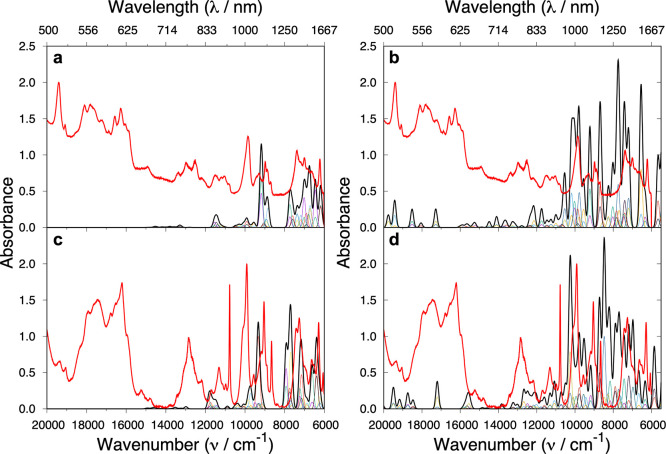
The UV/vis/NIR solution spectra of 1Pu-3Pu, Figure 4, are consistent with their Pu(III) formulations, and exhibit signature f−f transition features in the NIR regions observed for other Pu(III) complexes, for example [PuI₃(THF)₄],¹⁵ [(η⁵-C₅Me₅)₂PuI(THF)],¹⁵ and Pu³⁺ in 1 M perchloric acid solutions.⁶¹ Specifically, Pu(III) complexes tend to exhibit a characteristic pattern of absorptions at ∼19 000 cm^–1, a pair of features centered around a barycenter at ∼17 000 cm^–1, ∼15 000 cm^–1, and several features centered at ∼12 000 cm^–1, ∼9000, and ∼7000 cm^–1; 1Pu-3Pu all exhibit those features with extinction coefficients of 30–120 M^–1 cm^–1. For 1Pu-3Pu, strong absorption bands begin at ∼24 000 cm^–1 and extend well into the UV region. These bands have extinction coefficients of ∼9000 M^–1 cm^–1 for 2Pu and 3Pu and are assigned as LMCT bands; for 1Pu, the broad LMCT band is weaker at ∼3500 M^–1 cm^–1, likely reflecting that 1Pu is a methanide complex compared to the carbene formulations of 2Pu and 3Pu. Compared to U(III) and Np(III), whose f–d transitions tend to occur around 15 000–22 000 cm^–1,⁷ the Laporte allowed f–d transitions of Pu(III) would be expected to occur above 24 000 cm^–1 due to the increasing f–d energy gap as the actinide series is traversed left to right.¹⁵ Indeed, 1Pu-3Pu shoulder absorptions at ∼27 600, 25 500, and 25 000 cm^–1, respectively, with extinction coefficients of 1400–1600 M^–1 cm^–1, are observed, and these are assigned as f–d transitions. As the ligand fields of 1Pu-3Pu change, becoming more electron-rich, the f–d energy gap would, simplistically, be expected to become smaller. Although the bands attributed to f–d transitions for 2Pu and 3Pu are on the edge of strong LMCT bands, it does indeed appear that the f–d bands are energetically ordered 1Pu > 2Pu > 3Pu, though the differences are relatively slight. Where the f–f transitions for 1Pu-3Pu are concerned, each group of features changes quite substantially between the three complexes, so it is not possible to comment on whether the f–f bands are broader or sharper, implying more or less vibronic coupling and covalency, respectively. Since the structure of the f-manifold would be expected to be rather complex in the SOC regime of Pu(III), this is probed with ab initio methods (see below). Lastly, to probe whether the solution UV/vis/NIR data for 2Pu and 3Pu, and hence the solution structures, are representative of the solid-state structures, we collected solid-state UV/vis/NIR spectra on single crystals of 2Pu and 3Pu (Figures S57 and S62); the low yield precluded acquisition of data for 1Pu. For both 2Pu and 3Pu, there is excellent agreement between the solution and solid-state UV/vis/NIR spectra, suggesting that there are no significant electronic changes (and by extension, speciation) upon dissolution of 2Pu or 3Pu in toluene or THF, respectively.

Figure 4.

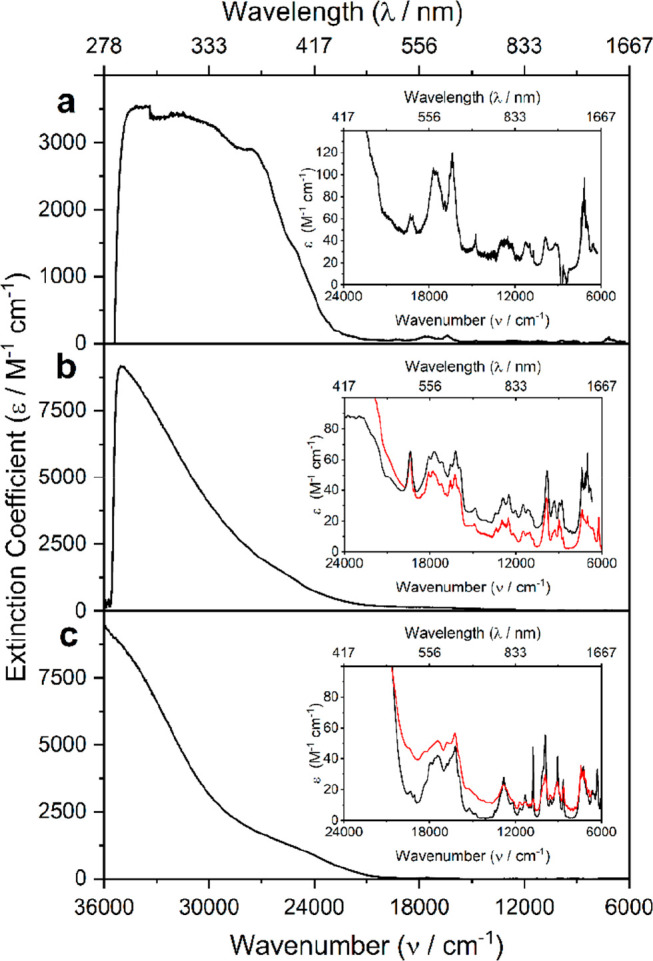
Solution (black) and solid-state (red) UV/vis/NIR spectra for 1Pu-3Pu: (a) 1Pu, (b) 2Pu, (c) 3Pu. The inset of each spectrum highlights the respective vis/NIR region.

Electronic Structure Analysis

Given that 1Pu does not have a structural congener with other f-metals, the following discussion focuses on 2Pu and 3Pu, since these complexes have structurally authenticated Np, Ce, Pr, and Sm analogs. To probe the electronic structures of 2Pu and 3Pu, and the Pr and Sm congeners, DFT calculations were performed (Tables S6–S14). DFT data for 2Np, 2Ce, 3Np, and 3Ce were reported previously²⁷ and are included here to aid comparisons of the Pu, Pr, and Sm calculations (see Supporting Information for full details). We also include the previously calculated data for 2U and 3U;²⁷ while they are not experimentally realized complexes, there can be confidence in their electronic structure analysis because they are internally validated by the Pu, Np, Ce, Pr, and Sm calculations and align with computational studies on experimentally realized uranium(III/IV/V/VI) analogs.^33,34,41 By making these comparisons, Table 2, we relate metals that are of similar ionic radii and make direct isoelectronic 5f⁵–4f⁵ comparisons. In general the optimized gas-phase bond lengths and angles agree well with the experimental solid-state metrics, and the analytical frequency calculations derived from those optimized coordinates are consistent with the experimental IR data. Thus, the calculations can be considered to represent reliable qualitative models of the electronic structures examined.

Table 2. Selected Computed Properties for 2M and 3M (M = U, Np, Pu, Ce, Pr, Sm).

	bond and indices		chargesd		spin densitiese		NBO M–C σ-bond component (%)f			NBO M–C π-bond component (%)f			QTAIMh
entrya	bondb	BIc	M	C	M	C	Mg	Cg	M s/p/d/f	M	C	M s/p/d/f	ρ	ε
2Ui	U=CBIPM	1.28	1.57	–2.00	3.28	–0.04	14	86	4/1/42/53	13	87	0/0/40/60	0.08	0.20
2Npi	Np=CBIPM	1.40	1.54	–1.96	4.36	–0.07	17	83	4/1/32/63	14	86	0/0/38/62	0.08	0.21
2Pu	Pu=CBIPM	0.79	1.48	–1.94	5.40	–0.13	20	80	3/1/26/70	15	85	0/0/36/64	0.08	0.10
2Cei	Ce=CBIPM	1.05	1.32	–1.82	1.07	–0.01	10	90	1/1/61/37	8	92	0/0/65/35	0.07	0.22
2Pr	Pr=CBIPM	0.80	1.30	–1.73	2.15	–0.06	12	88	1/1/48/50	10	90	0/0/49/51	0.07	0.22
2Sm	Sm=CBIPM	0.83	1.17	–1.63	5.56	–0.35	23	77	1/1/18/80	25	75	0/0/16/84	0.07	0.16
3Ui	U=CBIPM	1.17	1.62	–1.67	3.08	–0.04	14	86	10/1/46/43	10	90	0/1/50/49	0.08	0.17
	U←CNHC	0.77		–0.50		–0.03	0	100					0.05	0.03
	U←CNHC	0.81		–0.48		–0.03	0	100					0.05	0.03
3Npi	Np=CBIPM	1.20	1.51	–1.64	4.22	–0.05	15	85	9/1/39/51	10	90	0/1/43/56	0.08	0.18
	Np←CNHC	0.69		–0.46		–0.03	0	100					0.04	0.03
	Np←CNHC	0.65		–0.44		–0.03	0	100					0.05	0.03
3Pu	Pu=CBIPM	0.59	1.38	–1.60	5.26	–0.05	15	85	10/1/36/53	10	90	1/1/39/59	0.07	0.10
	Pu←CNHC	0.33		–0.41		–0.03	0	100					0.05	0.02
	Pu←CNHC	0.28		–0.40		–0.03	0	100					0.04	0.02
3Cei	Ce=CBIPM	0.96	1.29	–1.53	1.01	–0.01	9	91	7/1/65/27	7	93	2/1/60/37	0.07	0.19
	Ce←CNHC	0.52		–0.38		–0.01	0	100					0.04	0.03
	Ce←CNHC	0.58		–0.36		–0.01	0	100					0.04	0.03
3Pr	Pr=CBIPM	0.68	1.19	–1.51	2.13	–0.04	10	90	7/1/57/35	8	92	3/1/56/40	0.07	0.21
	Pr←CNHC	0.35		–0.32		–0.01	0	100					0.04	0.08
	Pr←CNHC	0.31		–0.33		–0.01	0	100					0.04	0.02
3Sm	Sm=CBIPM	0.71	1.06	–1.42	5.50	–0.28	17	83	5/1/27/67	22	78	2/0/14/84	0.06	0.14
	Sm←CNHC	0.26		–0.28		–0.03	0	100					0.04	0.02
	Sm←CNHC	0.23		–0.30		–0.02	0	100					0.03	0.04

^aAll compounds geometry optimized without symmetry constraints at the BP86 TZP/ZORA (all-electron) level.

^bM–C bond: M=C_BIPM = diphosphonioalkylidene of (BIPM^TMS)^2–; M←C_NHC = I^Me4 NHC carbene.

^cNalewajski–Mrozek bond indices.

^dMDC_q charges.

^eMDC_m spin densities. Note that a positive value indicates an excess of spin density and a negative value the loss of spin density.

^fNatural bond orbital (NBO) analysis.

^gValues of 0% for the total M contribution to the M–C bond means the M contribution is below the cutoff threshold of NBO (5%).

^hQuantum theory of atoms in molecules (QTAIM) bond critical point topological electron density (ρ) and ellipticity (ε) analysis.

ⁱData for the Np and Ce complexes were reported previously.²⁷

Addressing 2Pu and 3Pu together, the computed Pu (1.48, 1.38) and C_BIPM (−1.94, −1.60) charges are consistent with the presence of formal Pu(III) ions and (BIPM^TMS)^2– dianions. The computed Pu spin densities (5.40, 5.26) are consistent with 5f⁵ ions with net donation of charge from the ligands, which is corroborated by the negative (−0.13, −0.05) C_BIPM spin densities. Where the Pu=C bonds are concerned, NBO analysis reveals σ- and π-bonds for 2Pu, Figure 5, that are 20 and 15% Pu character, respectively, and within those components 5f- dominates over 6d-contributions (5f/6d: 70/26 and 64/36%). NBO analysis of 3Pu also finds σ- and π-bonds (Figure S81), with 15 and 10% Pu character, and within those components the 5f-character still dominates over 6d-components (5f/6d: 53/36 and 59/39), though less so than in 2Pu. Thus, the NBO analysis clearly reveals the presence of σ- and π-bonding components in 2Pu and 3Pu, although the pyramidalization of the carbene in 3Pu renders the overlap not as efficient as the T-shaped carbene in 2Pu which is optimally aligned for double bonding. Considering the Pu ions in 2Pu and 3Pu are Pu(III), the Pu % contributions to the Pu=C double bonds are significant. However, the Pu=C bond orders (0.79, 0.59) suggest the presence of very polarized double bonds,⁶² that is each bond order component is a low subinteger value. The topological quantum theory of atoms in molecules (QTAIM) data (ρ: 0.08, 0.07; ε: 0.10, 0.10) reveal low bond critical point electron densities (ρ), and the ellipticity (ε) values are only just entering the double bond range. This suggests that the Pu=C bonds in 2Pu and 3Pu should be considered to be borderline double bond interactions rather than full double bonds. Where the Pu–C_IMe4 bonds of 3Pu are concerned, those bonds are evidently highly electrostatic, reflecting the formal dative nature of the carbene to Pu donation, and as such the Pu–C_IMe4 bond orders are low (av ∼0.31).

Figure 5.

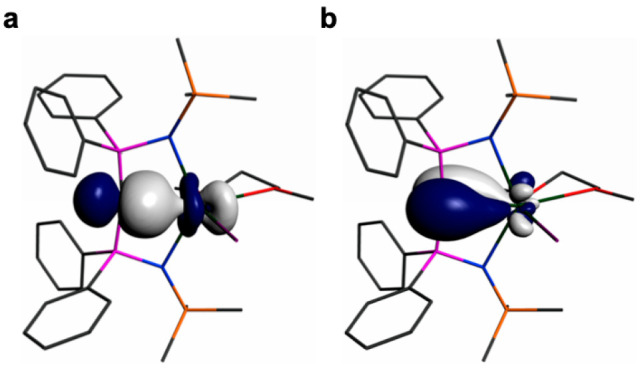
NBO representations of the Pu=C_BIPM bonding interactions in 2Pu: (a) Pu=C_BIPM σ-bond; (b) Pu=C_BIPM π-bond.

Next, it is instructive to compare the 5f members of 2M and 3M (M = U, Np, Pu). The computed charges and spin densities are as would be expected for these 5f³, 5f⁴, and 5f⁵ complexes, respectively, so we focus on the NBO, bond order, and QTAIM metrics. For each 2M and 3M series the bond orders follow the trend Np > U > Pu. In contrast, for the 2M compounds the metal contributions to the M=C bonds is ordered Pu > Np > U for the σ- and π-bonds. The situation is less clear for the 3M series, where for the M=C σ-bonds are ordered Pu ∼ Np > U and the π-bonds are all similar, and this likely reflects that the M=C bonds are not optimally aligned so they are less responsive to the nature of the coordinated metal. However, irrespective of how varied the Pu % contributions are to the M=C bonds in 2M and 3M, it is clear that the 5f/6d compositions vary; specifically, on moving from U to Np to Pu, all the σ- and π-bonds exhibit decreasing 6d-character and corresponding increasing 5f-contributions, reflecting that as the actinide series is traversed left to right the 5f- and 6d-orbitals fall and increase in energy, respectively.⁶³⁻⁶⁸ The QTAIM ρ and ε values show little variation for the 2M and 3M complexes overall, except for the ε values, where according to this topological metric U and Np have better developed double bond interactions compared to Pu.

Extending the above analysis to include the Ce, Pr, and Sm members, for both the 2M and 3M series the Ce=C bond order is less than the corresponding U=C and Np=C bonds but greater than the Pu=C congeners. For the 2M series the Pr=C and Sm=C bond orders are then essentially the same as the Pu=C bond order, and for the 3M series the Pr=C and Sm=C bond orders are slightly larger than the analogous Pu=C bond order. Nevertheless, overall the bond orders can be arranged as Np > U > Ce > Pu ∼ Pr ∼ Sm. However, NBO analysis reveals a more complex picture. For the 2M compounds, the Ce=C and Pr=C have, like-for-like, smaller metal contributions to the σ- and π-components of the M=C bonds than U, Np, and Pu; however, the Sm=C σ- and π-components have the largest metal contributions of all the 2M complexes, with Pu next largest, producing the trend Sm > Pu > Np > U > Pr > Ce. We note that the 4f and 5f metals display parallel trends, that is for M % Pu > Np > U and Sm > Pr > Ce, so the heavier metals have larger M % contributions within a 4f or 5f group. We also note that as found for the 5f metals, the 5d-contributions decrease and the 4f-components increase on moving from Ce to Pr to Sm, which again likely reflects the 4f- and 5d-orbital energies falling and increasing, respectively, left to right in the lanthanide series. The QTAIM ρ values are fairly invariant across the 2M series, being slightly lower for the 4f vs 5f metals, however a clear difference can be found in the ε values, which are substantial, indicating well-developed double bond interactions for U, Np, Ce, and Pr, and lower for Pu and Sm suggesting that those M=C double bond interactions are less well-developed. Turning to the 3M series of complexes, in essence the same patterns are observed, though with less variability due to the suboptimal M=C bonding geometry.

The bonding picture that emerges from the above analysis is clearly more complex than the traditional picture of the bonding of lanthanides are ionic and early actinides being covalent and becoming more ionic left to right. However, it is clear that the more f-character, and less d-component, there is in these M=C bonds the greater the M % contribution is, but conversely the lesser the bond order and bond ellipticity are. Traversing the f-blocks, the 4/5f-orbital energies fall with increasing atomic number and d-orbital energies increase.⁶³⁻⁶⁸ Hence, with respect to the likely carbene energies, the data imply that the orbital energy matching (orbital mixing) increases, and gives greater f-orbital contributions as atomic number increases, however the spatial overlap is reduced. Based on the structural data the implication is that the orbital energy/spatial overlap tension is just tipped to a net increase of covalency for Pu, compared to the lanthanides, and this is consistent with the outcomes of the reactions with PhCHO above, but this is evidently based on superior orbital energy matching but poorer spatial overlap of metal–ligand orbitals. Thus, when considering how covalent the Pu=C bonds are compared to U, Np, Ce, Pr, and Sm analogs it would seem to be that they are most covalent except for Sm on orbital energy matching grounds. When considering just the actinides, it would seem that the orbital energy matching increases moving from U to Np to Pu. However, the spatial overlap increases when moving from U to Np then decreases Np to Pu. The result is then that Np is the most covalent overall.

In order to provide further insight into the electronic structures of 2Pu and 3Pu that are experimentally benchmarked and validated, we turned to modeling the UV/vis/NIR data using complete active space self-consistent field spin–orbit (CASSCF-SO) calculations (Tables S15–S30). Furthermore, since we have experimentally realized 2Sm and 3Sm, and noting that their UV/vis/NIR spectra are significantly simpler than those of 2Pu and 3Pu, this presented an opportunity to directly probe and compare the interplay of CF, SOC, and IER for isostructural 4f⁵ and 5f⁵ complexes. The corresponding calculations for 2Pr and 3Pr were also undertaken to provide further benchmarking of the calculations, though poor solubility prevented reliable experimental UV/vis/NIR data for 3Pr being obtained. In general, absorption features are computed to ∼500 cm^–1 of experiment, and the relative energy spacings are overall reproduced by the calculations.

The f⁵ free-ion configuration for Pu(III) and Sm(III) defines low-lying ⁶H and ⁶F terms, and higher lying ⁴G, ⁴F, ⁴I, etc. terms. For 2Sm, 3Sm, 2Pu, and 3Pu, CASSCF(5,7)-SO calculations give a ⁶H ground term as expected (Tables S18–S23). As both Pu(III) and Sm(III) are Kramers ions, we find doubly degenerate CF states. The ground states for 2Sm, 3Sm, 2Pu, and 3Pu all exhibit m_J mixing owing to their low symmetry, and to compare between compounds we quantize the projection of the total angular momentum along the M-I axis. In 2Sm and 2Pu, the ground states are dominated by |m_J| = 5/2, whereas 3Sm and 3Pu exhibit a much higher degree of mixing in their ground states. The ground state of 3Sm has a 43% contribution from |m_J| = 1/2 and a smaller contribution from |m_J| = 3/2 (25%), while 3Pu has equal 26% contributions from |m_J| = 5/2 from |m_J| = 1/2. As expected from the low symmetry, the ground state g-values are rhombic (Tables S25–S30), affording g-values of g_1,2,3 = 0.07, 0.58, 0.90 and 0.01, 0.26, 0.71 for 2Sm and 3Sm, respectively, and g_1,2,3 = 0.19, 0.47, 0.67 and 0.09, 0.36, 0.55 for 2Pu and 3Pu, respectively (Figures S92 and S93).

In the case of the isoelectronic 4f and 5f ions, it is of considerable interest to compare the relative strengths of IER, SOC, and CF splitting. Here, we define the IER as the energy difference between the barycenters of the ⁶H and ⁶F terms, the SO splitting as the energy difference between the barycenters of the ⁶H_5/2 and ⁶H_7/2 multiplets, and the CF splitting as the total splitting range of the ⁶H_5/2 multiplet. We find the SO and CF splitting for the Pu(III) complexes are approximately double the values for the Sm(III) complexes (Table 3), since there are more significant relativistic effects and larger radial extension of the 5f- vs 4f-orbitals, respectively. However, we find that the IER is approximately 50% larger for Sm(III) than Pu(III): this likely arises as a consequence of the more spatially confined nature of 4f-electrons compared to their 5f counterparts.

Table 3. Interelectron Repulsion (IER), Spin Orbit (SO), and Crystal Field (CF) Splitting (cm^–1) of 2M and 3M (M = Pu, Pr, Sm).

	CASSCF-SO			MS-CASPT2-SO			XMS-CASPT2-SO
entrya	IER	SO	CF	IER	SO	CF	IER	SO	CF
2Sm	6647	874	407	5150	874	306	5741	784	434
2Pu	4499	1687	763	2468	1687	575	3238	1687	770
3Sm	6651	874	538	5226	874	563	5835	874	560
3Pu	4504	1685	968	2616	1685	1002	3371	1685	1166
2Pr	5848	2008	710	4469	2008	612	4694	2008	844
3Pr	5857	2009	834	4437	2009	931	4874	2009	942

^aAll calculations performed at crystal structure geometry.

For Pr(III) the 4f² configuration defines a ground ³H term with a low-lying ³F term, and a proximal ¹G term, while ¹D, ³P, and ¹I terms are much higher in energy; our CASSCF(2,7)-SO calculations considered the lowest three terms, finding a ground ³H₄ multiplet as expected for both 2Pr and 3Pr (Tables 3 and S15–S17). Owing to the non-Kramers nature of the 4f² configuration and the low-symmetry of the complexes, the CF removes the degeneracy of all states in the ³H₄ multiplet.

In all cases, the complexes fall into the same IER > SO > CF regime. Comparing the two ligand sets across all complexes, the I^Me4 ligands in 3M provide a larger CF splitting than the DME ligands in 2M. Addition of CASPT2 corrections generally reduces the effective IER in all cases, reducing the energy of excited states, while the effect on SOC is negligible (as this is determined using the atomic mean-field method), and the effect on CF splitting is varied (Table 3). From comparison to experimental UV/vis/NIR spectra, we find the MS-CASPT2-SO results are most accurate, Figures 6–8, and hence focus on discussion of these data rather than the CASSCF-SO and XMS-CASPT2-SO data (Figures S94–S99).

Figure 6.

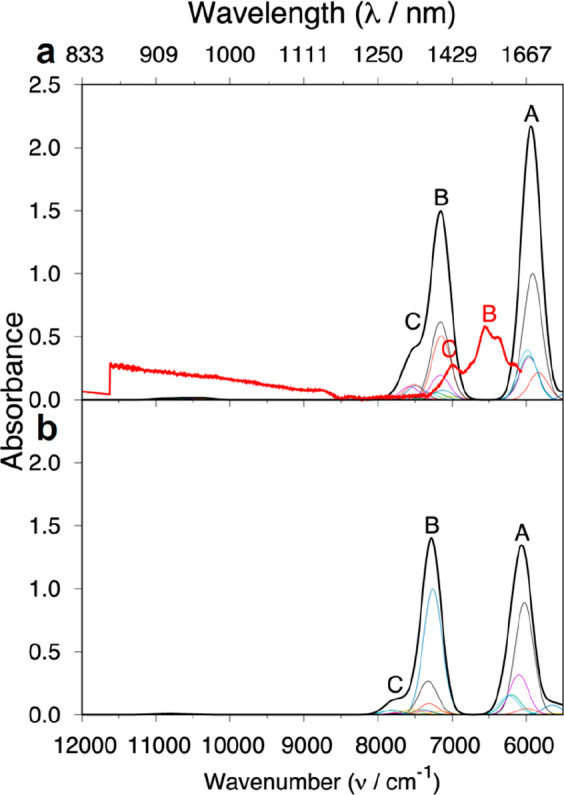
Experimental (red) and computed MS-CASPT2-SO (black) UV/vis/NIR data for 2Pr and 3Pr: (a) 2Pr; (b) 3Pr (no experimental data available). Individual transitions (rainbow, below black) and total absorption spectrum were Gaussian-broadened with a half-width of 250 cm^–1.

Figure 8.

Experimental (red) and computed MS-CASPT2-SO (black) data for 2Pu and 3Pu: (a) 2Pu, (b) 2Pu with inclusion of an additional 16 quartet states, (c) 3Pu, and (d) 3Pu with inclusion of an additional 16 quartet states. Individual transitions (rainbow, below black) and total absorption spectrum were Gaussian-broadened with a half-width of 150 cm^–1.

The experimental UV/vis/NIR spectrum of 2Pr is dominated by absorptions in the 6000–7500 cm^–1 range, consisting of one major peak with several shoulders, Figure 6a. The lowest-energy calculated transition A corresponds to ³H₄ → ³F₂ excitations, which are known to occur at lower energy (∼5000 cm^–1),¹¹ which is out of the range of the experiment. The second calculated peak B corresponds to the main peak observed in the experiment, which arises from ³H₄ → ³F₃ excitations. The fine structure is likely a result of CF splitting of the J = 3 state but could correspond to different conformers in solution or phonon sidebands. The higher energy shoulder C corresponds to transitions with mixed ³F₄ and ¹G₄ final states, which become mixed because they are nearby in energy and have ΔJ = 0 and hence are mixed in first-order by SOC. Although ³F₄ is the major component (49–66% ³F₄ vs 30–42% ¹G₄), the ³H₄ → ¹G₄ transitions are formally spin forbidden (ΔS ≠ 0) and this explains the weaker intensity of this feature. The experimental data shows further features near 16 500 and 20 000 cm^–1 (Figure S67) which likely correspond to the ¹D and ³P terms, respectively.¹² However, we have not attempted to compute these highly excited states as their inclusion in state-averaged CASSCF calculations would negatively affect the quality of the lower-lying excited states.

The UV/vis/NIR absorption spectra for 2Sm and 3Sm show four main features each between 6000 and 8500 cm^–1, Figure 7 (peaks A–D), and a further lower-intensity peak between 9500 and 10 000 cm^–1, Figure 7 (peak E). Similar to the spectrum of Sm(III) in LaF₃,^69,70 the low-lying states (⁶H_5/2, ⁶H_7/2, ⁶H_9/2, ⁶H_11/2, ⁶H_13/2) are energetically well-separated, but ⁶F_1/2, ⁶F_3/2, ⁶H_15/2, and ⁶F_5/2 overlap. For 2Sm, peak A corresponds to multiple low-intensity transitions corresponding to excitations to mixed final states composed of mostly ⁶H_15/2 character with a smaller contribution of ⁶F_1/2 (in some cases up to 30%). The final states corresponding to peak B are approximately a 50:50 mixture of the ⁶H_15/2 and ⁶F_3/2 terms. The remaining peaks are more straightforward and involve pure final states: ⁶H_5/2 → ⁶F_5/2 (C), ⁶H_5/2 → ⁶F_7/2 (D), and ⁶H_5/2 → ⁶F_9/2 (E). These transitions are known to lie at ∼7000, ∼8000, and ∼9500 cm^–1 for Sm(III) in LaF₃,^69,70 whereas our MS-CASPT2-SO results place them at ∼7800, ∼8600, and ∼9700 cm^–1. For 3Sm, the final states for peak A have a slightly different composition: several have >92% ⁶H_15/2 character, and some are ∼75% ⁶F_1/2 character. The final states corresponding to peak B are also mixed, however in contrast to the relatively equal composition for 2Sm, some of the transitions instead correspond to a state dominated by the ⁶H_15/2 term (∼70–80%). More similarly to 2Sm, peaks C–E for 3Sm correspond to transitions with the same pure final states as discussed above: ⁶H_5/2 → ⁶F_5/2 (C), ⁶H_5/2 → ⁶F_7/2 (D), and ⁶H_5/2 → ⁶F_9/2 (E).

Figure 7.

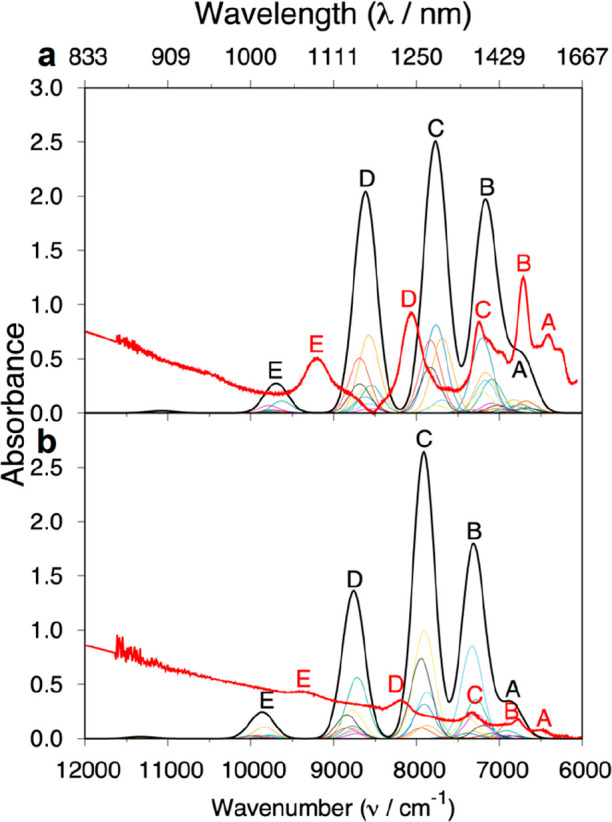
Experimental (red) and computed MS-CASPT2-SO (black) UV/vis/NIR data for 2Sm and 3Sm: (a) 2Sm; (b) 3Sm. Individual transitions (rainbow, below black) and total absorption spectrum were Gaussian-broadened with a half-width of 250 cm^–1.

The UV/vis/NIR spectra for the 5f⁵ Pu(III) compounds 2Pu and 3Pu contain significantly more features than their 4f⁵ Sm(III) analogues, Figure 8; this results from the stronger SO mixing, larger CF splitting and smaller IER. Interestingly, it is trivial to observe the differences between the spectra of the two compounds here, which must owe to the difference in the CF, a feature that is usually difficult to observe in 4f complexes (cf. Figures 6 and 7). Despite the complexity of the spectra, we observe good agreement between our MS-CASPT2-SO calculated spectra and the experimental data at low energies, Figure 8a and 8c. The intensity patterns are in good agreement and suggest minimal error in the calculated energies. Our experimental UV/vis/NIR spectra do not probe transitions below ∼6000 cm^–1; however ab initio calculations can assign this low-energy region for 2Pu as transitions from the ground ⁶H_5/2 multiplet to ⁶H_7/2 (∼1500–2400 cm^–1), ⁶H_9/2 (∼3600–4500 cm^–1), and to ⁶F_1/2 (∼5200 cm^–1). Because of larger CF splitting, these transitions are shifted to slightly higher energies in 3Pu: ⁶H_7/2 (∼1600–2700 cm^–1), ⁶H_9/2 (∼3900–4900 cm^–1), and ⁶F_1/2 (∼5600 cm^–1). These lower-energy multiplets are reasonably pure (>80%), but compared to the lanthanides, the 2Pu and 3Pu transitions are more mixed overall (Table S22). Furthermore, the amount of mixing seems to be slightly larger for 2Pu compared to 3Pu, and although less prominent, this is also seen for 2Sm compared to 3Sm. With the large amount of mixing, broad assignments can be made to regions of the spectra. The cluster of transitions within ∼6000–8000 cm^–1 correspond to excitations to mixed ⁶F_5/2 and ⁶H_11/2 terms, and the transitions within ∼9000–16 000 cm^–1 correspond to excitations to states made up of a mixture of ⁶F_9/2, ⁶H_13/2, and ⁶F_7/2 terms. Peaks with weaker intensity near 11 500–12 000 cm^–1 represent transitions to ⁶F_11/2 and ⁶H_15/2 terms. To examine the higher-energy region of the spectra (>17 000 cm^–1), we have performed calculations with an additional 16 quartet states, however, inclusion of these states deteriorates the quality of the lower-energy states, so these calculations are used to characterize the higher-energy states only, Figure 8b and 8d. The transitions in this region have final quartet states that are extremely mixed (Table S28) and produce weak absorption intensities in comparison to experiment. It is also possible that other transitions (e.g., f–d transitions) contribute to the absorption intensity in this part of the spectra.

Conclusions

We have established the synthesis and characterization of non-actinyl transneptunium multiple bonds, and plutonium NHC complexes, thus adding plutonium diphosphonioalkylidene and NHC linkages to the limited number of structurally authenticated organoplutonium complexes reported to date. Supporting the presence of Pu=C double bonds in the plutonium-diphosphonioalkylidene complexes, complex 2Pu engages in metallo-Wittig bond metathesis involving the highest atomic number element to date, reacting with benzaldehyde to produce the corresponding alkene 4 along with “PuOI”. In contrast, the corresponding reactions with 2Ce and 2Pr do not produce the alkene 4, possibly suggesting covalency differences in trivalent actinide– and lanthanide–carbon double bonds that lead to experimentally observable different outcomes in reactivity where more covalency in actinide–carbon double bonds leads to well-controlled metathesis but more ionic lanthanide analogs engage in more aggressive C–H activation reactions.

These Pu=C double and Pu–C dative bonds, along with new (praseodymium and samarium) and previously reported (uranium, neptunium, and cerium) diphosphonioalkyldiene congeners, have provided an opportunity to make lanthanide–actinide and actinide–actinide comparisons between metals with similar ionic radii and isoelectronic 4f⁵ vs 5f⁵ electron-counts within conserved ligand fields over 12 complexes. The bonding picture that emerges is clearly more complex than the traditional picture of the bonding of lanthanides being ionic and early actinides having relatively more covalent character and then becoming more ionic from left to right. Specifically, traversing the actinide series showed lower bond orders and ellipticities for these M=C bonds. Accompanying these changes were greater f- and lower d-orbital character with larger metal percentage contributions to the M=C bonds. Natural bond orbital and electron density topology analyses suggest that the orbital-energy matching (orbital mixing) and spatial-overlap terms increase from uranium to neptunium. In contrast, moving to plutonium improves the orbital-energy matching term and decreases the spatial-overlap. Overall, neptunium is the most covalent actinide in the series; however, the covalency of the plutonium complexes is still significant. Comparisons to the lanthanide analogs reveal a similarly intricate interplay of orbital-energy and spatial-overlap terms that is more complex than the traditional picture of lanthanide and actinide bonding.

Multiconfigurational calculations on 2M and 3M (M = Pu, Sm) account for the considerably more complex experimentally measured UV/vis/NIR spectra for 2Pu and 3Pu compared to 2Sm and 3Sm. The SO and CF splittings for the Pu(III) complexes are approximately double the values for the Sm(III) complexes, owing to more substantial relativistic effects and larger radial extension of the 5f- vs 4f-orbitals, respectively. However, the IER is approximately 50% larger for Sm(III) than Pu(III), likely arising from the more spatially confined nature of 4f-electrons compared to their 5f counterparts.

The recent reports of isolable Np=O,²⁶ Np=C,²⁷ and now Pu=C linkages, with their associated synthetic methodologies coupled to modern analytical techniques, pave the way for a wider expansion of transuranium metal–ligand multiple bond chemistry. Such advances will complement the major advances of uranium and thorium chemistry in recent years and have important ramifications for our understanding of the coordination and organometallic chemistry of the actinide elements.

Data Availability Statement

All other data are provided in the Supporting Information or are available from the authors on reasonable request.

Supporting Information Available

The Supporting Information is available free of charge at https://pubs.acs.org/doi/10.1021/jacs.3c12719.

• Experimental and computational details and X-ray crystallographic, spectroscopic, magnetic, and quantum chemical calculations (PDF)

The authors declare no competing financial interest.