Visual simulation of intraocular lenses: from on-bench performance to computational and experimental validations

Abstract

This study presents a systematic method to simulate various intraocular lenses (IOLs) available in the market. Five IOLs (two trifocals, one bifocal, one enhanced monofocal, and one extended depth of focus (EDOF)) were evaluated in terms of through focus visual Strehl (TFVS) utilizing the OptiSpheric IOL PRO2 device (Trioptics GmbH). Then, the estimated TFVS (ETFVS) and the temporal coefficients necessary for temporal multiplexing were computed, and through an iterative process, the SimVis TFVS was obtained. Finally, a high-speed focimeter was used to measure the opto-tunable lens responses to the temporal profile, and the experimental SimVis TFVS was acquired. Therefore, results are analyzed in terms of ETFVS (computed from the VSR-OTF), SimVis TFVS (computed from the TCs through temporal multiplexing), and experimental SimVis TFVS (acquired from the high-speed focimeter setup). The ETFVS and the SimVis TFVS curves demonstrated excellent alignment across all IOLs with cross-correlation coefficients > 0.94. Similarly, the experimental SimVis TFVS and the SimVis TFVS curves showed high correlation with cross-correlation coefficients > 0.97 and root mean square error (RMSE) < 0.05 for each lens. We demonstrated that different IOL designs can be visually simulated using its TFVS to obtain the corresponding temporal coefficients for simulations through temporal multiplexing using the SimVis technology.

1. Introduction

Presbyopia and cataract are inevitable conditions related to the aging of the human eye. Intraocular lenses (IOLs), replacing the natural crystalline lens -that has lost its elasticity in presbyopic subjects or is opacified due to cataracts-, are an effective solution for both conditions, with the multifocal IOLs gaining increasing recognition in recent years due to advancements in technology and improved patient outcomes. As opposed to monofocal IOLs that are conventional lenses focusing at only one distance, multifocal IOLs have the characteristic of focusing light on more than one focus to achieve good vision at more than one distance. Depending on the design, IOLs aim to provide the patient with good vision at far, near, and/or intermediate distances, or at a continuous range of distances by extending the depth of focus (EDOF IOLs). The working principle of multifocal IOLs entails projecting simultaneously on the retina focused and defocused images. The resultant visual experience is difficult to explain to patients, and the acceptance (i.e., how well the patient can tolerate the multifocality) is difficult to predict. Thus, the dissatisfaction after an IOL implantation is an unavoidable risk. Even though clinicians are trying to inform the patients and align the IOL choice with patient`s lifestyle and habits, their only tools are descriptions in marketing materials, visual experience opinions from other patients and available clinical outcomes. But since the visual experience through an IOL -including the optical coupling with the optics of the eye and the perceptual combination with the visual system - is unique for every individual [1,2], the evaluation of the subjective visual experience of different IOL designs before surgical implantation is essential, especially due to the fact that there is a plethora of IOLs in the market [3].

SimVis Technology enables see-through visual simulations of multifocal IOL corrections, which allow patients to experience the real world binocularly (∼20° field of view) through the multifocal corrections before IOL implantation [2,4,5]. SimVis Technology is based on a temporal-multiplexing approach, using opto-tunable lenses for the programmable simulation of different multifocal lens profiles [6]. Temporal-multiplexing is directly connected to the flicker fusion threshold of the human eye; the high-speed changes in power (50 Hz; faster than the flicker fusion threshold of the human eye) of the opto-tunable lens produce a multifocal image that is perceived as static [7]. As a result, SimVis Technology can simulate various multifocal corrections in a wearable device, and effectively transfer the multifocal visual experience to patients [6,8]. Thus, it can provide valuable guidance to both patients and eye care practitioners in selecting the most suitable correction.

To incorporate the simulation of an IOL in SimVis, as described in previous publications [9], the first step is to estimate the performance of the IOL at all distances. Many optical quality metrics (such as the MTF at a specific spatial frequency or the area under the MTF curve) could be used [10,11] but the Through Focus Visual Strehl (TFVS) metric provides good correlation with the perceived defocus and with perceptual judgements of subjective image quality [10,12]. The second step is to compute the set of theoretical temporal coefficients required for temporal multiplexing to match the TFVS of the IOL, named the SimVis temporal profile. Next, the optical quality of the simulation is measured experimentally on-bench using a high-speed focimeter [13], and the dynamic and temperature effects of the opto-tunable lens are compensated using custom developed algorithms [13,14]. When the experimental SimVis TFVS curve matches the IOL TFVS measurement, the simulation is experimentally validated. Finally, the through-focus visual acuity curves on presbyopic volunteers with SimVis simulators are compared with those of patients implanted with the corresponding real intraocular lens, providing the clinical validation of the technology [9].

In a recent publication, Sawides et al. [9] presented a method to simulate IOL designs through temporal multiplexing using only publicly available information (scientific papers, reports, FDA documents, etc.). Using the TF-MTF at 15cpd as input data, the TFVS of the IOL was estimated in order to compute the temporal coefficients that offer the best approximation to the actual lens design [9]. The abundance of available scientific literature and the several on-bench or commercial systems accessible to measure the TF-MTF are advantageous aspects of the proposed method from Sawides et al. However, the non-uniformity in techniques used for TF-MTF calculations in the publicly available information (TF-MTF measurements are performed using different systems, on different eye models, with different wavelengths and for different pupil sizes; including some of the published literature that uses the through focus area under the full MTF over using a single spatial frequency [15,16]) poses a challenge.

In this paper, we present a systematic methodology to simulate different IOLs that exist in the market, irrespective of their working principle. The performance at all distances of five IOLs (two trifocals, one bifocal, one enhanced monofocal and one EDOF) was measured in terms of TFVS derived from the measurements of the TF-MTF for a wide range of spatial frequency (up to 60cpd instead of the single 15cpd to provide a broader insight into IOL performance), and then custom-developed techniques were used to simulate and validate the profile of the five lenses through the SimVis Gekko visual simulator. This systematic and standardized methodology allows to simulate different commercial lens designs with the SimVis Technology.

2. Methods

To simulate an IOL with the SimVis Technology we first measure the performance of the IOL using TFVS (described in section 2.2- IOL metrology); we then compute the estimated TFVS (ETFVS), and the temporal coefficients required for temporal multiplexing (described in section 2.3 - computational validation); and finally, we measure the opto-tunable lens responses to the temporal profile using a high-speed focimeter (described in section 2.4 - experimental validation).

2.1. IOLs under study

To validate the method, five IOLs with different characteristics were studied; the AT LISA tri 839MP (trifocal, diffractive) from Carl Zeiss Meditec; the FINEVISION POD F (trifocal, diffractive) from BVI Medical Inc-PhysIOL; the Precizon Presbyopic NVA (bifocal, refractive segmented) from Ophtec BV; the TECNIS Eyhance ICB00 (enhanced monofocal, refractive) from Johnson & Johnson Surgical Vision; and the AcrySof IQ Vivity DTF015 (EDOF, refractive) from Alcon Laboratories Inc. Information about the lens manufacturer, model, power, type and optical technology can be found in Table 1.

Table 1. Specifications of the five IOLs that were simulated in this study.

Manufacturer	Model	Power	Type	Optical technology
Carl Zeiss Meditec	AT LISA tri 839MP	+20D	Trifocal	Diffractive
BVI Medical Inc/PhysIOL	FINEVISION (POD F)	+20D	Trifocal	Diffractive
Ophtec BV	Precizon Presbyopic NVA	+20D	Bifocal	Refractive segmented
Johnson & Johnson Surgical Vision	TECNIS Eyhance (ICB00)	+20D	Enhanced Monofocal	Refractive
Alcon Laboratories Inc	AcrySof IQ Vivity DTF015	+20D	EDOF	Refractive

2.2. IOL metrology

The performance of the IOLs was measured at The David J Apple International Laboratory for Ocular Pathology (University of Heidelberg, Germany) [17]. The metrology device was the OptiSpheric IOL PRO2 (Trioptics GmbH, Germany) which measures the effective focal length with a tolerance of ±0.3%, and the modulation transfer function (MTF) within ±2%. The MTF and the phase transfer function (PTF) were derived from the Fourier transform of a crosshair-target image projected by the lens under test [18]. The measurements were conducted according to the ISO 11979-2 standard for test methods for the optical performance of IOLs [19] in terms of wavelength by choosing a central wavelength of 546 nm provided by a polychromatic light source and an interference filter (10-nm bandwidth). The optical performance was assessed at the typical 3 mm aperture and other two different sizes of 3.5 and 4 mm at the IOL plane [20], using an aberration-free corneal model. The IOL was positioned within a mechanical holder filled with a balanced salt solution. A microscope-objective lens and a charge-coupled device camera (VA-1MCM120-A0-C; Vision Systems Technology) constitute the acquisition module. In the case of non-rotationally symmetric IOLs, two meridians were measured to ensure that the metrology data is influenced from all optical characteristics of the IOL (different segments, different zones). Therefore, SimVis can accurately simulate through focus optical power distribution (i.e., multifocality), although the point spread functions (and thus positive dysphotopsia) are not completely equivalent.

The repeatability of the assessment was derived from three consecutive measurements of the MTF at 15 and 30 cycles/degree (50 and 100 lp/mm respectively). The MTF and the PTF were determined using the MTF criterion at 15 cycles/degree(i.e., maximizing the MTF at 15cpd to obtain the best focus for the dioptric power under evaluation), and the MTF was measured for a defocus range of interest, typically from −2 to +5D for trifocals, from −2 to +4D for EDOFs and from −2D to +2.6D for enhanced monofocal with a 0.2D step (at the IOL plane). The IOL's optical transfer function (OTF) was calculated:

$$OTF(f)=MTF(f)\cdot e^{iPTF(f)}$$

for a spatial frequency (f) up to 60 cycles/degree (200lp/mm). The real part of the OTF was used to derive the Visual Strehl Ratio (VSR) weighted by the neural contrast sensitivity function (CSF_N) as described by Thibos et al. [21]:

$$VSOTF=\frac{{\int \int }_{-\mathrm{\infty }}^{\mathrm{\infty }}CS{F}_N(f_x,f_y)\cdot OTF(f_x,f_y)d{f}_{x}d{f}_y}{{\int \int }_{-\mathrm{\infty }}^{\mathrm{\infty }}CS{F}_N(f_x,f_y)\cdot OT{F}_{DL}(f_x,f_y)d{f}_{x}d{f}_y}$$

To obtain the CSF_N, the Mannos and Sakrison et al. [22] model was applied:

$$CS{F}_N(f)=2.6\cdot (0.0192+0.114\cdot f)\cdot e^{-{(0.114\cdot f)}^{1.1}}$$

2.3. Computational validation

The computational validation was performed at 2EyesVision utilizing custom-developed automated routines in MATLAB (MathWorks, USA). For each lens, the process was performed for all three different apertures (3, 3.5, and 4 mm at the IOL plane which correspond to 3.4, 4 and 4.6 mm on the entrance-pupil plane respectively). The first step of the process was to compute the Estimated TFVS (ETFVS) from the through focus VSOTF passing from the IOL plane to the entrance-pupil plane (i.e., the plane on which the SimVis Gekko simulates the IOL) in a 0.05 D step [23]. The MTF was measured and the VSOTF calculated in a 0.2D step, therefore a smooth interpolation was applied to get the ETFVS in a 0.05D step. Following that, the temporal coefficients (TCs) that are necessary for temporal multiplexing were computed using the 0.05D sampling. The TCs correspond to the time that the opto-tunable lens spends in each addition and were optimized through an iterative process to obtain the SimVis TFVS that mimic the ETFVS in the dioptric range of interest [6], using typically 15 iterations to reach the optimized SimVis TFVS. Additionally, the opto-tunable lens dynamics were considered and the dynamic effect on the temporal profile was corrected accordingly [8]. Finally, the deviations in optical power of the opto-tunable lens due to external and internal temperature changes were compensated for [14].

Yi et al. reported that the VSR limit of normal visual function is 0.12 [24], that corresponds to a visual acuity of approximately 0.2 logMAR [11,12]. Thus, to evaluate the TCs in a correct defocus range we have set the criterion > 0.10 i.e., the TCs were calculated so that the VSR curve replicated the measurements in the defocus where VSR was above 0.10. Additionally, a second criterion for our optimization process is that the dioptric range which contributes to the iteration process and the TCs optimization is between −0.5 D to ≈ + 2.5 D for EDOFs and enhanced monofocal and between −0.5 D to ≈ + 3.5 D for trifocals, based on the defocus range of interest for each IOL. These dioptric ranges are also used to favor the extended depth of focus and the multifocality, and to have a better match on the peaks of the curves during optimization.

2.4. Experimental validation

The experimental validation of the opto-tunable lens response to the temporal profile was performed at 2EyesVision. A high-speed focimeter and custom-developed programs in MATLAB were used, and opto-tunable lens responses to the temporal profiles of all three pupil diameters were validated. The high-speed focimeter setup consists of an illuminated slit (object); a 4f projection system that creates a pupil plane on which the under-evaluation opto-tunable lens is placed; a second 4f system with conjugated pupil plane to the opto-tunable lens (Optotune, EL-3-10); a prism; and a high-speed camera (3823 fps) to capture the images of the slit. The setup and the procedure of the evaluation have been previously described in detail by Dorronsoro et al [13]. The simulation is considered accurate and validated if the experimental SimVis TFVS (programmed lens) matches the SimVis TFVS of the IOL (peaks shift < 0.20 D, Root Mean Square Error < 0.05) in the dioptric range of interest. [25].

3. Results

3.1. IOL metrology

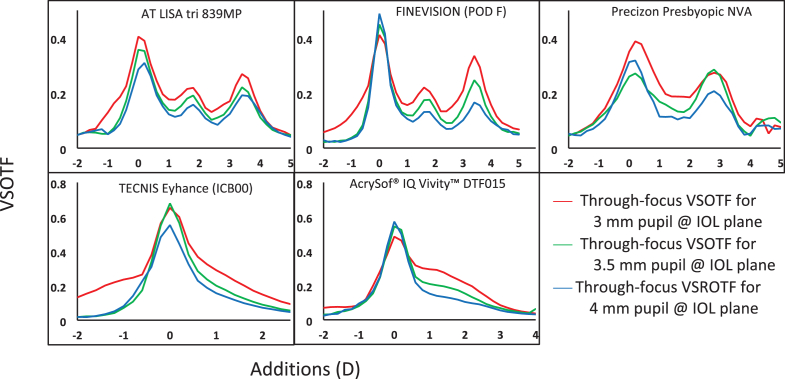
The optical-quality results of the IOLs can be found on Fig. 1. The plots represent the through focus VSR of the IOLs calculated based on the OTF for 3 mm (red curve), 3.5 mm (green curve) and 4 mm (blue curve) apertures at the IOL plane.

Fig. 1.

Through-focus VSR of the IOLs calculated based on the OTF for 3 mm (red curve), 3.5 mm (green curve) and 4 mm (blue curve) apertures at the IOL plane. Each graph represents one IOL.

3.2. Computational validation

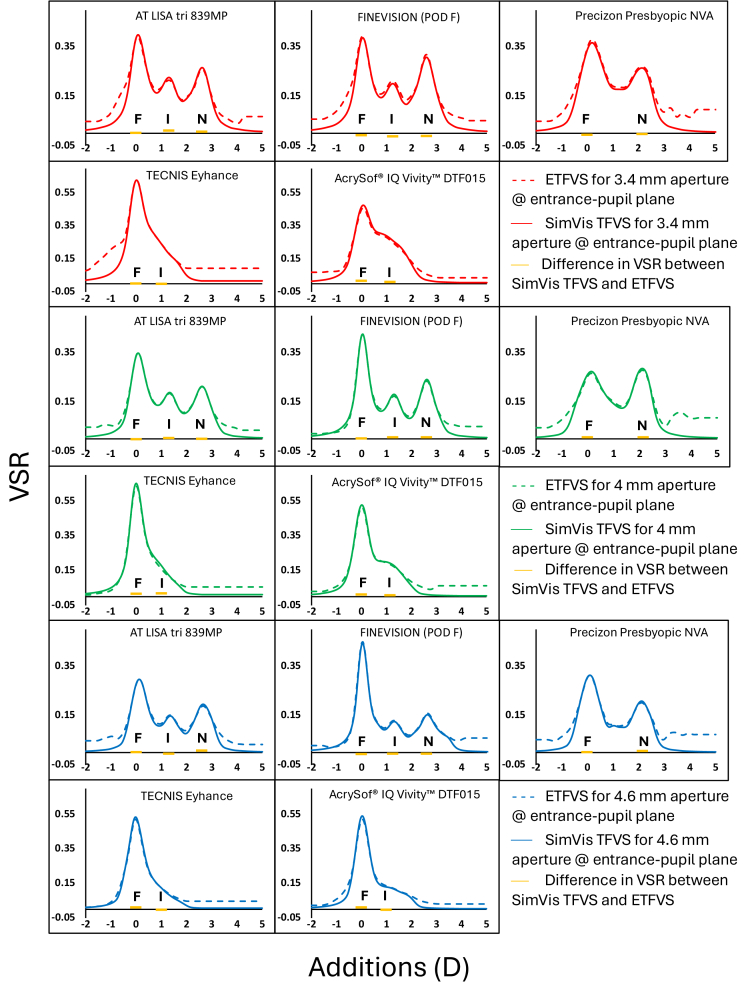
Figure 2 presents the results from the computational validations utilizing custom-developed automated routines in MATLAB. The ETFVS (computed from the VSOTF) is represented by the dashed curve, and the SimVis TFVS (computed from the TCs through temporal multiplexing) is represented by the solid curve. Both curves were recalculated for the entrance-pupil plane (since the IOL is simulated on this plane by SimVis Gekko), and the results for 3.4 mm (red curve), 4 mm (green curve) and 4.6 mm (blue curve) pupils (correspond to 3 mm, 3.5 mm and 4 mm apertures at the IOL plane respectively) are presented. The curves demonstrate excellent alignment between measurements and simulations across all IOLs in the dioptric range of interest, as set by the optimization criterion (i.e. the SimVis TFVS approaches VSR = 0 earlier, below −0.5D and beyond 3.5D for trifocals and 2.5D for EDOFs, compared to the ETFVS). The cross-correlation coefficients between the ETFVS and the SimVis TFVS in the dioptric range of interest are 0.97, 0.98, 0.96, 0.95 and 0.99 for 3.4 mm pupil, 0.99, 0.99, 0.94, 0.99, 0.98 for 4 mm pupil and 0.98, 0.98, 0.94, 0.99, 0.99 for 4.6 mm pupil for AT LISA tri 839MP, FINEVISION (POD F), Precizon Presbyopic NVA, TECNIS Eyhance (ICB00) and AcrySof IQ Vivity DTF015, respectively. The RMSE was ≤ 0.05 for all pupil sizes and IOLs, except for the TECNIS Eyhance (ICB00) which had RMSE = 0.07 for the 3.4 mm pupil. The ETFVS curve of TECNIS Eyhance (ICB00) at 3.4 mm pupil showed a pronounced elevation in the negative defocus direction (and thus the higher RMSE); this part of the curve did not influence the computational validation since it does not meet our criteria (described in section 2.3 - computational validation).

Fig. 2.

Computational validation results. ETVS (computed from the VSOTF) and SimVis TFVS (computed from the TCs through temporal multiplexing) are represented by the dashed and the solid curves, respectively. Data is recalculated for 3.4 mm (red curve), 4 mm (green curve) and 4.6 mm (blue curve) pupils (entrance-pupil plane where SimVis simulates the IOL). The orange lines represent the VSR differences between the SimVis TFVS and the ETFVS (SimVis TFVS minus ETFVS). This difference was calculated for far (F), intermediate (I) and near (N) distances for the trifocals, for far and near for the bifocal and for far and intermediate for the EDOFs.

3.3. Experimental validation

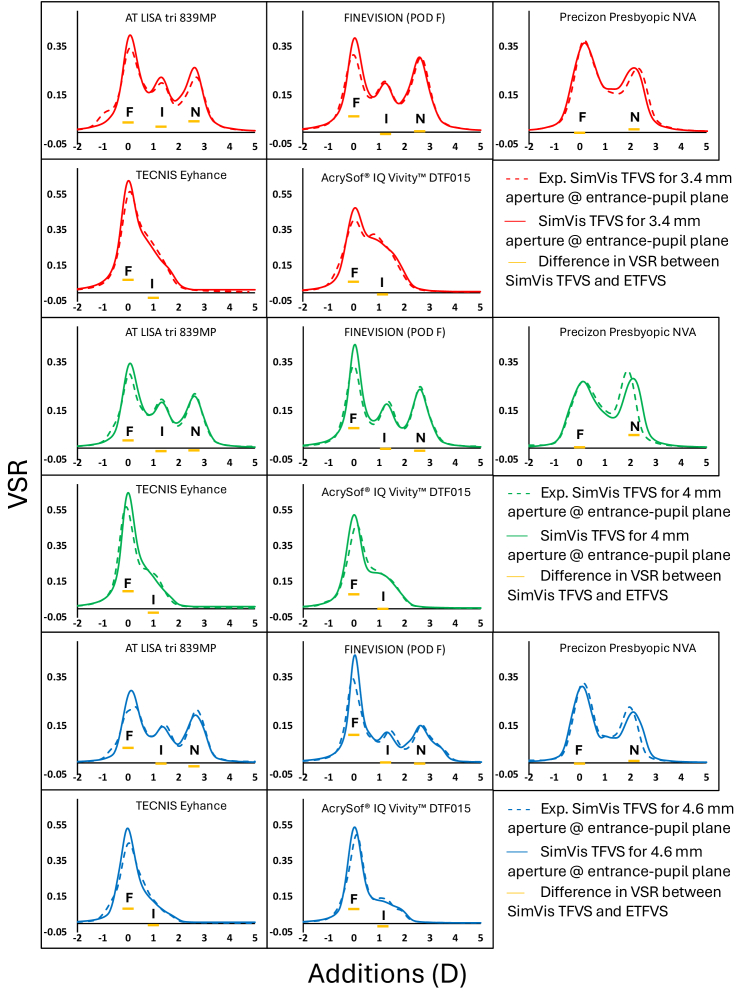
The results from the experimental validations for all pupil sizes are shown in Fig. 3. The dashed curve represents the experimental SimVis TFVS acquired from the high-speed focimeter setup, and the solid curve represents the SimVis TFVS computed from the TCs through temporal multiplexing. The curves aligned well with cross-correlation coefficient 0.99, and RMSE < 0.05 for each lens and pupil size, except for Precizon Presbyopic NVA that had cross-correlation coefficient 0.97 for 4 mm pupil.

Fig. 3.

Experimental validation results for 3.4 mm (red curve), 4 mm (green curve) and 4.6 mm (blue curve) pupils (entrance-pupil plane where SimVis simulates the IOL). Experimental SimVis TFVS (acquired from the high-speed focimeter setup) and SimVis TFVS (computed from the TCs through temporal multiplexing) are represented by the dashed and the solid curves, respectively.). The orange lines represent the VSR differences between the SimVis TFVS and the Experimental SimVis TFVS (SimVis TFVS minus Experimental SimVis TFVS). This difference was calculated for far (F), intermediate (I) and near (N) distances for the trifocals, for far and near for the bifocal and for far and intermediate for the EDOFs.

4. Discussion

The aim of this paper was to introduce a systematic approach for simulating all types of IOL that exist in the market. The performance of the IOL was measured with the OptiSpheric IOL PRO2 (Trioptics GmbH) [17]. By employing our custom-developed techniques we successfully simulated the profile of the IOL with the SimVis Gekko visual simulator. Enhanced monofocal, bifocal and trifocal IOLs with refractive and diffractive optics were simulated to prove the validity of our method. In short, the results showed great correspondence between the ETFVS (computed from the VSOTF) and the SimVis TFVS (computed from the TCs through temporal multiplexing), as well as between the Experimental SimVis TFVS (acquired from the high-speed focimeter setup) and the SimVis TFVS. In almost all cases the cross-correlation coefficient was higher than 0.99 and the RMSE < 0.05.

As described earlier, the metrology measurements were performed at the David J Apple International Laboratory for Ocular Pathology of the University of Heidelberg. The optical performance of the IOLs was measured in green light (546 nm), and thus the SimVis simulation is based on data acquired under monochromatic conditions even though it aims to simulate IOLs in a polychromatic environment. However, we should acknowledge that some IOLs have been designed to manipulate chromatic aberration to extend the depth of focus [26], and that the chromatic aberration of refractive and diffractive IOLs is known to change with different materials and additions [17,27]. However, it has been demonstrated both theoretically (by Akondi et al., 2017 [6]) and experimentally (Sawides et al., 2021 [9]) that the technology is capable of simulating the through-focus visual Strehl corresponding to polychromatic light. In particular, those studies demonstrated no significant difference between the TFVS corresponding to 555 nm and the polychromatic TFVS for a trifocal diffractive lens (Finevision, Physiol/BVI).

Moreover, currently we use an aberration-free corneal model for evaluating the IOLs, and thus any factors that can affect the VSR are solely due to the IOL being measured. It is worth noting, that in various studies other corneal models that incorporate spherical aberration of different magnitudes are used [28,29], however our purpose was to minimize the effects of spherical aberration in the metrology measurements, since the simulation will interact with the natural spherical aberration of the patient. The aforementioned aspects are a subject of consideration for the future, aiming at investigating ways to improve the simulation of IOLs. As the last step of our process, clinical validation follows (more details in the last paragraph of the discussion section) to ensure that the impact of the limitations in our simulations is trivial.

The method described in this paper is relying on bench measurements for acquiring the performance data of the IOL. An alternative approach could be acquiring data directly from the manufacturer. This strategy becomes particularly relevant when there are differences between the nominal powers of the IOLs (depending on the magnitude of the ametropia of the patient) and/or if we would like to consider the spherical aberration of the IOL and the interaction with the human eye, especially in the case of patients that have undergone LASIK or have keratoconus [30,31]. In this way SimVis Gekko could provide more personalized simulations, custom-made for each patient. Another alternative that has been already described [9], is using IOL data that can be found in published literature.

The last phase of our process is clinical validation which has been previously described by Sawides et al. [9]. In this phase, presbyopes are wearing SimVis Gekko while the IOL is simulated, and their through-focus visual acuity is evaluated. The results are then compared to through-focus visual acuity curves of patients implanted with the corresponding IOL (data from published scientific articles). The cross-correlation coefficient between the two curves (SimVis simulation and literature data through-focus visual acuity curves) is calculated as a curve-shape similarity metric, and comparisons are made in terms of Root Mean Square Error. If the comparison meets certain criteria (cross-correlation coefficient > 0.90, RMSE < 0.10) the clinical validation is approved, and the simulation is incorporated in SimVis Gekko. Despite small differences in the height of the peaks between the Experimental SimVis TFVS and the SimVis TFVS that can occur, the impact in visual perception is negligible when clinical validations with patients are performed [9,32].

5. Conclusion

In conclusion, we presented a systematic methodology to visually simulate different IOL designs that exist in the market through a three-step validation process. The TFVS of each IOL was used to obtain the temporal coefficients necessary for the simulations through temporal multiplexing using the SimVis Technology. This enables patients to experience the visual effects of the IOL before implantation and facilitates their choice, ensuring they are more comfortable with the decision-making process.

Funding

Ministerio de Ciencia e Innovación10.13039/501100004837 (Retos CPP2021-008388, Torres Quevedo, PTQ2021-011963, PID2020-115191RB-I00); Klaus Tschira Stiftung10.13039/501100007316.

Disclosures

P. Papadogiannis: employee at 2EyesVision, E. Gambra: shareholder at 2EyesVision, employee at 2EyesVision, G. Łabuz: non-financial support from Contamac outside the submitted work, W. Yan: nothing to disclose, D. Martin: employee at 2EyesVision, I. Sisó-Fuertes: nothing to disclose, A. de Castro: nothing to disclose, X. Barcala: nothing to disclose, G. U. Auffarth reports grants, personal fees, non-financial support and consulting fees from Johnson&Johnson and Alcon, grants, personal fees, and non-financial support from Carl Zeiss Meditec, Hoya, Kowa, Oculentis/Teleon, Rayner, Santen, Sifi, Ursapharm, grants, and personal fees from Biotech, Oculus, EyeYon grants from Acufocus, Anew, Contamac, Glaukos, Physiol, Rheacell, outside the submitted work, C. Dorronsoro: shareholder at 2EyesVision, employee at 2EyesVision, L. Sawides: shareholder at 2EyesVision.

Data availability

Data underlying the results presented in this paper are not publicly available at this time but may be obtained from the authors upon reasonable request.