Abstract
Antiferromagnetic magnons possess a distinctive feature absent in their ferromagnetic counterparts: the presence of two distinct handedness modes, the right-handed (RH) and left-handed (LH) precession modes. The magnon handedness determines the sign of spin polarization carried by the propagating magnon, which is indispensable for harnessing the diverse functionalities in magnonic devices, such as data encoding, magnon polarization-based logic systems, and quantum applications involving magnons. However, the control of coherently propagating magnon handedness in antiferromagnets has remained elusive. Here we demonstrate the manipulation and electrical readout of propagating magnon handedness in perpendicularly magnetized synthetic antiferromagnets (SAF). We find that the antiferromagnetic magnon handedness can be directly identified by measuring the inverse spin Hall effect (ISHE) voltage, which arises from the spin pumping effect caused by the propagating antiferromagnetic magnons in the SAF structure. The RH and LH modes of the magnon can be distinguishable when the SAF structure is sandwiched by heavy metals with the same sign of spin Hall angle. This work unveils promising avenues for harnessing the unique properties of antiferromagnetic magnons.
Subject terms: Spintronics, Magnetic devices
Antiferromagnetic magnons have two distinct chiralities, left handed and right handed. Here, Shiota and coauthors demonstrate both the manipulation and electrical readout of this handedness in a synthetic antiferromagnet.
Introduction
The control of magnetization by spin current, which is a flow of spin angular momentum, plays an important role in both basic and applied point of view1,2. In magnetic materials, spin waves or magnons are known to be the carriers of spin currents. Magnon spin currents are attracting attention as a propagation method with less dissipation than conduction electron spin currents3–8, because magnon propagation does not involve charge transfer resulting in the absence of Joule heating. Therefore, magnons are expected to be a promising platform for realizing low-power devices, logic circuits, and computing applications9–20. In contrast to ferromagnets (FMs), collinear antiferromagnets possess two precession modes, right-handed (RH) precession and left-handed (LH) precession21–23. This allows to manipulate the magnon polarization degree of freedom, which is analogous to its optical counterpart. The control of the magnon handedness is a critical issue in applications using magnon polarization degree of freedom, such as magnon field-effect transistor24,25, domain wall as magnon polarizer and retarder26, bidirectional domain wall motion27,28, and quantum system with magnon29. Previous studies have shown that utilizing antiferromagnetic materials or synthetic ferrimagnets enables the generation of right- and left-handed magnons of infinite wavelength, where the spins are excited uniformly in phase throughout the entire sample, making them electrically detectable30–32. However, the manipulation and electrical detection of the propagating magnon handedness have yet to be demonstrated due to their field immunity and ultrafast spin dynamics, which is a major challenge for practical applications.
In this study, we report the first demonstration of polarization-resolved detection and handedness manipulation of propagating antiferromagnetic magnons. We prepared the synthetic antiferromagnet (SAF) sandwiched by heavy metal (HM) layers. Propagating magnons in SAF structure are excited by applying microwave current to a stripline antenna and emit handedness-dependent spin current via spin pumping effect into HM layers. The spin current is converted to electric current through the inverse spin Hall effect (ISHE)33. We find that the RH and LH modes of the magnon provide two distinguishable resonance peaks in the ISHE voltage, particularly when the sign of spin Hall angle in two HM layers is the same; therefore, the magnon handedness can be electrically detected. We further demonstrate the manipulation of magnon handedness by tuning the excitation frequency under conditions where the degeneracy of two magnon modes is lifted. These results lead to a new path for utilizing antiferromagnetic magnons in future polarization-based magnonic devices.
Results
Concept of magnon excitation and detection
Our proposal for the excitation and detection of the handedness-dependent magnon is based on the fact that two (RH and LH) precession modes in colinear antiferromagnets can have different resonance conditions, as shown in Fig. 1a. There are two distinct modes corresponding to RH and LH precession of the antiferromagnetic resonance, except at a certain magnetic field where they are degenerated. The opposite linear dependence of the two modes on the magnetic field arises from the fact that the angular momenta of the two modes point in opposite directions, resulting in different Zeeman energies. Therefore, the degeneracy of these two modes can be lifted by applying an external magnetic field. Accordingly, we can selectively excite one of the two modes by appropriately choosing the excitation frequency. The detection of the handedness is achieved by utilizing the ISHE effect, as described in the following.
Fig. 1. Handedness manipulation of propagating antiferromagnetic magnon.
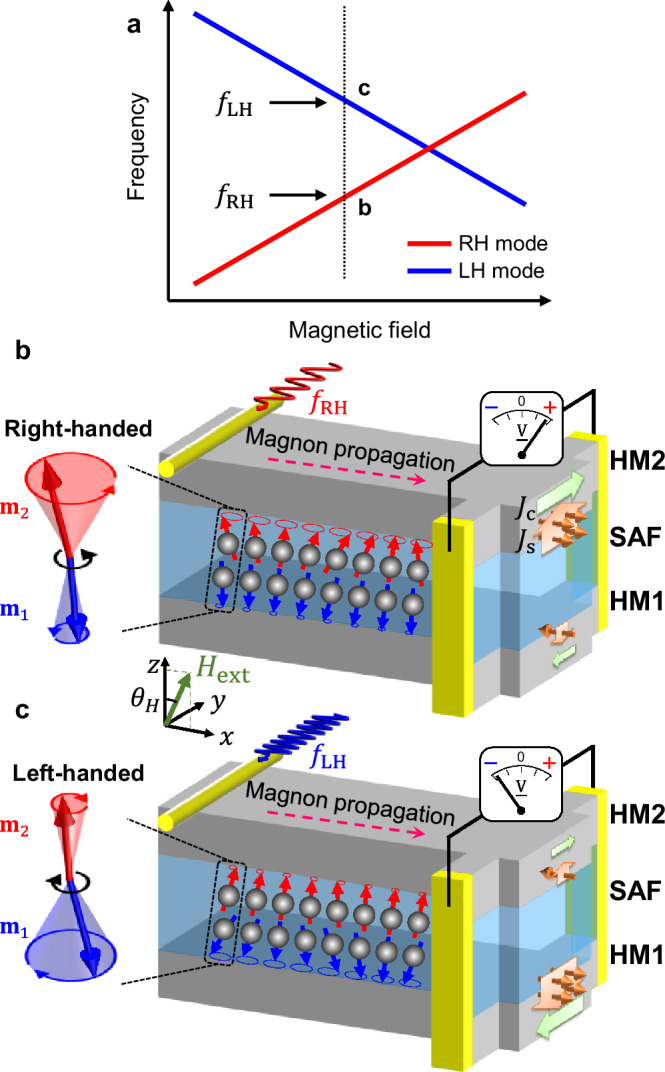
a Magnetic resonance frequency for right-handed (RH) and left-handed (LH) precession modes in colinear antiferromagnets as a function of the magnetic field. b, c Left panels, illustrations of magnon eigenmode with RH and LH precession modes. The handedness of propagating antiferromagnetic magnons in perpendicularly magnetized synthetic antiferromagnet (SAF) can be manipulated by tuning the excitation microwave frequency to either or at a fixed magnetic field, as indicated by the black dotted line in (a), which corresponds to the condition where the degeneracy of two magnon modes is lifted. The handedness-dependent spin current is emitted through the spin pumping effect into heavy metal (HM) layers and its -polarized component shown in the figure is converted to charge current through the inverse spin Hall effect.
We consider a magnetic thin film consisting of perpendicularly magnetized SAF sandwiched by two HMs. The magnetizations in two FM layers in the SAF structure are coupled antiferromagnetically. The magnons in the SAFs are excited by applying a microwave magnetic field from an antenna, as schematically shown in Fig. 1b, c. Coherently propagating antiferromagnetic magnons in the -direction emit spin currents into HMs due to the spin pumping effect. The spin current is converted to charge current by the spin-to-charge conversion due to the ISHE33. The resulting transverse voltage in the -direction is proportional to
| 1 |
where is the spin Hall angle of the lower (upper) HM materials, while is the time average of the component of the spin polarization pumped from the lower (upper) FM layer. The external magnetic field is applied in the -plane with a tilted angle from the perpendicular axis, which tilts the precession axis of the magnetization and makes be finite34. Note that the signs of two terms on the right-hand side of Eq. (1) are opposite. This is because two FM layers pump the spin current into the opposite directions ( and for the upper and lower FM layers, respectively). The direction of the electric current generated by the ISHE depends on this pumping direction and the spin Hall angle of two HMs.
Equation (1) clarifies the relationship between the sign of the ISHE voltage and the magnon handedness for a fixed magnetic field. We notice that, for both RH and LH modes, two magnetizations in SAFs precess in the same direction, however, the oscillation amplitude is different23, as schematically shown in the left panel of Fig. 1b, c. For RH (LH) mode the magnetization oriented to () direction is an active precession state and exhibits a larger oscillation amplitude. Accordingly, in the case of antiparallel magnetization configuration shown in Fig. 1b, c, the second (first) term on the right-hand side of Eq. (1) becomes the dominant contribution in the ISHE voltage for RH (LH) mode due to the large amount of resulting from the spin pumping effect. If and have the same sign such as the Pt sandwiched SAF structure, these differences in the dominant contribution change the sign of the ISHE voltage depending on the magnon handedness (RH or LH), as shown in Fig. 1b, c. On the other hand, if and have the opposite signs, both two terms on the right-hand side of Eq. (1) contribute to the ISHE voltage with the same sign. This makes it difficult to distinguish the RH and LH modes. Therefore, the use of HMs with the same sign of the spin Hall angle is a key to efficiently detect the magnon handedness.
Sample details
Based on the above concept, we perform the experiments. Thin films were deposited by dc magnetron sputtering on thermally oxidized Si substrate with the following structures (thickness in nm): Ta(2.0) / Pt(5.0) / [Co(0.3) / Ni(0.6)]8.5 / Ru(0.42) / [Co(0.3) / Ni(0.6)]8.5 / Pt(5.0) (hereafter referred to as Pt-SAF-Pt). The use of decimal period number, such as 8.5, indicates that the superlattice structure has a Co layer on both the top and bottom of film stack, with one side having an additional half period. Even though the 0.42-nm-thick Ru spacer layer is atomically thin, the first oscillation peak of the antiferromagnetic interlayer exchange coupling and atomically smooth interfaces have been confirmed in the prior works35,36. For comparison, we also prepared perpendicularly magnetized SAF films with different combinations of HM layers, such as Ru-SAF-Pt and Pt-SAF-Ru. Note that the signs of the spin Hall angle in Ru and Pt are opposite37, which affects the measurement as discussed above. After the deposition process, the out-of-plane magnetic hysteresis loops were measured to determine the magnetization configuration as a function of the external magnetic field (see Supplementary S1). The two different antiparallel magnetization configurations, namely head-to-head (H-H) and tail-to-tail (T-T), can be obtained depending on the field sweep direction after applying the large enough magnetic field to saturate the magnetization (see Supplementary S1). The magnetic resonance measurements were also conducted under the external magnetic field along the out-of-plane direction to quantify the values of magnetic parameters, such as the perpendicular magnetic anisotropy field and the interlayer exchange field (see Supplementary S2).
Inverse spin Hall detection of propagating magnons
Next, we experimentally investigate the electrical detection of the propagating magnon handedness. We fabricated the devices with the Hall bar structure and microwave antenna, as shown in Fig. 2a (see Supplementary S3 for the details of device fabrication). The magnons are excited by applying a microwave current to the stripline antenna and the lock-in amplifier is used to detect the Hall voltage . The detection of propagating magnon is achieved by measuring the ISHE voltage. Here the distance of the magnon propagation is 0.4 μm.
Fig. 2. Inverse spin Hall detection of propagating magnons in Pt-SAF-Pt.
a Schematic of the measurement setup and scanning electron microscope image of the devices. b The signal after background subtraction under the tilted magnetic field of = 30° at the microwave frequency of 13 GHz. The black and red curves indicate the spectra measured for the downward and upward field sweep direction, respectively. The inset shows the detected signal under the tilted magnetic field. spectra under the tilted magnetic field = 30° for T-T antiparallel magnetization configuration (c) and H-H antiparallel magnetization configuration (d). The dashed lines in (c, d) cprrespond to the magnetic field for the experiments performed in Fig. 4.
The detected signal under the tilted magnetic field of = 30° at the microwave frequency of = 13 GHz is shown in the inset of Fig. 2b. We notice that the hysteresis loop similar to the magnetization curve are observed. This is the contribution of the anomalous Nernst effect to the Hall voltage, because the application of microwaves causes local heating of the magnon excitation antenna, leading to the in-plane thermal gradient in the -direction. This contribution can be eliminated by subtracting a linear background signal in the field range of the antiparallel magnetization configuration. The signals after background subtraction are hereafter referred to as . As shown in Fig. 2b, the resonance peaks in spectra are observed for the upward and downward field sweep directions, corresponding to the T-T and H-H antiparallel magnetization configurations, respectively. It should be noted that the spin rectification signal due to anisotropic magnetoresistance and anomalous Hall effect is absent detected in our devices, because the microwave antenna is electrically isolated from the Hall bar structure by the 50-nm-thick SiO2 layer (see Supplementary S3).
To clarify the relationship between the magnon handedness and the sign of ISHE resonance peak, we next measured spectra at various excitation frequencies. Figure 2c, d shows color plots of spectra obtained from upward and downward field sweeps, respectively. Two precession modes are observed in the magnetic field range in which the two magnetizations are antiparallel. The resonance frequency that increases (decreases) with increasing magnetic field corresponds to the RH (LH) precession mode (see Supplementary Fig. S2a, b for a comparison of the magnon handedness with the magnetic resonance measurements under the out-of-plane magnetic field). The sign reversal of the resonance peaks is observed in positive and negative magnetic field regions because the in-plane component of the magnetization in the SAFs is reversed. More remarkably, the sign of the resonance peaks is also reversed depending on the handedness of the precession modes in the positive (negative) magnetic field region for T-T (H-H) antiparallel magnetization configuration, respectively. These results are consistent with our proposal mentioned in the previous section, and thus, indicate the possibility to distinguish the magnon handedness in Pt sandwiched SAFs electrically (see Supplementary S4 for additional experimental data under = 150°).
Numerical simulations
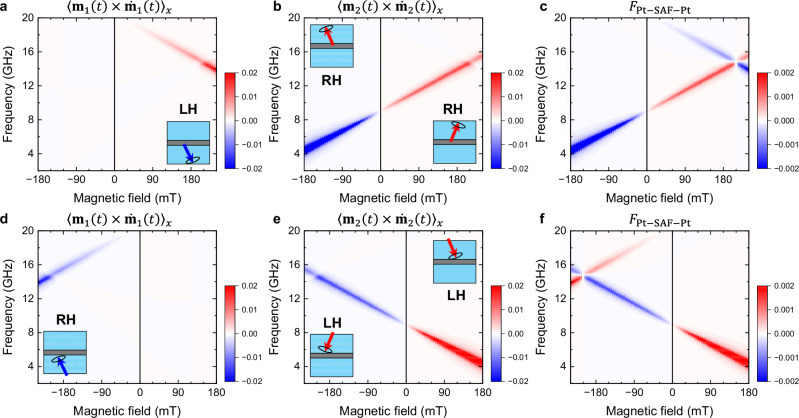
The detections of the magnon handedness demonstrated above were based on the fact that the oscillation amplitudes of the magnetization in two FM layers are different depending on the magnon handedness23. However, it is experimentally difficult to quantify the amounts of the pumped spin current from the upper and lower FM layers separately. Therefore, we performed numerical simulations of the Landau-Lifshitz-Gilbert equation (see methods). Figure 3a, b shows the time average of the component of the spin polarization pumped from the lower FM layer and upper FM layer , respectively, for T-T antiparallel magnetization configuration. Since the magnetization oriented to () direction exhibits a larger oscillation amplitude for RH (LH) mode (see Supplementary S5 for the precession trajectory), only the RH (LH) precession mode shows the visible peaks in Fig. 3b (Fig. 3a), respectively. Note that the sign of spin current spin polarization is positive regardless of the magnon handedness when we focus on the positive magnetic field region because the in-plane component magnetization in the active precession layer is oriented in the same direction regardless of the precession mode, as schematically shown in insets of Fig. 3a, b. In the case of the H-H antiparallel magnetization configuration, the relationship of magnetization orientation is opposite to that of the T-T antiparallel magnetization configuration. Therefore, only the RH (LH) precession mode shows the visible peaks in Fig. 3d (Fig. 3e), respectively. Then, the calculated based on Eq. (1) for both antiparallel magnetization configurations (Fig. 3c, f) well reproduce the observed behavior in the experiments, where the spin Hall angles are assumed to be = 0.1 (see Supplementary S6 for Ru-SAF-Pt and Pt-SAF-Ru structures). These calculations reveal that the spin pumping effect from the upper or lower FM is quantitatively dominant depending on the magnon handedness and the sign of the ISHE voltage for HM1-SAF-HM2 structure is determined by the spin Hall angle in HMs.
Fig. 3. Calculated spin polarization and expected ISHE voltage arising from the propagating antiferromagnetic magnons in Pt-SAF-Pt.
Color plots of calculated (a, d), (b, e), and based on Eq. (1) (c, f) for T-T antiparallel magnetization configuration (a–c) and H-H antiparallel magnetization configuration (d–f). The insets show the illustration of SAFs with active precession magnetization state for RH and LH modes.
Propagating magnon decay length
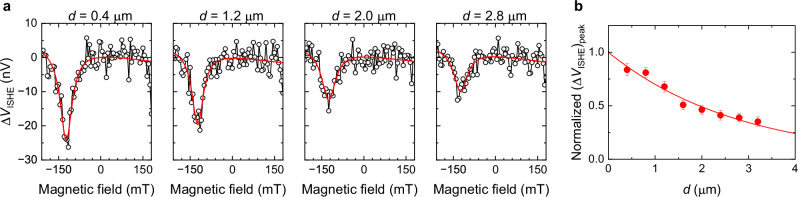
A requirement for realizing magnon-based devices is to achieve a long length scale for transferring the information. In conventional magnon-based devices, the length scale is the magnon decay length, which is determined from the group velocity and dissipation of the propagating magnons. It is, however, difficult to estimate the characteristics of the propagating magnons, such as dispersion relation and group velocity, in the present system. This is because the ISHE detection used in the present work is not a phase-sensitive detection method, in contrast with the propagating spin wave spectroscopy using a vector network analyzer38. Thus, instead, we performed a micromagnetic simulation analysis (see Supplementary S7). In addition, we also performed the measurements of the propagating magnon spectra for the devices with different to evaluate the decay length of the ISHE resonance signals (Fig. 4a). The peak voltage of each spectrum is obtained from the fit of the Lorentzian function, and as a function of is plotted in Fig. 4b. The intensity decreases exponentially and the decay length of the is estimated to be 2.8 μm, which is about one order of magnitude larger than the numerically calculated value (0.2–0.3 μm; see Supplementary S7). One possible reason for the longer decay length in our experiment is the contribution of the ISHE voltage not only from the magnons at the cross-section of the Hall bar but also from the magnon in the region away from the Hall bar, because the upper and lower HMs cover the entire surface of the SAF structure. From a different perspective, even though the magnon decay length is short, the propagating magnon signal measured by the ISHE can be transmitted over a longer distance using the method proposed in this study. Therefore, our proposed method is advantageous from the perspective of the information transfer beyond the decay length, alongside the ability to distinguish the magnon handedness.
Fig. 4. Inverse spin Hall detection of propagating magnons in the device with different d.
a spectra under the tilted magnetic field = 30° at 13.0 GHz for the devices with different . The red lines represent the fitting results using the symmetric Lorentzian function, and the values of peak amplitude are extracted. b Distance dependence of . The edge of the antenna corresponds to = 0, and the values of are normalized at = 0.0. The red line represents the fit of the exponential decay function.
Switching the handedness of propagating antiferromagnetic magnons
Here, in the middle of Fig. 5, we also demonstrate the manipulation of the antiferromagnetic magnon handedness by tuning the excitation frequency to either 13.6 GHz or 18.0 GHz under the tilted magnetic field of 140 mT for the T-T antiparallel magnetization configuration. The sign of the voltage changes as the excitation frequency switches. Thus, we can switch the magnon handedness by switching the excitation frequency. The relationship between the frequency and the magnon handedness is reversed when the magnetization configuration is H-H under the tilted magnetic field of −140 mT, as shown in the bottom of Fig. 5. In summary, we have succeeded in manipulating the handedness of the propagating magnon and in electrically reading out its information, which means that we now have the technology to realize theoretically proposed novel devices using the magnon polarization degree of freedom24–29.
Fig. 5. Switching the handedness of propagating antiferromagnetic magnons.

As shown in the top panel, the excitation microwave frequency is tuned to either 13.6 GHz or 18.0 GHz, and is detected in T-T antiparallel magnetization configuration at = 140 mT (middle panel) and in H-H antiparallel magnetization configuration at = −140 mT (bottom panel), with the external magnetic field tilted at = 30°.
Discussion
Recently, the nonlocal transport of the magnon spin current in antiferromagnets has been observed39–43, and an electrical detection of the magnon polarization with uniform precession has been realized by the coherent spin pumping effect in heterostructure with heavy-metal and antiferromagnetic materials30–32,44,45. In addition, coherent propagation of antiferromagnetic magnon has also been demonstrated using bulk antiferromagnets such as dysprosium orthoferrite (DyFeO3)46 or hematite (α-Fe2O3)47–49. However, the observation of coherently propagating magnon polarization of antiferromagnets has yet to be achieved so far. We overcome the issue by employing the SAF structure sandwiched by the Pt layer. Our demonstrated method of handedness control and electrical detection of antiferromagnetic magnons is a major step towards harnessing the full potentials of antiferromagnetic magnons, such as the high-speed spin dynamics, robustness against external perturbations, and the polarization degree of freedom.
A technical issue in our demonstration is that we need to apply a tilted magnetic field to the film plane. This is because it makes the time-averaged in-plane component of the pumped spin polarization finite and results in the generation of the dc ISHE voltage34. Polarization-resolved detection of magnon in a system with completely perpendicular magnetization is highly desired to enable demonstrations without the need for the magnetic field. One possible approach to achieve field-free detection is to utilize an inverse phenomenon of the spin anomalous Hall effect or the spin-orbit precession effect in ferromagnets50–55, which can convert the out-of-plane spin polarization to a charge current. We believe this makes the experimental system simpler and encourages the further investigation of the propagating magnon handedness.
In summary, we have demonstrated a polarization-resolved detection of propagating antiferromagnetic magnons in perpendicularly magnetized SAFs by using spintronics transducers based on the ISHE. Our experimental observations and numerical simulations provide clear evidence that the magnon handedness can be identified by the sign of the ISHE voltage from the SAF structure sandwiched by the HM layers with the same sign of the spin Hall angle. In addition, we have demonstrated the switching and electrical detection of the antiferromagnetic magnon handedness by tuning the excitation frequency. These demonstrations provide a new way to detect and control the spin polarization of magnon spin currents and open up the attractive possibility of antiferromagnet-based magnon devices.
Methods
Experimental setup
In the measurement setup (Fig. 2a), the microwave antenna was connected to a signal generator (Anritsu; 68347 C), and a pulse-modulated microwave was applied, whereas the Hall probe was connected to a lock-in amplifier (Stanford Research; SR830) to detect the transmission of propagating magnons along the -axis. The applied microwave power is 10 mW (10 dBm). To improve the signal-to-noise ratio, the measurements were repeated 4 times and averaged spectra are plotted in the figure.
Numerical simulations
For numerical study, we solved the Landau-Lifshitz-Gilbert equation with the unit vector of magnetization of lower and upper FM layers, and , given by
| 2 |
where , , and are the vacuum permeability, the gyromagnetic ratio, and the Gilbert damping constant, respectively. The effective magnetic field acting on the magnetization is given by
| 3 |
where is the external magnetic field, is the effective perpendicular magnetic anisotropy fields, and is the interlayer exchange field with the interlayer exchange coupling energy per unit area ( indicates the antiferromagnetic coupling), the saturation magnetization , and the thickness of FM layer . Here, we use a uniform precession motion for simplicity, which is sufficient to distinguish the contributions of the upper and lower HM layers. Thus, the dynamic dipolar interaction56 is excluded from the effective magnetic field. Equation (2) was numerically solved by substituting Eq. (3) by the fourth-order Runge-Kutta method with the time interval = 0.1 ps under the application of alternating magnetic field along the -axis, where = 1.0 mT.
Supplementary information
Acknowledgements
This work was partly supported by JSPS KAKENHI Grant Numbers JP22H01936, JP23K23204, JP23KK0093, JP24H02233, JP20H05665, and JP24H00007; a research granted from The Murata Science Foundation; MEXT Initiative to Establish Next-generation Novel Integrated Circuits Centers (X-NICS) Grant Number JPJ011438; and the Collaborative Research Program of the Institute for Chemical Research, Kyoto University.
Author contributions
Y.S. and T.O. conceived the project. Y.S. deposited the multilayers and fabricated the devices. Y.S. performed the measurements and data analysis. Y.S. and T.T. performed the simulation. All authors contributed jointly to the interpretation of the results. Y.S., T.T., and T.O. wrote the manuscript with the assistance of the other authors.
Peer review
Peer review information
Nature Communications thanks Edoardo Albisetti, Junxue Li and Yang Meng for their contribution to the peer review of this work. A peer review file is available.
Data availability
The data that support the findings of this work are available from the corresponding authors upon reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
The online version contains supplementary material available at 10.1038/s41467-024-54125-0.
References
- 1.Ralph, D. C. & Stiles, M. D. Spin transfer torques. J. Magn. Magn. Mater.320, 1190–1216 (2008). [Google Scholar]
- 2.Gambardella, P. & Miron, I. M. Current-induced spin-orbit torques. Philos. Trans. A Math. Phys. Eng. Sci.369, 3175–3197 (2011). [DOI] [PubMed] [Google Scholar]
- 3.Kruglyak, V. V., Demokritov, S. O. & Grundler, D. Magnonics. J. Phys. D. Appl. Phys.43, 264001 (2010). [Google Scholar]
- 4.Kajiwara, Y. et al. Transmission of electrical signals by spin-wave interconversion in a magnetic insulator. Nature464, 262–266 (2010). [DOI] [PubMed] [Google Scholar]
- 5.Chumak, A. V. et al. Direct detection of magnon spin transport by the inverse spin Hall effect. Appl. Phys. Lett.100, 082405 (2012). [Google Scholar]
- 6.Cornelissen, L. J., Liu, J., Duine, R. A., Youssef, J. B. & van Wees, B. J. Long-distance transport of magnon spin information in a magnetic insulator at room temperature. Nat. Phys.11, 1022–1026 (2015). [Google Scholar]
- 7.Chumak, A. et al. Magnon spintronics. Nat. Phys. 11, 453–461 (2015).
- 8.Yu, H., Xiao, J. & Schultheiss, H. Magnetic texture based magnonics. Phys. Rep.905, 1–59 (2021). [Google Scholar]
- 9.Schneider, T. et al. Realization of spin-wave logic gates. Appl. Phys. Lett.92, 022505 (2008). [Google Scholar]
- 10.Khitun, A., Bao, M. & Wang, K. L. Magnonic logic circuits. J. Phys. D Appl. Phys.43, 264005 (2010). [Google Scholar]
- 11.Khitun, A. & Wang, K. L. Non-volatile magnonic logic circuits engineering. J. Appl. Phys.110, 034306 (2011). [Google Scholar]
- 12.Sato, N., Sekiguchi, K. & Nozaki, Y. Electrical demonstration of spin-wave logic operation. Appl. Phys. Express6, 063001 (2013). [Google Scholar]
- 13.Jamali, M., Kwon, J. H., Seo, S.-M., Lee, K.-J. & Yang, H. Spin wave nonreciprocity for logic device applications. Sci. Rep.3, 3160 (2013). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Nakane, R., Tanaka, G. & Hirose, A. Reservoir computing with spin waves excited in a garnet film. IEEE Access6, 4462–4469 (2018). [Google Scholar]
- 15.Brächer, T. & Pirro, P. An analog magnon adder for all-magnonic neurons. J. Appl. Phys. 124, 152119 (2018).
- 16.Mahmoud, A. et al. Introduction to spin wave computing. J. Appl. Phys.128, 161101 (2020). [Google Scholar]
- 17.Papp, Á., Porod, W. & Csaba, G. Nanoscale neural network using non-linear spin-wave interference. Nat. Commun.12, 6422 (2021). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Wang, Q., Chumak, A. V. & Pirro, P. Inverse-design magnonic devices. Nat. Commun.12, 2636 (2021). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 19.Han, J., Zhang, P., Hou, J. T., Siddiqui, S. A. & Liu, L. Mutual control of coherent spin waves and magnetic domain walls in a magnonic device. Science366, 1121–1125 (2019). [DOI] [PubMed] [Google Scholar]
- 20.Fan, Y. et al. Coherent magnon-induced domain-wall motion in a magnetic insulator channel. Nat. Nanotechnol.18, 1000–1004 (2023). [DOI] [PubMed] [Google Scholar]
- 21.Baltz, V. et al. Antiferromagnetic spintronics. Rev. Mod. Phys.90, 015005 (2018). [Google Scholar]
- 22.Rezende, S. M., Azevedo, A. & Rodríguez-Suárez, R. L. Introduction to antiferromagnetic magnons. J. Appl. Phys.126, 151101 (2019). [Google Scholar]
- 23.Gurevich, A. D. & Melkov, G. A. Magnetization Oscillations and Waves (CRC Press, 1996).
- 24.Cheng, R., Daniels, M. W., Zhu, J.-G. & Xiao, D. Antiferromagnetic spin wave field-effect transistor. Sci. Rep.6, 24223 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Yu, W., Lan, J. & Xiao, J. Magnetic logic gate based on polarized spin waves. Phys. Rev. Appl.13, 024055 (2020). [Google Scholar]
- 26.Lan, J., Yu, W. & Xiao, J. Antiferromagnetic domain wall as spin wave polarizer and retarder. Nat. Commun.8, 178 (2017). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Oh, S.-H., Kim, S. K., Xiao, J. & Lee, K.-J. Bidirectional spin-wave-driven domain wall motion in ferrimagnets. Phys. Rev. B100, 174403 (2019). [Google Scholar]
- 28.Yu, W., Lan, J. & Xiao, J. Polarization-selective spin wave driven domain-wall motion in antiferromagnets. Phys. Rev. B98, 144422 (2018). [Google Scholar]
- 29.Daniels, M. W., Cheng, R., Yu, W., Xiao, J. & Xiao, D. Nonabelian magnonics in antiferromagnets. Phys. Rev. B98, 134450 (2018). [Google Scholar]
- 30.Li, J. et al. Spin current from sub-terahertz-generated antiferromagnetic magnons. Nature578, 70–74 (2020). [DOI] [PubMed] [Google Scholar]
- 31.Vaidya, P. et al. Subterahertz spin pumping from an insulating antiferromagnet. Science368, 160–165 (2020). [DOI] [PubMed] [Google Scholar]
- 32.Liu, Y. et al. Switching magnon chirality in artificial ferrimagnet. Nat. Commun.13, 1264 (2022). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Saitoh, E., Ueda, M., Miyajima, H. & Tatara, G. Conversion of spin current into charge current at room temperature: inverse spin-Hall effect. Appl. Phys. Lett.88, 182509 (2006). [Google Scholar]
- 34.Ando, K. et al. Angular dependence of inverse spin-Hall effect induced by spin pumping investigated in a Ni81Fe19Pt thin film. Phys. Rev. B78, 014413 (2008). [Google Scholar]
- 35.Yakushiji, K., Kubota, H., Fukushima, A. & Yuasa, S. Perpendicular magnetic tunnel junctions with strong antiferromagnetic interlayer exchange coupling at first oscillation peak. Appl. Phys. Express8, 083003 (2015). [Google Scholar]
- 36.Guan, Y. et al. Ionitronic manipulation of current-induced domain wall motion in synthetic antiferromagnets. Nat. Commun.12, 5002 (2021). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Liao, L. et al. Efficient orbital torque in polycrystalline ferromagnetic-metal/Ru/Al2O3 stacks: theory and experiment. Phys. Rev. B105, 104434 (2022). [Google Scholar]
- 38.Vlaminck, V. & Bailleul, M. Spin-wave transduction at the submicrometer scale: experiment and modeling. Phys. Rev. B81, 014425 (2010). [Google Scholar]
- 39.Yuan, W. et al. Experimental signatures of spin superfluid ground state in canted antiferromagnet Cr2O3 via nonlocal spin transport. Sci. Adv.4, eaat1098 (2018). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Lebrun, R. et al. Tunable long-distance spin transport in a crystalline antiferromagnetic iron oxide. Nature561, 222–225 (2018). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Han, J. et al. Birefringence-like spin transport via linearly polarized antiferromagnetic magnons. Nat. Nanotechnol.15, 563–568 (2020). [DOI] [PubMed] [Google Scholar]
- 42.Wimmer, T. et al. Observation of antiferromagnetic magnon pseudospin dynamics and the Hanle effect. Phys. Rev. Lett.125, 247204 (2020). [DOI] [PubMed] [Google Scholar]
- 43.Gückelhorn, J. et al. Observation of the nonreciprocal magnon Hanle effect. Phys. Rev. Lett.130, 216703 (2023). [DOI] [PubMed] [Google Scholar]
- 44.Boventer, I. et al. Room-temperature antiferromagnetic resonance and inverse spin-Hall voltage in canted antiferromagnets. Phys. Rev. Lett.126, 187201 (2021). [DOI] [PubMed] [Google Scholar]
- 45.Wang, H. et al. Spin pumping of an easy-plane antiferromagnet enhanced by dzyaloshinskii-moriya interaction. Phys. Rev. Lett.127, 117202 (2021). [DOI] [PubMed] [Google Scholar]
- 46.Hortensius, J. R. et al. Coherent spin-wave transport in an antiferromagnet. Nat. Phys.17, 1001–1006 (2021). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 47.Wang, H. et al. Long-distance coherent propagation of high-velocity antiferromagnetic spin waves. Phys. Rev. Lett.130, 096701 (2023). [DOI] [PubMed] [Google Scholar]
- 48.Hamdi, M., Posva, F. & Grundler, D. Spin wave dispersion of ultra-low damping hematite (α-Fe2O3) at GHz frequencies. Phys. Rev. Mater.7, 054407 (2023). [Google Scholar]
- 49.El Kanj, A. et al. Antiferromagnetic magnon spintronic based on nonreciprocal and nondegenerated ultra-fast spin-waves in the canted antiferromagnet α-Fe2O3. Sci. Adv.9, eadh1601 (2023). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Taniguchi, T., Grollier, J. & Stiles, M. D. Spin-transfer torques generated by the anomalous hall effect and anisotropic magnetoresistance. Phys. Rev. Appl.3, 044001 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 51.Humphries, A. M. et al. Observation of spin-orbit effects with spin rotation symmetry. Nat. Commun.8, 911 (2017). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Amin, V. P., Zemen, J. & Stiles, M. D. Interface-generated spin currents. Phys. Rev. Lett.121, 136805 (2018). [DOI] [PubMed] [Google Scholar]
- 53.Iihama, S. et al. Spin-transfer torque induced by the spin anomalous Hall effect. Nat. Electron.1, 120–123 (2018). [Google Scholar]
- 54.Baek, S.-H. C. et al. Spin currents and spin-orbit torques in ferromagnetic trilayers. Nat. Mater.17, 509–513 (2018). [DOI] [PubMed] [Google Scholar]
- 55.Wang, W. et al. Anomalous spin-orbit torques in magnetic single-layer films. Nat. Nanotechnol.14, 819–824 (2019). [DOI] [PMC free article] [PubMed] [Google Scholar]
- 56.Benson, H. & Mills, D. L. Spin waves in thin films; dipolar effects. Phys. Rev.178, 839–847 (1969). [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The data that support the findings of this work are available from the corresponding authors upon reasonable request.