Abstract
Some years ago, it was conjectured by the first author that the Chern–Simons perturbation theory of a 3-manifold at the trivial flat connection is a resurgent power series. We describe completely the resurgent structure of the above series (including the location of the singularities and their Stokes constants) in the case of a hyperbolic knot complement in terms of an extended square matrix (x, q)-series whose rows are indexed by the boundary parabolic -flat connections, including the trivial one. We use our extended matrix to describe the Stokes constants of the above series, to define explicitly their Borel transform and to identify it with state–integrals. Along the way, we use our matrix to give an analytic extension of the Kashaev invariant and of the colored Jones polynomial and to complete the matrix valued holomorphic quantum modular forms as well as to give an exact version of the refined quantum modularity conjecture of Zagier and the first author. Finally, our matrix provides an extension of the 3D-index in a sector of the trivial flat connection. We illustrate our definitions, theorems, numerical calculations and conjectures with the two simplest hyperbolic knots.
Introduction
Resurgence of Chern–Simons perturbation theory
Quantum Topology originated by Jones’s discovery of the famous polynomial invariant of a knot [Jon87], followed by Witten’s 3-dimensional interpretation of the Jones polynomial by means of a gauge theory with a topological (i.e., metric independent) Chern–Simons action [Wit89]. The connection between this topological quantum field theory and the Jones polynomial appears both on the level of the exact partition function and its perturbative expansion which both determine, and are determined by, the (colored) Jones polynomial. Indeed, the exact partition function on the complement of a knot colored by the defining representation of the gauge group at level k coincides with the value of the Jones polynomial at the complex root of unity . On the other hand, the perturbative expansion along the trivial flat connection is a formal power series whose coefficients are Vassiliev knot invariants which are determined by the colored Jones polynomial of a knot expanded as a power series in h where [BN95]. More generally, the loop expansion of the colored Jones polynomial is a formal power series introduced by Rozansky [Roz98] and further studied by Kricker [Kri, GK04], where plays the role of the monodromy of the meridian. An important feature of the power series is that it is determined by (but also uniquely determines) the colored Jones polynomial. Likewise, the power series is determined by (and determines) the Kashaev invariant of a knot [Kas95], interpreted as an element of the Habiro ring [Hab08].
In [Gar08a] the first author conjectured that the factorially divergent formal power series is resurgent, whose Borel transform has singularities arranged in a peacock pattern, and can be re-expanded in terms of the perturbative series corresponding to the remaining non-trivial flat connections of the Chern-Simons action. Although this is a well-defined statement, resurgence was a bit of the surprise and a mystery. We should point out that the above series are well-defined (for via formal Gaussian integration using as input an ideal triangulation of a 3-manifold [DG13], and for using the Kashaev invariant itself) and their coefficients are (up to multiplication by a power of ) algebraic numbers. However a numerical computation of their coefficients is difficult (about 280 coefficients can be obtained for the simplest hyperbolic knot), hence it is difficult to numerically study them beyond the nearest to the origin singularity of their Borel transform.
The resurgence question has attracted a lot of attention in mathematics and mathematical physics and some aspects of it were discussed by Jones [Jon09], Witten [Wit11], Gukov, Putrov and the third author [GMnP], Costin and the first author [CG11] and Sauzin [Sau15]. Further aspects of resurgence in Chern–Simons theory were studied in [Mn14, GMnP, GH18, GZ23, GZ24].
When , the resurgence structure of the series was given explicitly in [GGMn21], where it was found that the location of the singularities was arranged in a peacock pattern, and the Stokes constants were integers. The latter were fully described by an matrix . The passage from a vector of power series to a matrix is inevitable, and points out to the possibility that the non-perturbative partition function of a theory yet-to-be defined and its corresponding perturbative expansion is matrix-valued and not vector-valued, as was discussed in detail in [GZ24, GZ23]. Let us summarise some key properties of the matrix .
Linear q-difference equation. The entries of are q-series with integer coefficients defined for . The matrix is a fundamental solution of a linear q-difference equation of order r, and its rows are labeled by the set of nontrivial .
Asymptotics in sectors: q-Stokes phenomenon. The function as approaches zero in a fixed cone, has a full asymptotic expansion as a sum of power series in , times power series in . However, passing from one cone to an adjacent one changes the -series. The dependence of the asymptotics on the cone is the q-Stokes phenomenon, analogous to the well-studied Stokes phenomenon in the theory of linear differential equations with polynomial coefficients (see, e.g., [Sib90]). In our case, the q-Stokes phenomenon is a consequence of the fact that is a fundamental matrix solution to a linear q-difference equation.
Analyticity. The product of with a diagonal automorphy factor and with , when and , although defined for , equals to a matrix of state-integrals and hence it analytically extends to in the cut plane . A distinguished entry of , where is the geometric representation of a hyperbolic 3-manifold, is the Andersen–Kashaev state-integral [AK14]. The latter is often identified with the unknown partition function of complex Chern–Simons theory. Thus, analyticity of W is interpreted as a factorisation property of state-integrals, or as a matrix-valued holomorphic quantum modular form [GZ24, Zagb].
Borel resummation. The matrix coincides (in a suitable ray) to the Borel resummation of the matrix of perturbative series. In particular, the Borel resummation of the perturbative series is not a q-series as has been claimed repeatedly in some physics literature, but rather a bilinear combination of q-series and -series.1
Relation with the 3D-index. The 3D-index of Dimofte–Gaiotto–Gukov can be expressed bilinearly in terms of and . A detailed conjecture is given in see [GGMn23, Conj.4].
x-extension. There is an extension of the above invariants by a nonzero complex number x, which measures the monodromy of the meridian in the case of a knot complement, and extends the q-series to functions of (x, q), where x behaves like a Jacobi variable. This results in a matrix whose properties extend those of the matrix and were studied in detail in [GGMn23].
A summary of our results
Our goal is to describe the Stokes constants and the resurgent structure of the missing asymptotic series in terms of completing the matrix to a square matrix with one extra row (namely ) and column, whose distinguished entry is conjecturally the Gukov–Manolescu series [GM21] (evaluated at ), and the remaining series in the top row are the descendants of the Gukov-Manolescu series.
Along the way of solving the resurgence problem for the series, we solve several related problems, which we now discuss.
A q-series that sees . This is a problem raised by Gukov and his collaborators (see e.g. [GPPV20, GM21]). More precisely, our Resurgence Conjecture 5 implies that the asymptotics as and in a sector of each of the q-series of the top row of the matrix is a linear combination of the series which includes the series.
A matrix-valued holomorphic quantum modular form. In [GZ23] the first author and Don Zagier studied a matrix of q-series with rows indexed by nontrivial flat connections, and conjectured that the corresponding value of the cocycle 2 at
 , which a priori is an analytic function on , actually extends to the cut plane . A problem posed was to find an extension of the matrix which includes the trivial flat connection. We do so in Sects. 2.2, 3.2 and 4.1 for the and knots.
, which a priori is an analytic function on , actually extends to the cut plane . A problem posed was to find an extension of the matrix which includes the trivial flat connection. We do so in Sects. 2.2, 3.2 and 4.1 for the and knots.An exact form of the Refined Quantum Modularity Conjecture. In [GZ24] a Refined Quantum Modularity Conjecture was formulated. The conjecture was numerically motivated by a smoothed optimal summation of the divergent series , and the final result was a matrix-valued periodic function defined at the rational numbers. We conjecture that if we replace the smoothed optimal truncation by the median Borel resummation, all asymptotic statements in [GZ24] become exact equalities, valid for finite (and not necessarily large) range of the parameters.
An analytic extension of the Kashaev invariant and of the colored Jones polynomial. A consequence of the above conjecture is an exact formula for the Kashaev invariant at rational points as a linear combinations of three smooth functions, multiplied by the top row of .
Conjecture 1
For every knot K and every natural number N we have:
| 1 |
where for and (as in [GZ24, Eq. (3.7)]) and is a vector of elements of the Habiro ring (tensor ) evaluated at , with .
The vector for the knot appears in Sect. 4.2 of [GZ24] and also as the top row of the matrix of Eq. (92), and for the knot it appears in Section 4.3 as well as the top row of the matrix of Eq. (104) of ibid.
For the and the knots, we find numerically that , and in complete agreement with the results of [GZ24]. A corollary of (1) is the Volume Conjecture to all orders in 1/N as .
Conjecture 2
For every knot K, there is a neighborhood of 0 in the complex plane, such that for every natural number N and for , we have
| 2 |
where for and , where , , , and with .
For the and the knots, we find numerically that , and .
Since , the above conjecture specialises to Conjecture 1 when . Note also that the above conjecture implies the Generalised Volume Conjecture when is fixed and . Indeed, is nonzero and . Note finally that the above conjecture explains the failure of exponential growth when u is a rational multiple of , known for all knots from theorems 1.10 and 1.11 of [GL11], and theorem 5.3 of [Mur11] valid for the knot. Indeed, when for integers r and s with r/s near zero, then is a periodic function of N (see [Hab02a]), and so is since . Moreover, when N is a multiple of which explains why in that case the colored Jones polynomial does not grow exponentially.
An extension of the 3D-index. Our completed matrix proposes a computable extension of the 3D-index in the sector of the trivial connection , whose mathematical or physical definition is yet-to-be given.
Challenges
Our solution to the above problems brings a new challenge: namely, the new square matrix is actually a submatrix of a larger matrix , one with block triangular form which is a fundamental solution to the linear q-difference equation satisfied by the descendants of the colored Jones polynomials [GK23]. Already for the case of the knot, one obtains a matrix instead of the original matrix , or of the completed matrix.
A second challenge is to interpret the integers appearing in the new Stokes constants associated to the trivial flat connection as BPS indices in the dual 3d super conformal field theory. Incorporating the trivial connection in the 3d/3d correspondence of [DGG14] is subtle, but we expect our explicit results to give hints on this problem.
We should point out that although a proof of resurgence of the asymptotic series is still missing, the current paper (as well as the prior ones [GGMn21, GGMn23]) provide a complete description of their resurgent structure (namely the location of the singularities and a calculation of the Stokes constants) with precise statements, complemented by extensive numerical computations (including a numerical computation of the Stokes constants). In addition, we provide proofs of the algebraic properties of the matrices of q-series and (x, q)-series.
Illustration with the two simplest hyperbolic knots
We will illustrate our ideas by giving a detailed description of these matrices and of their algebraic, analytic and asymptotic properties for the case of the two simplest hyperbolic knots, the and the knots. Let us summarise our findings for the knot.
We complete the matrix of (x, q)-series to the matrix by adding the trivial flat connection. Our completed matrix is a fundamental solution of a third order linear q-difference equation.
A distinguished entry of is the Gukov–Manolescu series.
The matrix determines explicitly (but conjecturally) the Stokes constants and hence the resurgence structure of the three perturbative formal power series.
The matrix conjecturally computes an extension of the 3D-index in a sector of the trivial flat connection.
We complete the matrix of descendant Andersen–Kashaev state-integrals to a matrix by adding new state-integrals which are implicit in work of Kashaev and show their bilinear factorisation property.
As a second example, we present our results for the knot. In this case, we complete the matrix to a one, and use it to describe explicitly the Stokes constants of the 4 asymptotic series in half of the complex plane, thus completing the resurgence question of those asymptotic series. However, the knot reveals a new puzzle: the matrix is a block of a matrix whose rows are a fundamental solution to a sixth order linear q-difference equation, namely the one satisfied by the descendant colored Jones polynomial of the knot [GK23, Eq. (14)]. Although the homogeneous linear q-difference equation for the colored Jones polynomial is fourth order, the one for the descendant colored Jones polynomial is sixth order, and both equations are knot invariants. In the case of the knot, the extra block is a matrix of modular functions, in fact of the famous Rogers–Ramanujan modular q-hypergeometric series. We do not understand the labeling of the two excess rows and columns (e.g., in terms of -flat connections). Since the formulas for the matrix appear rather complicated, we will not give the x-deformation here, and postpone to a future publication a systematic definition of the matrix of (x, q)-series for all knots.
We should point out that the definition of the top row of the matrices for the knot, and the matrix for the knot, as well as an extension of the above results to the case of closed hyperbolic 3-manifolds have been taken from the thesis of the last author [Whe23].
The knot
A matrix of q-series
In this section we recall in detail what is known about the resurgence of the two asymptotic series of the knot, labeled by the geometric and the complex-conjugate flat connections. As explained in the introduction, the answer is determined by a matrix of q-series which was discovered in a long story and in several stages in a series of papers [GZ23, GK17, GGMn21, GGMn23]. A detailed description of the numerical discoveries and coincidences is given in [GZ23] and will not be repeated here. In that paper, the following pair of q-series for was introduced and studied by the first author and Zagier [GZ23]
| 3 |
where
| 4 |
These series were found to be connected to the knot in at least two ways, discussed in detail in [GZ23]. On the one hand, they express bilinearly the Andersen-Kashaev state-integral [GK17] and the total 3D-index of Dimofte-Gaiotto-Gukov [DGG13]. On the other hand, their radial asymptotics as (where is in a ray in the upper half-plane) is a linear combination of the two asymptotic series and of the Kashaev invariant, where is the geometric representation of the fundamental group of the knot complement and is the complex conjugate. The resurgence of the factorially divergent asymptotic series and , including a complete description of the Stokes automorphism and the Borel resummation was given by the first three authors in [GGMn21]. Surprisingly, the Stokes matrices were expressed bilinearly in terms of a matrix of explicit descendant q-series whose definition we now give. Consider the linear q-difference equation
| 5 |
In [GGMn21] it was shown that it has a basis of solutions for given by3
| 6 |
where are as in Equation (4). Note that , and that are Laurent series in q (with finitely many negative powers of q), meromorphic on with only possible pole at . We will extend them to analytic functions on by
| 7 |
The matrix is given by  where
where
| 8 |
coincides with the transpose of the matrix of [GGMn23, Eq. (48)] after interchanging of the two rows. A complete description of the resurgent structure of the series for , of their Borel resummation and their expression in terms of a matrix of state-integrals (with one distinguished entry being the Andersen–Kashaev state-integral [AK14]) was given in [GGMn21, GGMn23].
A matrix of q-series
In this section we define the promised matrix of q-series and give several algebraic properties thereof. In his thesis [Whe23], the fourth author introduced the series
| 9 |
which is the coefficient of in the -deformed q-series
| 10 |
which appears in [GZ23]. Here, . Adding the descendant variable , leads to the q-series
| 11 |
As in the case of for , it is a meromorphic function on with only possible pole at , and extends to an analytic function on satisfying (7) with .
The sequence is a solution of the inhomogenous equation obtained by replacing the right hand side of (5) by 1. This follows easily by using creative telescoping of the theory of q-holonomic functions implemented by Koutschan [Kou10].
We can assemble the three sequences of q-series into a matrix
| 12 |
whose bottom-right matrix is . The next theorem summarises the properties of .
Theorem 3
The matrix is a fundamental solution to the linear q-difference equation
| 13 |
has and satisfies the analytic extension
| 14 |
Proof
Equation (13) follows from the fact the last two rows of are solutions of the q-difference equation (5) and the first is a solution of the corresponding inhomogenous equation. Moreover, the block form of implies that where the last equality follows from [GGMn21, Eq. (14)]. Equation (14) follows from the fact that all three sequences of q-series satisfy (7).
We now give the inverse matrix of in terms of Appell-Lerch like sums. The latter appear curiously in the mock modular forms and the meromorphic Jacobi forms of Zwegers [Zwe01], and in [DMZ].
Theorem 4
We have
| 15 |
for the q-series () defined by
| 16 |
The q-series are expressed in terms of Appell-Lerch type sums:
| 17 |
Proof
Since is a matrix with determinant , it follows that the inverse matrix is given by (15) for the q-series () given by (16).
Observe that has first column , first row (1, 0, 1), and the remaining part is a companion matrix. It follows that its inverse matrix has first column and first row (1, 1, 0). This, together with (13) implies that
| 18 |
It follows that satisfy the first order inhomogeneous linear q-difference equation
| 19 |
Let denote the right hand side of the top Equation (17). Then we have
Therefore is independent of m. Moreover, . The top part of Equation (17) follows.
Likewise, let denote the right hand side of the bottom part of Equation (17). Then we have
Therefore is independent of m. Moreover, . Equation (17) follows.
The asymptotic series
The knot has three asymptotic series for corresponding to the trivial flat connection , the geometric flat connection and its complex conjugate . The asymptotic series for are defined in terms of perturbation theory of a state-integral [DG13] and can be computed via formal Gaussian integration in a way that was explained in detail in [GGMn21, GZ24] and will not be repeated here. They have the form
| 20 |
where
| 21 |
with being the hyperbolic volume of , and with is a power series with algebraic coefficients with first few terms
| 22 |
(a total of 280 terms have been computed), while .
We now discuss the new series corresponding to the zero volume trivial flat connection. This series can be defined and computed (for any knot) using either the colored Jones polynomial or the Kashaev invariant. Let us recall how this works.
Let denotes the Jones polynomial colored by the n-dimensional irreducible representation of , and normalised to 1 at the unknot. Setting , one obtains a power series in h, whose coefficient of is a polynomial in n of degree at most k. In other words, we have
| 23 |
where depends on the knot and, as the knot varies, defines a Vassiliev invariant of type (i.e., degree) i [BN95]. Then, the perturbative series is given by
| 24 |
With this definition, to compute the coefficient of in , one needs to compute the first k colored Jones polynomials for up to , polynomially interpolate and extract the coefficient . An efficient computation of the colored Jones polynomial is possible if one knows a recursion relation with respect to n (such a relation always exists [GL05]) together with some initial conditions. This gives a polynomial time algorithm to compute .
An alternative method is the so-called loop expansion of the colored Jones polynomial
| 25 |
where and is the Alexander polynomial of the knot. This expansion was introduced by Rozansky [Roz98] (see also Kricker [Kri, GK04]) and it is related to the Vassiliev power series expansion (23) by
| 26 |
Then the perturbative series is given in terms of the loop expansion by
| 27 |
as follows from the above equations together with the fact that .
A third method uses a theorem of Habiro [Hab02b, Hab08] which lifts the Kashaev invariant of a knot to an element of the Habiro ring . There is a canonical ring homomorphism defined by , which sends to and the image of the lifted element of the Habiro ring under this homomorphism equals to the series . For the case of the knot, the corresponding element of the Habiro ring is given by
| 28 |
and its expansion when gives the power series with first few terms
| 29 |
We end this section with a comment. Going back to the case of a general knot, it was shown in [GK23] that the colored Jones polynomial is equivalent (in the sense of knot invariants) to a descendant sequence of colored Jones polynomials and of Kashaev invariants (indexed by the integers) which is q-holonomic. These descendant Kashaev invariants play a key role in extending matrices of periodic functions whose rows and columns are indexed by nonrtivial flat connections to a matrix that includes the trivial flat connection. This is explained in detail in [GZ24].
Borel resummation and Stokes constants
In this section we discuss the asymptotic expansion as of the vector G(q) of q-series and relate it to the vector of the asymptotic series, where
| 30 |
with given in (3), and the additional series given in (9).
The three power series , can be resummed by Borel resummation. On the other hand, according to the resurgence theory, the value of the Borel resummation of an asymptotic power series depends crucially on the argument of the expansion variable. If the Borel transform of the power series has a singular point located at , the values of the Borel resummation of the power series whose expansion variable has an argument slightly greater and less than the angle differ by an exponentially small quantity, called the Stokes discontinuity. Usually the difference is identical with the Borel resummation of another power series in the theory, a phenomenon called the Stokes automorphism.
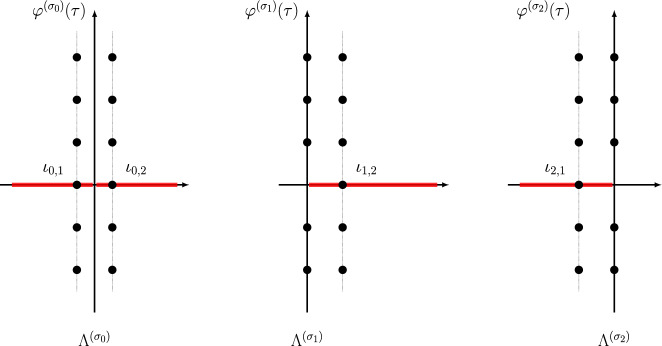
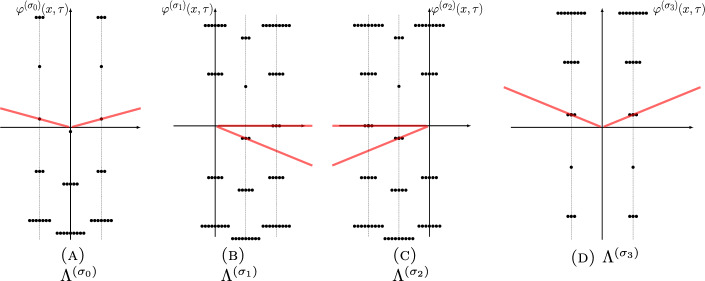
In the case of the power series , , the singularities of the Borel transforms of , were already studied in [GGMn21, GGMn23], and they are located at
| 31 |
as shown in the middle and the right panels of Fig. 1, while the singularities of the Borel transform of are located at (see also [Gar08a, Conj. 4])
| 32 |
as shown in the left panel of Fig. 1, where
| 33 |
All the rays (Stokes rays) passing through the singularities in the union
| 34 |
form a peacock pattern, cf. Fig. 2, and they divide the complex plane of Borel transform into infinitely many cones. The Borel resummation of the vector is only well-defined within one of these cones.
Fig. 1.
Singularities of the Borel transforms of for of the knot . Red lines are (some) Stokes rays
Fig. 2.
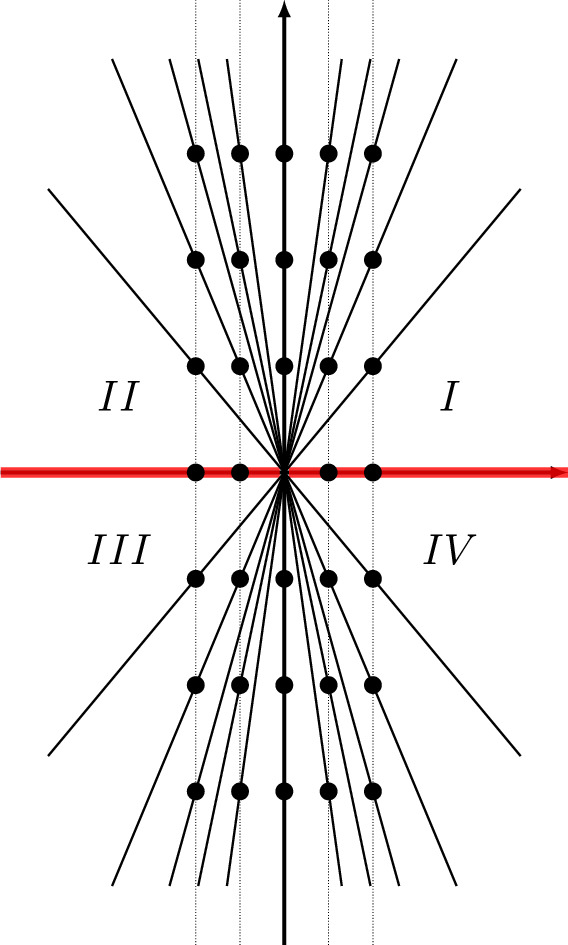
Stokes rays and cones in the -plane for the 3-vector of asymptotic series of the knot . Red lines are (some) Stokes rays
Recall that the Borel transform of a Gevrey-1 power series
| 35 |
is defined by
| 36 |
If it analytically continues to an -analytic function along the ray where , we define the Borel resummation by the Laplace integral
| 37 |
The Borel resummation of the trans-series is defined to be
| 38 |
In the following we will also use the notation when the argument of is in the cone R and it is a continuous function of .
Coming back to the vector of q-series G(q), we find that the asymptotic expansion of G(q) when and in a cone R can be expressed in terms of . Moreover, this asymptotic expansion can be lifted to an exact identity between q-series and linear combinations of Borel resummation of multiplied by power series in (thought of as exponentially small corrections) with integer coefficients. This is the content of the following conjecture.
Conjecture 5
For every cone and every , we have
| 39 |
where
| 40 |
and is a matrix of (resp., )-series if (resp., ) with integer coefficients that depend on R.
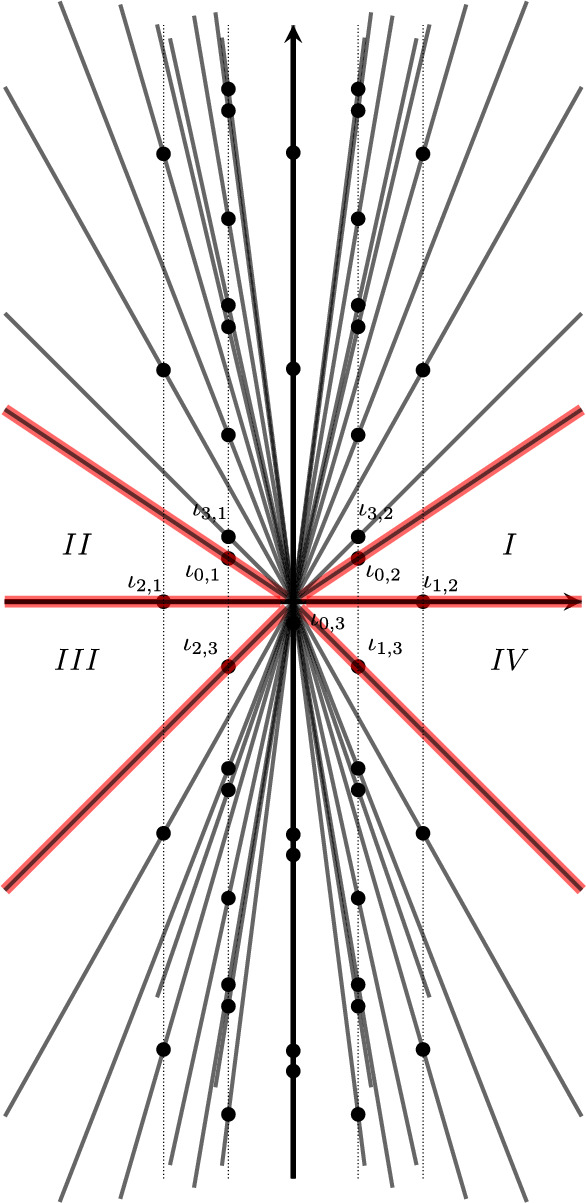
As in [GGMn21, GGMn23], we pick out in particular four of these cones, located slightly above and below the positive or the negative real axis, labeled in clockwise direction by I, II, III, IV as indicated in Fig. 2. We work out the exact matrices in these four cones.
Conjecture 6
Equation (39) holds in the cones where the matrices are given in terms of as follows
| 41a |
| 41b |
| 41c |
| 41d |
We now discuss the Stokes automorphism. To any singularity in the Borel plane located at , we can associate a local Stokes matrix
| 42 |
where is the elementary matrix with (i, j)-entry 1 () and all other entries zero, and is the Stokes constant. Let us assume the locality condition that no two Borel singularities share the same argument, or if there are, their Stokes matrices commute. This is indeed the case in our example. Then for any ray of angle , the Borel resummations of with whose argument is raised slight above () or lowered sightly below () are related by the following formula of Stokes automorphism
| 43 |
Because of the locality condition, we don’t have to worry about the order of the product of local Stokes matrices.
More generally, consider two rays and whose arguments satsify , we define the global Stokes automorphism
| 44 |
where both sides are analytically continued smoothly to the same value of . The global Stokes matrix satisfies the factorisation property [GGMn21, GGMn23]
| 45 |
where the ordered product is taken over all the local Stokes matrices whose arguments are sandwiched between and they are ordered with rising arguments from right to left.
Given (39) with explicit values of for , in general we can calculate the global Stokes matrix via
| 46 |
Here in the subscript of the global Stokes matrix on the left hand side, R stands for any ray in the cone. For instance, we find that the global Stokes matrix from cone I anti-clockwise to cone II is
| 47 |
This Stokes matrix has the block upper triangular form
| 48 |
Let us note that this form implies that () form a closed subset under Stokes automorphisms (this was called in [GMn] a “minimal resurgent structure”). They are controled by the submatrix of in the bottom right and one can verity that it is indeed the Stokes matrix in [GGMn21]. In addition we can also extract Stokes constants (, ) responsible for Stokes automorphisms into from Borel singularities in the upper half plane, and collect them in the generating series
| 49 |
We find
| 50 |
Similarly, we find that the global Stokes matrix from cone III anti-clockwise to cone IV is
| 51 |
It also has the form as (48), and the submatrix of in the bottom right is the Stokes matrix given in [GGMn21]. We also extract Stokes constants (, ) responsible for Stokes automorphisms into from Borel singularities in the lower half plane, and collect them in the generating series
| 52 |
We find
| 53 |
We can also use (46) to compute the global Stokes matrix and we find
| 54 |
Note that this can be identified as associated to the ray and it can be factorised as
| 55 |
Since the local Stokes matrices and commute, the locality condition is satisfied. We read off the Stoke discontinuity formulas
| 56 |
with
| 57 |
and the second identity has already appeared in [GH18, GGMn21].
Finally, in order to compute the global Stokes matrix , we need to take into account that the odd powers of on both sides of (39) give rise to additional factors when one crosses the branch cut at the negative real axis, and (46) should be modified by
| 58 |
and we find
| 59 |
Similarly this can be identified as associated to the ray and it can be factorised as
| 60 |
Note that the local Stokes matrices and also commute. We read off the Stokes discontinuity formulas
| 61 |
where the second identity has already appeared in [GGMn21].
The Andersen–Kashaev state-integral
In this section we briefly recall the properties of the state-integral of Andersen–Kashaev for the knot [AK14, Sect. 11.4], defined by
| 62 |
Here, is Faddeev’s quantum dilogarithm [Fad95], in the conventions of e.g. [AK14, Appendix A]. With this choice of contour, the integrand is exponentially decaying at hence the integral is absolutely convergent. State-integrals have several key features:
They are analytic functions in .
Their restriction to factorises bilinearly as finite sum of a product of a q-series and a -series, where and ; see [BDP14, Pas12, GK17].
Their evaluation at positive rational numbers also factorises bilinearly as a finite sum of a product of a periodic function of and a periodic function of ; see [GK15].
State-integrals are equal to linear combinations of the median Borel summation of asymptotic series.
State-integrals come with a descendant version which satisfies a linear q-difference equation.
Let us explain these properties for the state-integral (62). The integrand is a quasi-periodic meromorphic function with explicit poles and residues. Moving the contour of integration above, summing up the residue contributions, and using the fact that there are no contributions from infinity, one finds that [GK17, Cor.1.7]
| 63 |
When is a positive rational number, the quasi-periodicity of the integrand, together with a residue calculation leads to a formula for given in [GK15]. More generally, in [GGMn21] we considered the descendant integral
| 64 |
where and the contour is asymptotic at infinity to the horizontal line where but is deformed near the origin so that all the poles of the quantum dilogarithm located at
| 65 |
are above the contour. These integrals factorise as follows:
| 66 |
The above factorisation can be expressed neatly in matrix form. Indeed, let us define
| 67 |
Using the q-difference equation (13), it is easy to see that and hence the domain of is independent of the integers and . Equation (66) implies that are given by the matrix (for ), up to left-multiplication by a matrix of automorphy factors.
Finally we discuss the relation between the Borel summation of the two asymptotic series for and the descendant state-integrals. Since the Borel transform of those series may have singularities at the positive real axis, we denote by their median resummation given by the average of the two Laplace transforms to the left and to the right of the positive real axis. Then, we have
| 68 |
A new state-integral
In the previous section, we saw how the matrix of products of q-series and -series (6) coincides with a matrix of state-integrals. Having found the q-series (9) which complement the series (6), it is natural to search for a new state-integral which factorises in terms of all three q-series for and their -versions. Upon looking carefully, the series for were produced from the Andersen–Kashaev state-integral because its integrand had a double pole, hence the contributions came from expanding (10) up to . If we expanded up to , we would capture the new series . Hence the problem is to find a state-integral of the whose integrand has poles of order 3. After doing so, one needs to understand how this story, which seems a bit ad hoc and coincidental to the knot, can generalise to all knots. It turns out that such a state-integral existed in the literature for many years, and in fact was devised by Kashaev [Kas97] as a method to convert the state-sums of the Kashaev invariants into state-integrals, using as a building block the Faddeev quantum dilogarithm function at rational numbers, multiplied by . Incidentally, similar integrals have appeared in [KMn16] and more recently in the work of two of the authors on the topological string on local ; see [GMn, Eq. 3.141]. The integrand of such state-integrals are meromorphic functions with the usual pole structure coming from the Faddeev quantum dilogarithm function, together with the extra poles coming from . The residues of the former give rise to products of q-series times -series, but the presence of of has two effects. On the one hand, it produces, in an asymmetric fashion, poles of the integrand of one order higher, contributing to sums of q-series or -series. On the other hand, the produced q and -series look like multidimensional Appell-Lerch sums. An original motivation for converting state-sum formulas for the Kashaev invariants into state-integral formulas was to use such an integral expression for a proof of the Volume Conjecture.
There are two examples that convert state-sums into state-integrals, one given by Kashaev in [Kas97] for the knot and further studied by Andersen–Hansen [AH06], and one in Kashaev–Yokota [KY] for the knot. In the case of the knot, the integral considered in [Kas97, AH06] is
| 69 |
For generic so that , the integrand has the following poles and zeros, all in the imaginary axis:
| 70 |
In the special case where where , which is the case where (69) is well-defined, the poles and zeros in the upper half plane conspire so that there are only finite many simple poles located at
| 71 |
and we can define the contour encircling these points as in Fig. 3(left). An application of the residue theorem gives that this integral calculates the Kashaev invariant of the knot,
| 72 |
Now, we can define a new analytic function by changing the contour of integration from to the horizontal contour slightly below the horizontal line ,
| 73 |
This is now defined for . Although both (69) and (73) share the same integrand, it has significant contributions from infinity in the upper half plane, so that we cannot deform the contour smoothly to the contour , and (69) and (73) are really different. On the other hand, the integrand does have vanishing contributions from infinity in the lower half plane. Consequently we can smoothly deform the new controur downwards, and collect the residues of the integrand on the lower half-plane, as shown in Fig. 3(right). This integral, in contrast to the Andersen–Kashaev state-integral, contains information about the trivial connection. In particular, we conjecture that, in the region of the complex -plane slightly above the positive real axis, the all-orders asymptotic of at is given by
| 74 |
Moreover, this can be upgraded to an exact asymptotic formula by using Borel resummation in the same region, and one has
| 75 |
It turns out that the change of contour in Fig. 3 implements the inversion of the Habiro series recently studied in [Par]: the integral over the contour leads to the Habiro series, while the integral over gives the “inverted” Habiro series, see also Sect. 3.4. This inversion between q-series and elements of the Habiro ring was observed 10 years ago by the first author in his joint work with Zagier [GZ23], under the informal name “upside-down cake”.
Fig. 3.
The contour appears in the integral formula (69) for the Kashaev invariant of the knot, and it encircles the N poles (71). By doing the integral along the contour and picking the poles in the lower half plane, one obtains a new state-integral with information about the trivial connection
A matrix of state-integrals
Having found a new state-integral whose asymptotics sees the asymptotic series , we now consider its descendants, and their factorisations to complete the story. The new state-integrals are defined as follows:
| 76 |
where is related to by and . The integration contour is chosen so that, at infinity, it is asymptotic to the line , where satisfies
| 77 |
This guarantees convergence of the integral. We choose so that all poles of the integrand in the lower half plane are below . Note that is the integral introduced in (73), so that the state-integrals with general are descendants of .
Theorem 7
The descendant state-integral (76) can be expressed in terms of the series (6), (11) as follows:
| 78 |
Proof
This follows by applying the residue theorem to the state-integral (76), along the lines of the proof of Theorem 1.1 in [GK17]. One closes the contour to encircle the poles in the lower half-plane, located at
| 79 |
The poles of the integrand come the poles and the zeros of the quantum dilogarithm as well as from the function. When they are triple (a double pole comes from the quantum dilogarithm and a simple pole from ), while those with are double, coming only from the quantum dilogarithm. The triple poles lead to the series . In order to obtain the final result, one also has to use the properties of under modular transformations, i.e.
| 80 |
Remark 8
The state-integral (76) can be evaluated for arbitrary rational values of by using the techniques of [GK15]. One finds for example, for ,
| 81 |
where V is the hyperbolic volume of .
Remark 9
Equation (75) can be written as
| 82 |
We now discuss an important analytic extension of the matrix defined for . We define
| 83 |
As in Sect. 2.5, we find that the domain of is independent of the integers and .
Theorem 10
extends to a holomorphic function on and equals to the matrix (for ), up to left-multiplication by a matrix of automorphy factors.
Proof
For the bottom block of four entries, this result is already known from [GGMn21, GGMn23], and it follows from (66) as was discussed in Sect. 2.5. The top two non-trivial entries of for are given by
| 84 |
In view of Theorem 7 and (66) they can be written as a sum of state-integrals and , multiplied by holomorphic factors. This proves the theorem.
The x-Variable
In this section we discuss an extension of the results of Sect. 2 adding an x-variable. In the context of the nth colored Jones polynomial, corresponds to an eigenvalue of the meridian in the asymptotic expansion of the Chern–Simons path integral around an abelian representation of a knot complement. In the context of the state-integral of Andersen-Kashaev [AK14], the x-variable is the monodromy of a peripheral curve. The corresponding state-integral factorises bilinearly into holomorphic blocks, which are functions of (x, q) and [BDP14]. In the context of quantum modular forms, x plays the role of a Jacobi variable.
The corresponding perturbative series are now x-deformed (see [GGMn23, Sect. 5.1]), but there are some tricky aspects of this deformation that we now discuss. The critical points of the action, after exponentiation, lie in a plane curve S in (the so-called spectral curve) defined over the rational numbers, where is equipped with coordinate functions x and y. The field of rational functions of S (assuming S is irreducible, or working with one component of S at a time) can be identified with where is the defining polynomial of S. The coefficients of the perturbative series are elements of and the perturbative series are labeled by the branches of the projection corresponding to (with discriminant , a rational function on S). Each such branch defines locally an algebraic function satisfying the equation , which gives rise to an embedding of the field of to the field of algebraic functions obtained by replacing y by . For each such branch , the perturbative series has the form
| 85 |
where . The volume is also a function of x given explicitly as a sum of dilogarithms and products of logarithms.
In the above discussion it is important to keep in mind that the asymptotic series (85) are labeled by branches of the finite ramified covering . Going around a loop in x-space that avoids the finitely many ramified points will change the labeling of the branches, and correspondingly of the asymptotic series. In the present paper (as well as in [GGMn23]), we define the asymptotic series in a neighborhood of of the geometric representation, and we do not discuss the x-monodromy question.
In the case of the knot, the asymptotic series associated to the geometric, and the conjugate flat connections are given by
| 86 |
with and
| 87 |
The corresponding perturbative series are defined by
| 88 |
where
| 89 |
with being a solution to the equation . Note that when , , and , the latter defined in Sect. 2.3.
The series
We begin by discussing the perturbative series which is a formal power series in whose coefficients are rational functions of x with rational coefficients. The series is defined by the right hand side of Equation (25) after setting . One way to compute the -th coefficient of that series is by computing the colored Jones polynomial, expanding in n and h as in (23) and then resumming as in (26), taking into account the fact that the latter sum is a rational function. An alternative way is by using Habiro’s expansion of the colored Jones polynomials [Hab02b] (see also [Hab08])
| 90 |
where are the Habiro polynomials of the knot K and is the nth colored Jones polynomial. The latter can be efficiently computed using a recursion (which always exists [GL05]) together with initial conditions. This is analogous to applying the WKB method to a corresponding linear q-difference equation [DGLZ09, Gar08b]. We comment that the colored Jones polynomials of a knot K have a descendant version defined by [GK23]
| 91 |
Correspondingly, the Kashaev invariant has a descendant version (an element of the Habiro ring) and the asymptotic series have a descendant version defined for all integers m in [GK23], which we will not use in the present paper.
Going back to the case of the knot, we have
| 92 |
and the corresponding perturbative series is given by .
A matrix of (x, q)-series
We now extend the results of Sect. 2.2 by including the Jacobi variable x which, on the representation side, determines the monodromy of the meridian of an representation .
Our first task is to define the matrix . For , we define
| 93 |
Our series contain as a special case the series in [GM21, Par20, Par]
| 94 |
We assemble these (x, q)-series into a matrix
| 95 |
whose bottom-right matrix is . The properties of are summarised in the next theorem.
Theorem 11
The matrix is a fundamental solution to the linear q-difference equation
| 96 |
has and satisfies the analytic extension
| 97 |
Proof
The proof is analogous to the proof of Theorem 3. Equation (96) follows quickly using the q-hypergeometric expressions and noting that has a boundary term so satisfies an inhomogenous version. The block form again reduces the calculation of the determinant of to a calculation of the determinant of given in [GGMn23]. Equation (97) follows from the symmetry of the q-hypergeometric functions
| 98 |
The Appell-Lerch like sums again appear in the inverse of . The proof is again completely analogous to the proof of Theorem 4.
Theorem 12
We have
| 99 |
for the q-series defined by
| 100 |
The q-series are expressed in terms of Appell-Lerch type sums:
| 101 |
Proof
Given the block form of and the determinant calculated previously in Theorem 11, Equation (100) follows from taking the matrix inverse. Observe that again has first column and first row (1, 0, 1). It follows that its inverse matrix has first column and first row (1, 1, 0). This, together with (96), implies that
| 102 |
which implies that satisfy the first order inhomogeneous linear q-difference equation
| 103 |
Let denote the right-hand side of the first Equation (101). Then we have
Therefore is independent of m. Moreover, for or for . Equations (101) follows from analytic continuation.
Now if we take the inverse of we can get similar identities for .
Corollary 13
| 104 |
| 105 |
Borel resummation and Stokes constants
In this section we extend the discussion in Sect. 2.4 to include x-deformation. We analyse the asymptotic expansion as and of the (x, q)-series presented in Sect. 3.2 and relate them to the -asymptotic series given in Sect. 3.1. For this purpose, it is more convenient to introduce the decorated (x, q)-series
| 106 |
where
| 107 |
They satisfy the recursion relation in m
| 108 |
where and means the inhomogeneous term is only present for . In addition, as well as also satisfy the q-difference equations with respect to x
| 109 |
where and means the inhomogeneous term is only present for . Note that when , (109) reduces to the inhomogeneous -polynomial in [GGMn23]. The associated decorated matrix is given by
| 110 |
and it has
| 111 |
We will focus on the vector of (x, q)-series
| 112 |
which is defined for and satisfies by
| 113 |
We will write
| 114 |
and we will show that the asymptotic expansion of in the limit is related to the vector of asymptotic series
| 115 |
with corrections given by where
| 116 |
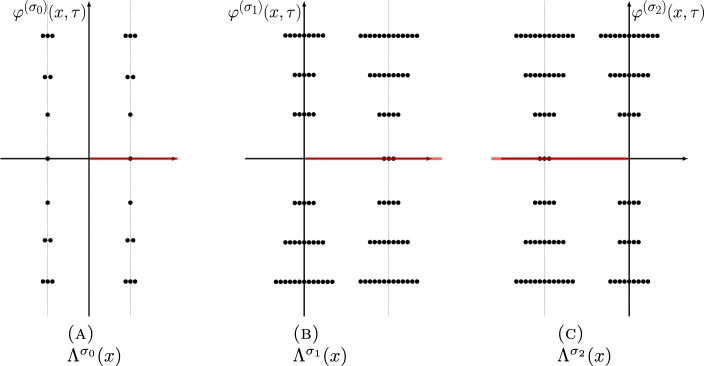
The asymptotic series can be resummed by Borel resummation. As we have explained in Sect. 2.4 the value of the Borel resummation depends on the singularities of the Borel transform of . The positions of these singular points, denoted collectively as , are smooth functions of x, and in the limit they are equal to defined in (34). When x is near 1, which is the regime we will be interested in, each singular point in splits to a finite set of points located at , where takes value in a finite subset of that depends on i, j, k. These singular points are aligned on a line and are apart from each other by a distance . We illustrate this schematically in Fig. 4. The complex plane of is divided to infinitely many cones by rays passing through these singular points, and the Borel resummation of , denoted by , is only well-defined within a cone R.
Fig. 4.
Singularities of the Borel transforms of for of the knot . Here we take small and real x. Red lines are some Stokes rays
We conjecture that the asymptotic expansion in the limit of the vector of (x, q)-series can be expressed in terms of . Furthermore, in each cone, the asymptotic expansion can be upgraded to exact identities between and linear transformation of Borel resummation of up to exponentially small corrections characterised by and .
Conjecture 14
For every , every cone and every we have
| 117 |
where
| 118 |
and is a matrix of (resp., )-series if (resp., ) with coefficients in that depend on R.
To illustrate examples of , we pick four of these cones, located slightly above and below the positive or negative real axis, labeled in counterclockwise direction by I, II, III, IV, cf. Fig. 5.
Fig. 5.
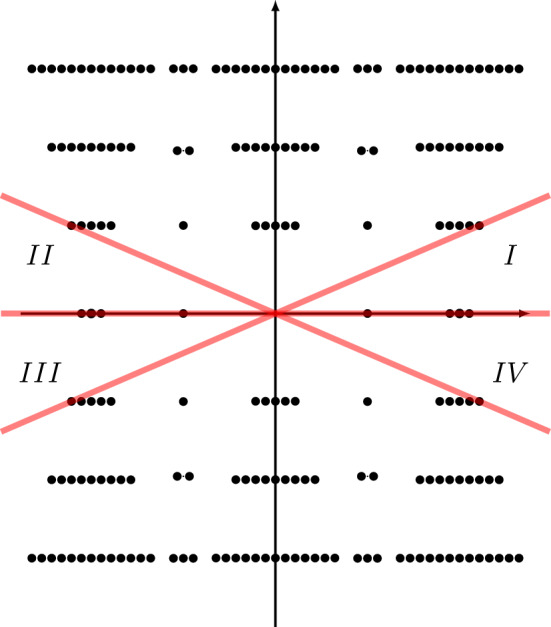
Stokes rays and cones in the -plane for the 3-vector of asymptotic series of the knot . Here we take small and real x
Conjecture 15
Equation (117) holds in the cones where the matrices are given in terms of as follows
| 119a |
| 119b |
| 119c |
| 119d |
Remark 16
It is sometimes stated in the literature that the Gukov–Manolescu series is obtained by “resumming” the perturbative series associated to the trivial connection, although it is not always clear what “resumming” means in that context. The above conjecture shows that, generically, involves the Borel resummation of all perturbative series , , as well as non-perturbative corrections in .
We now discuss the Stokes automorphism of the Borel resummation . The discussion is similar to the one in Sect. 2.4. To any singular point of the Borel transform of locatd at , we can associate a local Stokes matrix
| 120 |
where is the elementary matrix with (i, j)-entry 1 () and all other entries zero, and is the Stokes constant. Let us again assume the locality condition. Then for any ray of angle , the Borel resummations of with whose argument is raised slightly above () or sightly below () are related by the following formula of Stokes automorphism
| 121 |
Because of the locality condition, we don’t have to worry about the order of product of local Stokes matrices.
In addition, given two rays and whose arguments satisfy , we define the global Stokes matrix by
| 122 |
where both sides are analytically continued smoothly to the same value of . The global Stokes matrix satisfies the factorisation property [GGMn21, GGMn23]
| 123 |
where the ordered product is taken over all the local Stokes matrices whose arguments are sandwiched between and they are ordered with rising arguments from right to left.
Given (117) with explicit values of for , in general we can calculate the global Stokes matrix via
| 124 |
For instance, we find the global Stokes matrix from cone I anti-clockwise to cone II is
| 125 |
This Stokes matrix has the block upper triangular form
| 126 |
One can verify that the submatrix of in the bottom right is the Stokes matrix in [GGMn21]. In addition we can also extract Stokes constants () responsible for Stokes automorphisms into from Borel singularities in the upper half plane, and collect them in the generating series
| 127 |
We find
| 128 |
Similarly, we find the global Stokes matrix from cone III anti-clockwise to cone IV is
| 129 |
It also has the form as (126). This, together with the same phenomenon in the upper half plane, implies that () form a minimal resurgent structure. The submatrix of in the bottom right is identical to the Stokes matrix given in [GGMn21]. We also extract Stokes constants (, ) responsible for Stokes automorphisms into from Borel singularities in the lower half plane, and collect them in the generating series
| 130 |
And we find
| 131 |
We can also use (124) to compute the global Stokes matrix and we find
| 132 |
Note that this can be identified as , associated to the ray , and it can be factorised as
| 133 |
Since the local Stokes matrices and commute, the locality condition is satisfied. We read off the Stoke discontinuity formulas
| 134 |
They reduce properly to (56) in the limit, and the second identity has already appeared in [GGMn21].
Finally, in order to compute the global Stokes matrix , we need to take into account that the odd powers of on both sides of (117) give rise to additional factors when one crosses the branch cut at the negative real axis, and (124) should be modified by
| 135 |
and we find
| 136 |
Similarly this can be identified as associated to the ray and it can be factorised as
| 137 |
Note that the local Stokes matrices and also commute. We read off the Stokes discontinuity formulas
| 138 |
| 139 |
They reduce properly to (61) in the limit, and the second identity has already appeared in [GGMn21].
state-integrals
In parallel to the discussion in Sects. 2.6 and 2.7, we now introduce a new state-integral which depends on , but also on a variable u. Let us consider the state-integral
| 140 |
where the contour of integral is not specified yet. The integrand reduces to that of (69) in the limit . For generic so that , the integrand has the following poles and zeros
| 141 |
We can choose for the integral the contour in the upper half plane that wraps the following poles, as in the left panel of Fig. 3,
| 142 |
By summing over the residues of these poles, the integral evaluates as follows
| 143 |
where we defined , as in [GGMn23, Eq. (2)]. When this is none other than the colored Jones polynomial of the knot
| 144 |
Alternatively, we can choose for the integral the contour as in the right panel of Fig. 3, which is asymptotic to a horizontal line slightly below , but deformed near the origin in such a way that all the poles
| 145 |
are below the contour . Let denote the corresponding state-integral. Similar to the discussion in Sect. 2.6, as the integrand has non-trivial contributions from infinity in the upper half plane, the two integrals and are different. On the other hand, since the integrand does have vanishing contributions from infinity in the lower half plane, we can smoothly deform the contour downwards so that can be evaluated by summing over residues at the poles , and we find
| 146 |
where are defined as in (100) with Roman letters A, B, C replaced by caligraphic letters . As mentioned above, the change of integration contour implements the Habiro inversion of [Par]: the integration over gives the Habiro series (144), while the integration over involves , which was interpreted in [Par] as an inverted Habiro series. This contribution comes from the poles in the lower half-plane.
The integral can also be identified with the Borel resummation of the perturbative series for . By inverting the matrix in (117), we can also express the Borel resummation in any cone R in terms of combinations of (x, q)- and -series, and they can be then compared with the right hand side of (146). For instance, in the cones I and IV respectively, we find
| 147a |
| 147b |
This also implies that for positive real ,
| 148 |
Finally, we can introduce the descendants of the integral as follows
| 149 |
The integrand has the same poles and zeros as in (141). To ensure convergence, the contour needs slight modification: it is asymptotic to a horizontal line slightly below , and it is deformed near the origin in such a way that all the poles (145) are below the contour . Similarly, by smoothly deforming the contour downwards we can evaluate this integral by summing up residues of all the poles in the lower half plane, and we find
| 150 |
An analytic extension of the colored Jones polynomial
In this section we discuss a Borel resummation formula for the colored Jones polynomial of the knot. The latter is defined by
| 151 |
Let be in a small neighborhood of the origin in the complex plane. It is related to and by
| 152 |
Then u is near 0, then x is close to 1, which is the regime that we studied in Sect. 3.3, and is close to 1/N. Note that is the analogue of n/k in [Guk05], and here we are considering a deformation from the case of .
Experimentally, we found that in cones I and IV respectively, we have
| 153a |
| 153b |
where . This, together with Conjecture 6 implies
| 154 |
which is Conjecture 2 for the knot.
We now make several consistency checks of the above conjecture. The first is that equation (154) is invariant under complex conjugation which moves from cone I to cone IV. The second is that the conjecture implies the Generalised Volume Conjecture. Indeed, in the limit
| 155 |
the right hand side of (153a),(153b) are dominated by the first term. If we keep only the exponential, this is the generalised Volume Conjecture [Mur11, Guk05]. Recall from [Mur11], the generalised Volume Conjecture reads, for u in a small neighborhood of origin such that ,
| 156 |
where and , with y a solution to . By the identification , and since A(x) is identical with H(y, x) (up to ), one can check that (153a),(153b) imply (156).
The -knot
A matrix of q-series
The trace field of the knot is the cubic field of discriminant , with a distinguished complex embedding (corresponding to the geometric representation of ), its complex conjugate and a real embedding . The knot has three boundary parabolic representations whose associated asymptotic series for correspond to the three embeddings of the trace field. In [GGMn21] these asymptotic series were discussed, and a matrix of q-series was constructed to describe the resurgence properties of the asymptotic series. The matrix is a fundamental solution to the linear q-difference equation [GGMn21, Eq. (23)]
| 157 |
and it is defined by4
| 159 |
where for
| 160 |
and
| 161 |
The Habiro polynomials and the descendant Kashaev invariants
The addition of the asymptotic series corresponding to the trivial flat connection requires a extension of the matrix . This is consistent with the fact that the colored Jones polynomial of satisfies a third order inhomogenous linear q-difference equation, and hence a 4th order homogeneous linear q-difference equation. However, the descendant colored Jones polynomials of satisfy a 5th order inhomogeneous recursion [GK23, Eq. (14)], hence a 6th order homogeneous recursion. In view of this, we will give a matrix of q-series and we will use its block to describe the resurgent structure of the asymptotic series .
Let us recall the Habiro polynomials, the descendant colored Jones polynomials, the descendant Kashaev invariants and their recursions. The Habiro polynomials are given by terminating q-hypergeometric sums
| 162 |
(see Habiro [Hab02a] and also Masbaum [Mas03]) where is the q-binomial function. In [GS06], it was shown that satisfies the linear q-difference equation
| 163 |
with initial conditions for and . Actually, the above recursion is valid for all integers if we replace the right hand side of it by . The recursion for the Habiro polynomials of , together with Equation (91) and [Kou10], gives that , which is the descendant colored Jones polynomial defined by (91), satisfies the linear q-difference equation
| 164 |
Using the values , , it follows that the right hand side of the above recursion is for all m. Setting , and renaming by , we arrive at the inhomogenous 5-th order q-difference equation satisfied by the descendant Kashaev invariant [GK23, Eq. (14)]
| 165 |
valid for all integers m. Our aim is to define an explicit fundamental matrix solution to the corresponding sixth order homogenous linear q-difference equation (165). To do so, we define a 2-parameter family of deformations of the Habiro polynomials which satisfy a one-parameter deformation of the recursion of the Habiro polynomials. Motivated by the q-hypergeometric expression (162) for the Habiro polynomials, we define deformations of the Habiro polynomials, for , with appropriate normalisations
| 166 |
where and . These deformations satisfy the recursion
| 167 |
obtained from (163) by replacing to . Note that when , we cannot solve for in terms of for as discussed in [Par].5 It follows that the function
| 168 |
is an inhomogenous solution of Equation (165). In particular, for we have
| 169 |
We see that is convergent for and all and for and all . Moreover, for and for . Substituting for f in the LHS of Equation (165) gives a RHS of
| 170 |
In particular, for Equation (170) is
| 171 |
and for Equation (170) is
| 172 |
A matrix of q-series
We now have all the ingredients to define the promised matrix of q-series for . Let us denote by the coefficient of in the expansion of . We now define
| 173 |
The next theorem relates the above matrix to the linear q-difference equation (165).
Theorem 17
The matrix is a fundamental solution to the linear q-difference equation
| 174 |
and has
| 175 |
Proof
Equation (174) follows from Equations (171), (172). The determinant is calculated using the determinant of and by considering the limiting behavior in m.
The construction of this matrix has used special q-hypergeometric formulae for the Habiro polynomials. However, this construction can be carried out more generally and will be developed in a later publication.
There is a similar, however more complicated, relation between with the first row replaced by Appell-Lerch type sums and as in Theorem 4. This indicates these matrices could come from the factorisation of a state-integral. We will not give this relation, since we do not need it for the purpose of resurgence. We will however, discuss an important block property of the matrix , after a gauge transformation. Namely, we define:
| 176 |
The first few terms of the matrix are given by
| 177 |
We next discuss a block structure for the gauged-transform matrix (176).
Conjecture 18
When , the matrix has a block form
| 178 |
Our next task is to identify the and the blocks of the matrix . The first observation is that the block is related to the matrix given in [GGMn21]. The second is that the block is related to modular forms. This is the content of the next conjecture.
Conjecture 19
The block for of of (176) has the form
| 179 |
(where is the matrix of [GGMn21] reviewed in Sect. 4.1) and the block has the form
| 180 |
where
| 181 |
are the famous Rogers-Ramanujan functions.
The remaining two entries of the block are higher weight vector-valued modular forms associated to the same -representation as the Rogers-Ramanujan functions, discussed for example in [Whe23]. Part of this conjecture is proved in Appendix A.
This block decomposition fits nicely with the “dream” in [Zaga]. Here we do see the interesting property that the and blocks contain some non-trivial gluing information. This implies that the diagrammatic “short exact sequence” will not always “split”. The block decomposition also implies that the resurgent structure of the asymptotic series associated to the q-series in the block in the top left does not depend on the other blocks. This block and in-particular the second column of will be the focus of Sect. 4.4.
We now consider the analytic properties of the function
| 182 |
If the work [GZ23] extended to the matrix, it would imply that the function W extends to an analytic function on . This would follow from an identification of W with a matrix of state-integrals, as was done in Sect. 2.7 for the knot. Although we do not know of such a matrix of state-integrals, we can numerically evaluate W when is near the positive real axis and test the extension hypothesis. Doing so for we have
| 183 |
where whereas
| 184 |
Borel resummation and Stokes constants
The knot has four asymptotic series for corresponding to the trivial, the geometric, the conjugate, and the real flat connections respectively, denoted respectively by for . Similar to the knot, the asymptotic series for can be defined in terms of a perturbation theory of a state-integral [KLV16, AK14] using the standard formal Gaussian integration as explained in [DGLZ09, GGMn21], and they have been computed in [GGMn21] with more than 200 terms. Let () be the roots to the algebraic equation
| 185 |
with numerical values
| 186 |
The asymptotic series for have the universal form6
| 188 |
where and
| 189 |
Their numerical values are given by
| 190 |
where the common absolute value of the imaginary parts of is the . Finally the power series with have coefficients in the number field and their first few coefficients are given by
| 191 |
The additional new series corresponds to the zero volume () trivial flat connection. As exlained in Sect. 2.3, it can be computed using the colored Jones polynomial or the Kashaev invariant. The first few terms are
| 192 |
We are interested in the Stokes automorphism of the Borel resummation of the 4-vector of asymptotic series
| 193 |
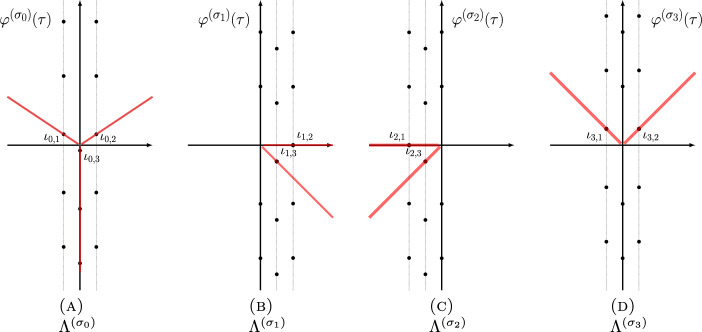
First of all, the Borel transform of each asymptotic series () has rich patterns of singularities. Similar to the case of knot discussed in Sect. 2.4, the Borel transforms of , have singularities located at
| 194 |
as shown in the right three panels of Fig. 6, while the Borel transform of have singularities located at (some of these singular points are actually missing as we will comment in the end of the section.)
| 195 |
as shown in the left most panel of Fig. 6, where
| 196 |
To any singularity located at in the union
| 197 |
we can associate a local Stokes matrix
| 198 |
where is the elementary matrix with (i, j)-entry 1 () and all other entries zero, and is the Stokes constant. Then the Borel resummation along the rays raised slight above and below the angle are related by the Stokes automorphism
| 199 |
where
| 200 |
and the locality condition is assumed.
Fig. 6.
Singularities of Borel transforms of for of the knot . Red lines are (some) Stokes rays
More generally, for two rays and whose arguments satisfy , we can define the global Stokes matrix as in (44), and it also satisfies the factorisation property (45). Since the factorisation is unique [GGMn21, GGMn23], we only need to compute finitely many global Stokes matrices in order to extract all the local Stokes matrices associated to the infinitely many singularities in and thus the corresponding Stokes constants. In particular, we can choose four cones I, II, III, IV slightly above the positive and the negative real axes as shown in Fig. 7, and compute the four global Stokes matrices
| 201 |
where a cone R in the subscript means any ray inside the cone.
Fig. 7.
Stokes rays and cones in the -plane for the 4-vector of asymptotic series of the knot . Red lines are (some) Stokes rays
On the other hand, each of the global Stokes matrices in (201) has the block upper triangular form
| 202 |
The sub-matrices in the right bottom have been worked out in [GGMn21]. For later convenience, we write down two of the four reduced global Stokes matrices,
| 203a |
| 203b |
In addition, as seen from Fig. 6, there are no singularities along the positive and negative real axes in relevant for ; all the singular points in are either in the upper half plane beyond the cones I, II or in the lower half plane beneath the cones III, IV. Consequently we only need to compute the first row of two Stokes matrices and . For this purpose, we find the following.
Conjecture 20
For every cone and every , we have
| 204 |
where () are (resp., )-series if (resp., ) with integer coefficients that depend on R.
A more elegant way to present is by the row vector , and it can be expressed in terms of a matrix
| 205 |
Conjecture 21
Equation (204) holds in the cones where the ,-series are given in terms of through (236) which are as follows
| 206a |
| 206b |
| 206c |
| 206d |
Equations (204), together with the reduced Stokes matrices for (), allow us to calculate entries in the first row of and by
| 207 |
In the following we list the first few terms of these and -series. In the upper half plane
| 208a |
| 208b |
| 208c |
In the lower half plane
| 209a |
| 209b |
| 209c |
Finally, we can factorise the global Stokes matrices to obtain local Stokes matrices associated to individual singular points in and extract the associated Stokes constants. The Stokes constants for () are already given in [GGMn21, GGMn23]. We collect the Stokes contants for in the generating series
| 210 |
And we find that in the upper half plane
| 211a |
| 211b |
| 211c |
while in the lower half plane
| 212a |
| 212b |
| 212c |
We comment that the results of and indicate that there are actually no singular points of the type in the upper half plane, but they exist in the lower half plane. Also note that the constant terms in and are Stokes constants associated to the singular points (). The Stokes constants associated to have already been computed in [GGMn21, GGMn23]. We can assemble all these Stokes constants in a matrix
| 213 |
which matches (after some changes of signs) the one appearing in [GZ24, Eq. (40)].
(x, q)-series
In this section we extend the results of Sect. 4.1 by including the Jacobi variable x. Recall that the matrix 7is a fundamental solution to the linear q-difference equation
| 216 |
and it is defined by
| 214 |
where the holomorphic blocks are given by
| 217a |
| 217b |
| 217c |
where for and and
| 218a |
| 218b |
| 218c |
To these series we wish to add an additional series which satisfies the inhomogenous q-difference equations of the descendant coloured Jones polynomial (164). This can be easily constructed using the deformations of the Habiro polynomials (166). We find a solution
| 219 |
(compare with Equation (91)) where and or and , and is the coefficient of in the expansion of . In particular, for we have
| 220 |
and we see the (x, q)-series coincides with in [Par20, Par].
This series can be included as the first row of a matrix of (x, q)-series. The latter might be related to the factorisation of the state integral proposed in Sect. 4.8.
However, we find that the matrices above and below the reals have different quantum modular co-cycles related by inversion. This implies that to do a full discussion on resurgence one needs to understand the monodromy of this q-holonomic system. Both these issue will be explored in later publications. For now, we give a description of the Stokes matrices restricted to in the upper half plane.
x-version of Borel resummation and Stokes constants
In this section we discuss the x-deformation version of Sect. 4.4. The asymptotic series for are extended to series with coefficients in . The series for are defined in terms of perturbation theory of a deformed state-integral [AK14] and they have been computed with about 200 terms for many values of x in [GGMn23]. Let () be three roots to the equation
| 221 |
ordered such that they reduce to (186) in the limit . The series () can be uniformly written as8
| 223 |
where and
| 224 |
The power series are
| 225 |
with and
| 226 |
The additional series , as in Sect. 3.1, can be computed either from the colored Jones polynomial or by using Habiro’s expansion of the colored Jones polynomials. We find
| 227 |
where the power series reads
| 228 |
We are interested in the Stokes automorphisms in the upper half plane of the Borel resummation of the 4-vector of asymptotic series
| 229 |
when x is close to 1. The singular points of the Borel transform of , collectively denoted as , are smooth functions of x and they are equal to in (197) in the limit . When x is slightly away from 1, each singular point in splits to a finite set of points located at , . We illustrate this schematically in Fig. 8. The complex plane of is divided by rays passing through these singular points into infinitely many cones. We will then pick the cones I and II located slightly above the positive and negative real axes, and compute the global Stokes matrix from cone I to cone II defined by
| 230 |
where
| 231 |
The global Stokes matrix factorises uniquely into a product of local Stokes automorphisms associated to each of the singular points in the upper half plane, from which the individual Stokes constants can be read off.
Fig. 8.
Singularities of Borel transforms of for of the knot . Here we take small and real x. Red lines are (some) Stokes rays
The global Stokes matrix in (230) also has the block upper triangular form
| 232 |
The sub-matrices in the right bottom have been worked out in [GGMn23], and they are given by
| 233a |
where
| 234 |
and is given by (216). To calculate the first row of , we use the additional holomorphic block .
Conjecture 22
For every cone and every , we have
| 235 |
where () are -series with coefficients in depending on the cone R.
We present in terms of the row vector , and it can be expressed in terms of a matrix
| 236 |
Conjecture 23
Equation (204) holds in the cones where the - series are given in terms of through (236) which are as follows
| 237a |
| 237b |
Equations (235), together with the reduced Stokes matrices for (), allow us to calculate entries in the first row of by
| 238 |
In the following we list the first few terms of these -series.
| 239 |
Finally, we can factorise the global Stokes matrices to obtain local Stokes matrices associated to individual singular points in and extract the associated Stokes constants. The Stokes constants for () are already given in [GGMn21, GGMn23]. We collect the Stokes contants for in the generating series
| 240 |
And we find that
| 241 |
An analytic extension of the Kashaev invariant and the colored Jones polynomial
In this section we discuss an analytic extension of the Kashaev invariant and of the colored Jones polynomial of the knot, illustrating Conjectures 1 and 2.
Recall that the colored Jones polynomial of the is given by
| 242 |
where
| 243 |
Let u be in a small neighborhood of the origin. It is related to and by
| 244 |
Then x is close to 1 and is close to 1/N. Note that
| 245 |
is the analogue of n/k in [Guk05], and here we are considering a deformation from the case of . We also have
| 246 |
When x is positive real, are not Borel summable along the positive real axis. Depending on whether is in the first or the fourth quadrant, we have
| 247a |
| 247b |
The two equations (247a), (247b) are related by the Stokes discontinuity formula
| 248 |
Combined, they imply
| 249 |
which is the assertion of Conjecture 2.
A new state-integral for the knot?
In the case of the figure eight knot, the new state-integral was obtained by first writing down an integral formula for its colored Jones polynomial, in Habiro form, and then changing the integration contour to pick the contribution from the poles in the lower half plane. This led in particular to the “inverted” Habiro series in (146). Although we do not have a similar complete theory for the knot, we can however write down an integral formula for its colored Jones polynomial which lead, after a change of contour, to the corresponding inverted Habiro series. In fact, it is possible to write such an integral for all twist knots (the knot corresponds to ).
Let us then consider the colored Jones polynomial of the twist knot in Habiro’s form [Mas03]:
| 250 |
where
| 251 |
It is easy to see that (250) can be written as a double contour integral
| 252 |
where
| 253 |
and the contours encircle the poles of the form (71) in the upper complex planes of the z and the w variables, respectively. We can now deform the contour to pick the poles in the lower half planes of z, w. The contribution from the simple poles of the functions in those half planes can be easily computed, and one finds in this way the inverted Habiro series,
| 254 |
This gives a general formula for all twist knots which agrees with the results of [Par] for (the knot) and (the knot).
It might be possible to find appropriate integration contours so that the integral of converges and provides the sought-for new state-integral which sees the series , as it happened in the case of the knot. In the case of the knot, these contours do exist and lead to a well-defined integral. We expect that an evaluation of such an integral by summing over the appropriate set of residues will give the inverted Habiro series (254), together with additional contributions, as in (146). However, the fact that the integrals are two-dimensional makes them more difficult to analyze. We expect to come back to this problem in the near future.
Acknowledgements
The authors would like to thank Jorgen Andersen, Sergei Gukov, Rinat Kashaev, Maxim Kontsevich, Pavel Putrov and Matthias Storzer for enlightening conversations. S.G. wishes to thank the University of Geneva for their hospitality during his visit in the summer of 2021. The work of J.G. has been supported in part by the NCCR 51NF40-182902 “The Mathematics of Physics” (SwissMAP). The work of M.M. has been supported in part by the ERC-SyG project “Recursive and Exact New Quantum Theory” (ReNewQuantum), which received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation program, grant agreement No. 810573. The work of C.W. has been supported by the Max-Planck-Gesellschaft.
Appendix A. q-Series Identities
In this appendix we will sketch the proofs of some q-series identities that appear in Conjecture 19. Since this not one of the main themes of the paper, our presentation will be rather brief. Our proofs of the q-hypergeometric identities will use the algorithmic approach of the Wilf-Zeilberger theory (see [WZ92, PWZ96]) and the computer implementation by Koutschan [Kou10]).
We outline part of the proof of Conjecture 19 for , namely
| 255 |
Proof
The definition of gives that
| 256 |
where
| 257 |
with
| 258 |
Likewise, we define
| 259 |
where
| 260 |
Therefore, we have
| 261 |
This implies that the first equality in (255) follows from the specialization of
| 262 |
This in turn follows (using Equations (257) and (259)) from the following
| 263 |
Equation (258) expresses the two-variable q-holonomic function as a one dimensional sum of a three variable proper q-hypergeometric function. It follows from [Kou10] that the annihilator ideal of is generated by the recursion relations
| 264 |
| 265 |
This coincides with the annihilator ideal of . Thus, the equality (263) for with follows from the two special cases and , that is from the identities
| 266 |
The first one of the above identities is due to Euler and can be derived using generating functions of partitions. The second one follows from the q-holonomic system
| 267 |
This concludes the proof of the first identity in (255). The remaining two identities follow (using the above steps) from the following ones
| 268 |
This concludes the sketch of the proof of (255).
In the course of the proof, we came up with the following conjecture which expresses as -linear combinations of .
Conjecture 24
For we have:
| 269 |
Funding
Open access funding provided by University of Geneva.
Declarations
Conflict of interest
To the best of all authors’ knowledge, the submitted article has no Conflict of interest.
Footnotes
A similar phenomenon was observed by Hatsuda–Okuyama [HO15].
for a suitable diagonal matrix of weights.
defined here is one half of in [GGMn21].
Our agrees with the one defined in [Par] when , however differs when .
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- [AH06].Andersen, J.E., Hansen, S.K.: Asymptotics of the quantum invariants for surgeries on the figure 8 knot. J. Knot Theory Ramific. 15(4), 479–548 (2006) [Google Scholar]
- [AK14].Andersen, J.E., Kashaev, R.: A TQFT from Quantum Teichmüller theory. Commun. Math. Phys. 330(3), 887–934 (2014) [Google Scholar]
- [BDP14].Beem, C., Dimofte, T., Pasquetti, S.: Holomorphic blocks in three dimensions. JHEP 12, 177 (2014). arXiv:1211.1986 [Google Scholar]
- [BN95].Bar-Natan, D.: On the Vassiliev knot invariants. Topology 34(2), 423–472 (1995) [Google Scholar]
- [CG11].Costin, O., Garoufalidis, S.: Resurgence of the Kontsevich-Zagier series. Ann. Inst. Fourier (Grenoble) 61(3), 1225–1258 (2011) [Google Scholar]
- [DG13].Dimofte, T., Garoufalidis, S.: The quantum content of the gluing equations. Geom. Topol. 17(3), 1253–1315 (2013) [Google Scholar]
- [DGG13].Dimofte, T., Gaiotto, D., Gukov, S.: 3-manifolds and 3D indices. Adv. Theor. Math. Phys. 17(5), 975–1076 (2013) [Google Scholar]
- [DGG14].Dimofte, T., Gaiotto, D., Gukov, S.: Gauge theories labelled by three-manifolds. Commun. Math. Phys. 325(2), 367–419 (2014) [Google Scholar]
- [DGLZ09].Dimofte, T., Gukov, S., Lenells, J., Zagier, D.: Exact results for perturbative Chern-Simons theory with complex gauge group. Commun. Number Theory Phys. 3(2), 363–443 (2009) [Google Scholar]
- [DMZ].Dabholkar, A., Murthy, S., Zagier, D.: Quantum black holes, wall crossing, and mock modular forms (2012). arXiv:arXiv:1208.4074, Preprint
- [Fad95].Faddeev, L.: Discrete Heisenberg-Weyl group and modular group. Lett. Math. Phys. 34(3), 249–254 (1995) [Google Scholar]
- [Gar08a].Garoufalidis, S.: Chern-Simons theory, analytic continuation and arithmetic. Acta Math. Vietnam 33(3), 335–362 (2008) [Google Scholar]
- [Gar08b].Garoufalidis, S.: Difference and differential equations for the colored Jones function. J. Knot Theory Ramific 17(4), 495–510 (2008) [Google Scholar]
- [GGMn21].Garoufalidis, S., Jie, G., Mariño, M.: The resurgent structure of quantum knot invariants. Commun. Math. Phys. 386(1), 469–493 (2021) [DOI] [PMC free article] [PubMed] [Google Scholar]
- [GGMn23].Garoufalidis, S., Jie, G., Mariño, M.: Peacock patterns and resurgence in complex Chern-Simons theory. Res. Math. Sci. 10(3), 29 (2023) [Google Scholar]
-
[GH18].Gang, D., Hatsuda, Y.: S-duality resurgence in
 Chern-Simons theory. J. High Energy Phys. 2018(7), 1–24 (2018) [Google Scholar]
Chern-Simons theory. J. High Energy Phys. 2018(7), 1–24 (2018) [Google Scholar] - [GK04].Garoufalidis, S., Kricker, A.: A rational noncommutative invariant of boundary links. Geom. Topol. 8, 115–204 (2004) [Google Scholar]
- [GK15].Garoufalidis, S., Kashaev, R.: Evaluation of state integrals at rational points. Commun. Number Theory Phys. 9(3), 549–582 (2015) [Google Scholar]
-
[GK17].Garoufalidis, S., Kashaev, R.: From state integrals to
 -series. Math. Res. Lett. 24(3), 781–801 (2017) [Google Scholar]
-series. Math. Res. Lett. 24(3), 781–801 (2017) [Google Scholar] - [GK23].Garoufalidis, S., Kashaev, R.: The descendant colored Jones polynomials. Pure Appl. Math. Q. 19(5), 2307–2334 (2023) [Google Scholar]
-
[GL05].Garoufalidis, S., Lê, T.T.Q.: The colored Jones function is
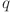 -holonomic. Geom. Topol. 9, 1253–1293 (2005). ((electronic))
-holonomic. Geom. Topol. 9, 1253–1293 (2005). ((electronic))
- [GL11].Garoufalidis, S., Lê, T.T.Q.: Asymptotics of the colored Jones function of a knot. Geom. Topol. 15(4), 2135–2180 (2011) [Google Scholar]
- [GM21].Gukov, S., Manolescu, C.: A two-variable series for knot complements. Quantum Topol. 12(1), 1–109 (2021) [Google Scholar]
- [GMn].Gu, J., Mariño, M.: Peacock patterns and new integer invariants in topological string theory (2021). arXiv:arXiv:2104.07437, Preprint
- [GMnP].Gukov, S., Mariño, M., Putrov, P.: Resurgence in complex Chern-Simons theory (2016). arXiv:arXiv:1605.07615, Preprint
- [GPPV20].Sergei Gukov, D., Pei, P.P., Vafa, C.: BPS spectra and 3-manifold invariants. J. Knot Theory Ramific. 29(2), 2040003, 85 (2020)
-
[GS06].Garoufalidis, S., Sun, X.: The
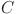 -polynomial of a knot. Algebr. Geom. Topol. 6, 1623–1653 (2006) [Google Scholar]
-polynomial of a knot. Algebr. Geom. Topol. 6, 1623–1653 (2006) [Google Scholar] - [Guk05].Gukov, S.: Three-dimensional quantum gravity, Chern-Simons theory, and the A-polynomial. Commun. Math. Phys. 255(3), 577–627 (2005) [Google Scholar]
-
[GZ23].Garoufalidis, S., Zagier, D.: Knots and their related
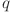 -series. SIGMA Symm. Integrab. Geom. Methods Appl. 19, 82 (2023) [Google Scholar]
-series. SIGMA Symm. Integrab. Geom. Methods Appl. 19, 82 (2023) [Google Scholar] - [GZ24].Garoufalidis, Stavros, Zagier, D.: Knots, perturbative series and quantum modularity. SIGMA Symm. Integrab. Geom. Methods Appl. 20, 055 (2024)
-
[Hab02a].Habiro, K.: On the quantum
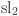 invariants of knots and integral homology spheres, Invariants of knots and 3-manifolds (Kyoto, 2001), Geom. Topol. Monogr., vol. 4, Geom. Topol. Publ., Coventry, pp. 55–68. (electronic) (2002)
invariants of knots and integral homology spheres, Invariants of knots and 3-manifolds (Kyoto, 2001), Geom. Topol. Monogr., vol. 4, Geom. Topol. Publ., Coventry, pp. 55–68. (electronic) (2002)
-
[Hab02b].Habiro, K.: On the quantum
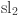 invariants of knots and integral homology spheres, Invariants of knots and 3-manifolds (Kyoto, 2001), Geom. Topol. Monogr., vol. 4, Geom. Topol. Publ., Coventry, pp. 55–68. (electronic) (2002)
invariants of knots and integral homology spheres, Invariants of knots and 3-manifolds (Kyoto, 2001), Geom. Topol. Monogr., vol. 4, Geom. Topol. Publ., Coventry, pp. 55–68. (electronic) (2002)
- [Hab08].Habiro, K.: A unified Witten-Reshetikhin-Turaev invariant for integral homology spheres. Invent. Math. 171(1), 1–81 (2008) [Google Scholar]
- [HO15].Hatsuda, Y.: Resummations and non-perturbative corrections. J. High Energy Phys. 2015(9), 1–29 (2015) [DOI] [PMC free article] [PubMed] [Google Scholar]
- [Jon87].Jones, V.: Hecke algebra representations of braid groups and link polynomials. Ann. Math. (2) 126(2), 335–388 (1987)
- [Jon09].Jones, V.: On the origin and development of subfactors and quantum topology. Bull. Am. Math. Soc. (N.S.) 46(2), 309–326 (2009)
- [Kas95].Kashaev, R.: A link invariant from quantum dilogarithm. Modern Phys. Lett. A 10(19), 1409–1418 (1995) [Google Scholar]
- [Kas97].Kashaev, R.: The hyperbolic volume of knots from the quantum dilogarithm. Lett. Math. Phys. 39(3), 269–275 (1997) [Google Scholar]
- [KLV16].Kashaev, R., Luo, F., Vartanov, G.: A TQFT of Turaev-Viro type on shaped triangulations. Ann. Henri Poincaré 17(5), 1109–1143 (2016) [Google Scholar]
- [KMn16].Kashaev, R., Mariño, M.: Operators from mirror curves and the quantum dilogarithm. Commun. Math. Phys. 346(3), 967–994 (2016). arXiv:1501.01014
- [Kou10].Koutschan, C.: HolonomicFunctions (user’s guide), Tech. Report 10-01, RISC Report Series, Johannes Kepler University Linz (2010)
- [Kri].Kricker, A.: The lines of the Kontsevich integral and Rozansky’s rationality conjecture (2000), arXiv:arXiv:math/0005284, Preprint
-
[KY].Kashaev, R., Yokota, Y.: On the Volume Conjecture for the knot
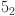 , Preprint (2012)
, Preprint (2012)
- [Mas03].Masbaum, G.: Skein-theoretical derivation of some formulas of Habiro. Algebraic Geometr. Topol. 3(1), 537–556 (2003) [Google Scholar]
-
[Mn14].Mariño, M.: Lectures on non-perturbative effects in large
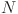 gauge theories, matrix models and strings. Fortsch. Phys. 62, 455–540 (2014). arXiv:1206.6272 [Google Scholar]
gauge theories, matrix models and strings. Fortsch. Phys. 62, 455–540 (2014). arXiv:1206.6272 [Google Scholar] - [Mur11].Murakami, Hitoshi: An introduction to the volume conjecture, Interactions between hyperbolic geometry, quantum topology and number theory, Contemp. Math., vol. 541, pp. 1–40. Amer. Math. Soc., Providence, RI (2011). arXiv:1002.0126
- [Par].Park, S.: Inverted state sums, inverted Habiro series, and indefinite theta functions. arXiv:arXiv:2106.03942
-
[Par20].Park, S.: Large color
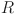 -matrix for knot complements and strange identities. arXiv:2004.02087
-matrix for knot complements and strange identities. arXiv:2004.02087
-
[Pas12].Pasquetti, S.: Factorisation of
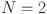 theories on the squashed 3-sphere. J. High Energy Phys. (4), 120 (2012)
theories on the squashed 3-sphere. J. High Energy Phys. (4), 120 (2012)
-
[PWZ96].Petkovsek, M., Wilf, H.S., Zeilberger, D.:
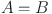 . A K Peters Ltd., Wellesley, MA,: ( With a foreword by Donald E. Knuth, With a separately available computer disk) (1996)
. A K Peters Ltd., Wellesley, MA,: ( With a foreword by Donald E. Knuth, With a separately available computer disk) (1996)
-
[Roz98].Rozansky, L.: The universal
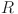 -matrix, Burau representation, and the Melvin-Morton expansion of the colored Jones polynomial. Adv. Math. 134(1), 1–31 (1998) [Google Scholar]
-matrix, Burau representation, and the Melvin-Morton expansion of the colored Jones polynomial. Adv. Math. 134(1), 1–31 (1998) [Google Scholar] - [Sau15].Sauzin, D.: Nonlinear analysis with resurgent functions. Ann. Sci. Éc. Norm. Supér. (4) 48(3), 667–702 (2015)
- [Sib90].Sibuya, Y.: Linear differential equations in the complex domain: problems of analytic continuation, Translations of Mathematical Monographs, vol. 82, American Mathematical Society, Providence, RI (1990). Translated from the Japanese by the author
-
[Whe23].Wheeler, C.: Modular
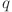 –difference equations and quantum invariants of hyperbolic three-manifolds, Ph.D. thesis, Rheinische Friedrich-Wilhelms-Universität Bonn (2023)
–difference equations and quantum invariants of hyperbolic three-manifolds, Ph.D. thesis, Rheinische Friedrich-Wilhelms-Universität Bonn (2023)
- [Wit89].Witten, E.: Quantum field theory and the Jones polynomial. Commun. Math. Phys. 121(3), 351–399 (1989) [Google Scholar]
- [Wit11].Witten, E.: Analytic continuation of Chern-Simons theory. AMS/IP Stud. Adv. Math. 50, 347–446 (2011). arXiv:1001.2933 [Google Scholar]
-
[WZ92].Wilf, H.S., Zeilberger, D.: An algorithmic proof theory for hypergeometric (ordinary and “
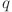 ’’) multisum/integral identities. Invent. Math. 108(3), 575–633 (1992) [Google Scholar]
’’) multisum/integral identities. Invent. Math. 108(3), 575–633 (1992) [Google Scholar] - [Zaga].Zagier, D.: From 3-manifold invariants to number theory, Lecture course (2021)
- [Zagb].Zagier, D.: Holomorphic quantum modular forms, In preparation
-
[Zwe01].Zwegers, S.: Mock
 -functions and real analytic modular forms,
-functions and real analytic modular forms,  -series with applications to combinatorics, number theory, and physics (Urbana, IL, 2000), Contemp. Math., vol. 291, pp. 269–277. Amer. Math. Soc., Providence, RI (2001)
-series with applications to combinatorics, number theory, and physics (Urbana, IL, 2000), Contemp. Math., vol. 291, pp. 269–277. Amer. Math. Soc., Providence, RI (2001)