Skip to main content
PMC home page
RSC Advances logoLink to RSC Advances
. 2024 Dec 19;14(54):39954–39967. doi: 10.1039/d4ra05974c

Numerical evaluation of bi-facial ZnO/MoTe2 photovoltaic solar cells with N-doped Cu2O as the BSF layer for enhancing VOCvia device simulation†

Arifuzzaman Rajib a,✉, Tapos Chandra Saha a, Md Mustafizur Rahman a, Hridoy Sarker a, Ruddro Dhali a, Md Sabbir Hossain Sumon a, Atowar Rahman b,✉
PMCID: PMC11657081  PMID: 39703733

Abstract

Molybdenum telluride (MoTe2) shows great promise as a solar absorber material for photovoltaic (PV) cells owing to its wide absorption range, adjustable bandgap, and lack of dangling bonds at the surface. In this research, a basic device structure comprising Pt/MoTe2/ZnO/ITO/Al was developed, and its potential was assessed using the SCAPS-1D software. The preliminary device exhibited a photovoltaic efficiency of 23.87%. The integration of a 100 nm thick nitrogen-doped copper oxide (N-doped Cu2O) layer as a hole transport/BSF layer improved the device performance of the MoTe2/ZnO photovoltaic solar cell (PVSC), increasing the open circuit voltage (VOC) from 0.68 V to 1.00 V and, consequently, its efficiency from 23.87% to 34.45%. Recombination and C–V analyses were conducted across various regions of the device with and without the BSF layer. The results of these analyses revealed that this improvement in the device performance mainly stemmed from a decrease in recombination losses at the absorber/BSF interface and an increase in the built-in potential of the device, resulting in improved VOC and photovoltaic efficiency. Additionally, the performance of the device in a bifacial mode was studied. The calculated bifacial factor (BF) values suggested that there were negligible additional losses affecting some parameters when the solar cell was under backside illumination and emphasized the potential for improved energy harvest in bifacial solar cells without significant drawbacks.

Molybdenum telluride (MoTe2) shows great promise as a solar absorber material for photovoltaic (PV) cells owing to its wide absorption range, adjustable bandgap, and lack of dangling bonds at the surface.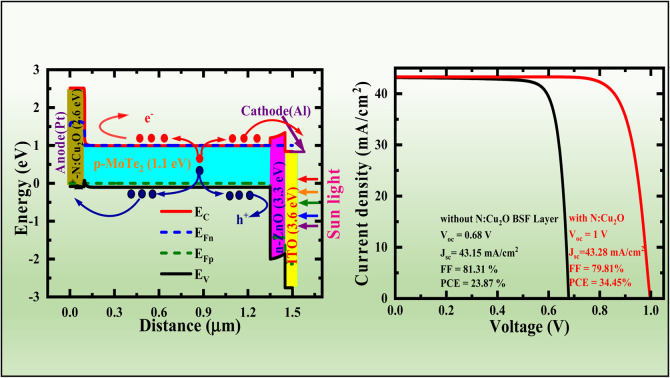graphic file with name d4ra05974c-ga.jpg

1. Introduction

Energy from non-renewable energy sources increases the temperature of the earth by the emission of SO2, CO2, and CH4 gases.1 The use of renewable resources, such as sunlight and wind, must be increased in order to bring down fossil fuel consumption. The relentless pursuit of sustainable energy sources has propelled significant advancements in the field of solar energy. Different technologies have been applied to utilize solar energy, but photovoltaic (PV) cells have received considerable attention from researchers due to their cost-effectiveness and availability.2–5 Nowadays, silicon-based solar cells dominate about 95% of the worldwide solar market. However, silicon (Si) possesses an indirect type bandgap, and its optical absorption is not as suitable as those of direct bandgap semiconductor materials. Industrial production of silicon wafers requires temperatures of about 1400 °C, and thick wafers are often used for commercial purposes, which increases costs and the amount of materials used.6–8 TFSCs have achieved notable progress in recent years, leading to the enhancement of cell performance and cost-effectiveness. TFSCs use a much thinner layer of semiconductor material in comparison with conventional silicon solar cells, making them cost-effective, lighter, and more flexible. The 2nd generation TFSCs are based on CdTe, CIGS, and lead chalcogenide PbX (X = S, Se). These are all direct bandgap semiconductors and have received considerable attention because of their exceptional performance in PV applications.9–12 High production costs, i.e., expensive materials and manufacturing processes associated with these technologies, primarily limit the commercialization of the 2nd generation TFSCs. Additionally, efficiency, reliability, and toxicity issues have hindered their widespread adoption in the market. These technologies have not yet achieved the same level of efficacy and long-term reliability as silicon-based solar cells, making them less attractive to commercial developers and investors.

To overcome these challenges, researchers have turned to earth-abundant, inexpensive, and eco-friendly materials like transition metal dichalcogenides (TMDCs) as the main absorber layer in solar cells. These materials exhibit excellent optical, mechanical, thermal, and electrochemical properties.13–16 TMDCs have a three-layer chalcogenide-transition metal-chalcogenide microstructure, in which very weak van der Waals forces form bonds between the layers. Therefore, doping can be easily achieved in order to tune their electrical and optical properties.17 Among the TMDCs, MoTe2 has garnered considerable attention as a promising absorber material for thin-film solar cells because it exhibits a p-type behavior with exceptional optoelectronic properties.18 In addition, it shows a bandgap in the range of 1 to 1.2 eV, superior optical absorption of ∼105 cm−1, and excellent carrier mobility.19 Very recently, the photovoltaic solar cell with perovskite as an absorber layer has been in focus due to its high band gap, high efficiency, and excellent stability.20–22

However, MoTe2 has not been explored well as an active layer for PV cells. In a recent study, a device structure of Cu2O/MoTe2/CdS showed a PV performance of 32.38% power conversion efficiency (PCE).19 However, toxic materials, such as cadmium, were used as window layers.19 In another study, a MoTe2/n-MoSe2 PV cell structure showed a cell performance of 26.97% PCE, which further improved to 28.75% PCE using a heavily doped N-doped Cu2O BSF layer.23 However, it is worth noting that the BSF layer is inefficient as most of the sunlight photons are absorbed by the MoSe2 window layer due to its very high absorption coefficient of ∼106 cm−1 and moderate bandgap Eg of ∼1.25 eV.24–27 Therefore, very few photons are reflected by the BSF layer. TFSCs can be fabricated using various methods, each with distinct advantages and applications, including sputtering, CVD, PVD, ALD, PLD, and epitaxial growth. Epitaxy, which achieves high crystallographic alignment between the deposited film and substrate lattice, employs techniques such as MBE, epitaxial CVD, and atomic layer epitaxy (ALE). Data in Table 1 indicates that the lattice mismatch at the ZnO/MoTe2 interface is 8.25%, while it is 19.43% at the MoTe2/N-doped Cu2O interface. This information aids in selecting an appropriate epitaxial growth technique for fabricating the proposed device structure with optimal crystallographic alignment among the layers.

Table 1. Lattice parameters of different layers and the percentage of lattice mismatches of their interfaces.

Layer Lattice parameters Reference Lattice mismatch (%)
a (Å) b (Å) c (Å)
MoTe2 3.53 3.53 13.96 28
ZnO 3.25 3.25 5.21 29 8.25 (ZnO/MoTe2)
N:Cu2O 4.29 4.29 4.29 30 19.43 (MoTe2/N:Cu2O)
Open in a new tab

These results suggest that PVSCs with MoTe2 as an absorber layer have great potential to produce non-toxic, low-cost, and high-performance PVSCs. However, the choice of the window layer is crucial. However, ZnO is a promising material as a window layer for its widespread use in Cu2O, c-Si, GaAs, and perovskite-based solar cells.31–34 From this point of view, electrically conductive and optically transparent material with a wide bandgap like ZnO is promising as a window layer for MoTe2-based PVSC.35–37 Here, an initial device structure of Pt/MoTe2/ZnO/ITO/Al has been constructed and extensively studied using the SCAPS-1D simulator. The research aims to identify the ideal device conditions for Pt/MoTe2/ZnO/ITO/Al photovoltaic solar cells (PVSCs). The device's performance has been analyzed via numerical simulations, considering variations in carrier concentration, thickness, and bulk defects of different constituent layers.

One of the major issues is the recombination of minority carriers at the back side, which limits the performance of MoTe2-based SCs.38 One way to minimize backside recombination and improve the performance is to passivate the backside of the device. In this simulation, N-doped Cu2O is incorporated into the initial device featuring the configuration of Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al to reduce the recombination of minority carriers and to enhance the collection of photo-generated holes at the back electrode. This is because the N-doped Cu2O shows high acceptor density and confirms better valence band energy alignment with MoTe2. The key performance parameters of the optimized PVSCs with different back metal work functions, different series and parallel resistances, and different operating temperatures are studied. To understand the potential of the device, the effect of incorporating ZnO as ETL and N-doped Cu2O as a BSF layer into the proposed device on the built-in potential at different junctions and the recombination rate at different interfaces is also studied. Finally, with an aim to further improve PV performance, one possible approach has been proposed and Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al PVSCs were tested as bifacial PVSCs.

2. Device design and numerical simulation

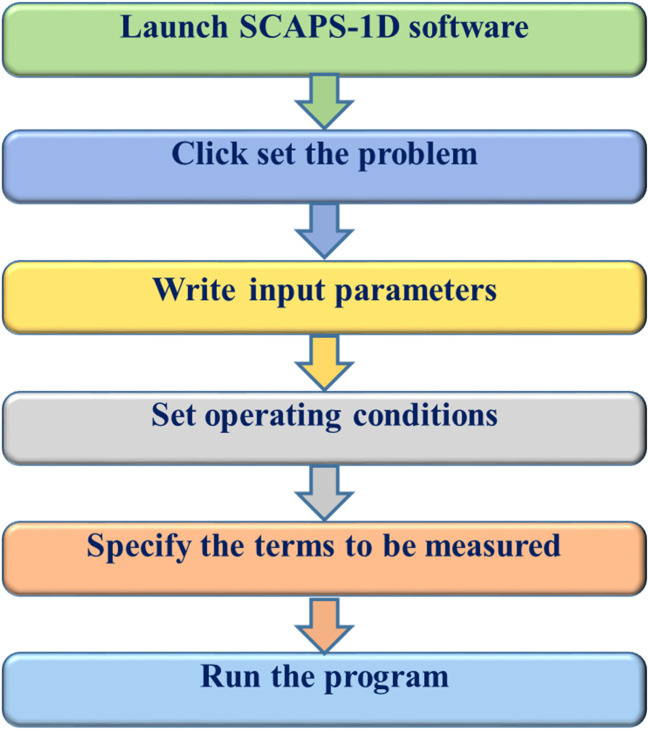
This study proposes a bi-facial ZnO/MoTe2 device with an N-doped Cu2O as a BSF layer, aiming to achieve an enhanced VOC through device simulation. This innovative design leverages the unique properties of the selected materials to maximize device performance. The numerical simulations of the proposed Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al PVSCs were performed by using SCAPS-1D software taking 1 sun (100 mW cm−2), AM 1.5 G standard spectrum, and temperature of the device to be 300 K.39 The step-by-step simulation approach during the SCAPS-1D software operation is presented in Fig. 1. To run the simulation, the scaps3200.exe file is executed, and the entire problem, including all the parameters of the solar cell, is defined. The working point-temperature, frequency, and illumination spectra are specified and remains constant during the entire simulation. The calculation is initiated, and upon completion, the desired data is collected from the specified sections. The SCAPS-1D software mainly solves three pilot equations in semiconductors called the drift–diffusion equation for holes and electrons, Poisson's equation, and free carrier's continuity equations.40,41 The governing equations are shown below:

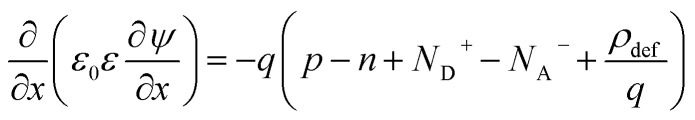2. 1
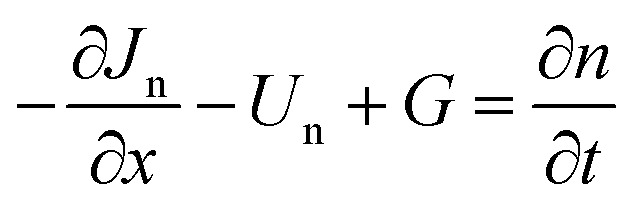2. 2
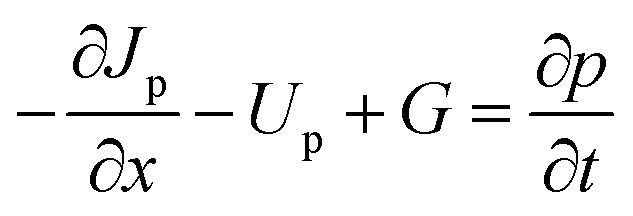2. 3
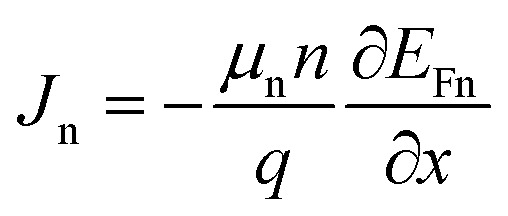2. 4
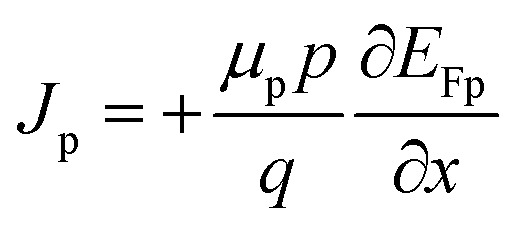2. 5

ε ascribes the dielectric constant, ψ stands for the electrostatic potential, NA and ND are the acceptor and donor density of states, respectively, q is the electrical charge, p is the density of free holes and n is the density of free electrons, Jn is the current density of electrons and Jp is the current density of holes, Pn and Pp define the electron and hole distribution, respectively, EF denotes the electric field, G is the generation rate, x is the thickness, μ is the carrier mobility, and U represents the rate of recombination.

Fig. 1. Schematic of the working approach of the SCAPS-1D simulator.23.

Fig. 1

Open in a new tab

The first three pilot equations are solved using the Newton–Raphson differentiation methods and Gummel-type iteration to calculate the quasi-Fermi levels and electrostatic potential of free carriers throughout the semiconductor. The electric field, carrier concentration, and other device potential parameters are then determined. Accurate selection of parameters for each layer of the PVSCs is crucial as the accuracy of the performance parameters depends on them. All physical parameters used in this simulation for each layer of the suggested Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al PVSCs are listed in Table 2. The data derived from the optical properties of MoTe2, ZnO, N-doped Cu2O, and ITO layers are taken from the existing literature for this simulation.17,42–45

Simulation parameters (baseline) for various constituent layers.

Parameters ITO46 ZnO35 MoTe2 23 N-doped Cu2O17
Thickness, (μm) 0.050 0.100 1.250 0.100
Band-gap, Eg (eV) 3.60 3.20 1.10 2.60
Electron affinity, χ (eV) 4.50 4.00 4.20 3.20
Dielectric constant, εr 8.90 9.00 13.0 6.60
Effective DOS at conduction band density, Nc (cm−3) 2.20 × 1018 4.00 × 1018 4.00 × 1016 2.50 × 1020
Effective DOS at valence band density, Nv (cm−3) 1.80 × 1019 2.00 × 1019 3.00 × 1018 2.50 × 1020
Electron thermal velocity, (cm s−1) 1.00 × 107 1.00 × 107 1.00 × 107 1.00 × 107
Hole thermal velocity, (cm s−1) 1.00 × 107 1.00 × 107 1.00 × 107 1.00 × 107
Electron carrier mobility, μn (cm2 V−1 S−1) 50.00 100.0 110.0 0.10
Hole carrier mobility, μp (cm2 V−1 S−1) 10.00 25.00 426.0 10.00
Donor concentration, ND (cm−3) 1.00 × 1021 1.00 × 1016 0.00 0.00
Acceptor concentration, NA (cm−3) 1.00 × 107 0.00 1.00 × 1015 1.00 × 1019
Defect density, Nt (cm−3) 1.00 × 1014 1.00 × 1014 1.00 × 1013 1.00 × 1015
Open in a new tab
Parameters MoTe2/ZnO N-doped Cu2O/MoTe2
Types of defect Neutral Neutral
Capture cross section of electrons (cm2) 1.00 × 1019 1.00 × 1019
Capture cross section of holes (cm2) 1.00 × 1019 1.00 × 1019
Energetic distribution 0.60 0.60
Open in a new tab

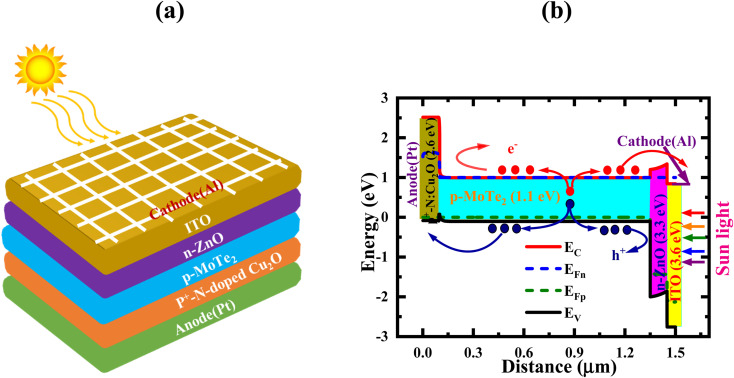
The device structure, as illustrated in Fig. 2(a), includes zinc oxide (ZnO) as a window layer, N-doped copper oxide (N-doped Cu2O) as a BSF layer, an ITO layer, and aluminum (Al) and platinum (Pt) as the front and back metal electrodes, respectively. The energy band structure of the proposed Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al PVSCs is portrayed in Fig. 2(b).

Fig. 2. Design of the Pt/N-doped Cu2O/MoTe2/ZnO/ITO/Al PVSC (a) schematic blocks and (b) simulated energy band diagram.

Fig. 2

Open in a new tab

3. Results and discussion

3.1. Performance optimization of the MoTe2-based solar cell

3.1.1. Impact of MoTe2 layer on the performance of MoTe2/ZnO PVSC

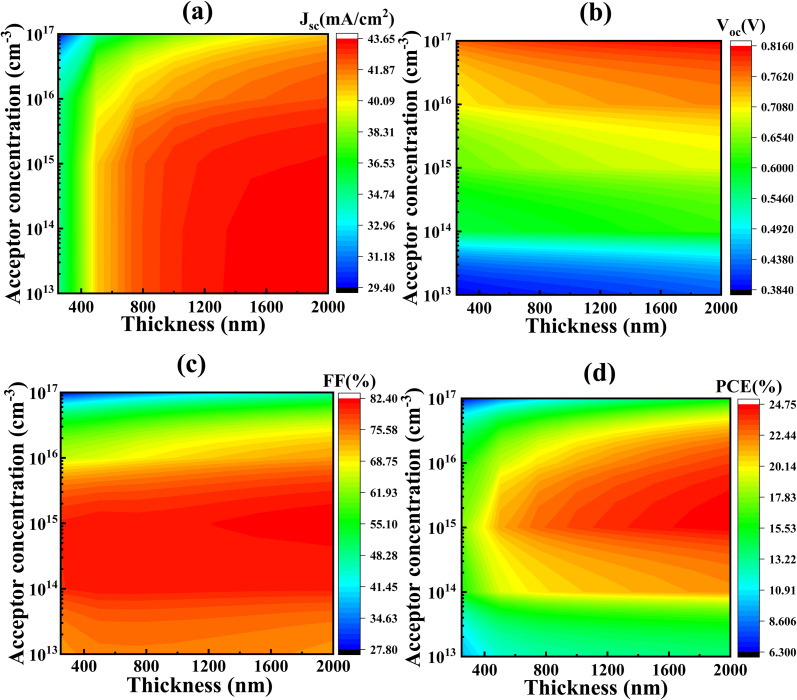
In Fig. 3, the contour plots illustrate the prime operation parameters of the MoTe2/ZnO-based PVSC in relation to the carrier concentration and thickness of the MoTe2 layer. The range for the thickness is from 100 to 2000 nm, and that for the carrier concentration is from 1013 to 1017 cm−3 of the MoTe2 layer. Fig. 3(a) presents that the short-circuit current density (JSC) rises exponentially with increasing thickness up to 1250 nm, beyond which it plateaus. This suggests that enhancing the thickness of the active layer may lead to increased photon absorption, which in turn could generate more electron–hole pairs, and consequently, a higher photocurrent.46 However, beyond a certain thickness, additional photon absorption does not significantly contribute to JSC, as many of the generated carriers may recombine before reaching the electrodes due to increased carrier transit time.47 It is also observed from Fig. 3(a) that for a MoTe2 absorber layer width of 1500 nm, the maximum value of JSC is 43.5 mA cm−2, which remains relatively steady up to a density of 1015 cm−3. Beyond this point, the value of JSC decreases systematically with a further increase in the carrier density of the MoTe2 layer. This is because the recombination losses originating from the coulomb interactions among photo-generated carriers, traps, and defects lead to increased carrier traps and recombination within the absorber layer at elevated carrier doping levels.48 Conversely, Fig. 3(b) and (c) demonstrate that the VOC and fill factor (FF) show nearly independent characteristics relative to the width of the MoTe2 layer. The VOC is related to JSC and reverse saturation current (J0) by,

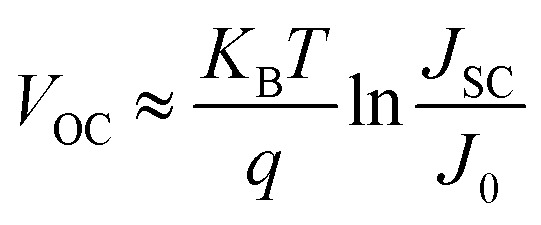3.1.1. 6

KB denotes the Boltzmann's constant, q stands for electric charge, and T denotes the absolute temperature.49Eqn (6) indicates that the VOC depends not only on the JSC but also on the J0. As the thickness of the MoTe2 layer increases, both JSC and J0 increase proportionally, which may balance the JSC/J0 ratio, leading to an almost constant VOC value.

Fig. 3. Contour representations of the variation of (a) JSC, (b) VOC, (c) FF, and (d) PCE depending on the width and doping concentration of the MoTe2 absorber layer. The low to high values of each parameter are indicated by the blue-to-red color coding.

Fig. 3

Open in a new tab

In Fig. 3(b), it is shown that increasing the doping concentration in a 1500 nm thick MoTe2 layer from 1013 cm−3 to 1017 cm−3 results in the VOC rising from 0.4 V to 0.794 V. This rise could be attributed to the shift in the quasi-Fermi energy level of holes as the acceptor concentration (NA) increases, or due to the heightened potential at the ZnO/MoTe2 junction, which is a consequence of a more robust depletion layer at higher doping densities, thereby improving charge separation and cell efficiency.17

In Fig. 3(c), the FF is observed to increase with the MoTe2 layer thickness at 1500 nm and the acceptor level rising from 1013 cm−3 to 1015 cm−3, climbing from 68.39% to 82.33%. This enhancement is linked to the reduced series resistance of the MoTe2 layer as the doping density increases.50 Nevertheless, a further increment in doping concentration from 1015 cm−3 to 1017 cm−3 causes a sharp decline in the FF value from 82.33% to 41.62%, indicating that too high a doping concentration may destabilize the system, leading to a rise in resistance and a fall in FF.

The influence of VOC, JSC, and FF on the PCE of the ZnO/MoTe2 device is depicted in the contour diagram of Fig. 3(d). According to Fig. 3(d), at a doping concentration of 1015 cm−3, there is an exponential increase in performance up to a thickness of 1500 nm, after which it plateaus. The observed result stems from the enhancement of photocurrent as the thickness increases; however, recombination may also increase in a thicker absorber layer due to the longer carrier transit time. Furthermore, Fig. 3(d) illustrates that the combined effects of VOC, JSC, and FF enhance the PCE up to a doping density of 1015 cm−3, beyond which the PCE begins to decline. The peak PCE of 24.75% was achieved with a MoTe2 layer thickness and doping concentration of 1500 nm and 1015 cm−3, respectively.

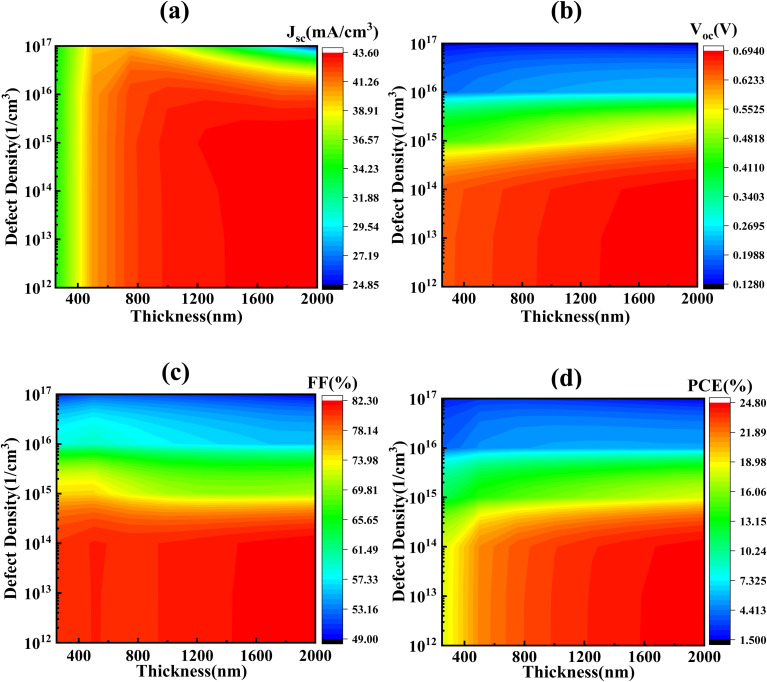
The defect density within the MoTe2 layer is a critical parameter that significantly impacts the device's performance. Fig. 4 presents contour plots of essential PV parameters against the thickness and defect density of the MoTe2 layer. As shown in Fig. 4(a), the JSC increases with thickness until a certain defect density of 1016 cm−3 is reached, beyond which it significantly decreases. Fig. 4(b)–(d) demonstrate that VOC and FF maintain PCE stability with thickness and up to a defect density of 1014 cm−3. However, larger defects cause a systematic decline in PCE across all thicknesses. Notably, at a MoTe2 layer thickness of 1500 nm, the VOC drops from 0.69 V to 0.52 V, and the FF decreases from 81.90% to 50.11% as the defect density increases from 1014 cm−3 to 1017 cm−3. This performance degradation results in a substantial efficiency drop, from 24.44% to 2.33%, as the defect density increases from 1014 cm−3 to 1017 cm−3, owing to Shockley–Read–Hall (SRH) recombination and the resulting rise in reverse saturation current.51,52 Thus, a thickness of 1250 nm, a carrier concentration of 1015 cm−3, and a defect density of 1014 cm−3 are identified as the optimal parameters for the MoTe2 absorber layer for the proposed device structure.

Fig. 4. Contour representations of the variation of (a) JSC, (b) VOC, (c) FF, and (d) PCE of the MoTe2/ZnO device as a function of thickness and defects of the MoTe2 layer. The low to high values of each parameter are indicated by the blue-to-red color coding.

Fig. 4

Open in a new tab

3.1.2. Influence of the ETL layer on the potential of the MoTe2/ZnO-based PVSC

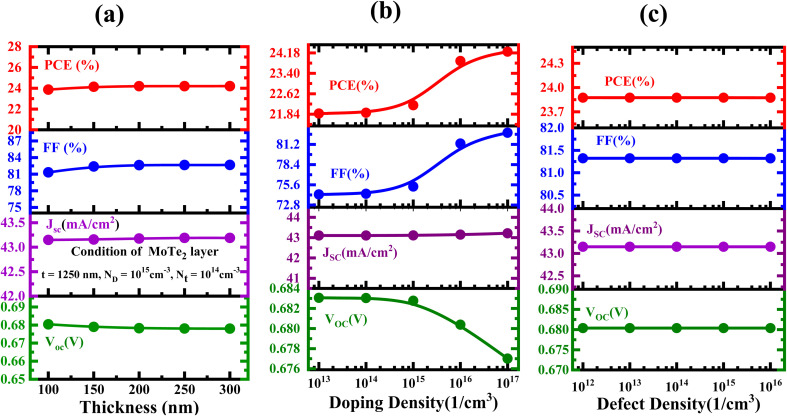
In Fig. 5, the impact of the carrier concentration, thickness, and bulk defect of the ZnO window layer on the performance of the MoTe2/ZnO-based PVSC is illustrated. Fig. 5(a) shows the deviation of the prime performance of the MoTe2/ZnO device with the thickness of the ZnO ETL layer while maintaining the optimized parameters of the absorber layer from the previous section. The performance parameters, specifically VOC, JSC, FF, and PCE, remain constant at 0.68 V, 43.2 mA cm−2, 82%, and 24%, respectively, across the ZnO layer thickness from 100 nm to 300 nm. The low visible light absorption and wide bandgap of the ZnO electron transport layer are responsible for minimally affecting cell performance.

Fig. 5. Variation of MoTe2/ZnO-based PVSC performance on the (a) thickness, (b) donor concentration, and (c) bulk defect density of the ZnO window layer.

Fig. 5

Open in a new tab

Fig. 5(b) demonstrates the change in VOC, JSC, FF, and PCE based on the doping concentration of the ZnO window layer, ranging from 1013 to 1017 cm−3. It is evident from Fig. 5(b) that the donor density does not affect JSC, while a slight decrement in VOC from 0.683 to 0.677 V is observed as the doping density increases. This originates from the coulomb traps due to high donor concentrations, which decrease electron mobility.53 Moreover, the FF significantly increases from 73 to 84% with the enhanced conductivity of the ZnO window layer due to the increase in doping density.54 The combined effect of VOC, JSC, and FF leads to an increase in PCE up to a particular doping density of 1016 cm−3, which then tends to saturate.

The impact of a rise in defects of the ZnO layer ranging from 1012 to 1016 cm−3 is illustrated in Fig. 5(c). As shown in Fig. 5(c), the performance parameters remain relatively unchanged across this range of defect densities in the ZnO layer. This suggests that the performance of the MoTe2/ZnO-based PVSC is not significantly affected by bulk defects in the ZnO layer. Consequently, a ZnO layer with a thickness of 100 nm, carrier concentration of 1016 cm−3, and bulk defect density of 1014 cm−3 is deemed optimal. Conversely, for the MoTe2 layer, a defect density of 1013 cm−3, doping concentration of 1015 cm−3, and thickness of 1250 nm are identified as the optimal performance conditions for further use.

3.2. MoTe2/ZnO-based PVSC performance dependent on the N-doped Cu2O as a back surface field (BSF) layer

Recombination at the device's rear side significantly hampers the PV performance of solar cells.17 The Back Surface Field (BSF) layer plays a vital role in solar cell design by enhancing the VOC and overall device performance. This section explores the significance of the BSF layer, detailing its function and impact on solar cell characteristics. The BSF layer's primary role is to minimize electron–hole pair recombination at the back contact, a process that leads to energy loss and reduced efficiency. Typically, the BSF layer is formed by doping the semiconductor material with a specific dopant. In p-type semiconductors like MoTe2, a heavily doped layer acts as the BSF, creating an electric field at the interface that repels minority carriers, leading to a higher VOC.

A BSF with a high carrier density (p+) is implemented at the back side of the device to reduce surface recombination and enhance performance. N-doped Cu2O is employed as the BSF layer to capitalize on its potential. The energy band structure of the Al/ITO/ZnO/MoTe2/N-doped Cu2O/Pt PVSC under sunlight illumination is depicted in Fig. 2, and the properties of the N-doped Cu2O layer are detailed in Table 2. A minimal conduction band offset (CBO) of approximately 0.06 eV is observed at the conduction band edge between the MoTe2 and ZnO layers, while a significant CBO of about 1.5 eV is present between the absorber and BSF layers. Consequently, electrons generated by sunlight in the conduction band are reflected by the large CBO at the N-doped Cu2O band edge, facilitating smooth transport across the ZnO/MoTe2 interface due to the small CBO, leading to efficient collection of photo-electrons at the front electrode. Conversely, holes generated in the valence band of the absorber layer are impeded by the ZnO layer and reflected back due to a valence band offset (VBO) of approximately 2.04 eV between ZnO/MoTe2, allowing for their efficient extraction by the N-doped Cu2O layer and transport to the back-electrode, as the VBO between the MoTe2 and N-doped Cu2O layer is insignificantly low.

3.2.1. Dependency of N-doped Cu2O BSF layer on the potential of MoTe2/ZnO-based PVSC

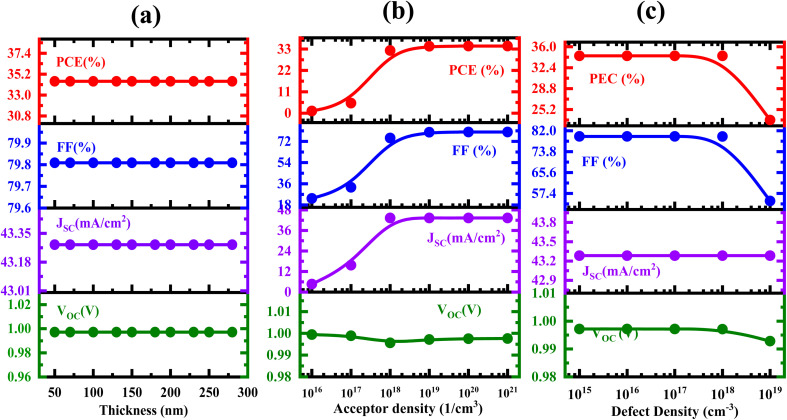
The effect of the thickness, doping density, and defect density of the N-doped Cu2O BSF layer on the performance of the MoTe2/ZnO-based PVSC is depicted in Fig. 6. Fig. 6(a) displays the variation of key performance of MoTe2/ZnO PVSC in accordance with the thickness of the N-doped Cu2O BSF layer while considering the optimized parameters of the MoTe2-absorber layer and ZnO-window layer from the previous sections. It is observed in Fig. 6(a) that the VOC, JSC, FF, and PCE remain constant at 0.99 V, 43.2 mA cm−2, 79.8%, and 34.45%, respectively, over the N-doped Cu2O BSF layer thickness from 50 nm to 280 nm. This result suggests that no photons from the sunlight were absorbed in the N-doped Cu2O BSF layer.

Fig. 6. Dependency of MoTe2/ZnO-based PVSC performance on the (a) thickness, (b) donor concentration, and (c) bulk defect density of the N-doped Cu2O BSF layer.

Fig. 6

Open in a new tab

Fig. 6(b) illustrates the change in key performance parameters of the MoTe2/ZnO PVSC with the carrier density of the N-doped Cu2O BSF layer ranging from 1016 cm−3 to 1021 cm−3. Except for VOC, all the key parameters gradually increased with an increase in the carrier density from 1016 cm−3 to 1018 cm−3, above which the values remained constant in the current density range of 1018 cm−3 to 1021 cm−3. These results suggest that the sufficient electric field due to the BSF layer at the N-doped Cu2O/MoTe2 interface might be attained after a certain carrier concentration of 1018 cm−3.

The effect of increasing the defect density of the N-doped Cu2O BSF layer on the key performance parameters is shown in Fig. 6(c). As shown in Fig. 6(c), the performance parameters remain relatively unchanged up to the defect density of 1018 cm−3 in the N-doped Cu2O BSF layer. For a large amount of defect density of 1019 cm−3, the VOC and FF, resulting in PCE, sharply declined from 34.45% to 23.87% due to Shockley–Read–Hall (SRH) recombination. Consequently, an N-doped Cu2O BSF layer with a thickness of 100 nm, carrier concentration of 1019 cm−3, and bulk defect density of 1015 cm−3 is considered optimal.

3.2.2. Impact of the BSF layer on the built-in potential of the device

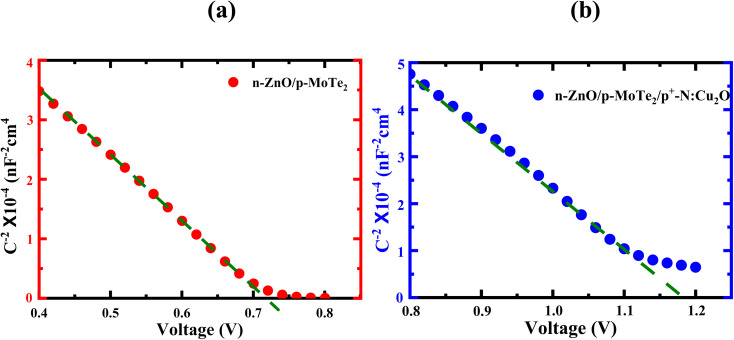
In Fig. 7, the capacitance–voltage (C–V) characteristics are presented using Mott–Schottky analysis to find the built-in potential (φbi) for two device configurations: one without a BSF layer (ZnO/MoTe2) and the other with a BSF layer (N-doped Cu2O/MoTe2/ZnO). The built-in potential (φbi) can be estimated from the intercept of the (1/C)2–V plot.55–59

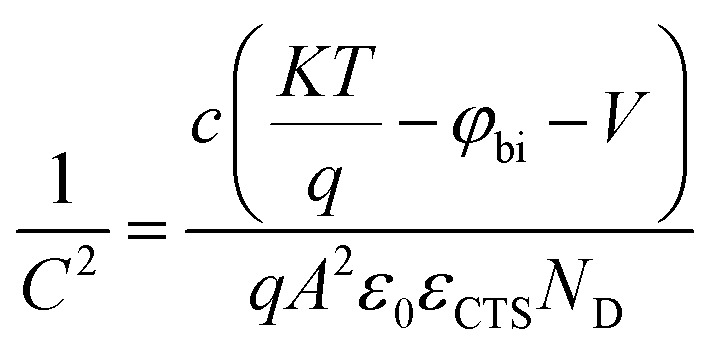3.2.2. 7

εCTS and ε0 represent the permittivity of the medium and vacuum, respectively, C is the resultant capacitance at the junctions, V defines the applied voltage, ND demonstrates the donor density, q = 1.6 × 1019 eV, and A describes the area of the diode.

Fig. 7. C–V analysis to evaluate the built-in potential of (a) ZnO/MoTe2 and (b) ZnO/MoTe2/N-doped Cu2O heterostructures.

Fig. 7

Open in a new tab

The linear section in Fig. 7(a), after fitting and extrapolation, reveals that the built-in potential (φbi) for the ZnO/MoTe2 device is approximately 0.72 V. However, the built-in potential for the ZnO/MoTe2/N-doped Cu2O device is determined to be 1.18 V. It is suggested that the cumulative effect of the ZnO/MoTe2 junction and MoTe2/N-doped Cu2O interface is responsible for improving the device's built-in potential, thereby aiding in the efficient separation of photo-induced carriers. The enhanced electric field created by the BSF layer promotes the drift of minority carriers towards the front contact, where they can contribute to the photocurrent. This results in a slightly improved JSC in the device. Additionally, due to the higher CB edge of the N-doped Cu2O compared to the MoTe2 edge, electrons generated in the absorber layer are repelled at the BSF layer's CB edge. This repulsion mitigates surface recombination at the rare interface, leading to a significantly higher VOC of the ZnO/MoTe2/N-doped Cu2O device. In other words, introducing a properly designed Back Surface Field (BSF) layer on the backside of the device generates an additional electric field at the BSF/Absorber (p+/p) interface.60 This electric field causes the reflection of minority carriers (electrons) approaching the BSF/Absorber (p+/p) interface. As a result, the recombination velocity, which refers to the number of carrier recombination per unit area and time, will decrease due to the presence of the electric field produced by the BSF layer. This reduction in recombination leads to an improvement in the open-circuit voltage (VOC) of the device.

3.2.3. Impact of N-doped Cu2O BSF layer on the Recombination rate at different regions of the device

Shockley–Read–Hall (SRH) recombination, known as defect-assisted recombination, is a key process in solar cells and can significantly reduce cell performance. Statistical analysis and analytical approximations of SRH recombination are crucial for understanding and quantifying recombination rates (R) in different regions of the solar cell, such as in the bulk region (Rb), depletion region (Rd), at the ETL/absorber interface (Ri,f), and the BSF layer/absorber interface (Ri,b).61 Our previous article details the methodology for estimating these recombination rates at different regions of the device.62 The VOC is related to the intensity of the light as follows:

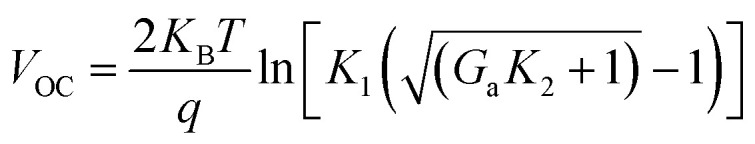3.2.3. 8

Here, KB represents the Boltzmann constant, q is the electron charge, Ga is the incident light intensity, and T is the absolute temperature. Coefficients K1 and K2 are derived from fitting the intensity-dependent VOC curves using eqn (8).

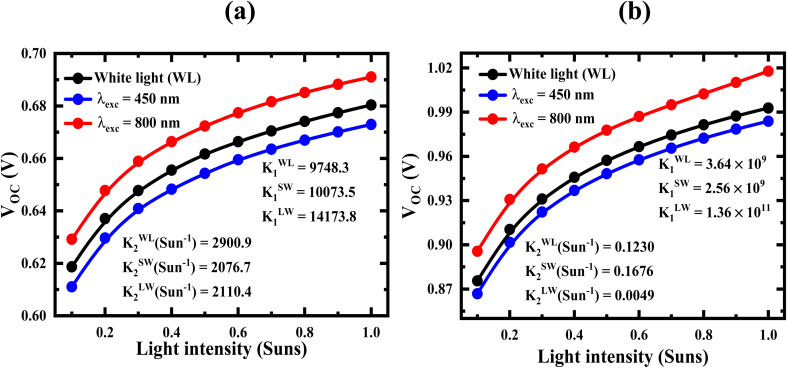
An intensity-dependent VOC analysis under monochromatic short and long wavelengths has been conducted to extract the recombination coefficient at the absorber/BSF interface (Ri,b0) and the surface recombination velocity (SRV) of electrons at the absorber/BSF interface. The study uses two monochromatic wavelengths, 450 nm and 800 nm, to illuminate the designed SCs for qualitative and quantitative assessments of recombination at the back interface without and with the BSF layer. Fig. 8 shows the VOC graphs dependent on light intensity (Ga) without and with the BSF layer. The K1 and K2 values, obtained from fitting the intensity-dependent VOC curves in Fig. 8 using eqn (8), are used to calculate the recombination coefficients Rd0, Ri,b0, and hence the recombination rates Rd, Ri,b as well as the surface recombination velocity of electrons at the back interface, which are presented in Table 3. Details of calculations of the recombination coefficient and surface recombination velocity are discussed in ES1.†

Fig. 8. Light intensity-dependent VOC for (a) ZnO/MoTe2 (b) ZnO/MoTe2/N-doped Cu2O device with different excitation wavelengths of white light (WL), λexc = 450 nm, and λexc = 800 nm at a constant temperature of 300 K.

Fig. 8

Open in a new tab
Table 3. Extracted values of K1 and K2 from Fig. 8 and recombination coefficient for MoTe2-based PVSCs with and without N-doped Cu2O BSF layer. Here, Rd0, Ri,b0, Rd, Ri,b, and Sbe are calculated at a bias voltage of 1.0 V.
Condition K SW K LW K WL K SW K LW K WL R d (cm−2 s−1) R i,b (cm−2 s−1) R d (cm−2 s−1) R i,b (cm−2 s−1) S b (cm s−1)
Without N-doped Cu2O-BSF layer 10 073 14 173.8 9748.3 2076.7 2110.4 2901 1.1 × 108 1.6 × 103 2.77 × 1016 3.98 × 1011 200
with N-doped Cu2O-BSF layer 2.56 × 109 1.36 × 1011 3.64 × 109 0.1676 0.0049 0.123 7.1 × 106 1.3 × 10−3 1.75 × 1015 3.35 × 105 1.69 × 10−3
Open in a new tab

Table 3 reveals that the recombination coefficient and rate in the depletion region and at the back interface, along with the SRV at the back interface for ZnO/MoTe2, are notably poor. However, by integrating an N-doped Cu2O layer (100 nm) with ZnO/MoTe2, forming a ZnO/MoTe2/N-doped Cu2O structure, a significant reduction in the recombination coefficient in the depletion region and at the back interface, from 1.1 × 108 cm−2 s−1 to 7.1 × 106 cm−2 s−1 and 1.6 × 103 cm−2 s−1 to 1.3 × 10−3, respectively, is observed. Consequently, the recombination rate in the depletion region and at the back interface is suppressed from 2.77 × 1016 cm−2 s−1 to 1.75 × 1015 and 3.98 × 1011 cm−2 s−1 to 3.35 × 105, respectively. Additionally, the SRV at the back interface is decreased from 200 cm s−1 to 1.69 × 10−3 cm s−1 by adding the N-doped Cu2O BSF layer. These findings imply that the electric field at the MoTe2/N-doped Cu2O interface enhances hole transport at the valence band from the MoTe2 absorber layer to the back contact via the N-doped Cu2O-BSF layer, mitigating the flow of minority carriers (electrons) towards the MoTe2/N-doped Cu2O junction at the conduction band, thus reducing the carrier recombination rate at the device's rear side, suppressing the dark current (J0). It is confirmed from eqn (6) that the open circuit voltage of the MoTe2-based PVSC in the presence of N-doped Cu2O is improved due to the suppression of the dark current.

3.2.4. Impact of BSF layer on the open-circuit voltage (VOC) of the device

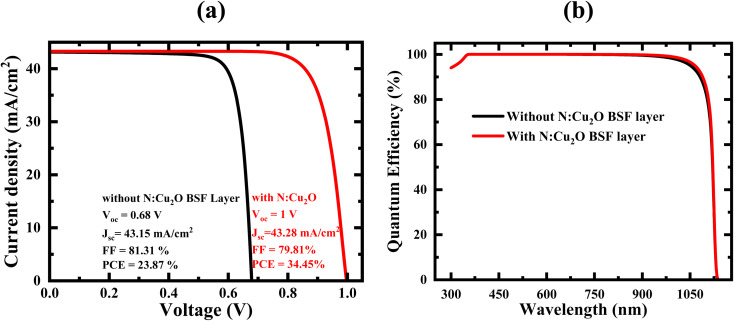
Fig. 9 compares the performance of the MoTe2/ZnO-based PVSC with and without the N-doped Cu2O BSF layer. The J-V characteristics of both ZnO/MoTe2 and ZnO/MoTe2/N-doped Cu2O PVSCs are displayed in Fig. 9(a), where the key performance parameters are also detailed. The ZnO/MoTe2 single-junction SC shows an efficiency of 23.87%, with a JSC of 43.15 mA cm−2, VOC of 0.68 V, and FF of 81.31%. Incorporating a 100 nm thick N-doped Cu2O as the BSF layer into the ZnO/MoTe2 SC significantly enhances the efficiency to 34.45%, with a JSC of 43.28 mA cm−2, VOC of 1.0 V, and an FF of 79.81%. The notable increase in efficiency is primarily due to the raised VOC (from 0.68 V to 1.0 V), which is a result of a stronger built-in potential (from 0.72 eV to 1.18 eV) in the ZnO/MoTe2/N-doped Cu2O heterostructure, thus reducing recombination losses.

Fig. 9. (a) Light-dependent output characteristic and (b) quantum efficiency curves for the proposed MoTe2/ZnO PVSC in the presence and absence of an N-doped Cu2O-BSF layer.

Fig. 9

Open in a new tab

Fig. 9(b) compares the quantum efficiency (QE) curves for both structures in the presence and absence of the N-doped Cu2O BSF layer. Simulations spanning a wavelength range of 300 nm to 1200 nm under AM1.5 solar illumination indicate similar QE curves for both cases. It is evident that the MoTe2/ZnO PVSC, in the presence and absence of the N-doped Cu2O BSF layer, exhibits nearly identical QE curves, indicating the N-doped Cu2O BSF layer does not contribute to photon absorption. However, it aids in enhancing the built-in potential by the additional N-doped Cu2O/MoTe2 junction, reducing recombination losses at the back side of the device to increase the VOC, leading to improved photovoltaic efficiency (Fig. 8).

3.3. Effect of metal work function at the back contact and operation temperature on the N-doped Cu2O/MoTe2/ZnO PVSC

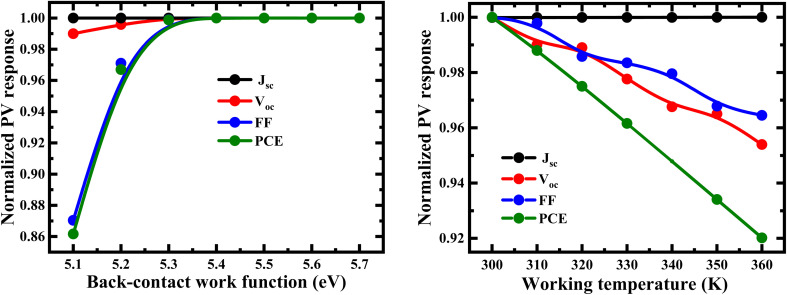
In Fig. 10(a), the graph shows the normalized PV response for the back-contact metal work function ranging from 5.1 eV to 5.7 eV. This is to understand the behavior at the interface of the back-contact/BSF layer, whether it is ohmic or rectifying. The carrier concentration, thickness, and bulk defect of each layer have been kept at the optimized values obtained previously from the simulation. From Fig. 10(a), it can be observed that all the PV parameters except for JSC linearly increase with the increasing anode's work function up to a certain value of 5.32 eV. Beyond this value, further improvement in the metal work function of the back electrode shows diminishing returns. It is also noted in Fig. 10(a) that a Schottky-type rectifying behavior is observed at the N-doped Cu2O/anode interface for work functions less than 5.32 eV. This leads to the recombination of carriers at the back-contact/BSF interface, resulting in reduced VOC, FF, and consequently, photovoltaic efficiency, as shown in Fig. 10(a). It is well known that the energy barrier (φB) between the back surface anode metal and N-doped Cu2O-BSF layer can be evaluated by:

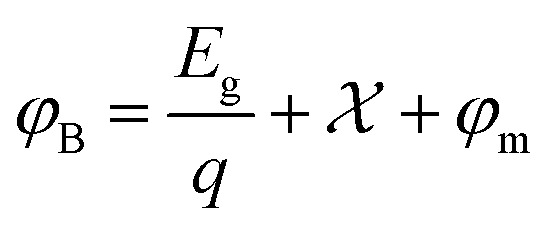3.3. 9

Here, χ defines the electron affinity of N-doped Cu2O (3.20 eV), Eg ascribes the bandgap of N-doped Cu2O (2.60 eV), and φm denotes the work function of the anode metal. According to eqn (9), for the anode metals with work function 5.32 eV, the energy barrier is about 0.48 eV; such a small value may not affect the VOC and FF of the device.62,63 Thereby, the maximum PVSC performance might be achieved for the anode with a metal work function of more than 5.32 eV. This finding suggests that a relatively high work function anode materials (>5.32 eV), such as Pt (5.65 eV),64 Au(111) (5.37 eV),65 Ni(111) (5.35 eV)66 and Pd(111) (5.40 eV),65 are required to establish the maximum efficiency of the N-doped Cu2O/MoTe2/ZnO PVSC.

Fig. 10. Key performance parameter responses of the N-doped Cu2O/MoTe2/ZnO PVSC in relation to (a) work function of the back-contact metal and (b) operating temperature.

Fig. 10

Open in a new tab

Conventionally, solar cells are typically designed to operate within a temperature range of 288 to 323 K when placed in the open air.67 The operating temperature is a critical factor as it greatly affects the PV response of the cells.68Fig. 10(b) displays the effect of operating temperature on the optimized N-doped Cu2O/MoTe2/ZnO PVSC. It is noticed from Fig. 10(b) that a linear decrement of the VOC and FF leads to a decrease in PCE as the working temperature increases. This observation suggests that at higher temperatures, electrons acquire sufficient energy to recombine with holes before leaving the depletion zone.69 The temperature coefficient CT (% K−1) of PCE is a key parameter for PV devices to assess their stability, indicating how elevated operating temperatures impact the device's PCE, and can be expressed under standard test conditions (STC: 298 K) as follows:60

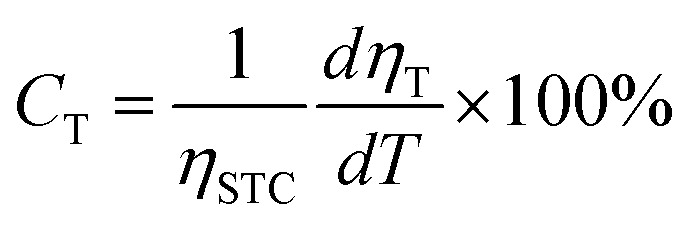3.3. 10

ηSTC ascribes the cell efficiency at standard test conditions (STC: 298 K) and 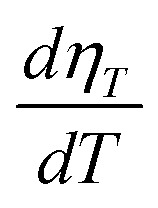Inline graphic is extracted from the slope by fitting the PCE curve in Fig. 10(b). Now, using eqn (10), the temperature coefficient of PCE of the cell is evaluated to be −0.133% K−1, which is less than that of traditional Si (−0.5% K−1) and CIGS (−0.443% K−1) solar cells.23,70

3.4. Bifacial nature of the ZnO/MoTe2/N-doped Cu2O solar cell

The bifacial device is a strong candidate for improving energy harvesting capabilities per unit area to replace mono-facial solar cells without increasing cost and manufacturing complexity. Two independent simulations for back and front side illuminations of 1 sun are considered. The term bifacial factor (BF) is carried out to understand the asymmetric photoelectric behavior of bifacial devices. The relative feedback of each side can be realized by the BF, which is estimated by the following equation.

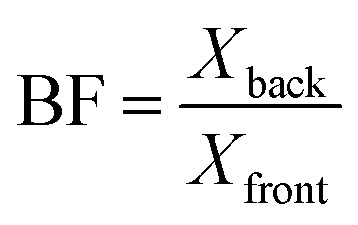3.4. 11

X defines any PV parameter (VOC, JSC, FF or PCE) of the device under the back and front illumination at 1 sun.

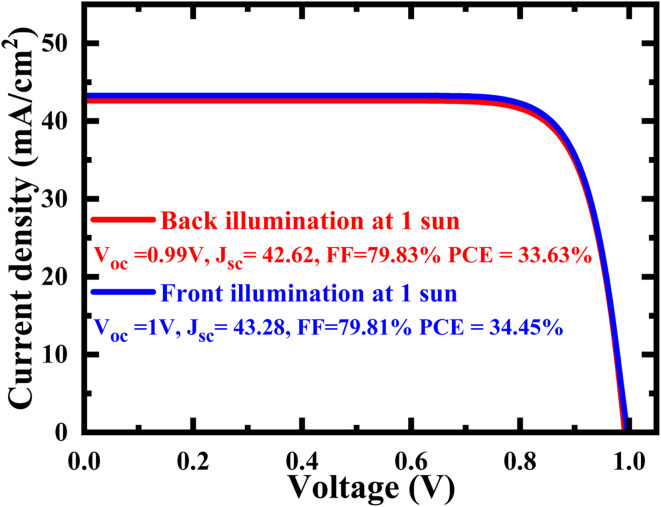
Fig. 11 shows the J–V curves of an optimized N-doped Cu2O/MoTe2/ZnO solar cell under both front and back illumination at 1 sun. The PV performance slightly declines with back illumination at 1 sun, suggesting minor absorption losses at the device's rear surface. The bifacial factor (BF) for VOC and FF is calculated to be 99.49% and 99.89%, respectively, indicating negligible additional losses impacting VOC and FF under backside illumination. The BF for JSC is approximately 98.4%, reflecting a slight reduction in JSC due to absorption losses at the solar cell's back surface. Lastly, a BF for PCE of 97.6% is reported, corroborating earlier studies.17,71

Fig. 11. J–V curves of the optimized N-doped Cu2O/MoTe2/ZnO PVSC under back-illumination at 1 sun and front illumination at 1 sun for a bifacial PVSC.

Fig. 11

Open in a new tab

4. Conclusion and recommendations

Despite the inherent advantages of MoTe2, developing high-efficiency MoTe2-based solar cells faces a crucial challenge: achieving a high open-circuit voltage (VOC). The VOC is a key performance parameter that directly influences the overall efficiency of a solar cell. A high VOC is essential for maximizing the power output and achieving competitive efficiencies. This study examines the obstacles related to attaining a high VOC in MoTe2 thin film solar cells. It examines the pivotal role of the Back Surface Field (BSF) layer in enhancing VOC and introduces a novel bi-facial ZnO/MoTe2 solar cell with an N-doped Cu2O BSF layer. Our methodology relies on extensive device simulations utilizing the SCAPS-1D software to offer insights into the electrical properties and performance of the proposed device architecture. This study numerically investigated the potential of a bi-facial ZnO/MoTe2 solar cell with an N-doped Cu2O BSF layer for achieving high VOC. The incorporation of the N-doped Cu2O BSF layer effectively suppressed carrier recombination at the back contact, leading to a significant enhancement in VOC. The proposed device structure demonstrated a considerable increase in VOC compared to conventional designs, potentially enabling higher power conversion efficiency. The simulation results highlighted the importance of optimizing the thickness and doping concentration of the BSF layer to maximize its effectiveness. This research provides valuable insights into the design and optimization of MoTe2-based solar cells. Future research efforts should focus on:

Experimental validation: conducting experimental fabrication and characterization of the proposed device structure to validate the simulation results.

Optimization of BSF layer: investigating different materials and deposition techniques for the BSF layer to further enhance its performance.

Integration with other technologies: exploring the integration of the bi-facial design with other advanced technologies, such as tandem solar cells or flexible substrates.

By addressing these recommendations, the development of high-performance MoTe2 solar cells with improved JSC and power conversion efficiency can be significantly accelerated, contributing to the advancement of renewable energy technologies.

Data availability

The data that support the findings of this study are available from the corresponding authors upon reasonable request.

Conflicts of interest

The authors declare that there is no conflicts of interest in this work.

Supplementary Material

RA-014-D4RA05974C-s001

Acknowledgments

This study was supported in part by the research centre, Bangabandhu Sheikh Mujibur Rahman Science and Technology University (BSMRSTU), Bangladesh.

†

Electronic supplementary information (ESI) available: Measurement procedures for recombination coefficient and surface recombination velocity details are available upon request. See DOI: https://doi.org/10.1039/d4ra05974c

References

  1. Rizi M. T. Abadi M. H. S. Ghaneii M. Two dimensional modeling of Cu2O heterojunction solar cells based-on β-Ga2O3 buffer. Optik. 2018;155:121–132. [Google Scholar]
  2. Shi Z. Jayatissa A. H. One-pot hydrothermal synthesis and fabrication of kesterite Cu2ZnSn(S,Se)4 thin films. Prog. Nat. Sci.:Mater. Int. 2017;27:550–555. [Google Scholar]
  3. Vanalakar S. A. Patil P. S. Kim J. H. Recent advances in synthesis of Cu2FeSnS4 materials for solar cell applications: a review. Sol. Energy Mater. Sol. Cells. 2018;182:204–219. [Google Scholar]
  4. Li J. Wang D. Li X. Zeng Y. Zhang Y. Cation substitution in earth-abundant kesterite photovoltaic materials. Adv. Sci. 2018;5:1700744. doi: 10.1002/advs.201700744. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Abdelraouf O. A. M. Allam N. K. Nanostructuring for enhanced absorption and carrier collection in CZTS-based solar cells: coupled optical and electrical modeling. Opt. Mater. 2016;54:84–88. [Google Scholar]
  6. Fessenden R. W. Sobhanadri J. Subramanian V. Minority carrier lifetime in thin films of Zn,P, using microwave and optical transient measurements. Thin Solid Films. 1995;266:176–181. [Google Scholar]
  7. Sai Gautam G. Senftle T. P. Carter E. A. Understanding the effects of Cd and Ag doping in Cu2 ZnSnS4 solar cells. Chem. Mater. 2018;30:4543–4555. [Google Scholar]
  8. Branker K. Pathak M. J. M. Pearce J. M. A review of solar photovoltaic levelized cost of electricity. Renewable Sustainable Energy Rev. 2011;15:4470–4482. [Google Scholar]
  9. Ma W. Luther J. M. Zheng H. Wu Y. Alivisatos A. P. Photovoltaic Devices Employing Ternary PbSxSe1-x Nanocrystals. Nano Lett. 2009;9:1699–1703. doi: 10.1021/nl900388a. [DOI] [PubMed] [Google Scholar]
  10. Ma W. Swisher S. L. Ewers T. Engel J. Ferry V. E. Atwater H. A. Alivisatos A. P. Photovoltaic Performance of Ultrasmall PbSe Quantum Dots. ACS Nano. 2011;5:8140–8147. doi: 10.1021/nn202786g. [DOI] [PubMed] [Google Scholar]
  11. McDonald S. Konstantatos G. Zhang S. Cyr P. Klem E. Levina L. Sargent E. Solution-Processed PbS Quantum Dot Infrared Photodetectors and Photovoltaics. Nat. Mater. 2005;4:138–144. doi: 10.1038/nmat1299. [DOI] [PubMed] [Google Scholar]
  12. Ning Z. Zhitomirsky D. Adinolfi V. Sutherland B. Xu J. Voznyy O. Maraghechi P. Lan X. Hoogland S. Ren Y. Sargent E. H. Graded Doping for Enhanced Colloidal Quantum Dot Photovoltaics. Adv. Mater. 2013;25:1719–1723. doi: 10.1002/adma.201204502. [DOI] [PubMed] [Google Scholar]
  13. Zhao W. Ribeiro R. M. Toh M. Carvalho A. Kloc C. Neto A. H. C. Eda G. Origin of Indirect Optical Transitions in Few-Layer MoS2, WS2, and WSe2. Nano Lett. 2013;13:5627–5634. doi: 10.1021/nl403270k. [DOI] [PubMed] [Google Scholar]
  14. Dominguez-Meister S. Justo A. Sanchez-Lopez J. Synthesis and tribological properties of WSex films prepared by magnetron sputtering. Mater. Chem. Phys. 2013;142:186–194. [Google Scholar]
  15. Chiritescu C. Cahill D. G. Nguyen N. Johnson D. Bodapati A. Keblinski P. Zschack P. Ultralow Thermal Conductivity in Disordered, Layered WSe2 Crystals. Science. 2007;315:351–353. doi: 10.1126/science.1136494. [DOI] [PubMed] [Google Scholar]
  16. Finn S. T. Macdonald J. E. Contact and Support Considerations in the Hydrogen Evolution Reaction Activity of Petaled MoS2 Electrodes. ACS Appl. Mater. Interfaces. 2016;8:25185–25192. doi: 10.1021/acsami.6b05101. [DOI] [PubMed] [Google Scholar]
  17. Rahman M. A. Performance analysis of WSe2-based bifacial solar cells with different electron transport and hole transport materials by SCAPS-1D. Heliyon. 2022;8:e09800. doi: 10.1016/j.heliyon.2022.e09800. [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Liu J. Wang Y. Xiao X. Zhang K. Guo N. Jia Y. Zhou S. Wu Y. Li Q. Xiao L. Conversion of Multi-layered MoTe2 Transistor Between P-Type and N-Type and Their Use in Inverter. Nanoscale Res. Lett. 2018;13(291):1–9. doi: 10.1186/s11671-018-2721-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Rahman N. Haque M. D. Rahman M. F. Islam M. M. Juthi M. A. N. Roy A. R. Akter M. A. Islam M. F. Assessing the performance of MoTe2 based solar cell with Cu2O hole transport layer through device simulation. Discover Mater. 2023;3(25):1–7. [Google Scholar]
  20. Gong C. Li H. Wang H. Zhang C. Zhuang Q. Wang A. Xu Z. Cai W. Li R. Li X. Zang Z. Silver coordination-induced n-doping of PCBM for stable and efficient inverted perovskite solar cells. Nat. Commun. 2024;15:4922. doi: 10.1038/s41467-024-49395-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  21. Wang K. Xu Z. Guo Z. Wang H. Qaid S. M. H. Yang K. Zang Z. Phosphonate Diacid Molecule Induced Crystallization Manipulation and Defect Passivation for High-Performance Inverted MA-Free Perovskite Solar Cells. Adv. Energy Mater. 2024;14:2402249. [Google Scholar]
  22. Zhang C. Li H. Gong C. Zhuang Q. Chen J. Zang Z. Crystallization manipulation and holistic defect passivation toward stable and efficient inverted perovskite solar cells. Energy Environ. Sci. 2023;16:3825–3836. [Google Scholar]
  23. Rajib A. Kafi M. A. A. Najesh M. M. H. Hasan M. R. Miah M. R. Rahman A. Performance analysis of 2D-MoTe2/2D-MoSe2 -based bifacial solar cells with N-doped Cu2O as BSF Layer by SCAPS-1D. Phys. Scr. 2024;99:055541. [Google Scholar]
  24. Rajib A. Kuddus A. Yokoyama K. Shida T. Ueno K. Shirai H. Mist chemical vapor deposition of Al1-xTixOy thin films and their application to a high dielectric material. J. Appl. Phys. 2022;131:105301. [Google Scholar]
  25. Rajib A. Kuddus A. Shida T. Ueno K. Shirai H. Mist Chemical Vapor Deposition of AlOx Thin Films Monitored by a Scanning Mobility Particle Analyzer and its Application to the Gate Insulating Layer of Field-effect Transistors. ACS Appl. Electron. Mater. 2021;3(2):658–667. [Google Scholar]
  26. Kuddus A. Rajib A. Yokoyama K. Shida T. Ueno K. Shirai H. Mist Chemical Vapor Deposition of Crystalline MoS2 Atomic Layer Films for Field-Effect Transistors. Nanotechnology. 2021;33(4):045601. doi: 10.1088/1361-6528/ac30f4. [DOI] [PubMed] [Google Scholar]
  27. Sultana B. Islam A. T. M. Haque Md. Kuddus A. Ali Md. Rahman F. Numerical study of MoSe2 -based dual-heterojunction with In2Te3 BSF layer toward high-efficiency photovoltaics. Phys. Scr. 2023;98:095935. [Google Scholar]
  28. Puotinen D. Newnham R. E. The crystal structure of MoTe2. Acta Crystallogr. 1961;14:691–692. [Google Scholar]
  29. Reeber R. R. Lattice parameters of ZnO from 4.20 to 296 0K. J. Appl. Phys. 1970;41:5063–5066. [Google Scholar]
  30. Wang Y. Ghanbaja J. Horwat D. Yu L. Pierson J. F. Nitrogen chemical state in N-doped Cu2O thin films. Appl. Phys. Lett. 2017;110:131902. [Google Scholar]
  31. Alam I. Mollick R. Ashraf Md. A. Numerical simulation of Cs2AgBiBr6-based perovskite solar cell with ZnO nanorod and P3HT as the charge transport layers. Phys. B. 2021;618:413187. [Google Scholar]
  32. Askari S. S. A. Kumar M. Das M. K. Numerical study on the interface properties of a ZnO/c-Si heterojunction solar cell. Semicond. Sci. Technol. 2018;33:115003. [Google Scholar]
  33. Dinh Lam N. Modelling and numerical analysis of ZnO/CuO/Cu2O heterojunction solar cell using SCAPS. Eng. Res. Express. 2020;2:025033. [Google Scholar]
  34. Manoua M. Jannane T. El-Hami K. Ahmed L. Investigation of n-ZnO/p-GaAs Heterojunction Solar Cell Using Two-Dimensional Numerical Simulation. Recent Adv. Photonics Optoelectron. 2023;75:3601–3611. [Google Scholar]
  35. Mozafari B. Shahhoseini A. Using Molybdenum Trioxide as a TCO Layer to Improve Performance of CdTe/CdS1 Thin-film Solar Cell. Signal Process. Renew. Energy. 2020;4(3):57–65. [Google Scholar]
  36. Parajuli D. Devendra K. C. Khattri K. B. Adhikari D. R. Gaib R. A. Shah D. K. Numerical assessment of optoelectrical properties of ZnSe–CdSe solar cell-based with ZnO antireflection coating layer. Sci. Rep. 2023;13:12193. doi: 10.1038/s41598-023-38906-z. [DOI] [PMC free article] [PubMed] [Google Scholar]
  37. Wahmane Y. A. Mouhib H. Ydir B. Hssi A. A. Atourki L. Ihlal A. Bouabid K. Comparison study between ZnO and TiO2 in CuO based solar cell using SCAPS-1D. Mater. Today Proc. 2022;52:166–171. [Google Scholar]
  38. Gholipoor M. Solhtalab N. Mohammadi M. H. High-performance parallel tandem MoTe2/perovskite solar cell based on reduced graphene oxide as hole transport layer. Sci. Rep. 2022;12:20455. doi: 10.1038/s41598-022-25015-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
  39. Kriegel I. Rodriguez-Fernandez J. Da Como E. Lutich A. A. Szeifert J. M. Feldmann J. Tuning the Light Absorption of Cu1.97S Nanocrystals in Supercrystal Structures. Chem. Mater. 2011;23:1830–1834. [Google Scholar]
  40. Burgelman M. Verschraegen J. Degrave S. Nollet P. Modeling thin-film PV devices. Prog. Photovoltaics. 2004;12(2–3):143–153. [Google Scholar]
  41. Burgelman M. Nollet P. Degrave S. Modelling polycrystalline semiconductor solar cells. Thin Solid Films. 2000;361:527–532. [Google Scholar]
  42. Liu F. Zhu J. Wei J. Li Y. Lv M. Yang S. Zhang B. Yao J. Dai S. Numerical simulation: Toward the design of high-efficiency planar perovskite solar cells. Appl. Phys. Lett. 2014;104:253508. [Google Scholar]
  43. Wu K. Ma H. Gao Y. Yang J. Highly-efficient heterojunction solar cells based on two-dimensional tellurene and transition metal dichalcogenides. J. Mater. Chem. A. 2019;7:7430–7436. [Google Scholar]
  44. Mondal B. K. Mostaque S. K. Hossain J. Unraveling the effects of a GeSe BSF layer on the performance of a CuInSe2 TFSCs: a computational analysis. Opt. Continuum. 2023;2:428–440. [Google Scholar]
  45. Bedia A. Bedia F. Z. Aillerie M. Benyoucef B. Morphological and Optical Properties of ZnO Thin Films Prepared by Spray Pyrolysis on Glass Substrates at Various Temperatures for Integration in Solar Cell. Energy Procedia. 2015;74:529–538. [Google Scholar]
  46. Hossain J. Rahman M. Moon M. M. A. Mondal B. K. Rahman M. F. Rubel M. H. K. Guidelines for a highly efficient CuI/n-Si heterojunction solar cell. Eng. Res. Express. 2020;2:045019. [Google Scholar]
  47. Sze S. M. Ng K. K. LEDs and lasers. Phys. Semicond. Devices. 2006;3:601–657. [Google Scholar]
  48. Wanda M. D. Ouédraogo S. Tchoff F. Zougmoré F. Ndjaka J. M. B. Numerical Investigations and Analysis of Cu2ZnSnS4 Based Solar Cells by SCAPS-1D. Int. J. Photoenergy. 2016:1–9. [Google Scholar]
  49. Patel B. H. Patel S. S. Growth and electrical properties of flash evaporated AgGaTe2 thin films. Cryst. Res. Technol. 2006;41(2):117–122. [Google Scholar]
  50. Rana A. Kumar A. Rahman M. W. Vashistha N. Garg K. K. Pandey S. Singh R. K. Non-approximated series resistance evaluation by considering high ideality factor in organic solar cell. AIP Adv. 2018;8:125121. [Google Scholar]
  51. Mott N. F. Metal-insulator transition. Rev. Mod. Phys. 1968;40(4):677. [Google Scholar]
  52. Rai S. Pandey B. K. Dwivedi D. K. Modeling of highly efficient and low cost CH3NH3Pb (I1-xClx)3 based perovskite solar cell by numerical simulation. Opt. Mater. 2020;100:109631. [Google Scholar]
  53. Ghosh A. Dipta S. S. Nikor S. S. S. Saqib N. Saha A. Performance analysis of an efficient and stable perovskite solar cell and a comparative study of incorporating metal oxide transport layers. J. Opt. Soc. Am. B. 2020;37(7):1966. [Google Scholar]
  54. Ahmmed S. Aktar A. Rahman M. F. Hossain J. Ismail A. B. M. A numerical simulation of high efficiency CdS/CdTe based solar cell using NiO HTL and ZnO TCO. Optik. 2020;223:165625. [Google Scholar]
  55. Sze S. and Kwok N., Physics of Semiconductor Devices, Wiley-Interscience, 3rd edn, 2007 [Google Scholar]
  56. Sze S. M. Crowell C. R. Kahng D. Current transport in metal-semiconductor barriers. J. Appl. Phys. 1964;35:2534. [Google Scholar]
  57. Islam A. T. M. Karim M. E. Rajib A. Nasuno Y. Ukai T. Kurosu S. Tokuda M. Fujii Y. Nakajima Y. Hanajiri T. Shirai H. Chemical mist deposition of organic for efficient front- and back-PEDOT:PSS/crystalline Si heterojunction solar cells. Appl. Phys. Lett. 2019;114:193901. [Google Scholar]
  58. Rajib A. Enamul K. M. Kurosu S. Ukai T. Tokuda M. Fujii Y. Hanajiri T. Ishikawa R. Ueno K. Shirai H. Synthesis of AlOx thin films by atmospheric-pressure mist chemical vapor deposition for surface passivation and electrical insulator layers. J. Vac. Sci. Technol., A. 2020;38:033413. [Google Scholar]
  59. Nayeen M. J. Mondal B. K. Basu S. R. Hossain J. Theoretical exploration of high VOC in Cu2SnS3 TFSCss towards high efficiency. Sol. Energy. 2023;265:112076. [Google Scholar]
  60. Rahman M A. Design and simulation of a high-performance Cd-free Cu2SnSe3 solar cells with SnS electron-blocking hole transport layer and TiO2 electron transport layer by SCAPS-1D. SN Appl. Sci. 2021;3(2):253. [Google Scholar]
  61. Paul S. Grover S. Repins I. L. Keyes B. M. Contreras M. A. Ramanathan K. Zhao R. N. Z. Liao F. Li J. V. Analysis of Back-Contact Interface Recombination in Thin-Film Solar Cells. IEEE J. Photovoltaics. 2018;8(3):871–878. [Google Scholar]
  62. Rahman M. A. Numerical modeling of ultra-thin CuSbS2 heterojunction solar cell with TiO2 electron transport and CuAlO2:Mg BSF layers. Opt. Mater. Express. 2022;12(8):2954–2972. [Google Scholar]
  63. Demtsu S. Sites J. Effect of back-contact barrier on thin-film CdTe solar cells. Thin Solid Films. 2006;510(1–2):320–324. [Google Scholar]
  64. Ofuonye B. Lee J. Yan M. Sun C. Zuo J. M. Adesida I. Electrical and microstructural properties of thermally annealed Ni/Au and Ni/Pt/Au Schottky contacts on AlGaN/GaN heterostructures. Semicond. Sci. Technol. 2014;29:095005. [Google Scholar]
  65. Zhaobin D. Wu F. Wang Y. C. Jiang H. Hong, Theoretical studies of the work functions of Pd-based bimetallic surfaces. J. Chem. Phys. 2015;142:214706. doi: 10.1063/1.4921895. [DOI] [PubMed] [Google Scholar]
  66. Etor D. Ekele M. Akintunde I. Impact of Metal Work-Function on Current Rectification by Metal-Insulator-Metal Diodes. J. Eng. Technol. 2022;7(2):430–434. [Google Scholar]
  67. Sze S. and Ng K. K., Physics of Semiconductor Devices, John Wiley and Sons, Inc., 1981 [Google Scholar]
  68. Singh P. Ravindra N. Temperature dependence of solar cell performance-an analysis. Sol. Energy Mater. Sol. Cells. 2012;101:36–45. [Google Scholar]
  69. Li J. Wang H. Luo M. Tang J. Chen C. Liu W. Liu F. Sun Y. Hand J. Zhang Y. 10% Efficiency Cu2ZnSn(S,Se)4 TFSCss fabricated by magnetron sputtering with enlarged depletion region width. Sol. Energy Mater. Sol. Cells. 2016;149:242–249. [Google Scholar]
  70. Fathi M. Abderrezek M. Djahli F. Ayad M. Study of TFSCss in high temperature condition. Energy Procedia. 2015;74:1410–1417. [Google Scholar]
  71. Phillips A. B. Subedi K. K. Liyanage G. K. Alfadhili F. K. Ellingson R. J. Heben M. J. Understanding and advancing bifacial TFSCss. ACS Appl. Energy Mater. 2020;3:6072–6078. [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

RA-014-D4RA05974C-s001
RA-014-D4RA05974C-s001.pdf (213.4KB, pdf)

Data Availability Statement

The data that support the findings of this study are available from the corresponding authors upon reasonable request.

Articles from RSC Advances are provided here courtesy of Royal Society of Chemistry

RESOURCES