Mountain gorilla tug-of-war: Silverbacks have limited control over reproduction in multimale groups

Abstract

To determine who fathers the offspring in wild mountain gorilla groups containing more than one adult male silverback, we genotyped nearly one-fourth (n = 92) of the mountain gorillas (Gorilla beringei beringei) living in the Virunga Volcanoes region of Africa. Paternity analysis of 48 offspring born into four groups between 1985 and 1999 revealed that, although all infants were sired by within-group males, the socially dominant silverback did not always monopolize reproduction within his group. Instead, the second-ranking male sired an average of 15% of group offspring. This result, in combination with previous findings that second-ranking males fare best by not leaving the group but by staying and waiting to assume dominance even if no reproduction is possible while waiting, is not consistent with expectations from a reproductive skew model in which the silverback concedes controllable reproduction to the second-ranking male. Instead, the data suggest a “tug-of-war” scenario in which neither the dominant nor the second-ranking male has full control over his relative reproductive share. The two top-ranked males were typically unrelated and this, in combination with the mixed paternity of group offspring, means that multimale gorilla groups do not approximate family groups. Instead, as long-term assemblages of related and unrelated individuals, gorilla groups are similar to chimpanzee groups and so offer interesting possibilities for kin-biased interactions among individuals.

Unequal distribution of reproduction is a feature of animal societies. Examining how social, ecological, and genetic factors jointly influence the partitioning of reproductive success in animal groups is essential to understanding the evolution of sociality (1). Over the last decade, various reproductive skew models (1–3) have been developed to predict how reproduction will be partitioned among individuals under different circumstances, greatly aiding efforts to go beyond asking what the patterns of reproductive sharing are toward asking why certain patterns are observed. That is, these models can facilitate the transition from descriptive to explanatory studies of reproductive strategies in wild animal, particularly primate, societies (4, 5).

Mountain gorillas (Gorilla beringei beringei) are unusual in that social groups can contain either a single or multiple fully adult males (silverbacks), and ≈40% of groups contain multiple adult males (6). Males may stay in their natal groups or emigrate to become solitary and possibly later form a new group (7). A single male is likely to monopolize reproduction in his group, as has been shown to occur in the one-male groups of the closely related western gorilla species (Gorilla gorilla) (8), but it is interesting to ask whether the socially dominant male monopolizes reproduction in multimale groups and, if not, why he might lose reproductive opportunities to his subordinate(s). Tug-of-war models [also known as “limited control” or “compromise” models (2, 9)] emphasize relative competitive abilities, and any reproductive sharing is proposed to reflect the inability of individual group members to successfully monopolize reproduction despite their best efforts to do so. This scenario best explains aspects of the long-term patterns of reproductive sharing among males in two particularly well studied primates featuring multimale groups, namely baboons (10) and macaques (11). It could also be applicable in mountain gorillas, where both dominants and subordinates have been observed to interfere with matings by the other (12).

In contrast to the tug-of-war model, the “concessions” model of reproductive skew emphasizes group stability benefits and ecological constraints upon breeding outside the group. The dominant individual is assumed to be potentially able to monopolize reproduction but instead strategically yields some reproductive opportunities to keep the subordinate in the group (1, 13). The concessions model may fit the patterns of reproduction in some species [e.g., paper wasp (14) and pukeko bird (15)] but has been ruled out in others [meerkats (16), woodpeckers (17), and social bees (18)]. The concessions model might explain the distribution of reproduction in some primate species, although this has not yet been tested by any quantitative data (5). In this light, it is notable that a key assumption of the concessions model, that the dominant male benefits from the presence of the subordinate, is clearly met in mountain gorillas. Multimale groups are more likely to attract and retain females (19, 20), the females become fertile at a younger age (21), and infants are less vulnerable to infanticide (20, 22, 23). Another assumption, that subordinates who reproduce are less likely to emigrate or challenge the dominant, is plausible in mountain gorillas, but data are lacking.

In mountain gorillas, females typically copulate with both dominant and subordinate males (12, 24), but whether this is because the dominant tolerates it (following a concessions model) or simply cannot prevent it (tug-of-war model) is unclear. The difficulty of quantifying parameters such as the degree of ecological constraints and the competitive abilities of individuals, along with a lack of predictions serving to clearly distinguish the models, makes the empirical testing of these models challenging (2, 9). However, one important prediction from the concessions model is that dominant individuals should concede less to related than to unrelated subordinates, because related subordinates will already gain some inclusive fitness benefits from the reproductive success of the dominant. In contrast, the tug-of-war model makes no predictions regarding the effect of relatedness upon reproductive sharing.

Patterns of relatedness among individuals within groups are also interesting for other reasons beyond testing of the concessions model. Although not incorporated into reproductive skew models (2), inbreeding avoidance could plausibly play a role in influencing paternity. Female mountain gorillas typically initiate copulations with males (24, 25). Therefore, breeding with their father or another close relative might be avoided by female mate choice in groups containing multiple adult males (24, 25). Mutual tolerance of related adult males in different groups has been proposed to explain the sometimes puzzlingly low levels of hostility observed in intergroup interactions in western gorillas (8), whereas the adult males living together in multimale mountain gorilla groups have been suggested to be relatives (7, 23).

Here we combine quantitative assessment of male reproductive output with consideration of social dominance rank and relatedness to evaluate the patterns of male reproductive success in four multimale wild mountain gorilla groups at the Karisoke Research Center, Rwanda, within the framework of the applicable reproductive skew models, namely tug-of-war and concessions models. Our goals are to use the results from noninvasive DNA analysis to quantify the variance in individual male reproductive success while considering the duration of male residency and total number of offspring in the group and then to use these data, in combination with observational information, to evaluate the effects of social dominance rank, age, number of potentially reproductive females, relatedness of potential sires to each other, and relatedness of potential sires to females upon probability of siring.

Materials and Methods

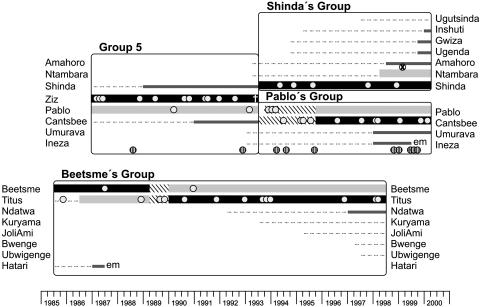
Behavioral and Demographic Data. The demography, ecology, and social dynamics of mountain gorilla groups at Karisoke Research Center, Volcanoes National Park, have been studied for more than three decades. Individuals in study groups are habituated to human observation, and male dominance relationships were inferred from behavioral observation (e.g., refs. 12, 19, 21–23, and 25; unpublished data). Analyses focused on four multimale groups for periods of 6–14 years (Fig. 1). Pablo's and Shinda's groups resulted from a fission of Group 5 after the death of the dominant silverback, Ziz, in 1993. Behavioral data collection was not possible during some time periods, and so the dominance relationships at the time of conception for nine offspring are unknown.

Fig. 1.

Reproductive careers of mountain gorilla males. Thick dark bars indicate the dominant silverback, whereas thick light bars indicate the second-ranking male. Bars are hatched during periods when the dominance relationships were unknown. Thin bars span the ages of 7–12 years. Males below the age of 7 are indicated by dotted lines. The circles represent offspring and are each placed on the line of the assigned father at the estimated time of conception. Striped circles are untyped offspring. The one typed offspring for which paternity could not be assigned, although the dominant male was excluded, is indicated by a circled x. em, emigration out of the group.

Sample Collection and Microsatellite Genotyping. Samples were collected and successfully analyzed from a total of 92 gorillas. Three types of sample material were used: ≈5-g portions of fresh feces (n = 87) stored in tubes containing either silica gel beads or 10 ml of RNAlater solution (Ambion, Austin, TX) (26), lyophilized feces originally collected in ethanol (n = 4), and shed hair (n = 1). Genomic DNA was extracted from fecal samples by using the Qiagen Stool Kit (Qiagen, Hilden, Germany) (26) and from hair using a simple digestion buffer (27).

The amount of amplifiable DNA in each extract was assessed through quantitative PCR, which permitted exclusion of unusable extracts of <12 pg/μl DNA as well as the identification of the number of independent PCR replications needed in the analysis of each extract to achieve 99% confidence in the homozygous microsatellite genotypes (28). Each allele of heterozygous genotypes was observed at least twice from independent PCRs. Individuals were genotyped at up to 15 microsatellite loci originally characterized in humans (von Willebrand factor; D1s550, D2s1326, D3s2459, D4s1627, D5s1470, D6s474, D6s1056, D7s794, D7s817, D7s2204, D10s1432, D14s306, D16s2624, and D18s851). Primer sequences, and PCR conditions are detailed in ref. 29 and Table 2, which is published as supporting information on the PNAS web site.

To avoid errors associated with gorilla misidentification, sample mix-up, or mislabeling, genotypes were verified in several ways. First, sex was assessed genetically by amplification of an X-Y homologous locus (30). Second, for many individuals (70%; n = 64), the genotype of the mother was also identified, allowing us to confirm that the samples from the offspring and mother shared an allele at each analyzed locus. Third, for most (25 of 28; 89%) of the individuals whose mother's genotype was not available, genotypes were confirmed by typing DNAs extracted from multiple samples. Finally, we used cervus (31) to conduct pairwise comparisons of all multilocus genotypes to confirm that samples from different individuals never yielded the same multilocus genotype.

Paternity. All sampled males who were of potentially reproductive age [>7 years; (24)] at the time of conception were considered candidate sires. In a first parentage analysis, we compared genotypes of offspring and potential within-group sires, including information from the mothers' genotypes whenever possible, to use allelic mismatches to exclude candidate males. Because this type of mismatch analysis cannot choose among two or more unexcluded males, we also used the program cervus to assess candidate fathers using a likelihood ratio approach (31). In one cervus analysis, we used as possible sires all males, regardless of group affiliation and, for each offspring, determined the most likely father and estimated the statistical support of the results assuming complete sampling of an average of four candidate males per offspring and 1% genotyping error. A second cervus analysis used the same parameters but limited the set of candidate sires to males resident in the group in which the offspring was conceived. Genotypes were available from all candidate sires present in the group at the time of conception for all 48 offspring evaluated. Individual paternity exclusion probabilities were calculated as in ref. 8, using allele frequencies from all individuals as well as from a subset of individuals (n = 38) who were not considered offspring in any analyses.

Relatedness Analyses. Relatedness (R) of all pairs of individuals was estimated based upon the extent of allele sharing and frequencies of alleles in the population (32). Although unrelated individuals, half-siblings, and full siblings are expected to have R estimates of 0, 0.25, and 0.5, respectively, in practice, estimates from natural populations deviate from these values because of inherent stochastic variation in the inheritance of alleles from a common ancestor and intrinsic limitations to allele frequency estimations (33). Likelihood analysis as implemented in kinship (34) was used to assess whether pairs of individuals were significantly more likely to represent a proposed relationship category (parent–offspring, full sibling, or half-sibling) or an unrelated pair. To avoid inconsistent results, these analyses used only genotypes for which both alleles at a locus had been determined.

Statistical Analyses. The genotype data were examined for departures from Hardy–Weinberg equilibrium and linkage disequilibrium between loci using exact tests as implemented in genepop, Version 3.3 (35). To quantify the variance in reproductive output among individuals while considering group productivity (i.e., number of offspring) and individual male residence periods, Nonacs' binomial skew B index (36) was calculated by using skew calculator 2003 (www.obee.ucla.edu/Faculty/Nonacs). Because three of the males in this study (Pablo, Cantsbee, and Shinda) spent time in more than one group (Fig. 1), the B index was calculated for the four groups separately, with each male in each group entered as a separate value, as well as for a “collapsed group,” including Group 5, Pablo's Group, and Shinda's Group, with each male considered as a single value.

A nonparametric Spearman partial rank correlation analysis examined the relationship of mean male reproductive success to mean rank or mean age, while controlling for the other variable. Means represent average yearly values and were used rather than total scores, because the males were studied for variable periods of time. A multivariate logistic regression evaluated how factors including number of potentially receptive adult females without offspring, number of males, and age differences between males influenced the dependent variable of dominant or subordinate siring. Analyses were performed by using spss Version 11.0 (SPSS, Chicago). The nine (18.8% of total) analyzed offspring conceived during periods in which the dominance relationships were unknown were excluded from these analyses.

Results

Genotypes. A total of 92 gorillas were genotyped at up to 15 loci. Almost all (97%; n = 88) individuals were typed at a minimum of five loci, and only 18 individuals were typed at fewer than eight loci. Genotypes were on average 63.8% complete, reflecting the fact that six of the loci were attempted only in a subset of ≈40 individuals (Table 3, which is published as supporting information on the PNAS web site). The figure of 63.8% does not include genotypes for which only one allele at a locus could be identified with certainty. Across the 15 loci, observed heterozygosities ranged from 0.61 to 0.82 (average, 0.71), with an average of 5.0 (range, 3–7) alleles per locus. When the genotypes of all individuals were analyzed for Hardy–Weinberg equilibrium (HWE), two loci exhibited significant heterozygote excess after Bonferroni correction (D4s1627, P = 0.002; D7s817, P = 0.0019). However, when the dataset including only individuals not considered as offspring in any analyses was tested, no loci were found to be in heterozygote excess. This suggests that the deviations from HWE can be attributed to the inclusion of related individuals in the complete dataset. No inconsistencies in expected patterns of allele sharing between mother and offspring (such as might arise from nonamplifying “null” alleles) were ever observed. Tests for genotypic disequilibrium by using either all genotypes or genotypes only from nonoffspring revealed no significant linkage after correcting for multiple comparisons.

Paternity. Paternity was assessed for 48 offspring born to 26 mothers in four groups between 1985 and 1999 (Fig. 1, Table 4, which is published as supporting information on the PNAS web site). We considered only offspring that survived at least to the age of 3 years for two reasons. First, this eliminated the possibility, arising out of interruptions of observations (due to civil unrest or other factors), that the births and early deaths of some offspring were undetected. Second, offspring mortality decreases markedly after age three (21, 23, 25), and so the number of offspring surviving to age three per male produces a consistent comparable measure of reproductive success. Ten additional offspring known to survive to age 3 during the relevant time period could not be analyzed, two because they died before commencement of sampling and eight because samples were unavailable or did not yield sufficient DNA (Fig. 1). In total, we analyzed 83% of the surviving offspring from the study time periods in these four groups.

Forty-seven of the 48 assessed offspring could be assigned to a single sire resident in the same social group (Fig. 1, Table 4). These assignments were based upon the following results. For 42 of the 48 offspring, all but one of the males was excluded by one or more mismatches and was also chosen by likelihood analysis with 95% confidence as the sire (Table 4). For three offspring (Kubaka, Rukundo, and Bikereri), all males but one were excluded for each, but lack of genotypes from the mothers resulted in 80% confidence for those sires in the likelihood paternity analysis. For one offspring (Turatsinze), neither of two males was excluded by mismatches, but in the likelihood analysis, the dominant silverback was judged significantly more likely to be the sire. One offspring (Urugamba) had mismatches to all candidates but in the likelihood analysis was assigned to the dominant male with high (95%) certainty despite a single mismatch apparently due to a mutation. For one offspring, Tegereza, the dominant silverback, Shinda, was excluded as the sire by two mismatches, but we could not determine which of the multiple unexcluded subordinate males was the father. Paternity exclusion probabilities ranged from 0.82 to 0.99 (mean, 0.98), with lower values reflecting little or no available maternal genotype information (Table 4).

Social Dominance and Reproductive Success. Males reproduced at ages ranging from 11.3 (Titus siring Kuryama) to ≈25 years (Beetsme siring Kirahure), and the mean siring age was 18.7 ± 2.8 years. All assigned offspring were sired by either the dominant or the second-ranking silverback (Fig. 1), although it should be noted that Titus showed the coloration of a maturing blackback rather than a silverback at the time of Kuryama's conception (D. Watts, personal communication). Considering only time periods for which the dominance relationships were known, subordinate males gained some portion of reproductive success in Beetsme's Group (3 of 13 offspring), Group 5 (2 of 13), and Shinda's Group (1 of 6). The frequency of subordinate sirings does not significantly differ between groups (Pablo's vs. Beetsme's, Fisher's exact test, P = 0.5211). Overall, 85% (n = 33) of offspring were sired by dominant silverbacks, and 15% (n = 6) were sired by nondominant males.

Although almost all of the dominant or second-ranking males sired some offspring, paternity was not evenly distributed within groups. Nonacs' B indices for all groups (Beetsme's Group = 0.341, Group 5 = 0.432, Pablo's Group = 0.337, Shinda's Group = 0.395, and “collapsed group” = 0.107) differed significantly from 0 (Shinda's group, P = 0.0028; all other P values, <0.001). All skew indices were largely consistent across the four separate groups (Table 5, which is published as supporting information on the PNAS web site), indicating that the overall degree of reproductive sharing among silverbacks within groups is similar among the four groups when adjusting for group size and male residency periods.

Rank, but not age, was found to influence reproductive success. Specifically, a Spearman rank analysis including all males who reached the age of 11 years during the time span covered by the study showed that rank was positively correlated with reproductive success (r_s = -0.594, P = 0.015, n = 16). This relationship remained when controlling for mean age (r_s = -0.5417; P = 0.037). In contrast, no relationship between mean age and mean reproductive success while controlling for mean rank was observed (r_s = 0.2355; P = 0.398). Logistic regression analysis including the age difference between the dominant and second-ranking silverback [exp(b) = 0.962, Wald χ² (1) = 0.293, P = 0.589], the number of females without infants in the group at the time of conception [exp(b) = 0.740, Wald χ² (1) = 0.666, P = 0.414], and the total number of silverbacks in the group [exp(b) = 1.306, Wald χ² (1) = 0.127, P = 0.721] indicated that none of these variables significantly contributed to whether the second-ranking male sired a given offspring.

Pairwise Relatedness and Kinship Assessments. We first compared the average R estimates of pairs of individuals of known familial relationships (parent–offspring, full siblings, and half-siblings) to evaluate how well the results met theoretical expectations (Table 6, which is published as supporting information on the PNAS web site). Although the average values for parent–offspring and half-siblings (the categories for which we had the most comparisons) were close to expectations, the actual dyadic values ranged widely (parent–offspring, n = 90, R = 0.43, SD = 0.18; half-siblings, n = 218, R = 0.23, SD = 0.27). The results imply that, because many R values are below the theoretical expectations, the mere examination of pairwise R values with these data without additional knowledge will not always lead to successful identification of related individuals (33).

Therefore, analyses were done by using likelihood tests of pedigree relationships as implemented in kinship to attempt to classify pairs of individuals as either significantly more likely to represent the primary hypothesis of a proposed relationship category, such as full siblings, or to represent the null hypothesis of unrelated (34). Even by using a dataset composed only of the 59 (82%) individuals completed at seven or more loci, we found that at P = 0.05, the estimated proportion of tests resulting in false rejection of the proposed hypotheses of full or half-siblings and erroneous acceptance of the null hypothesis (type II error) was 0.0629 and 0.4903, respectively, with even higher levels of error associated with smaller P values. In other words, in a high proportion of cases, pairs of individuals who were actually related as full or half-siblings were not identified as such. However, for the primary hypothesis of parent–offspring, tests with P = 0.05 or 0.01 were associated with relatively small type II error rates of <0.05.

Nonetheless, it was possible to use the genetic information, in combination with information from long-term observation, to make some cautious assessments of whether adults within the same group are relatives. When comparing silverbacks within groups, we found that the dominant male was only occasionally related to the second-ranking male, who was usually his successor (Table 1). In Group 5, Ziz and Pablo are possibly paternal siblings according to knowledge of potential sires present at the times of their conceptions and do have an estimated R value near the 0.25 expected for half-siblings, although the null hypothesis of no relationship was not rejected by using KINSHIP. The two highest-ranking silverbacks in Pablo's group (Pablo and Cantsbee) are also possible paternal siblings, and a half-sibling relationship was supported with significance by using KINSHIP. In sum, at most two of the five pairs of dominant and second-ranking males examined could be related as half-siblings. In no cases were the dominant and second-ranking males a father–son pair.

Table 1. Family relationships of dominant males and top-ranked subordinate males within groups.

					Siblings?
Original group	Dominant male	Second-ranking male(s)	Estimated R	Father–son?	Maternal	Paternal
Beetsme's	Beetsme	Titus	–0.07	No	No	Unknown
Group 5	Ziz	Pablo	0.18	No	No	Possibly
Pablo's	Pablo	Cantsbee	0.41	No	No	Probably*
Shinda's	Shinda	Amahoro	0.01	No	No	Possibly
		Ntambara	–0.42	No	No	Possibly

Genotypes were compared to determine whether dyads represented possible father–son pairs. Observational data indicated whether pairs shared a mother or possibly a father.

^*The only pair here estimated as significantly (P < 0.05) more likely to represent half-siblings than nonrelatives using kinship

In a second analysis considering relationships among adults within social groups, we examined whether the sirings by nondominant males could be explained by avoidance of breeding by related dyads of adult females and dominant males. The adult female and dominant silverback were clearly related in only one of the six instances in which a subordinate sired the offspring. In that single case, the female Mahane produced the offspring Ukuri with her possible paternal brother Pablo (R = 0.21, kinship full or half-sibling tests, both not significant), rather than with her likely father Ziz (R = 0.58, kinship parent–offspring test P < 0.01). In all other cases, relatedness values between the adult females and the dominant males were low, and kinship tests did not reject the null hypothesis of no relationship for tests considering parent–offspring, full sibling, or half-sibling as primary hypotheses. Of the sirings by the dominant male, very few of those 36 offspring can be shown to result from matings between females and a related dominant male. Two cases involve the same female, Walanza, mating with Ziz to produce offspring Ugenda and Nahimana (Walanza–Ziz, R = 0.30; kinship parent–offspring or full-sibling tests both P < 0.05), whereas the second-ranking silverback (Pablo) was unrelated to the female. In two other cases, females were related to both the available dominant as well as the second-ranking silverback (Pantsy produced Turatsinze with her known maternal brother Ziz, whereas the second-ranking male was her probable paternal sibling Pablo, R = 0.31, kinship half-sibling test, P < 0.05; Ntobo produced Urugero with relative Cantsbee, R = 0.45, kinship full-sibling test, P < 0.05, whereas the second-ranking male was relative Pablo, R = 0.50, kinship full-sibling test, P < 0.05). In sum, 5 of the 47 offspring for which paternity could be assigned appear to be the result of matings between relatives, and inbreeding avoidance by siblings does not appear to influence the pattern of sirings by nondominant males.

Discussion

Paternity analysis of 48 offspring born between 1985 and 1999 into four groups of wild mountain gorillas containing multiple adult males revealed that the socially dominant silverbacks did not completely monopolize reproduction within their groups. Of the 39 offspring sired when dominance relationships were known, 15% were sired by nondominant silverbacks. In five of the six cases of nondominant sires, the offspring was conclusively assigned to the second-ranking silverback. The second-ranking silverback is also a possible sire in the sixth case, although three other lower-ranking blackback males were also not excluded. We found no evidence of extra-group paternity, which is consistent with the rarity of observed extra-group copulations in mountain gorillas (37).

Mountain gorillas have been proposed to exhibit a form of age-graded social system, in which the subordinate adult males within groups are the offspring or siblings of the dominant male, although some groups are known to contain unrelated adult males (7, 23). However, none of the four groups examined here contained father–son pairs as the dominant and next-ranking silverback, which is not surprising, because the estimated age differences are only 3–9 years. Knowledge of the identities of the mothers of most silverbacks and the candidate sires for each, along with genetic analyses, showed that at most two of the five pairs of dominant and second-ranking males examined could be related as half-siblings (Table 1). Although from this limited sample it seems that top-ranking silverbacks within a group are only sometimes (and not very closely) related, this pattern will change over time if maturing blackbacks within these groups emerge as top-ranked subordinate silverbacks while the dominant remains unchanged.

Distinguishing between the skew models is not straightforward, but several lines of evidence suggest that reproductive partitioning among the silverbacks in these groups is less well explained by a concessions model than by a tug-of-war model in which neither the dominant nor the subordinate has full control over their relative reproductive success. First, although reproduction is not shared evenly among males (as indicated by B indices significantly higher than 0 in all four groups), overall reproductive skew is not as extreme as would be expected under a concessions model. A concessions model would predict that dominant silverbacks in multimale mountain gorilla groups would concede no, or only a slim, reproductive share to subordinates, because subordinate silverbacks already have an incentive to remain in the group (e.g., queuing for dominance) and are unlikely to gain reproductive success by emigrating (20, 38). The finding that subordinates reproduce in at least three of four groups at rates ranging from at least 15–23% of offspring does not fit this expectation and indicates that dominant silverbacks probably cannot prevent these sirings, rather than that they allow them. Another argument against the concessions model is the finding that older, formerly dominant, deposed males sometimes sire (Beetsme siring Kirahure at 25 years of age), even though reproductive opportunities would not be needed as an incentive for the subordinate to stay, because formerly dominant males already have an incentive to remain in the group (e.g., reduce infanticide risk to their own offspring) and are perhaps even less able to attract emigrating females as solitary males.

Under the concessions model, it is expected that the proportion of offspring sired by the dominant would be higher in groups containing related silverbacks, because the subordinate silverbacks would gain compensatory indirect fitness benefits. Although the observed frequency of subordinate sirings does not differ significantly between groups, the one group (Pablo's) with no observed subordinate sirings is also the group in which the dominant and second-ranking males are most convincingly shown to represent relatives (half-siblings). Although this may appear to favor the concessions model, we suggest that the impression of complete reproductive success by the dominant in Pablo's Group is misleading, because, during an earlier phase in Pablo's Group (1993–1995), there were indeed sirings by both silverback males, but the lack of observational information on the dominance relationships at the time precludes consideration of these seven offspring. In addition, eight surviving offspring from Pablo's Group could not be sampled, whereas at most two offspring were missed from the other groups (Fig. 1). Reflecting these facts, Nonac's skew index for this group is consistent with values from the other groups, indicating that levels of skew were similar after adjusting for group size, productivity, and male tenures.

If we accept that reproduction by subordinate silverbacks reflects the dominant's inefficacy at monopolizing reproduction, we can then ask what factors determine whether the dominant sires. Because they influence competitive ability and might confound the apparent effect of rank, factors such as the dominant's age and the age difference between the dominant and second-ranking silverback were investigated but not found to be significant in the partial correlation and multivariate analyses, respectively.

Subordinate sirings may reflect cases of inbreeding avoidance, that is, subordinates might have greater opportunities (through increased tolerance by the female, the dominant, or both) to mate with females that are related to the dominant silverback. However, most (five of six) of the cases of subordinate sirings cannot be attributed to avoidance of breeding by a related dominant male and female pair and, in the sixth case, the mother was related to both males. Offspring were produced by matings between siblings in the cases of 5 of 47 offspring and, for 3 of those offspring, the second-ranking male in the group was also related to the female. No offspring were produced by matings between father–daughter or mother–son pairs. Interestingly, for three of the five offspring produced by matings between relatives, the parents are known to come from different natal groups, showing that female transfer is not always effective in hindering inbreeding.

Along with relatedness and reproductive skew, other less readily quantifiable factors seen as important in reproductive skew models include ecological constraints on breeding outside the group, group productivity benefits, relative fighting ability of potential breeders, and the probability and value of resource inheritance (39). However, many factors (e.g., relatedness, group productivity, and fighting ability) become less relevant in situations in which subordinates do best by queuing for dominance rather than establishing new groups (40). Demographic data agree with recent modeling approaches in showing that, even in the absence of reproductive concessions, staying and queuing is a better strategy for subordinates than dispersing and attempting to form a new group (20, 23, 38).

A topic requiring further investigation is the role of female mate choice in determining patterns of reproduction in multimale mountain gorilla groups. The death of the silverback in a one-male group typically leads to disintegration of the group and, when females join other groups, any unweaned infants typically fall victim to infanticide by adult males (22). However, there are no documented cases of such infanticide occurring within groups containing multiple silverbacks, even when formerly subordinate males gain the dominant position either through a dominance shift or the death of the dominant silverback (6). This may be a result of successful female efforts to create paternity uncertainty in within-group males by copulating with both dominants and subordinates (41).

Although simultaneous estrus in group females is rare (24), and neither the total number of potentially reproductive females nor the total number of adult males in the group significantly influenced whether the dominant male sired, this does not necessarily mean that males can monitor single females successfully. Males exhibit sexually coercive behavior toward females, as would be expected under models of male–female sexual conflict in species with potentially infanticidal males (41), but females and not males initiate most copulations between fully adult individuals (24, 25). Indeed, mate choice by female primates is often subtle yet effective. This is well demonstrated by female chimpanzees who seem highly promiscuous throughout estrus but are in fact rather choosy on the days they are most likely to conceive (42).

Conclusion

The genetic data presented here and the behavioral observations to date best fit a scenario in which dominant and subordinate mountain gorilla males compete directly to maximize their immediate shares of reproduction, with limits possibly imposed by female mate choice, and so any single male is prevented from completely monopolizing reproduction in multimale groups. The lack of paternity monopolization means that, as in chimpanzees (43), not all similarly aged offspring in a group are paternal siblings. This is in contrast to western gorillas, where all paternities in each group examined thus far can be attributed to a single male (8). The resulting mixture of kin and nonkin within multimale mountain gorilla groups makes it possible that individuals bias their behavior toward kin and presents an ideal opportunity for future investigation of the role of paternal kinship upon social relationships.