Rare earth benzene tetraanion-bridged amidinate complexes

Abstract

The benzene tetraanion-bridged rare earth inverse arene amidinate complexes [{Ln(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] (2-Ln, Ln = Gd, Tb, Dy, Y; Piso = {(NDipp)₂C^tBu}, Dipp = C₆H₃ⁱPr₂-2,6) were prepared by the reduction of parent Ln(iii) bis-amidinate halide precursors [Ln(Piso)₂X] (Ln = Tb, Dy; X = Cl, I) or [Ln(Piso)₂I] (Ln = Gd, Y) with 3 eq. KC₈ in benzene, or by the reaction of the homoleptic Ln(ii) complexes [Ln(Piso)₂] (Ln = Tb, Dy) with 2 eq. KC₈ in benzene. The arene exchange reaction of 2-Tb with toluene gave crystals of [{Tb(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₇H₈)] (3-Tb), while no reactions were observed when C₆D₆ solutions of 2-Y were separately treated with biphenyl, naphthalene or anthracene. The reactivity study shows that 2-Y can behave as a four-electron reductant to reduce 1,3,5,7-cyclooctatetraene (COT). Complexes 2-Ln were characterized by single crystal X-ray diffraction, elemental analysis, SQUID magnetometry, UV-vis-NIR, ATR-IR, NMR, density functional theory (DFT) and ab initio calculations. These data consistently show that 2-Ln formally contain Ln(iii) centres with arene-capped inverse-sandwich Dipp–Ln(iii)–(C₆H₆)⁴⁻–Ln(iii)–Dipp configurations, and DFT calculations on a model of 2-Y revealed strong Y–(C₆H₆)⁴⁻ δ-bonding interactions between the filled π-orbitals of the benzene tetraanion and vacant 4d orbitals of the Y(iii) ions. A strong intermolecular coupling interaction between the two Tb(iii) centres in 2-Tb (J_tot = −6.84 cm⁻¹) was evidenced by a step in a magnetization vs. field plot of 2-Tb at ca. 3.4 T at 2 K, which we attribute to an anti-ferromagnetic transition of the magnetic moment; we also determined an exchange coupling constant J_ex = −0.25(1) cm⁻¹ for 2-Gd.

Arene capped inverse-sandwich rare earth complexes stabilized by Ln–[C₆H₆]⁴⁻ covalent δ-bonding.

Introduction

Sandwich compounds, which contain metals/metalloids bound by carbocyclic rings in a multihapto-fashion, have played a pivotal role in organometallic chemistry for nearly 75 years.^1,2 Substituted cyclopentadienyl (C₅R₅, Cp^R) and cyclooctatetraenyl (C₈R₈, COT^R) ligands have been most extensively investigated for f-element sandwich complexes due to their negatively charged aromatic carbon rings, which are well-suited to the predominantly ionic bonding for these metals.^3–7 Sandwich complexes of benzene and other substituted arene rings (C₆R₆) are well-developed for the d-transition metals,⁸ but because such ligands are typically neutral and aromatic with six π electrons, their rare earth (RE: group 3 and lanthanides; Ln) and actinide (An) chemistry is relatively immature.^9,10 For example, classic bis(benzene)metal complexes were established for the d-block metals in the 1950s,¹¹ but it took another 30 years for analogous RE complexes to be discovered, e.g. [M(C₆H₃^tBu₃-1,3,5)₂] (M = La–Lu, Y, Sc; I, Fig. 1).^12–15 A U(iii) complex containing a single arene, [U(C₆H₆)(AlCl₄)₃], was first reported in 1971,¹⁶ whilst the permethylated analogues [M(C₆Me₆)(AlCl₄)₃] (M = U, Sm) were structurally authenticated in 1987.¹⁷

Fig. 1. Selected examples of RE and An arene-coordinated sandwich (I), inverse-sandwich (II–IV), and inverse triple-decker (V) structures relevant to this work.

Extended RE and An sandwich complexes containing stacks of metal ions and aromatic rings have been synthesized; the simplest “inverse-sandwich” examples contain two f-block metals and one bridging carbocyclic ring.¹⁸ And the simplest “triple-decker” examples contain two f-block metals and three carbocyclic rings, with one ring bridging the two metals and two terminal rings.^19–24 To date RE multiple-decker complexes have been exclusively constructed using bridging COT^R ligands as these large dianionic rings are well-suited for stabilizing Ln(iii) or Ln(ii) ions,^25–27 including [Ln₂^III(COT′′)₃] (Ln = Y, La, Ce, Nd, Sm, Tb, Dy, Ho, Er, Tm, Lu; COT′′ = C₈H₆(SiMe₃)₂-1,4),^{21,22,28–31} [Sm₃^III/II/III(COT^TIPS)₄] (COT^TIPS = C₈H₆(SiⁱPr₃)₂-1,4)^20,32 and [cyclo-Ln^II(μ-η⁸:η⁸-COT^TIPS)]₁₈.³³ f-Block complexes made up of three arene rings have remained elusive to date, though multiple-decker sandwich complexes with bridging arenes such as [V₂(Cp)₂(μ-C₆H₆)]³⁴ and [Cr₂(C₆H₃Me₃-1,3,5)₂(μ-C₆H₃Me₃-1,3,5)]³⁵ are well-known for the d-block metals.^36,37

Over the last twenty-five years, many examples of RE and An complexes have been reported that formally contain reduced arene anions and variable metal oxidation states,^10,38–43 accommodating a variety of arene formal charges including e.g. 7π-electron radical monoanions (C₆H₆⁻˙) in [K(18-crown-6)(η²-C₆H₆)₂][{(Cp^tt)₂La^II}₂(μ-η⁶:η⁶-C₆H₆)] (Cp^tt = C₅H₃^tBu₂-1,3);¹⁸ 8π e⁻ dianions (C₆H₆²⁻) in [{[(NⁱPr)₂CN(SiMe₃)₂]₂Ln}(μ-η⁶:η⁶-C₆H₆)] (Ln = Y, Dy, Er),⁴⁴ [K(18-crown-6)]₂[{(Cp′′)₂Ln^II}₂(μ-η⁶:η⁶-C₆H₆)] (Ln = La, Ce; Cp′′ = C₅H₃(SiMe₃)₂-1,3; II),^45–47 and 10π e⁻ tetraanions (C₆H₆⁴⁻) in [{(Ts^R)₂U}₂(μ-η⁶:η⁶-C₆H₅Me)] (Ts^R = Tos^Tol, HC(SiMe₂NAr′)₃, Ar′ = C₆H₄Me-4; Tos^Xy, HC(SiMe₂NAr′′)₃, Ar′′ = C₆H₃Me₂-3,5; III),^48,49 [{(BDI)Ln^III(THF)_n}₂(μ-η⁶:η⁶-C₆H₆)] (Ln = Y, Sm; BDI = HC{C(Me)N[C₆H₃(ⁱC₅H₁₁)₂-2,6]₂}; IV)^50–52 and [{(C₅ⁱPr₅)Ln}(μ-η⁶:η⁶-C₆H₆)] (Ln = Y, Gd, Tb, Dy, Tm);⁵³ C₆H₆⁴⁻ rings have also been reported for Th arene complexes.^41,54 In most f-block complexes that contain reduced arenes these anions tend to be stabilized by two metal ions in a bridging motif, since the parent arene has a high reduction potential (e.g. benzene, E^Θ = −3.42 V vs. SHE)⁵⁵ and metal ions with vacant valence orbitals provide stabilization by accepting electron density via δ-bonding.^44–54 This metal-based stabilization is also seen in an inverse triple-decker complex [K(2.2.2-crypt)]₂[{(KL₃Ce)(μ-η⁶:η⁶-C₇H₈)}₂Ce] (V), which formally contains two bridging toluene dianions sandwiching three Ce(ii) ions.⁵⁶ There are a number of reports of RE and An complexes containing terminal arene anions,^57–62 though mononuclear [K(18-crown-6)][(Cp′′)₂Ln^III(C₆H₆)] (Ln = La, Ce) have been shown to contain (C₆H₆)²⁻ dianions.⁶³

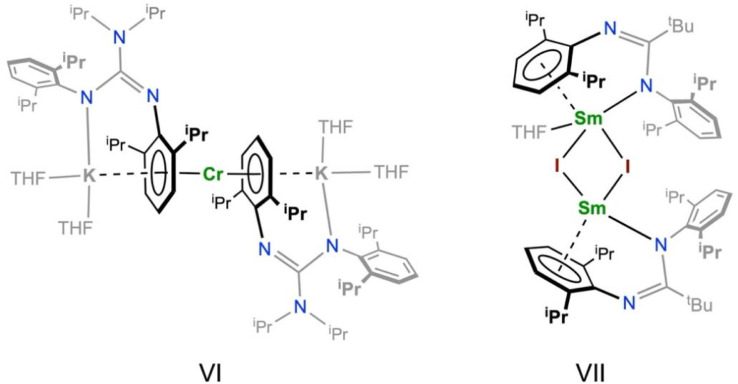
It has previously been shown that neutral arene rings of bulky terphenyl ligands such as NHAr^iPr6 (Ar^iPr6 = C₆H₃(C₆H₂ⁱPr₃-2,4,6)₂-2,6) can coordinate to RE and An ions to make a bis(arene) sandwich motif with two pendant N-donor ligands for Ln(ii) (La = Sc, Y, La, Sm, Eu, Tm, Yb)^64,65 and U(ii)⁶⁶ ions. Similarly, amidinate/guanidinate ligands with arene N-substituents have also shown that they can form bis(arene) d-transition metal complexes, e.g. the Cr(0) complex [Cr(η⁶-Priso)₂][K(THF)₂]₂ (VI; Priso = {(NDipp)₂CNⁱPr₂}, Dipp = C₆H₃ⁱPr₂-2,6), and f-block complexes with a κ¹-N,η⁶-chelating binding motif, e.g. the Sm(ii) complex [Sm(κ¹-N,η⁶-Piso)(THF)(μ-I)₂Sm(κ¹-N,η⁶-Piso)] (VII; Piso = {(NDipp)₂C^tBu}) (Fig. 2).^67,68 We therefore opted to use the bulky amidinate Piso⁻ as a supporting ligand in this work.^69,70

Fig. 2. Sterically demanding guanidinate (VI) and amidinate (VII) metal complexes with η⁶-bound N-arene substituents.

Here we present the synthesis and characterization of a series of structurally analogous Ln inverse-sandwich arene amidinate complexes, [{Ln(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] (2-Ln, Ln = Gd, Tb, Dy, Y) and [{Tb(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₇H₈)] (3-Tb). These complexes show arene-capped inverse structures with two Ln(iii) ions sandwiched in between three arene rings, with a bridging arene tetraanion and two terminal Piso ligands binding in a κ¹:η⁶-C₆ fashion. Magnetic studies of 2-Tb and 2-Gd revealed that the C₆H₆⁴⁻ bridge promotes a large intermolecular interaction between the two Ln(iii) centres, with steps at ∼±3.4 T observed in magnetization curves in 2-Tb and an exchange coupling constant J_ex = −0.25(1) cm⁻¹ determined in 2-Gd, while a weak antiferromagnetic interaction is seen between the two Dy(iii) centres in 2-Dy.

Results and discussion

Synthesis

The heteroleptic Ln(iii) bis-amidinate halide complexes [Ln(Piso)₂Cl] (1-Ln-Cl, Ln = Tb, Dy), [Ln(Piso)₂I] (1-Ln-I, Ln = Gd, Tb, Dy, Y) were prepared by the respective salt metathesis reactions of parent LnCl₃ ⁷¹ or LnI₃ ⁷² with two equivalents of KPiso⁷³ in toluene at reflux, by modifying literature protocols (Scheme 1).⁷⁴1-Ln-I (Ln = Tb, Dy) were reported previously.⁷⁴ The benzene-bridged arene-capped inverse-sandwich Ln(iii) amidinate complexes 2-Ln were synthesized by the reduction of 1-Ln-X with 3 eq. KC₈ in benzene; dark blue or purple crystals of 2-Ln were isolated in ca. 40% yields following work-up and recrystallization from dark purple (2-Gd, 2-Tb and 2-Dy) or dark blue (2-Y) pentane solutions at −35 °C (see Experimental section: synthesis for a more detailed description). As shown in our previous study, mononuclear divalent complexes [Ln(Piso)₂] (Ln = Tb, Dy) were obtained if [Ln(Piso)₂I] (Ln = Tb, Dy) are reduced with 1.1 eq. KC₈ in benzene.⁷⁵ During the synthesis of 2-Tb and 2-Dy, the colourless [Ln(Piso)₂I] precursors first converted to dark green [Ln(Piso)₂], and then to dark purple 2-Ln; these complexes can be alternatively prepared by the stoichiometric reaction of [Ln(Piso)₂] with KC₈ in benzene (Scheme 1). These reactions therefore formally proceed by the transfer of two electrons from the two Ln(ii) centres and two electrons from two K(0) to form 1 eq. of 2-Ln, with concomitant elimination of 2 eq. KPiso. By contrast, when 1-Y-I was treated with 1.1. eq. KC₈ in benzene only 2-Y and 1-Y-I could be assigned in the reaction mixture by NMR spectroscopy, and the putative dark green Y(ii) complex [Y(Piso)₂] was not detected by X-band EPR spectroscopy, which only revealed a sharp feature with g = 2.0048 (Fig. S81†); Y(ii) complexes tend to show g values slightly lower than 2.00.⁷⁶ We posit that once [Y(Piso)₂] is formed it reacts rapidly with KC₈ to give 2-Y, as Y(ii) is more reactive than Tb(ii) and Dy(ii) according to the standard reduction potential of the Y(iii)/(ii) couple (−2.66 V; −3.06 V) being lower than those of Tb(iii)/(ii) (−2.55 V; −2.95 V) and Dy(iii)/Dy(ii) (−2.56 V; −2.96 V) (The former vs. SHE and the latter vs. Fc⁺/Fc).⁷⁷ The differences in reduction potentials could be even larger for Y(iii)/(ii) and Ln(iii)/(ii) (Tb, Dy) ions in the [Ln(Piso)₂] coordination environment. The exchange reaction of 2-Tb in neat toluene at room temperature was evidenced by the isolation of crystals of 3-Tb after 24 h. We determined from colour changes of reaction mixtures that 3-Tb and its diamagnetic analogue 3-Y could also be prepared by the respective reactions of parent 1-Ln-X with excess KC₈ in toluene, analogously to the synthesis of 2-Ln. Elemental analysis and ATR-IR spectroscopy was performed on microcrystalline samples of 1-Ln-X, 2-Ln and 3-Tb, and further characterization data were obtained as appropriate. As we only isolated a small amount of 3-Tb we limited further characterization of this complex to single crystal XRD and UV-vis-NIR spectroscopy only (see below).

Scheme 1. Synthesis of 2-Ln from [Ln(Piso)₂Cl] (1-Ln-Cl, Ln = Tb, Dy) and [Ln(Piso)₂I] (1-Ln-I, Ln = Gd, Tb, Dy, Y).

Structural characterization

The solid-state structures of 1-Ln-X, 2-Ln and 3-Tb were determined by single crystal X-ray diffraction (see Fig. 3 for a depiction of 2-Tb-Cl, Fig. 4a and b for 2-Tb and Fig. S56–S62† for other structures). Crystals of [Ln(Piso)₂Cl] (Ln = Tb, Dy) are solvent-free, whereas 1-Ln-I (Ln = Gd, Y), 2-Ln and 3-Tb contain stoichiometric toluene, hexane or pentane in their crystal lattices, respectively. Complexes 1-Ln-X are five-coordinate, with the Ln centres bound by one chloride or iodide and four N-donor atoms from the two chelating κ²-N,N′-Piso ligands (Fig. 3 and S56–S58†), in common with the previously reported structures of [Ce(Piso)₂Cl] and [Ln(Piso)₂I] (Ln = Tb, Dy).^74,75 The coordination geometry is intermediate between trigonal bipyramidal and square pyramidal (τ₅ = 0.85 for Gd, 0.84 for Tb, 0.88 for Dy and 0.86 for Y).⁷⁸ Complexes 2-Ln and 3-Tb feature a triple-decker structure with a μ-η⁶:η⁶-arene forming the middle deck and two terminal η⁶-bound Dipp substituents of the Piso ligands, whereby the Piso ligands bind to the two Ln ions in a κ¹-N,η⁶-fashion to complete the Ln coordination spheres.

Fig. 3. Molecular structure of 1-Tb-Cl with selective atom labelling (C = gray, N = blue, Cl = yellow, Tb = violet). Thermal ellipsoids set at 50% probability level and hydrogen atoms omitted for clarity.

Fig. 4. (a) Molecular structure of 2-Tb; (b) central core of 2-Tb with selected atom labelling; and, (c) comparison of structural parameters between (C₆H₆)⁴⁻ and free benzene, which are extracted from the crystallographic data of 2-Tb and Chemcraft software, respectively.¹¹⁵ The displacement ellipsoids are set at the 50% probability level, and hydrogen atoms and lattice solvent are omitted for clarity. Key: Tb(iii) = violet, N = blue, C = gray.

The structural parameters for 2-Ln and 3-Tb are summarized in Table 1. The Ln–N distances of 2-Ln (Gd: 2.476(2)–2.488(2) Å; Tb: 2.446(5)–2.480(5) Å; Dy: 2.418(7)–2.438(7) Å and Y: 2.430(2)–2.442(2) Å) are longer than those of 1-Ln-X (Gd: 2.333(2)–2.444(2) Å; Tb: 2.333(3)–2.402(2) Å; Dy: 2.332(3)–2.388(3) Å; Y: 2.296(2)–2.397(2) Å), due to the change in Piso binding mode. By contrast with [Ln₂(η⁸:η⁸-COT)₂(μ,η⁸:η⁸-COT)],^28–31 the central cores of 2-Ln and 3-Tb feature bent geometries, with C_cent1–Ln1–C_cent2 angles of 137.16(2)° (2-Gd), 135.34(2)° (2-Tb), 134.45(3)° (2-Dy) and 134.17(2)° (2-Y) and Ln1–C_cent2–Ln2 angles of 177.50(2)° (2-Gd), 177.99(2)° (2-Tb), 178.20(2)° (2-Dy) and 177.42(1)° (2-Y), due to the steric requirements of the κ¹-N,η⁶-bound Piso ligand. The μ-η⁶:η⁶-bridging arenes in these complexes bind to the two Ln ions asymmetrically with Ln–C_arene distances of 2.446(3)–2.630(3) Å (2-Gd), 2.452(6)–2.632(7) Å (2-Tb), 2.419(9)–2.590(9) Å (2-Dy), 2.403(2)–2.604(2) Å (2-Y), 2.421(3)–2.615(2) Å (3-Tb), and with mean Ln–C_cent2 distances of 2.070(1) Å (2-Gd), 2.067(1) Å (2-Tb), 2.039(1) Å (2-Dy), 2.032(1) Å (2-Y) and 2.054(1) Å (3-Tb) (Fig. S59–S62† and Table 1). These are ∼0.4 Å shorter than the respective mean distances between the Ln centres and the terminal C₆-rings, due to the greater electrostatic attraction of tetraanionic (C₆H₆)⁴⁻vs. neutral Dipp rings. The carbocyclic C–C bond lengths of the bridging arenes in 2-Ln and 3-Tb are 1.430(9)–1.471(13) Å and 1.455(5)–1.466(4) Å, respectively, which are longer than the terminal Dipp rings of 1.378(16)–1.441(12) Å and the aromatic C–C distances of free benzene (1.391(1) Å)⁷⁹ (Fig. 4c). The bridging arenes in 2-Ln are not planar, e.g. in 2-Tb showing a dihedral angle of 12° between the planes defined by C9–C10 and C9, C8, C7, C12 (Fig. 4c), consistent with the arene ring being reduced. We cannot confidently assign (R–C₆H₅)²⁻ or (R–C₆H₅)⁴⁻ ring charges from structural data alone, as both rings can exhibit structural distortions from planarity as shown previously for other inverse-sandwich f-block arene complexes,^45–53 and (R–C₆H₆)⁴⁻ rings can be planar because of 10π-electron aromatic system.⁵⁴ Therefore, we used a combination of methods to probe whether Ln(ii)–C₆H₆²⁻–Ln(ii) or Ln(iii)–C₆H₆⁴⁻–Ln(iii) formulations best-describe the electron structures of 2-Ln (see below).

Table 1. Selected bond distances (Å) and angles (°) for 2-Ln (Ln = Gd, Tb, Dy, Y) and 3-Tb.

Complex	2-Gdd	2-Tb	2-Dy	2-Y	3-Tb
Ln1–N1	2.484(4)/2.488(2)	2.480(5)	2.446(7)	2.442(2)	2.469(2)
Ln2–N2	2.476(2)/2.488(2)	2.446(5)	2.424(7)	2.430(2)	2.463(3)
Ln1–Ca	2.793(3)–2.864(3)	2.766(7)–2.863(7)	2.744(9)–2.811(9)	2.744(2)–2.818(3)	2.793(4)–2.852(3)
	Avg. 2.833(3)	Avg. 2.817(6)	Avg. 2.768(9)	Avg. 2.775(3)	Avg. 2.819(3)
Ln1–Cb	2.446(3)–2.630(3)	2.452(6)–2.604(7)	2.419(9)–2.590(9)	2.426(3)–2.604(2)	2.437(3)–2.615(2)
	Avg. 2.532(3)	Avg. 2.516(7)	Avg. 2.501(9)	Avg. 2.498(2)	Avg. 2.519(3)
Ln2–Cb	2.450(3)–2.627(3)	2.456(6)–2.632(7)	2.422(9)–2.573(9)	2.403(2)–2.589(2)	2.421(3)–2.602(3)
	Avg. 2.532(3)	Avg. 2.527(7)	Avg. 2.507(9)	Avg. 2.501(2)	Avg. 2.516(3)
Ln2–Cc	2.812(3)–2.876(3)	2.795(7)–2.887(7)	2.727(9)–2.840(9)	2.739(2)–2.865(2)	2.798(3)–2.858(2)
	Avg. 2.844(3)	Avg. 2.830(6)	Avg. 2.770(9)	Avg. 2.793(3)	Avg. 2.826(3)
Ln1–Ccent1a	2.489(1)/2.461(1)	2.438(1)	2.392(1)	2.389(1)	2.436(1)
Ln1–Ccent2b	2.062(1)/2.067(1)	2.049(1)	2.035(1)	2.029(1)	2.054(1)
Ln2–Ccent2b	2.083(1)/2.070(1)	2.085(1)	2.042(1)	2.035(1)	2.055(1)
Ln2–Ccent3c	2.472(1)/2.474(1)	2.466(1)	2.405 (1)	2.420(1)	2.450(1)
C––C(ring)a	1.387(5)–1.389(5)	1.380(9)–1.425(9)	1.382(14)–1.430(13)	1.391(4)–1.426(3)	1.393(5)–1.432(4)
	Avg. 1.404(5)	Avg. 1.404(9)	Avg. 1.408(13)	Avg. 1.408(3)	Avg. 1.406(4)
C––C(ring)b	1.440(5)–1.469(5)	1.430(9)–1.469(10)	1.444(13)–1.471(13)	1.450(3)–1.468(3)	1.455(5)–1.466(4)
	Avg. 1.453(5)	Avg. 1.449(10)	Avg. 1.463(14)	Avg. 1.461(3)	Avg. 1.460(5)
C––C(ring)c	1.389(5)–1.433(4)	1.400(10)–1.434(9)	1.369(14)–1.429(12)	1.394(14)–1.428(11)	1.393(4)–1.428(5)
	Avg. 1.404(5)	Avg. 1.411(10)	Avg. 1.401(13)	Avg. 1.409(4)	Avg. 1.408(4)
Ln1–Ccent2–Ln2	177.50(2)/179.00(2)	177.99(2)	178.18(2)	177.42(1)	175.07(1)

^aThe C atoms and C_centroid1 belong to the Dipp group bound to Ln1.

^bThe C atoms and C_centroid2 belong to the bridging arene anion.

^cThe C atoms and C_centroid3 belong to the Dipp group bound to Ln₂.

^dTwo independent molecules of 2-Gd in the asymmetric unit.

Solution characterisation and reactivity studies

Solutions of 1-Ln-X and 2-Ln in C₆D₆ were characterised by NMR spectroscopy. The ¹H and ¹³C{¹H} NMR spectra of 1-Y-I and 2-Y in C₆D₆ all show resonances that are typical for diamagnetic Y(iii) complexes, supporting the assignment of S = 0 singlet ground states of the {Dipp–Y(iii)–(C₆H₆)⁴⁻–Y(iii)–Dipp} motif, with the expected arene resonances presenting ¹J_YC coupling constants (⁸⁹Y, I = ½, 100% abundant) while no ²J_YH coupling observed. In 1-Y-I, the ¹³C{¹H} NMR spectrum features a doublet resonance at δ_C = 182.69 ppm (²J_YC = 2 Hz) due to the quaternary CN₂ fragment of Piso showing a chelating κ²-N,N′-binding mode to the Y(iii) centre (Fig. S6†). By contrast, for 2-Y a singlet peak was observed in the ¹³C{¹H} NMR spectrum at 172.29 ppm as a κ¹-N,η⁶-binding mode is adopted. Due to the strong interaction between the Y(iii) centres and C₆H₆⁴⁻, triplet resonances of bound C₆H₆⁴⁻ in 2-Y were seen at δ_C = 65.51 ppm (¹J_YC = 5.1 Hz), but a singlet resonances at δ_H = 2.05 ppm without ²J_YH coupling observed, which are shifted considerably from free benzene singlet resonances seen at δ_C = 127.69 ppm and δ_H = 7.15 ppm, respectively (Fig. S11 and S12†). The chemical shift is primarily influenced by the shielding effect, which is closely related to the charge density around the hydrogen atoms. The charge distribution calculations reveal electron-rich C₆H₆⁴⁻ rings compared to the arene rings of terminal Dipp groups (Tables S30 and S31†), increasing shielding and leading to an upfield chemical shift. Similar triplet resonances were previously observed in the ¹H and ¹³C{¹H} NMR spectrum of complex IV for the Y(iii)-bound (C₆H₆)⁴⁻ moiety at δ_C = 65.74 ppm (¹J_YC = 4.8 Hz) and δ_H = 2.37 ppm (¹J_YH = 1.6 Hz),⁵⁰ though we note that there is a large disparity in both the chemical shift and coupling constant for the ¹H NMR resonances. Four additional arene resonances were observed in the ¹³C{¹H} NMR spectrum of 2-Y for the ipso-, ortho-, meta- and para-positions of the Dipp groups that are bound to the Y(iii) centres, and these resonances are shifted by ca. 20 ppm downfield of the respective four peaks of the unbound Dipp groups. The meta-positions of the terminally bound Dipp groups also present a doublet of triplets (dt) resonance in the ¹H NMR spectrum of 2-Y at δ_H = 6.99 ppm (²J_HH = 7.7 Hz, ¹J_YH = 4.2 Hz) for the Fig. S11.† We were unable to interpret the ¹H and ¹³C{¹H} NMR spectra of 1-Dy-Cl, 1-Tb-Cl, and 2-Dy due to paramagnetic broadening, whilst the ¹H NMR spectrum of 2-Tb could be tentatively assigned by relative integrals, though these signals were also paramagnetically broadened and shifted to various extents between −90 and 290 ppm (Fig. S7†). The bridging (C₆H₆)⁴⁻ ring gives a sharp singlet peak at δ_H = 63.67 ppm, indicating that in solution dynamic processes lead to (C₆H₆)⁴⁻ not retaining the asymmetry seen in the solid state structure, as otherwise there would be several small peaks of varying intensity.

Arene exchange reactivity studies were performed on C₆D₆ solutions of 2-Y, and these reaction mixtures were monitored by ¹H and ¹³C{¹H} NMR spectroscopy (Fig. S15–S19†); such exchange processes have previously been reported for a wide range of inverse-arene RE and An complexes.^10,38,39,61 However, arene exchange did not proceed when separate 1 : 1 mixtures of 2-Y and biphenyl, naphthalene or anthracene were heated to 80 °C for over 100 hours, demonstrating the kinetic stability of 2-Y in C₆D₆ (Fig. S16–S18†). Similar results for polyaromatic hydrocarbons (PACs) such as naphthalene, anthracene and biphenyl have previously been reported for other Ln and An complexes with tetraanionic (C₆H₆)⁴⁻ bridging units.^50,52,54 To extend this study and to account for the formation of 3-Tb, we monitored the exchange reaction of a C₇D₈ solution of 2-Y in an NMR tube at 80 °C for over 100 hours (Fig. S19†). No peaks corresponding to 3-Y were observed, with the NMR spectra appearing to show 2-Y and some minor decomposition products, indicating that arene exchange did not proceed under these conditions. A similar situation is also found for a solution of 2-Tb in C₇D₈ in an NMR tube, where no obvious arene exchange occurred at 80 °C for over 100 hours (Fig. S22†). However, a sample of 2-Y dissolved in toluene at room temperature and stirred for 12 h showed that arene exchange proceeds readily under these conditions to give 3-Y, analogously to the formation of 3-Tb. Volatiles were removed in vacuo and the reaction mixture was monitored by ¹H and ¹³C{¹H} NMR spectroscopy to reveal signals at δ_C = 123.04 ppm and δ_H = 5.2 ppm that may correspond to the Y(iii)-bound (C₆H₅CH₃)⁴⁻ moiety of 3-Y (Fig. S13 and S14†). However, the arene exchange did not proceed to completion, with traces of the (C₆H₆)⁴⁻ moiety of 2-Y seen at δ_C = 65.58 ppm and δ_H = 2.05 ppm, with a 2-Y : 3-Y ratio of 37 : 63. This indicates that using large amounts of toluene with stirring could increase the rate of the exchange reaction and allow it to proceed at a lower temperature. Similar stability differences between benzene anions and alkyl-substituted aromatic rings have been reported in previous reactivity studies of f-block inverse-sandwich complexes.^50,80,81

The separate reactions of 2-Y with COT under molar ratios of 1 : 2 and 1 : 4 were performed in C₆D₆. The reaction mixtures changed colour from dark blue to pale yellow within 5 min, indicating that 2-Y has been fully consumed. Both reactions were monitored by ¹H NMR spectroscopy, giving relatively clean spectra (Fig. S20†). No traces of (C₆H₆)⁴⁻ remained at 2.05 ppm but a new signal for (C₈H₈)²⁻ was seen at 6.59 ppm, showing that C₈H₈ had been reduced and (C₆H₆)⁴⁻ had been oxidised. For the 1 : 4 reaction of 2-Y with COT, about 53% free COT was also detected at 5.63 ppm, which lowers to 5% in the 1 : 2 reaction. This indicates that 2-Y acts as a four electron reductant in terms of reducing COT. This is similar to the recently reported (C₆H₆)⁴⁻ bridged Sm₂ complexes [{(BDI)Sm(THF)_n}₂(μ-η⁶:η⁶-C₆H₆)], in which 1 equiv. (C₆H₆)⁴⁻ can also reduce 2 equiv. COT to yield a mononuclear product [(BDI)Sm(COT)].⁵⁰

We also investigated the reactivity of 2-Y with THF, by treating 2-Y (12 mg, 0.01 mmol) with a drop of THF-d₈ in C₆D₆ (0.6 mL) or neat THF-d₈ (0.6 mL). The colour of the former solution lowered in intensity but remained blue, while the latter reaction mixture became colourless after several minutes. These two reactions were monitored by ¹H NMR spectroscopy (Fig. S21†), where in the former a weak trace of triplet signal of C₆H₆⁴⁻ remained, whilst no signal for C₆H₆⁴⁻ was seen for the neat THF-d₈ solution. This shows that 2-Y is not stable in THF as it reacts with this ethereal solvent, via oxidation of C₆H₆⁴⁻.

UV-vis-NIR spectroscopy

The UV-vis-NIR absorption spectra of separate hexane or benzene solutions of 1-Ln-X, 2-Ln and 3-Tb were determined from 250–800 nm (12 500–40 000 cm⁻¹) (Fig. 5 and S33–S55†). Strong and sharp absorptions are found below 300 nm (ε > 6000 M⁻¹ cm⁻¹) for all complexes. Similar absorptions have been observed for other Piso⁻ complexes, as well as both HPiso and KPiso, and these are therefore assigned to intraligand π to π* transitions (Fig. 5).⁷⁴ The Ln(iii) complexes 1-Ln-X appear colourless and their electronic absorption spectra are essentially featureless in the visible and NIR region, with no Laporte-forbidden f–f transitions observed for 1-Gd-I, 1-Tb-Cl or 1-Dy-Cl (Fig. S32–S34†).³ Hexane or benzene solutions of the arene-bridged complexes 2-Ln and 3-Tb are dark green to dark blue, and show broad absorption bands stretching across most of the visible region from 500–700 nm; the intensities of these transitions likely vary with solvent due to differences in dielectric constants and intermolecular interactions. Amongst this series of complexes, 2-Y gives the largest extinction coefficients, with maxima in benzene at ṽ_max ∼17 240 cm⁻¹/∼580 nm (ε = 11 500 M⁻¹ cm⁻¹) (Fig. S49–S51†); similar features are seen for 2-Gd (ṽ_max 17 120 cm⁻¹; ε = 1296 M⁻¹ cm⁻¹ in benzene), 2-Tb (ṽ_max 17 240 cm⁻¹; ε = 8350 M⁻¹ cm⁻¹ in benzene and ε = 5015 M⁻¹ cm⁻¹ in hexane) (Fig. S36–S48†), 2-Dy (ṽ_max 17 540 cm⁻¹/570 nm; ε = 2180 M⁻¹ cm⁻¹) (Fig. S43–S48†) and 3-Tb (ṽ_max 16 670 cm⁻¹/600 nm; ε = 970 M⁻¹ cm⁻¹) (Fig. S52†). These absorptions are mainly attributed to the charge transfer transitions between the bridging arenes and the phenyl of terminal Piso ligand, which differ from the broad bands covering the visible region in [{(BDI)M(THF)_n}₂(μ-η⁶:η⁶-arene)] (M = Y, Sm) based on C₆H₆²⁻ and R–C₆H₅⁴⁻,⁵⁰ and are bathochromically shifted compared to ṽ_max ≈ 540 nm in [{(Cp′′)₂La}₂(μ-η⁶:η⁶-C₆H₆)]²⁻.⁴⁵ The spectra of 2-Ln are comparable to those of parent Ln(ii) complexes [Ln(Piso)₂] (Ln = Tb, Dy), but the peak maxima shift to higher energy from ca. 700 nm to 580 nm, and the extinction coefficients of 2-Tb are much higher than seen for [Tb(Piso)₂] (2100 M⁻¹ cm⁻¹). Similar to [Ln(Piso)₂], the high thermostability of 2-Ln was demonstrated by heating benzene solutions of 2-Ln 80 °C for 12 hours; the intense colours were maintained and the UV-vis-NIR spectra show the same absorption features as fresh solutions, but with lower extinction coefficients (Fig. S53–S55†).⁷⁵

Fig. 5. Experimental UV-vis-NIR spectra of solutions of (above) 1-Gd-I, 2-Gd, 1-Tb-Cl, 2-Tb, 1-Dy-Cl, 2-Dy and 3-Tb in benzene at room temperature; (bottom) experimental (purple) and calculated (orange) UV-vis spectra of 2-Y in benzene at room temperature, with pertinent theoretical excitations shown as vertical lines. The full width at half maximum (FWHM) in the simulated UV-vis spectrum is set at 0.5 eV.

Magnetism

To better understand the electronic structures and arene anion exchange coupling between the Ln centres of 2-Ln, static and dynamic magnetic susceptibility data were collected for microcrystalline samples of 1-Tb-Cl, 1-Dy-Cl, 2-Gd, 2-Tb, 2-Dy and 2-Y suspended in eicosane (Fig. 6 and S63–S80†). The magnetic susceptibility temperature product (χ_MT) was determined for 1-Ln-Cl and 2-Ln between 1.8 and 300 K under an applied dc field of 1000 Oe. The χ_MT products at 300 K of 1-Tb-Cl, 1-Dy-Cl, 2-Tb and 2-Dy are 12.27, 13.80, 21.98 and 28.39 cm³ mol⁻¹ K, respectively. The values for 1-Ln-Cl are comparable to the corresponding expected free-ion χ_MT values for 4f⁸ Tb(iii) (11.81 cm³ mol⁻¹ K) and 4f⁹ Dy(iii) (14.17 cm³ mol⁻¹ K), and that of 2-Dy essentially matches the theoretical value for two Dy(iii) ions (28.34 cm³ mol⁻¹ K), whilst the value for 2-Tb is slightly below that predicted for two Tb(iii) ions (23.62 cm³ mol⁻¹ K). The free-ion χ_MT values of Tb(ii) and Dy(ii) under both 4fⁿ⁺¹ (4f⁹, Tb(ii), 14.17 cm³ mol⁻¹ K; 4f¹⁰, Dy(ii), 14.06 cm³ mol⁻¹ K) and 4fⁿ5d¹ (4f⁸5d¹, Tb(ii), 12.19–14.43 cm³ mol⁻¹ K; 4f⁹5d¹, Dy(ii), 14.55–17.02 cm³ mol⁻¹ K) valence electronic structures are not consistent with those measured for 2-Ln.^75,82,83 Additionally, the solution magnetic susceptibilities of 2-Tb and 2-Dy were determined at 298 K by the Evans method;⁸⁴ the values obtained (χ_MT = 23.24 and 28.24 cm³ mol⁻¹ K) are in line with the solid-state magnetic data and that expected for two uncoupled Tb(iii) and Dy(iii) ions (χ_MT = 23.62 and 28.34 cm³ mol⁻¹ K).

Fig. 6. (a) Temperature dependent susceptibility of χ_MT in an applied dc magnetic field of 1 kOe for 2-Dy, 2-Tb and 2-Gd. The black solid lines represent the best fit from ab initio calculations (2-Dy and 2-Tb) or eqn (1) (2-Gd). (b) Plot of natural log of the inverse relaxation time vs. temperature for 1-Tb-Cl and 1-Dy-Cl. The plots are from the ac susceptibility measurements with applied field (0 Oe for 1-Dy-Cl and 2 kOe for 2-Tb-Cl). The lines are the best fit.

For 2-Gd, the χ_MT product at 300 K is 15.89 cm³ K mol⁻¹, which is excellent agreement with the theoretical value of 15.76 cm³ K mol⁻¹ for two uncoupled Gd(iii) ions (⁸S_7/2, g = 2). When the temperature is decreased, the χ_MT value keeps almost constant until around 75 K, and then starts to decrease more rapidly which is mainly attributed to intramolecular antiferromagnetic (AFM) coupling (Fig. 6a). The fitting of χ_M⁻¹versus T above 50 K based on the Curie–Weiss law gives a negative Weiss constant θ = −1.21 K and a Curie constant C = 15.83 cm³ K mol⁻¹. Furthermore, the analytical Van Vleck equation based on the equivalent operator approach and the spin Hamiltonian of Ĥ = −JŜ_Gd1Ŝ_Gd2 was used to reproduce the dc magnetic susceptibility data (eqn (1)). The best fit gives the parameter of J = −0.25(1) cm⁻¹ when the Landé factor g is fixed as 2.00, which is smaller but comparable to the values J = −0.642(6) cm⁻¹ and −2.94(2) cm⁻¹ for other biphenyl or benzene inverse-sandwich Gd complexes {[(NN^TBS)Gd]₂(μ-biphenyl)}⁻ (NN^TBS = fc(NSi^tBuMe₂)₂, fc = 1,1′-ferrocenediyl),^53,85 especially the latter has the shortest Gd–arene distances 2.039(6) Å observed to date. These values are much larger than those reported previously for Gd₂ systems with non-radical bridges,⁸⁶ indicating that the tetraanionic arene⁴⁻ bridge is capable of transmitting strong magnetic exchange. In addition, measurements on 2-Y at 300 K and 150 K and under 1000 Oe or 10 000 Oe showed it was diamagnetic. These data indicate that 2-Ln feature Ln(iii)–(C₆H₆⁴⁻)–Ln(iii) rather than Ln(ii)–(C₆H₆²⁻)–Ln(ii) configurations.

The χ_MT values of 2-Dy decrease gradually with temperature from 300 K to 2 K, where a large χ_MT value of 24.33 cm³ mol⁻¹ K persists, while the χ_MT values of 2-Tb decrease more markedly to reach 9.50 cm³ mol⁻¹ K at 2 K. These data indicate that there is a stronger antiferromagnetic (AFM) interaction in 2-Tb than 2-Dy (Fig. 6a, S65 and S66†). For 1-Dy-Cl, the χ_MT value decreases steadily with temperature to ca. 4 K followed by a sharp drop-off to 6.60 cm³ mol⁻¹ K at 2 K, likely because of slow magnetic dynamics (Fig. S63†). By contrast, 1-Tb-Cl exhibits a smooth downturn in χ_MT below ca. 20 K (Fig. S64†). Together, the static magnetic data for 1-Tb-Cl and 2-Tb indicate that magnetic dynamics are faster than the timescale of the experiment, and that 1-Dy-Cl exhibits slower magnetization dynamics than 1-Tb-Cl and 2-Ln. The zero-field cooled (ZFC) and field-cooled (FC) magnetic susceptibility traces support this assertion, showing bifurcation at 4 K for 1-Dy-Cl (Fig. S67†), while bifurcations are not found for 1-Tb-Cl and 2-Ln.

The magnetic relaxation rates of 1-Ln-Cl and 2-Ln (Ln = Tb, Dy) were probed by ac magnetic susceptibility experiments. Amongst these complexes, 1-Dy-Cl and 1-Tb-Cl exhibit single-molecule magnet (SMM) behaviour. For 1-Dy-Cl, slow magnetic relaxation with peaks in were observed under zero dc field between 2 and 23 K (Fig. S69†), while for 1-Tb-Cl the non-Kramers Tb(iii) ion has non-zero splitting between the lowest-lying pseudo-doublet states (|+m_J> and |−m_J>), so peaks were observed between 2 and 19 K under an optimal dc field of 2000 Oe (Fig. S68†). No ac signals were seen for 2-Tb and 2-Dy due to the large tunnelling splitting (Δ_tun) with strong QTM for exchange-coupled ground states (Tables S26 and S27†).⁸⁷ The ac data of 1-Ln-Cl were fitted with a generalized Debye model⁸⁸ in CC-FIT2 ^89,90 to give the temperature dependence of magnetic relaxation times, which can be described with the general equations τ⁻¹ = CTⁿ + τ_QTM⁻¹ and τ⁻¹ = CTⁿ and fitted using CC-FIT2 to give C = 10^{−2.253±0.042} s⁻¹ K⁻ⁿ; n = 4.394 ± 0.034; τ_QTM = 10^{−1.531±0.014} s for 1-Dy-Cl, and C = 10^{−0.727±0.054} s⁻¹ K⁻ⁿ and n = 3.502 ± 0.051 for 1-Tb-Cl (Fig. 5b, S72 and S73†). In these equations, two terms are combined: the through-barrier two-phonon Raman mechanism (phenomenological parameters C and n), and τ_QTM, the QTM timescale. However, the over-barrier Orbach mechanism τ⁻¹ = τ₀⁻¹ exp[−U_eff/T] (where U_eff is the effective barrier to magnetic reversal and τ₀ is the attempt time of the phonon bath) is not included due to an order of magnitude faster Raman process in 1-Tb-Cl compared to its' respective iodide analogues [Tb(Piso)₂I].⁷⁵ The Ln–X distances in [Ln(Piso)₂I] are much longer than those in 1-Ln-Cl, leading to weaker Ln–X interactions, a smaller transverse field, slower magnetic relaxation and higher U_eff = 491 ± 32 and 800 ± 16 K for [Tb(Piso)₂I] and [Dy(Piso)₂I], respectively.⁷⁵

Variable temperature magnetization vs. dc field measurements of 1-Ln-Cl and 2-Ln were performed with field sweep rates of 22 Oe s⁻¹; all traces showed butterfly-shaped hysteresis loops at 2 K, confirming efficient QTM at zero field (Fig. S74–S80†). As expected, by 2 K the hysteresis loops for the non-Kramers Tb(iii) complexes 1-Tb-Cl are closed in the zero field region and this loop is only slightly open at higher fields at this temperature (Fig. S78†). For 1-Dy-Cl the hysteresis loops are completely closed at 4 K (Fig. S79†), which is consistent with the ZFC-FC bifurcation temperature and substantially lower than [Dy(Piso)₂I], which shows loops that are opened at higher fields up to 10 K.⁷⁵ The hysteresis loops of 2-Tb and 2-Dy are nearly closed at all fields by 2 K (Fig. S80†). A step-like loop is found in 2-Tb at 2 K as the applied magnetic field sweeps between ±5 T (Fig. S80† bottom), while the magnetization of 2-Dy is saturated above ∼1.5 T (10.5 μ_B at 5 T) without a step-shape (Fig. S80† above). The same 2-Tb step was observed in magnetization curves from 0 to 7 T at 2 K, but this is not present at 4 K and 7 K due to thermal excitation (Fig. S76 and S77†). The magnetization of 2-Tb doesn't appear to be saturated even at 7 T, with the steps in magnetization for 2-Tb located around ±3.4 T through calculation of its first derivative; this was attributed to the AFM interactions introduced by the arene-bridge.

Electronic structure calculations

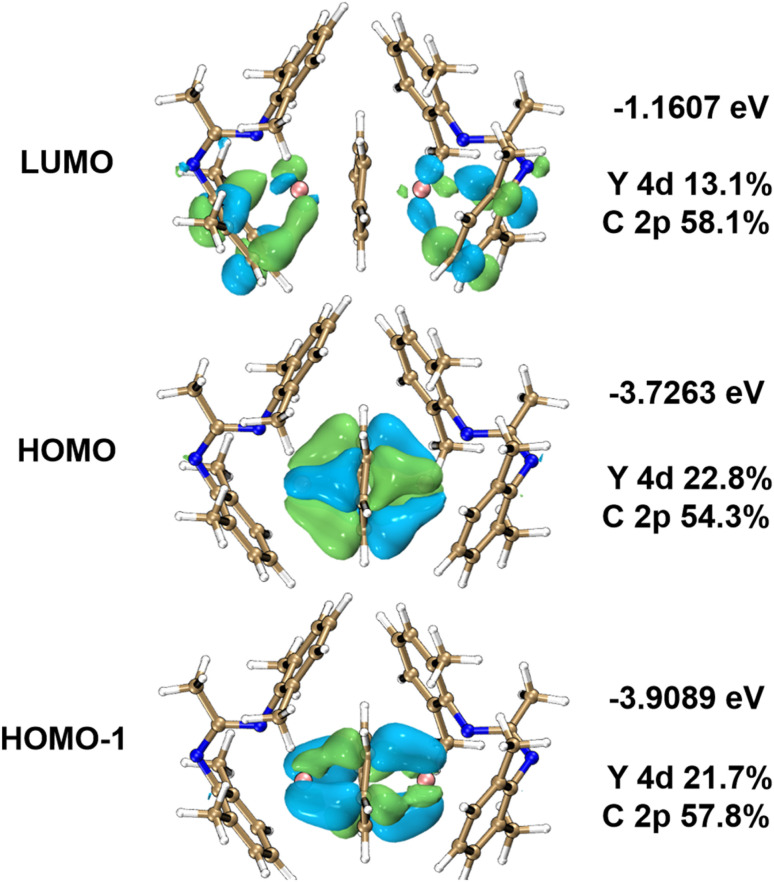
To gain further insights into the bonding features of 2-Ln and to verify the electronic states of the arene bridges, we performed density functional theory (DFT) calculations on a model complex of 2-Y using Orca 5.0,⁹¹ whereby the ^tBu and ⁱPr groups on the Piso ligands were replaced to methyl groups for simplification. The closed-shell electron configuration was considered in the geometry optimization, and the corresponding optimized structure (2-Y^opt) gives similar structural parameters compared with the single crystal XRD data for 2-Y (Fig. S82†); minor differences of bond lengths and angles were attributed to changes in steric and electronic effects from replacement of R-groups. For example, the Y⋯Y distance and mean Y–N and Y–C_cent2 bond lengths calculated for 2-Y^opt are 4.00, 2.40 and 2.00 Å, which are slightly shorter than the experimental values for 2-Y of 4.06(1), 2.44(1) and 2.03(1) Å, respectively. Meanwhile, the mean C–C bond distances of the bridging arenes for 2-Y^opt and 2-Y are both around 1.46 Å, larger than the typical C–C bond lengths of ∼1.39 Å for a neutral benzene ring;⁷⁹ such C–C distances are also close to the other similar systems.^50,92,93 The computational results concur with magnetic and spectroscopic data that 2-Ln show Ln(iii)–(C₆H₆⁴⁻)–Ln(iii) configurations. The frontier molecular orbitals of 2-Y^opt are depicted in Fig. 7, where both the highest occupied molecular orbital (HOMO) and HOMO−1 show a characteristic δ-bonding feature between the tetraanionic arene bridge and Y(iii) ions.^50,92,93 These orbitals are close in energy (−3.73 for HOMO and −3.91 eV for HOMO−1), and show respective compositions of 22.8% and 21.7% for the Y(iii) 4d orbitals, and 54.3% and 57.8% for the 2p orbitals of the bound C₆H₆⁴⁻. By contrast the lowest unoccupied molecular orbital (LUMO) consists of weaker π-bonding interactions between the aryl group of the Piso ligands and the Y(iii) centres, with orbital density more localized in the π* orbitals of the terminal arene rings. The LUMO is situated at a much higher energy of −1.16 eV and is comprised of 13.1% 4d orbital character of the Y(iii) ions and 58.1% 2p orbitals of the bound C₆H₆⁴⁻. The δ-bonding feature in these molecular orbitals is similar to that seen previously for other inverse arene f-block complexes, including similar group 3 systems such as [{(BDI)M(THF)_n}₂(μ-η⁶,η⁶-benzene)] and [[(NN^TBS)M]₂(μ-η⁶,η⁶-C₆H₅Ph)]²⁻ (M = Y and Sm), etc.^50,92,93

Fig. 7. DFT-calculated frontier molecular orbitals (FMOs) for 2-Y^opt (isovalue = 0.04 a.u.). Orbital energies and corresponding compositions with Mulliken partitions are given on the right. Colour codes: Y, pink; N, blue; C, tan; H, white.

The natural population analysis (NPA) and atoms-in-molecules (AIM) charges were calculated for 2-Y^opt (Tables S30 and S31†). The NPA charge on the entire bound arene is −1.43, which is close to other dimeric Ln₂ complexes possessing such tetraanionic benzene bridges, like [{(BDI)M(THF)_n}₂(μ-η⁶,η⁶-benzene)] (−1.87 and −1.56 for M = Y and Sm)⁵⁰ and [{(NN^TBS)M}₂(μ-η⁶,η⁶-C₆H₅Ph)]²⁻ (−1.90 and −1.56 for M = Y and Sm),^92,93etc. Meanwhile, the average NPA charge of Y is 1.24, much lower than 3, which reflects the charge transfer between the Piso and (C₆H₆)⁴⁻ ligands and adjacent Y(iii) ions. In contrast, the corresponding calculated AIM charges show slightly higher values, 1.81 for Y and −1.95 for the whole bound benzene. The Mayer bond orders (MBOs) of the Y–C, Y–N and C–C bonds of the bridging arene in 2-Y^opt were calculated (Table S9†). The mean Y–N and Y–C MBOs are 0.15 and 0.14, which agree with polarized covalent bonding, while the bridging C–C arene bonds show MBOs of around 1.08. These latter values are much smaller than those expected for a neutral aromatic ring (MBOs of 1.38–1.46 at different computational levels),⁹⁴ showing that the intra-ring π-bonds have been weakened, and consistent with the longer mean C–C distances and distorted C₆ ring of C₆H₆⁴⁻.^50,92,93 Moreover, certain real space functions based on electron densities ρ(r) at bond critical points (BCPs) were investigated by AIM topology analysis to quantitatively investigate the bonding characteristics of its coordinated bonds (Table S32†).^95,96 As expected, all BCPs of Y–N and Y–C bonds possess small ρ(r) values of less than 0.06 a.u., corresponding to typically weak interactions.^97,98 In particular, the ρ(r) values at Y–C(C₆H₆⁴⁻) BCPs are comparable to the complex [{(BDI)Sm(THF)_n}₂(μ-η⁶,η⁶-C₆H₆)], where the ρ(r) of 0.0488 a.u. for the Sm(iii)–C₆H₆⁴⁻ bonding is almost twice as large as those for M(ii)–C₆H₆²⁻ bonding (0.0277 a.u. for Ca, 0.0353 a.u. for Yb, 0.0251 a.u. for Sr and 0.0279 a.u. for Sm).⁵¹ The positive Laplacian functions of the electron density ∇_ρ(r)² at the BCPs suggest the electrons are locally depleted, a feature of closed-shell interactions.^99,100 The negative energy density function H(r) and the ratio of |V(r)|/G(r) larger than one for Y–N and Y–C(C₆H₆⁴⁻) interactions reflect the existence of covalent components in these weak interactions, while the Y–C(Piso) values have the opposite ranges and exhibit more ionic features.^101,102 The BCPs reveal typical features of dative covalent bonds for Y–C(C₆H₆⁴⁻), demonstrating that the electronic structure of Ln(ii)–(C₆H₆)²⁻–Ln(ii) is impossible here where much stronger covalency between Ln and bound arene should be observed.

Time-dependent (TD)-DFT calculations were conducted on 2-Y^opt considering the solvent effect from benzene through the SMD solvent model (Table S10†).¹⁰³ As expected, the simulated UV-vis spectrum matches well with the experimental one (Fig. 5 bottom). The calculated transition with the largest oscillator strength (f) is located around 583 nm. This absorption has contributions from the excitation of HOMO−1 → LUMO (34.3%), HOMO → LUMO+3 (31.9%) and HOMO → LUMO+1 (21.9%), corresponding to the π → π* transitions from the bridging arenes to the terminal coordinated C₆ rings. The second strongest transition at 556 nm possesses the same excitation type with HOMO → LUMO+3 (53.4%). The higher energy transitions (<290 nm) are largely localized within Piso-based orbitals, with some excitation to the vacant Y(iii) 4d orbitals.

The magnetic dynamics and relaxation mechanisms of 1-Dy-Cl, 2-Dy, 1-Tb-Cl and 2-Tb were probed by complete active space self-consistent field spin–orbit (CASSCF-SO) calculations.^104,105 For both dimeric complexes, the single-ion magnetic anisotropy of individual {Ln(Piso)(C₆H₆)} fragments were computed. The results revealed Ln(iii) ground states with strong magnetic axiality approaching the Ising limit, with g_z values close to 20 and 18 for Dy(iii) and Tb(iii), respectively. The highly pure wavefunction compositions of the Ln(iii) ground states are well-described as m_J = |±15/2> and |±6> for Dy(iii) and Tb(iii), respectively (Tables S11–S16†). The corresponding principal magnetic axes are all coincident with the coordinated nitrogen atoms of the Piso ligands, indicating that they impose a strong crystal field, even stronger than equatorial coordinated chloride in 1-Dy-Cl and 1-Tb-Cl as well as the two arene rings with delocalized electron distributions in 2-Dy and 2-Tb (Fig. S83–S85†). For 1-Tb-Cl and the two fragments of 2-Tb, relatively large tunnelling gaps (Δ_tun) of 0.01 and 0.02 cm⁻¹ were calculated between the m_J = |±6> ground states, which correspond to rather strong QTM (Tables S14–S16†), while superior SMM properties were predicted for 1-Dy-Cl and the two fragments of 2-Dy, with calculated U_eff values of 585, 495 and 492 K (Fig. S86 and S87†). A relatively small ground-state tunnelling transition moment of 1.77 × 10⁻⁴μ_B can be observed in 1-Dy-Cl and this guarantees its zero-field SMM behaviour in ac susceptibility measurements. To determine the intramolecular magnetic couplings in 2-Dy and 2-Tb, the POLY_ANISO program based on the Lines model was used to fit their dc molar magnetic susceptibility data.^106–108 The best fit gives the coupling parameters listed in Table 2, and the total antiferromagnetic (AFM) interactions (J_tot) of −0.64 (2-Dy) and −6.84 cm⁻¹ (2-Tb) originate from the competition between ferromagnetic (FM) dipole–dipole couplings and AFM exchange interactions. We note that the calculated g_z values of exchange-coupled ground states for 2-Dy and 2-Tb are 26.403 and 7.528, respectively (Tables S26 and S27†), and this deviation from zero is ascribed to the misaligned anisotropic axes of the Ln(iii) ions (Fig. S85†). DFT-BS (broken symmetry) calculations were performed on a Gd(iii) analogue of 2-Y^opt (2-Gd*) to verify the AFM contributions of exchange couplings (Table S28†), and after scaling by the corresponding factors, the respective J_ex values for 2-Dy and 2-Tb are estimated as −5.02 and −3.48 cm⁻¹. By contrast, the cycloheptatrienyl trianion (C₇H₇³⁻, Cht), of 10π-aromatic system same as (C₆H₆)⁴⁻, is reported to promote significant ferromagnetic exchange coupling between Ln ions in the inverse-sandwich complex [KLn₂^III(μ-η⁷:η⁷-Cht){N(SiMe₃)₂}₄] (Ln = Dy, Gd, Er).^109,110 As described in II (C₆H₆)⁻ and (C₆H₆)²⁻ feature radical S = 1/2 and diradical S = 1 and were also shown to transfer extremely strong magnetic exchange coupling, −400 cm⁻¹ and −497 cm⁻¹ between La(ii) and (C₆H₆)⁻/(C₆H₆)²⁻ respectively.^42,45 The exchange and dipolar interactions of 2-Ln can also be compared to the related tetraanionic biphenyl bridged Dy₂ complexes [{(NN^TBS)Dy}₂{(μ-biphenyl)K₂(solvent)₂}] (solvent = Et₂O, toluene, Dy₂-biph; 18-crown-6, Dy₂-biph-crown₂) (Table 2). Both Dy₂-biph and Dy₂-biph-crown₂ show similar structural parameters of the bridging arene⁴⁻ to 2-Dy, with mean C–C distances of 1.46 Å and torsion angles of 11 and 12°, respectively, and present AFM dipolar coupling and FM exchange coupling.⁸⁵ For 2-Dy and 2-Tb the opposite situation is observed, with FM dipolar and AFM exchange coupling. This discrepancy can be attributed to differences in the N-donor ligands and the amount of electron density in bridged arene⁴⁻ rings (biphenyl vs. benzene) in these complexes,⁸⁵ showing that tetraanionic arene bridges can be designed to promote AFM or FM intramolecular magnetic couplings in Ln₂ complexes. Despite this, the tunnelling splitting (Δ_tun) for exchange-coupled ground states are large, with magnitudes of ∼10⁻⁶ (2-Dy) and ∼10⁻⁵ cm⁻¹ (2-Tb) (Tables S26 and S27†), which leads to strong QTM and poor zero-field SMM performance for both complexes.

Table 2. The intramolecular magnetic coupling constants in 2-Dy and 2-Tb (cm⁻¹), and their comparison to similar tetraanionic biphenyl bridged Dy₂ complexes Dy₂-biph and Dy₂-biph-crown₂.

Complex	POLY_ANISO			DFT-BS
	J ex	J dip	J tot	J ex
2-Tb	−7.73	0.89	−6.84	−3.48
2-Dy	−1.38	0.74	−0.64	−5.02
Dy2-biph85	1.06	−2.48	−1.42	—
Dy2-biph-crown285	7.25	−2.50	4.75	—

Conclusion

In summary, we have reported straightforward synthetic routes to C₆ aromatic ring-based arene-capped inverse-sandwich Ln(iii) complexes by the treatment of [Ln(Piso)₂X] or [Ln(Piso)₂] with KC₈ in arene solvents. The Ln(iii) ions in these inverse arene complexes are also bound by pendant amide donor atoms due to κ¹-N,η⁶-binding of their bulky amidinate ligands. The triple-decker (Dipp)Ln(μ-Arene)Ln(Dipp) frameworks feature near-linear Ln⋯Arene_centroid⋯Ln and highly bent Arene_centroid⋯Ln⋯Dipp_centroid geometries. Structural parameters, spectroscopic and magnetic data are consistent with the bridging arene rings being formally tetraanionic and the two terminal Dipp rings being neutral. TD-DFT calculations on a model of the Y(iii) analogue showed excellent agreement with experimental UV-vis spectra. Analysis of the DFT-calculated frontier orbitals of [{Y(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] indicated that strong Ln-arene δ-bonding interactions are present in this complex, with electron density donated from filled π-orbitals of formal (C₆H₆)⁴⁻ fragments to formally vacant Y(iii) 4d orbitals.

Magnetic studies on paramagnetic [{Ln(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] revealed that the bridging benzene tetraanion promotes large AFM interactions between the two Ln(iii) centres, and an exchange coupling parameter of J_ex = −0.25(1) cm⁻¹ was extracted from the χ_MT curve of 2-Gd. These results highlight the advantages of the flexible binding mode of the Piso ligand to enable the stabilization of inverse-sandwich Ln arene complexes and provide fresh insights into magnetic communication between two Ln centres promoted by a benzene tetraanion. Arene exchange reactions of [{Y(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] with selected PACs in benzene did not proceed under the conditions employed, indicating that these complexes have high kinetic stability, though arene exchange proceeds readily for [{Tb(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] in neat toluene. The bridging C₆H₆⁴⁻ ligand functions as an electron reservoir in [{Ln(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)], and is a potent four electron reductant.

Experimental Section

General methods

Dry toluene and pentane were obtained from a solvent purification system, where they were passed through columns containing alumina and molecular sieves, and then stored over K mirrors. Benzene and hexane were dried by refluxing over potassium, and were stored over K mirrors following distillation. All solvents were degassed before use. For NMR spectroscopy C₆D₆ was dried by refluxing over K. NMR solvents were vacuum transferred and degassed by three freeze–pump–thaw cycles before use. All experiments were performed under an atmosphere of dry argon with the rigid exclusion of air and moisture, using standard Schlenk and glovebox techniques. ¹H (400 MHz) and ¹³C{¹H} (101 MHz) NMR spectra were obtained on an Avance III 400 MHz spectrometer at 298 K. The magnetic susceptibilities of C₆D₆ solutions of 1-Ln-Cl and 2-Ln for Ln = Tb and Dy were determined at 298 K by the Evans method;⁸⁴ the lower than expected values obtained for 1-Ln-Cl are attributed to precipitation of sample during the measurement. UV-vis-NIR spectroscopy was performed on samples in Youngs tap-appended 10 mm pathlength quartz cuvettes on an Agilent Technologies Cary Series UV-vis-NIR Spectrophotometer from 175 to 3300 nm. ATR-IR spectra were recorded as microcrystalline powders using a Bruker Alpha II spectrometer. Elemental analysis experiments were performed on an EA 3000 elemental analyser at the Instrument Analysis Center, Xi'an Jiaotong University.

Single crystal XRD

The crystal data for 1-Ln-X, 2-Ln and 3-Tb are compiled in ESI Tables S1 to S3.† Crystals of 1-Ln-X were collected on an Oxford Diffraction SuperNova Atlas CCD diffractometer using mirror-monochromated MoKα radiation (λ = 0.71073 Å), 2-Ln and 3-Tb were collected on a Rigaku XtaLAB Synergy-DW VHF equipped with a HyPix-6000HE photon counting pixel array detector with mirror-monochromated CuKα (λ = 1.5418 Å) radiation, and 1-Gd-I and 2-Gd were recorded on a Bruker SMART CCD diffractometer with MoKα radiation (λ = 0.71073 Å). All data collections were performed at 100 K. The structures were solved by direct methods and were refined by full-matrix least-squares on all unique F² values, with anisotropic displacement parameters for all non-hydrogen atoms, and with constrained riding hydrogen geometries. CrysAlisPro¹¹¹ was used for control and integration, and SHELXT^112,113 was employed through OLEX2¹¹⁴ for structure solution and refinement. Chemcraft and DIAMOND was used for crystallographic images and structural parameters comparasion.^115,116

Magnetic measurements

Magnetic measurements were performed on a Quantum Design MPMS3 and PPMS superconducting quantum interference device (SQUID) magnetometer. Samples of 1-Dy-Cl (28 mg), 2-Dy (22.1 mg), 1-Tb-Cl (22.6 mg), 2-Gd (16.0 mg), 2-Tb (27.5 mg) and 2-Y (22.4 mg) were ground to powders, loaded in borosilicate glass NMR tubes, and covered in ground eicosane (10, 10.6, 8.9, 12.5, 4.5, 12.2 and 12.1 mg respectively) in a glovebox. The tubes were removed from the glovebox, kept under a protected atmosphere, and transferred onto a Schlenk line, where the eicosane powder was melted under an argon atmosphere. The tubes were flame-sealed to ∼3 cm under reduced pressure. Kapton tape was wrapped around the top of the tubes, to hold them inside plastic straws by friction, and the straws were attached to the end of the sample rod. The raw data were corrected for the diamagnetic contribution of the sample holder and eicosane using calibrated blanks. The data were corrected for the sample shape using the Quantum Design MPMS3 and PPMS Geometry Simulator resulting in correction values of 0.956–1.039; for each individual sample: height 2.32 mm, diameter 4.08 mm (1-Gd-I), height 3.94 mm, diameter 4.08 mm (1-Tb-Cl), height 2.89 mm, diameter 4.09 mm (2-Tb), height 6.19 mm, diameter 4.09 mm (1-Dy-Cl) and height 4.03 mm, diameter 4.08 mm (2-Dy). Finally, the data were corrected for the intrinsic diamagnetic contribution of the sample, estimated as the molecular weight (g mol^–1) multiplied by 0.5 × 10^–6 cm³ mol^–1 K. The dc magnetic susceptibility data of 2-Gd was fitted with eqn (1):

1

EPR spectroscopy

Continuous wave EPR spectra were recorded on a Bruker EMX PLUS spectrometer operating at X-band (ca. 9.85 GHz). Modulation amplitudes of 4 G were used at microwave powers of 6.325 mW. Spectra were recorded for a reaction mixture containing 1-Y-I and 2 eq. KC₈ in benzene at room temperature (100 mg for 1-Y-I, 23 mg for KC₈; the reaction was stopped after 24 h when the mixture gradually changed from colourless to dark blue, and then settled for 3 hours). Approximately 0.3 mL supernatant solution from this reaction mixture was loaded in a 4 mm diameter and 250 mm height J. Young tap quartz tube in an Ar glovebox.

Calculations

All calculations were performed using Orca 5.0 program.⁹¹ For geometry optimization calculation towards the model of 2-Y, the hybrid PBE0 functional¹¹⁷ was employed with the D3BJ dispersion correction.^118,119 Scalar relativistic effects were taken into account with the zeroth order regular (ZORA) Hamiltonian.¹²⁰ The SARC-ZORA-TZVP basis set was employed for Y, together with the def2-TZVP basis set for C and N atoms and def2-SVP basis set for H atoms.^121–123 The SARC/J auxiliary basis set¹²² and the RijCosX approximation^124,125 were also used throughout. The keyword tightscf was used to improve the convergence threshold and to obtain credible results. The natural population analysis (NPA) was performed with NBO 6.0¹²⁶ and the rest of wavefunction analysis was carried out through Multiwfn program.¹²⁷ The frontier molecular orbitals of 2Y^opt were represented and rendered by the visual molecular dynamics (VMD) software.¹²⁸ For TD-DFT calculations, the optimized coordinates of 2-Y^opt were used and 75 excited states were considered under SMD solvent model.¹⁰³ In DFT-BS calculations, the same sets were treated to the established model complex 2-Gd*. Ultimately, isotropic exchange coupling constants for 2-Dy and 2-Tb were estimated through the Yamaguchi formula and further scaled for Dy(iii) and Tb(iii) by the factors of 49/25 and 49/36 respectively.¹²⁹

For CASSCF-SO calculations, the individual fragments of 2-Dy and 2-Tb were established according to their X-ray single crystal data without optimization, with one of the paramagnetic Ln(iii) centres replaced with diamagnetic Lu(iii) in each case. Scalar relativistic effects were considered with the second-order Douglas–Kroll–Hess (DKH) Hamiltonian.^130–132 The SARC2-DKH-QZVP basis set was employed for Dy, Tb and Lu, with the DKH-def2-TZVP basis set used for C and N atoms and the DKH-def2-SVP basis set for H atoms.^123,133 The keyword AutoAux¹³⁴ and the RijCosX approximation^124,125 were also used throughout. To save computational resource, only 21 sextets or 7 septets were considered in the state-averaged calculations for Dy(iii) and Tb(iii) complexes, respectively.^104,105 The static magnetic properties of Dy(iii) or Tb(iii), such as crystal field parameters and g tensors were obtained in the SINGLE_ANISO module.^135,136 The intramolecular exchange and dipole–dipole couplings between both paramagnetic ions in 2-Dy and 2-Tb were then computed by fitting with the experimental dc molar magnetic susceptibilities using POLY_ANISO program.^106–108

Synthesis

Anhydrous TbCl₃, DyCl₃, YI₃ and KPiso were prepared according to literature methods,^71–73 as was KC₈ (Note: highly reactive and pyrophoric, and must be handled and disposed of with care).¹³⁷ GdI₃ and cyclooctatetraene was purchased from a commercial source (Aladdin Scientific) and was used as received.

[GdPiso₂I]·C₇H₈ (1-Gd-I)

GdI₃ (1.076 g, 2 mmol) and KPiso (1.832 g, 4 mmol) were combined in a 200 mL Rotaflo tap-pressure flask containing a glass-coated magnetic stirring bar, and toluene (50 mL) was added under argon. The reaction mixture was heated gradually to 150 °C with vigorous stirring. After 5 days at 150 °C, the flask was cooled to room temperature, the reaction mixture was filtered, and concentrated under vacuum until copious precipitation of white solid (∼10 mL). The precipitate was re-dissolved by heating the solution to 100 °C. The flask was then wrapped with Al foil and allowed to cool slowly to room temperature overnight. Colourless block crystals of the product formed and were isolated by filtration, washed with cold pentane (2 × 5 mL) and dried under vacuum (1.29 g, 53% based on GdI₃). C₆₅H₉₄IN₄Gd: calcd (%) C 64.22, H 7.79, N 4.61 found (%) C 63.88, H 7.35, N 4.81. UV-vis-NIR ṽ_max/cm⁻¹: 34 200 (ε = 11 700 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3339w, 3058w, 2958s, 2868m, 1651w, 1614m, 1584w, 1456m, 1430m, 1408m, 1393w, 1330m, 1305w, 1251w, 1200w, 1168w, 1106w, 1039w, 932m, 826w, 800m, 758s, 716w.

[TbPiso₂Cl] (1-Tb-Cl)

TbCl₃ (0.53 g, 2 mmol) and KPiso (1.832 g, 4 mmol) were combined in a 200 mL Rotaflo tap-appended pressure flask containing a glass-coated magnetic stirring bar, and toluene (50 mL) was added under argon. The reaction mixture was heated gradually to 150 °C with vigorous stirring. After 5 days at 150 °C, the flask was cooled to room temperature, the reaction mixture was filtered, and concentrated under vacuum until copious precipitation of white solid (∼10 mL). The precipitate was re-dissolved by heating the solution to 100 °C. The flask was then wrapped with Al foil and allowed to cool slowly to room temperature overnight. Colourless block crystals of the product formed and were isolated by filtration, washed with cold pentane (2 × 5 mL) and dried under vacuum (0.920 g, 45% based on TbCl₃). ¹H and ¹³C{¹H} NMR spectra could not be interpreted. C₅₈H₈₆ClN₄Tb: calcd (%) C 67.39, H 8.39, N 5.42; found (%) C 67.14, H 8.22, N 5.49. χ_MT product = 9.15 cm³ mol⁻¹ K (Evans method). UV-vis-NIR ṽ_max/cm⁻¹: 35 200 (ε = 8330 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3339w, 2957s, 2868m, 1614m, 1584w, 1457s, 1424s, 1390m, 1338s, 1299m, 1242w, 1168w, 1099w, 1041w, 932w, 798w, 756s, 744w.

[DyPiso₂Cl]·C₅H₁₂ (1-Dy-Cl)

DyCl₃ (0.54 g, 2 mmol) and KPiso (1.832 g, 4 mmol) were combined in a 200 mL Rotaflo tap-appended pressure flask containing a glass-coated magnetic stirring bar, and toluene (50 mL) was added under argon. The reaction mixture was heated gradually to 150 °C with vigorous stirring. After 5 days at 150 °C, the flask was cooled to room temperature, the reaction mixture was filtered, and concentrated under vacuum until copious precipitation of white solid (∼10 mL). The precipitate was re-dissolved by heating the solution to 100 °C. The flask was then wrapped with Al foil and allowed to cool slowly to room temperature overnight. Colourless block crystals of the product formed and were isolated by filtration, washed with cold pentane (2 × 5 mL) and dried under vacuum (0.770 g, 37% based on DyCl₃). Crystals of 1-Dy-Cl suitable for single crystal XRD were obtained by recrystallizing from pentane, and elemental analysis results obtained were in agreement with a pentane molecule being present in the lattice. ¹H and ¹³C{¹H} NMR spectra could not be interpreted. C₆₃H₉₈ClN₄Dy: calcd (%) C 68.20, H 8.83, N 5.05 found (%) C 68.12, H 8.77, N 5.14. χ_MT product = 11.12 cm³ mol⁻¹ K (Evans method). UV-vis-NIR ṽ_max/cm⁻¹: 35 200 (ε = 8860 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3345m, 2953s, 2868m, 1614s, 1582m, 1466m, 1436m, 1399w, 1317s, 1242w, 1202w, 1166w, 1099w, 1050w, 926w, 759s.

[YPiso₂I]·C₇H₈ (1-Y-I)

YI₃ (0.94 g, 2 mmol) and KPiso (1.832 g, 4 mmol) were combined in a 200 mL Rotaflo tap-pressure flask containing a glass-coated magnetic stirring bar, and toluene (50 mL) was added under argon. The reaction mixture was heated gradually to 150 °C with vigorous stirring. After 5 days at 150 °C, the flask was cooled to room temperature, the reaction mixture was filtered, and concentrated under vacuum until copious precipitation of white solid (∼10 mL). The precipitate was re-dissolved by heating the solution to 100 °C. The flask was then wrapped with Al foil and allowed to cool slowly to room temperature overnight. Colourless block crystals of the product formed and were isolated by filtration, washed with cold pentane (2 × 5 mL) and dried under vacuum (1.060 g, 46% based on YI₃). ¹H NMR (400 MHz, C₆D₆), δ, ppm: 0.83 (s, 18H, C(CH₃)₃), 1.16 (d, ³J_HH = 6.8 Hz, 12H, CH(CH₃)₂), 1.28 (d, ³J_HH = 6.8 Hz, 36H, CH(CH₃)₂), 3.57 (m, 8H, CH(CH₃)₂), 6.99 (m, 12H, C₆H₃-aryl). ¹³C{¹H} NMR (101 MHz, C₆D₆), δ, ppm: 23.42 (CH(CH₃)₂), 28.74 (C(CH₃)₃), 30.92 (CH(CH₃)₂), 44.63 (C(CH₃)₃), 123.94, 125.31, 128.18, 143.10 (C₆H₃-aryl), 182.69 (d, ²J_YC = 2.0 Hz, N–C–N). C₆₅H₉₄IN₄Y: calcd (%) C 68.05, H 8.26, N 4.88 found (%) C 67.74, H 8.19, N 4.92. UV-vis-NIR ṽ_max/cm⁻¹: 36 200 (ε = 6820 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3340w, 2959m, 2868m, 1615s, 1585m, 1459m, 1431m, 1410m, 1393w, 1329m, 1306w, 1244w, 1201w, 1169w, 1096w, 1040w, 945m, 827w, 700m, 768s, 724s.

[{Gd(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] (2-Gd)

A solution of 1-Gd-I (0.607 g, 0.5 mmol) in benzene (20 mL) was added dropwise to a stirred suspension of KC₈ (0.203 g, 1.5 mmol) in benzene (10 mL) at room temperature. The mixture was allowed to stir at 25 °C for 3 days (reaction mixture gradually changed from colourless to blue and bronze KC₈ slowly converted to black graphite). The reaction mixture was allowed to settle overnight and filtered to another flask. The solvent was removed under vacuum and the residue was extracted by stirring with hexane (25 mL) for 15 min. The solution was filtered and concentrated to ∼5 mL under vacuum, then left at −35 °C to crystallize. Dark blue octahedral crystals of 2-Gd were isolated after several days (0.132 g, 43% based on 1-Gd-I). C₆₄H₉₂N₄Gd₂: Anal. Calcd (%) C 62.40, H 7.53, N 4.55; found (%) C 62.12, H 7.45, N 4.62. UV-vis-NIR ṽ_max/cm⁻¹: 17 120 (ε = 1296 M⁻¹ cm⁻¹), 34 200 (ε = 22 200 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3340w, 2957s, 2868m, 1615s, 1585w, 1462w, 1430w, 1392m, 1382m, 1361m, 1320m, 1243w, 1209w, 1187w, 1096w, 945m, 799w, 767s, 756s, 723w.

[{Tb(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)] (2-Tb)

A solution of 1-Tb-Cl (0.517 g, 0.5 mmol) in benzene (20 mL) was added dropwise to a stirred suspension of KC₈ (0.203 g, 1.5 mmol) in benzene (10 mL) at room temperature. The mixture was allowed to stir at 25 °C for 3 days (reaction mixture gradually changed from colourless to blue and bronze KC₈ slowly converted to black graphite). The reaction mixture was allowed to settle overnight and filtered to another flask. The solvent was removed under vacuum and the residue was extracted by stirring with hexane (25 mL) for 15 min. The solution was filtered and concentrated to ∼5 mL under vacuum, then left at −35 °C to crystallize. Dark blue octahedral crystals of 2-Tb were isolated after several days (0.145 g, 47% based on 1-Tb-Cl). Alternative method: 2-Tb can be also synthesized by the reaction between TbPiso₂ (0.1 g, 0.1 mmol) and KC₈ (0.027 g, 0.2 mmol) in benzene (0.051 g, 83% based on TbPiso₂). ¹H NMR (400 MHz, C₆D₆), δ, ppm: −91.37 (s, 18H, C(CH₃)₃), 1.19 (d, J = 145.3 Hz, 12H, CH(CH₃)₂), 34.99 (s, 8H, CH(CH₃)₂), 52.44 (s, 18H, CH(CH₃)₂), 51.69 (s, 18H, CH(CH₃)₂), 63.67 (s, 6H, C₆H₆⁴⁻), 131.57 (br, 4H, p-C₆H₃-aryl), 250.12 (br, 8H, m-C₆H₃-aryl). ¹³C{¹H} NMR spectra could not be interpreted. C₆₄H₉₂N₄Tb₂: Anal. Calcd (%) C 62.23, H 7.51, N 4.54; found (%) C 61.52, H 7.15, N 4.60. χ_MT product = 23.24 cm³ mol⁻¹ K (Evans method). UV-vis-NIR ṽ_max/cm⁻¹: 17 240 (ε = 8340 M⁻¹ cm⁻¹), 35 590 (ε = 16 280 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3336w, 2957s, 2862m, 1621m, 1582w, 1439s, 1378s, 1353s, 1308w, 1250w, 1199w, 1166m, 1099w, 1020w, 941w, 795m, 759s, 717w.

[{Dy(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)]·C₆H₁₄ (2-Dy)

A solution of 1-Dy-Cl (0.519 g, 0.5 mmol) in benzene (20 mL) was added dropwise to a stirred suspension of KC₈ (0.203 g, 1.5 mmol) in benzene (10 mL) at room temperature. The mixture was allowed to stir at 25 °C for 3 days (reaction mixture gradually changed from colourless to purple and bronze KC₈ slowly converted to black graphite). The reaction mixture was allowed to settle overnight and filtered to another flask. The solvent was removed under vacuum and the residue was extracted by stirring with hexane (25 mL) for 15 min. The solution was filtered and concentrated to ∼5 mL under vacuum, then left at −35 °C to crystallize. Dark purple octahedral crystals of 2-Dy were isolated after several days (0.156 g, 49% based on 1-Dy-Cl). Alternative method: 2-Dy can be also synthesized by the reaction between DyPiso₂ (0.1 g, 0.1 mmol) and KC₈ (0.027 g, 0.2 mmol) in benzene (0.05 g, 79% based on DyPiso₂). ¹H NMR (400 MHz, C₆D₆) spectra could not be interpreted, δ, ppm: 0.85 (m, 40H, C(CH₃)₃), 3.55 (d, J = 70.7 Hz, 2H), 5.57 (s, 1H), 115.19 (s, 7H), 205.02 (s, 10H). ¹³C{¹H} NMR spectra could not be interpreted. C₇₀H₁₀₆N₄Dy₂: Anal. Calcd (%) C 63.20, H 8.04, N 4.22; found (%) C 62.91, H 7.88, N 4.35. χ_MT product = 28.24 cm³ mol⁻¹ K (Evans method). UV-vis-NIR ṽ_max/cm⁻¹: 17 540 (ε = 2180 M⁻¹ cm⁻¹), 35 840 (ε = 13 020 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 2959s, 2866m, 1478s, 1412s, 1341s, 1308m, 1238w, 1199w, 1153s, 1089m, 1038w, 938m, 843w, 783m, 756m, 719s, 653w, 628w, 562w.

[{Y(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₆H₆)]·C₆H₁₄ (2-Y)

A solution of 1-Y-I (0.574 g, 0.5 mmol) in benzene (20 mL) was added dropwise to a stirred suspension of KC₈ (0.203 g, 1.5 mmol) in benzene (10 mL) at room temperature. The mixture was allowed to stir at 25 °C for 3 days (reaction mixture gradually changed from colourless to blue and bronze KC₈ slowly converted to black graphite). The reaction mixture was allowed to settle overnight and filtered to another flask. The solvent was removed under vacuum and the residue was extracted by stirring with hexane (25 mL) for 15 min. The solution was filtered and concentrated to ∼5 mL under vacuum, then left at −35 °C to crystallize. Dark blue octahedral crystals of 2-Y were isolated after several days (0.160 g, 56% based on 1-Y-I). ¹H NMR (400 MHz, C₆D₆), δ, ppm: 1.34 (d, ³J_HH = 6.9 Hz, 48H, CH(CH₃)₂), 1.48 (s, 18H, C(CH₃)₃), 2.05 (s, 6H, C₆H₆⁴⁻), 3.32 (m, 8H, CH(CH₃)₂), 5.88 (t, ¹J_YH = 7.6 Hz, 2H, p-C₆H₃-aryl), 6.73 and 6.91 (d, ³J_HH = 7.6 Hz, 4H, m-C₆H₃-aryl), 6.99 (dt, ³J_HH = 7.7 Hz, ¹J_YH = 4.2 Hz, 2H, p-C₆H₃-aryl). ¹³C{¹H} NMR (101 MHz, C₆D₆), δ, ppm: 13.94, 20.10, 22.38, 23.58, 24.63, 26.31, 28.07, 28.67 (CH(CH₃)₂), 31.75 (C(CH₃)₃), 34.08 (CH(CH₃)₂), 41.95 (C(CH₃)₃), 65.51 (t, ¹J_YC = 5.1 Hz, C₆H₆⁴⁻), 111.61, 115.83, 122.80, 139.51 (C₆H₃-aryl), 123.77, 142.66, 145.12, 157.09 (Y–C₆H₃-aryl), 172.29 (N–C–N). C₇₀H₁₀₆N₄Y₂: Anal. Calcd (%) C 71.16, H 9.04, N 4.74; found (%) C 70.96, H 9.34, N 4.65. UV-vis-NIR ṽ_max/cm⁻¹: 17 240 (ε = 11 500 M⁻¹ cm⁻¹), 35 970 (ε = 21 400 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3340w, 2959m, 2868m, 1616s, 1585m, 1461m, 1431m, 1411m, 1394w, 1361w, 1341m, 1330m, 1307w, 1243w, 1201w, 1096w, 1040w, 946w, 932m, 827w, 800m, 767s, 716w.

[{Tb(κ¹:η⁶-Piso)}₂(μ-η⁶:η⁶-C₇H₈)]·2C₅H₁₂ (3-Tb)

2-Tb (0.050 g, 0.04 mmol) was dissolved in toluene (10 mL) and then stirred for 24 hours at room temperature to give a blue solution. The solvent was removed under vacuum and the residue was extracted by stirring with pentane (25 mL) for 15 min. The solution was filtered and concentrated to ∼2 mL under vacuum, then left at −35 °C to crystallize. Dark blue crystals of 3-Tb were isolated after several days (0.041 g, 74% based on 2-Tb). Alternative method: 3-Tb can be also independently synthesized by stirring 1-Tb-Cl (0.103 g, 0.1 mmol) and KC₈ (0.04 g, 0.3 mmol) in toluene for 3 days, as determined by the formation of a dark blue reaction mixture. C₇₄H₁₁₈N₄Tb₂: Anal. Calcd (%) C 64.33, H 8.61, N 4.05; found (%) C 63.54, H 8.47, N 4.02. UV-vis-NIR ṽ_max/cm⁻¹: 16 670 (ε = 970 M⁻¹ cm⁻¹), 36 100 (ε = 9100 M⁻¹ cm⁻¹). ATR-IR (microcrystalline, cm⁻¹): 3339w, 2953m, 2870m, 1615s, 1584m, 1411w, 1394w, 1330m, 1307w, 1244w, 1201w, 1170m, 1158m, 1096w, 1054w, 1040w, 932m, 882w, 801m, 768s, 715w.

Arene exchange reactions of 2-Y

Separate mixtures of 2-Y (23 mg, 0.02 mmol) and or biphenyl (3 mg, 0.02 mmol) or naphthalene (2.6 mg, 0.02 mmol) or anthracene (3.6 mg, 0.02 mmol) were dissolved in C₆D₆ (ca. 0.6 mL) and the dark blue solution was transferred to a J. Young NMR tube. Arene exchange reactions were also conducted between 2-Y (12 mg, 0.01 mmol) and C₆D₆ (0.6 mL) or C₇D₈ (0.6 mL). The arene exchange reactions were monitored by ¹H NMR spectroscopy at 80 °C over the course of 100–150 h. There was no exchange when C₆D₆ solutions of 2-Y were separately treated with biphenyl, naphthalene or anthracene after 150 h (Fig. S15–S18†), and no arene exchange was observed between 2-Y and C₆D₆ or C₇D₈, in spite of some minor decomposition (Fig. S19†). The reaction between 2-Y (12 mg, 0.01 mmol) and an excess of toluene (5 mL) was also performed in an Rotaflo tap-appended ampoule. The reaction mixture was allowed to stir for 12 hours at room temperature, then toluene was removed under vacuum and the residue was dissolved in 0.6 mL C₆D₆ for ¹H and ¹³C{¹H} NMR spectroscopy, where the arene exchange was shown to have occurred with a signal corresponding to (C₇H₈)⁴⁻ detected (Fig. S13 and S14†). Alternative method: 3-Y can also be independently synthesized by stirring 1-Y-I (0.11 g, 0.1 mmol) and KC₈ (0.04 g, 0.3 mmol) in toluene for 3 days, as determined by the formation of a dark blue reaction mixture.

Reactions of 2-Y with COT

Two separate reactions were conducted between 2-Y and COT with molar ratios of 1 : 2 and 1 : 4. C₆D₆ (0.3 mL) solutions of COT (2 mg, 0.02 mmol; or 4 mg, 0.04 mmol) were separately added dropwise to two C₆D₆ (0.3 mL) solutions of 2-Y (12 mg, 0.01 mmol) in J. Young NMR tubes. The reaction mixtures were agitated by hand and the dark blue reaction mixtures changed colour to pale yellow within 5 min. For the 1 : 2 reaction: ¹H NMR (400 MHz, C₆D₆), δ, ppm: 0.62 (s, 9H, C(CH₃)₃), 1.20 (d, ³J_HH = 6.67 Hz, 12H, CH(CH₃)₂), 1.32 (d, ³J_HH = 6.83 Hz, 12H, CH(CH₃)₂), 2.88 (m, 4H, CH(CH₃)₂), 5.63 (s, C₈H₁₀, COT), 6.60 (s, 8H, C₈H₈, COT²⁻), 7.00 (m, 6H, C₆H₃-aryl); For 1 : 4 reaction: ¹H NMR (400 MHz, C₆D₆), δ, ppm: 0.61 (s, 9H, C(CH₃)₃), 1.21 (d, ³J_HH = 6.77 Hz, 12H, CH(CH₃)₂), 1.33 (d, ³J_HH = 6.84 Hz, 12H, CH(CH₃)₂), 2.88 (m, 4H, CH(CH₃)₂), 5.63 (s, 10H, C₈H₁₀, COT), 6.60 (s, 8H, C₈H₈, COT²⁻), 6.99 (m, 6H, C₆H₃-aryl).

Data availability

NMR spectra, X-ray crystallographic data, UV-vis-NIR and IR spectra, magnetic data, EPR spectroscopy and DFT calculation details are documented in the ESI.† CCDC 2402307 (1-Gd-I), 2370381 (1-Tb-Cl), 2370379 (1-Dy-Cl), 2370380 (1-Y-I), 2402308 (2-Gd), 2370378 (2-Tb), 2370382 (2-Dy), 2370383 (2-Y) and 2370384 (3-Tb) contain the ESI† crystallographic data for this paper. These data can be obtained free of charge viahttps://www.ccdc.cam.ac.uk/data_request/cif, or by emailing data_request@ccdc.cam.ac.uk, or by contacting The Cambridge Crystallographic Data Centre, 12 Union Road, Cambridge, CB2 1EZ, UK; fax: +44 1223 336033.

Author contributions

P.-B. J. synthesized and characterized the complexes. P.-B. J. collected, solved, and refined the single crystal XRD data. G. K. G. and P.-B. J. performed SQUID magnetometry measurements. P.-B. J. and Q.-C. L. interpreted the magnetic data. Q.-C. L. performed the theoretical calculations and interpretation. R. E. P. W., D. P. M. and Y.-Z. Z. supervised the study. P.-B. J., Q.-C. L. and D. P. M. wrote the manuscript with input from all the authors.

Conflicts of interest

The authors declare no competing interests.