Abstract
Objectives
To compare the stress distribution and crack propagation in cracked mandibular first molar restored with onlay, overlay, and two types of occlusal veneers using two different CAD/CAM materials by Finite Element Analysis (FEA).
Materials and methods
A mandibular first molar was digitized using a micro CT scanning system in 2023. Three-dimensional dynamic scan data were transformed, and a 3D model of a cracked tooth was generated. Finite element models of four different models (onlay, overlay, and two types of occlusal veneer restored teeth) were designed. Two different CAD/CAM materials, including Lava Ultimate (LU) and IPS e.max CAD (EMX), were specified for both models. Each model was subjected to three different force loads on the occlusal surfaces. Stress distribution patterns and the maximum von Mises (VM) stresses were calculated and compared.
Results
Compared to the base model, all restorations showed that high-stress concentration moved from the lower margin of the crack area towards the top of the crack area. The EMX-restored onlay, overlay, and occlusal veneer 2 had the lower stress in the cracked area and the lower average von Mises stress levels at the lower margin along the cracked line, especially under the 225N lateral force (P < 0.05). The occlusal veneer 1 filled with resin had a poorer stress distribution and higher stress concentration of stress at the remaining crack than the occlusal veneer 2 without resin filled inside.
Conclusions
The EMX restorations with onlay, overlay, and occlusal veneer 2 showed lower stress concentration at the lower margin of crack surface compared to the LU-restored models. The occlusal veneer with internal resin filler exhibited higher stress on the end of the lower margin of the crack surface.
Clinical relevance
Our results suggest that onlay, overlay ceramic restorations and occlusal veneer (without resin filling inside) may be a favorable method to prevent further crack propagation.
Trial registration
A protocol was specified and registered with the Chinese Clinical Trial Registry (ChiCTR) on 2022–04-12 (registration number: ChiCTR2200058630).
Keywords: Finite element analysis, Cracked tooth, Restoration design, Occlusal veneer, Mechanical properties
Introduction
An incomplete tooth fracture, or “cracked tooth,” is “a thin fracture plane of unknown depth, originating from the crown, extending through the tooth structure, and may extend sub-gingivally, and possibly continue to develop a connection to the pulpal space and/or periodontal ligament” [1, 2]. Cracked teeth are a prevalent problem, with nearly 70% of dental patients having at least one cracked posterior tooth, of which 21% have symptoms [3]. The most common types of posterior teeth are mandibular molars, maxillary molars, and maxillary premolars [4, 5]. In the earlier stage, a cracked tooth can lead to sharp pain during the bite, cold irritation pain, or deep periodontal pockets associated with cracks [6, 7]. Due to the variety of symptoms, diagnosing a cracked tooth is not simple. There is no effective clinical method to predict crack extension, although there are some applications, such as dye tests, transillumination, or microscopic detection [7, 8]. Early diagnosis of cracked teeth is essential as it relates to the treatment selection and long-term prognosis of cracked teeth [9].
Treatment options for cracked teeth are complex. They depend on the sites, directions, degree of the cracks, and symptoms. The prognosis depends on three factors: the extent of the crack, the choice of coronal restoration, and the time of initiating treatment [10]. In previous studies, if the crack did not involve the pulp, it could be conventionally restored using composite restorations [11, 12] or external splints such as crowns [13] and overlays [14]. If the crack penetrates the dental pulp, even with root canal treatment, it is difficult to remove the crack line altogether, complicating the prognosis. Therefore, early detection and timely treatment of cracked teeth are crucial to maintain pulp vitality and prevent or delay the further expansion of cracked teeth [13].
Treating cracked teeth remains very challenging in daily practice. The efficacy of treating cracked teeth is primarily based on clinical observations. It is impossible to directly compare the treatments used in different studies due to differences in criteria, sample sizes, and treatment methods. As a result, there are differences among general dentists, prosthodontists, and endodontists in the treatment of fissured teeth [15]. In addition, the researchers did not explain the dynamic mechanisms that inhibit crack expansion when proposing treatment protocols. Niek J M Opdam et al. performed direct composite resin restoration on 41 patients with cracked teeth. They found that the group with cuspal coverage had no failure records, and 94% of the teeth without cuspal coverage retained pulp vitality after seven years. They believe that direct bonding composite resin restorations may be a good choice for treating painful cracked teeth [11]. However, some researchers have recommended the use of cusp-protected indirect restorations. Antonio Signore et al. found that painful cracked teeth treated with bonded indirect resin composite onlays were free of symptoms and vital, and the 6-year survival rate of over 90% [16]. According to Krell and Rivera's research results, a full crown appears less effective in maintaining pulp vitality. Of 127 symptomatic cracked teeth, 20% required pulp treatment within six months [6]. During crown placement, a large amount of tooth tissue must be removed, which may result in pulp damage and require corresponding root canal treatment. From the above research results, it can also be seen that there is currently no recognized early intervention treatment method for early cracked teeth.
Occlusal veneers are thin coverings that can cover the entire cusp due to their strength and ability to stabilize the cusp, serving as an option for cracked teeth [17, 18]. More and more studies use occlusal veneers to treat severe tooth wear and reestablish the occlusal vertical dimension [19, 20]. Researchers have used occlusal veneers for the treatment of early cracked teeth, but no clinical studies have been reported so far. Therefore, we envisioned whether they could be validated in vitro by the 3D finite element method [21].
Three-dimensional finite element analysis (FEA) is an established and non-invasive method for simulating the teeth and evaluating the mechanical forces on teeth and restorative materials [22–25]. Some researchers have explored the correlation between oral functional behaviors, tooth factors, and cracked teeth and found that eating hard food, occlusal restoration, and cuspal inclination were associated with posterior cracked teeth [2]. Some studies have explored the stress distribution in the restorations of cracked teeth with fiber-reinforced composites and onlays [26]. However, to our knowledge, no studies have reported the distribution of occlusal stress when onlays, overlays, and occlusal veneers are used to repair vital cracked teeth. The treatment of cracked teeth aims to prevent masticatory force from concentrating in the cracked area, and finite element analysis is a valuable method for mechanically evaluating the adequacy of cracked tooth treatment and predicting the prognosis.
This experiment employed three-dimensional finite element analysis to study the stress distribution of vital cracked teeth and crack propagation before and after onlay, overlay, and two types of occlusal veneer restorations. It will provide a biomechanical theoretical reference for selecting a restoration method for cracked teeth with living pulp.
Materials and methods
Numerical modelling by FEA
This study was approved by the ethics committee of Nanjing Stomatological Hospital, Affliated Hospital of Medical School, Institute of Stomatology, Nanjing University (No. NJSH-2023NL-014–1). Three-dimensional (3D) FE models were generated from a freshly extracted sound human mandibular first molar in this study. A three-dimensional CAD model of the first mandibular molar was created by using a micro-computed tomography (micro CT) scanning system (NewTom 5G, Quantitative Radiology, Verona, Italy) and SolidWorks 2017 software (SolidWorks, 2017; Dassault Systèmes) for generating dentin, pulp, and enamel. The scanned dataset was converted to the Standard Tessellation Language (STL) file format. To evaluate authenticity, these files were loaded into reverse engineering software (Geomagic Studio, 2013; Rainbow et al., USA). Accurate geometries were obtained by removing nails and unwanted features from the model and then optimizing and smoothing the model.
The STP geometric model file saved in the Geomagic Studio 2013 software was opened in SolidWorks 2017 software (Dassault Systems SolidWorks Corp., Waltham, MA). Following the software prompts, the geometric model was characterized, curve diagnostics were performed, and surfaces were repaired and saved. Enamel and pulp were calculated to obtain a model of the tooth. Regarding the crown, the buccolingual and mesiodistal diameters were 10.1 and 11.9 mm respectively, and cervico-occlusal length was 7.8 mm.
A cracked tooth model was simulated using the surface stretch and resection commands by SolidWorks 2017 software. The experimental assumption was that the central single-ended crack was located near the central fossa, crossed the distal marginal ridge, and was deep enough to reach below the enamel-dentin junction, and the crack was 100 μm at its widest point. We used a solid modeling approach to create the crack. Finally, the file was saved, and the file format is saved as the SLDPRT part. Figure 1 shows the cracked tooth model used in this study.
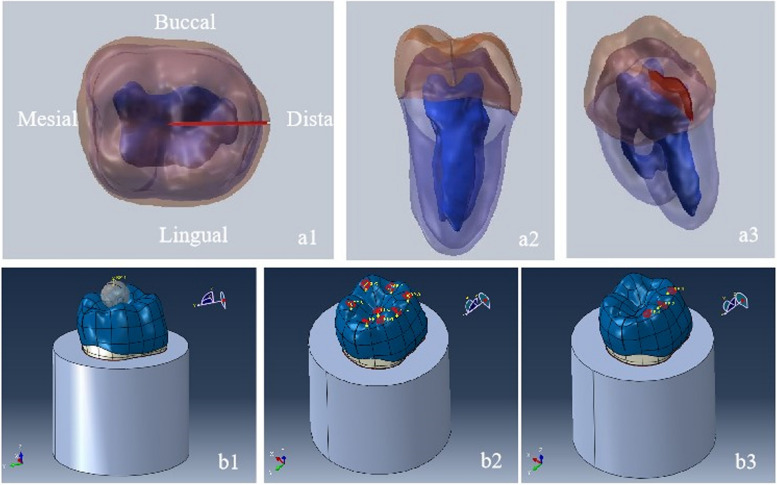
Fig. 1.
The 3D tooth model of crack formation and force load. a1-a3 The model of the cracked teeth; b1 Ball-shaped occlusal load of 800 N was applied to the central groove area of the model. (b2) A total force of 600 N was applied to 8 points. b3 A force of 225 N was applied to the buccal plane of the buccal cusp at 45° to the longitudinal axis of the model
Construction of the restoration models
In order to combine the datum and surface of the established cracked tooth model, sketching is carried out using commands such as segmentation, Boolean operation, rounded corners, excision, and lofting, etc. Finally, solidification is carried out through the surface excision operation. By performing design operations on the current model, a restoration model of the mandibular first molar can be obtained, which contains four restoration types (Fig. 2).
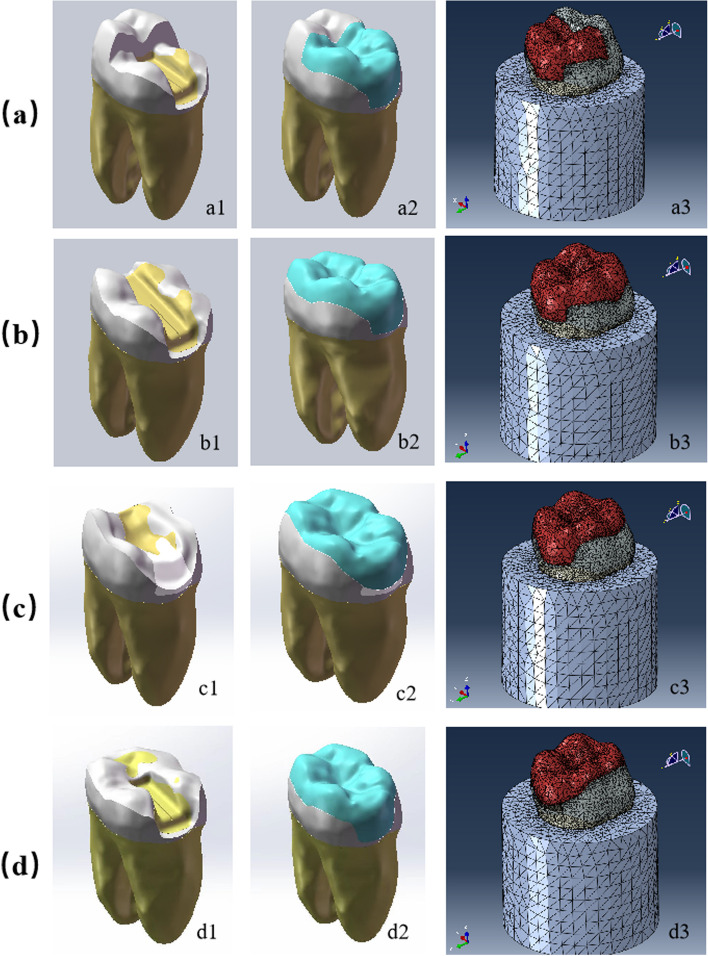
Fig. 2.
Various restorative designs. a Onlay form; b Overlay form; c Occlusal veneer 1 form; d Occlusal veneer 2 form
A form (Onlay): Regarding the onlay form, the inlay form was designed with a 2.0 mm occlusal reduction, a 2.0 mm buccal reduction, and a 1.5 mm reduction for the distobuccal and distolingual cusps. The overall preparation angle towards the occlusal surface is 6°, and all the edges of the cave wall and the inner line corner are rounded. The yellow part is a cavity-shaped adhesive coated inside, and the split high inlay restoration is assembled into the defect area to obtain it (Fig. 2a).
B form (Overlay): Similar to onlays, the inlay form of the overlay was designed with a 2.0 mm uniformly occlusal reduction based on its external curvature (2 mm on buccal cups, 1.5 mm on lingual cusp) (Fig. 2b).
C1 form (Occlusal veneer 1): Firstly, the model was filled with flowable resin composite after the cracks were ground out, and then the occlusal surface was uniformly ground out by 1 mm and prepared to wrap around the shaft wall with a 1.5 mm height, a 1 mm shoulder width, and a 6° shaft surface convergence. And the butt joints were prepared according to the inclination of the occlusal surface (Fig. 2c).
C2 form (Occlusal veneer 2): Similar to the overlay, the occlusal surfaces were uniformly ground out by 1 mm, and the axial walls were prepared to be wrapped; the axial wall height is 1.5 mm (Fig. 2d).
Table 1 lists the number of nodes and elements in different zones of the model.
Table 1.
Restoration design and materials of experimental eight models
| Model | Restoration design | Adhesive layer | Nodes | Elements |
|---|---|---|---|---|
| Base | 79,528 | 369,743 | ||
| A form | Onlay | 3 M RelyX Unicem | 121,024 | 519,850 |
| B form | Overlay | 3 M RelyX Unicem | 141,216 | 601,849 |
| C1 form | Occlusal veneer 1 | Dental Resin Luting | 169,757 | 734,233 |
| C2 form | Occlusal veneer 2 | 3 M RelyX Unicem | 140,030 | 591,263 |
Elastic properties of the materials
The geometric model was imported into the Abaqus software (SIMULIA, Dassault Systèmes, Johnston, RI, USA). In the property module, the material property parameters of enamel, dentin, pulp, periodontal ligament, and alveolar bone were established respectively and assigned to the corresponding components. The offset surface in the SolidWorks software was utilized for surface trimming and thickening to obtain the cement layers of 3 M RelyX Unicem (with a thickness of 120 µm) and Dental Resin Luting Materials (with a thickness of 20 µm). For model A form (onlay), model B form (overlay), and model C2 form (occlusal veneer 2), the porcelain restoration is directly bonded to dentin, and the bonding layer is a 3 M RelyX Unicem cement layer with a thickness of 120 µm. For model C, after the cracks are ground out, the model is filled with flowable resin composite. The resin and the dentin at the bottom of the cavity are bonded by a dental resin luting material with a thickness of 20 µm. The bonding layer between the resin restoration and the porcelain restoration is also a layer with a thickness of 120 µm.
In the Assembly module, the imported model components were assembled. In the Step module, the Static-General analysis type was established. Then, in the Interaction module, the contact of each component of the model was defined as a Tie connection, that is, binding contact (Fig. 1). The teeth and materials of the different zones of the tooth assembly were assumed to be linearly elastic, isotropic, and homogeneous [25, 26]. Table 2 lists the material values (modulus of elasticity and Poisson's ratio) from the relevant literature we have referenced. The bottom of the alveolar bone was fixed, and loads of 800 N, 600 N, and 225 N were respectively applied at the relevant positions of the molar enamel as mentioned later to simulate the states of masticatory force, maximum chewing force and lateral chewing.
Table 2.
The mechanical properties of the investigated materials
| Material | MOE(MPa) | Poisson’s ratio (μm) | Thickness (μm) |
|---|---|---|---|
| Enamel [27] | 84,100 | 0.30 | |
| Dentin [27] | 18,600 | 0.31 | |
| Bone [28] | 13,700 | 0.30 | |
|
Pulp [29] Parodontium |
2.07 68.9 |
0.45 0.45 |
|
|
Lava Ultimate [30] IPS e.max CAD (EMX) [31] |
12,770 95,000 |
0.30 0.25 |
|
| Flowable resin composite (SDR) [32] | 8000 | 0.20 | |
| 3 M RelyX Unicem [33] | 5000 | 0.27 | 100 |
|
Dental Resin Luting Materials [27] Steel ball |
8300 200,000 |
0.35 0.3 |
20 |
Loading process
To simulate the mandibular first molar during masticatory movements, three loads were applied to the cracked teeth model, as shown in Fig. 1. The loading process is as follows:
For the analysis of masticatory force, a stainless steel ball with a radius of 2 mm was prepared to apply a force of 800 N. The contact between the steel ball and the occlusal surface had a frictional coefficient of 0.3 [34] (Fig. 1b1).
To simulate the maximum chewing force, a vertical pressure load of 600 N, with 75 N at each loading point, was applied to 8 different areas on the occlusal surface. The force was respectively loaded on the inner inclined lingual cusp, slightly to the outer side of the top of the mesiodistal buccal cusp, at the central fossa, and on the inner side of the mesiodistal marginal ridge [35, 36] ( Fig. 1b2).
A total force of 225 N was applied to the buccal plane of the buccal cusp at a 45° angle to the longitudinal axis of the teeth, and a total of 3 points (75 N each) were allocated to simulate the lateral chewing load [35] [36] (Fig. 1b3).
Statistical analysis
An analysis was carried out regarding the stress distribution of the occlusal surface, the bottom surface of the restoration, and the longitudinal section of the mesial teeth of all models. The von Mises stress values at the lower margin of the crack surface were measured at 15 points in each model (Figs. 8 and 9). Paired t-tests and Bonferroni corrections were employed to statistically compare the dental models using SPSS 23.0 software (SPSS Inc., Chicago, Illinois). A P value less than 0.05 was considered statistically significant.
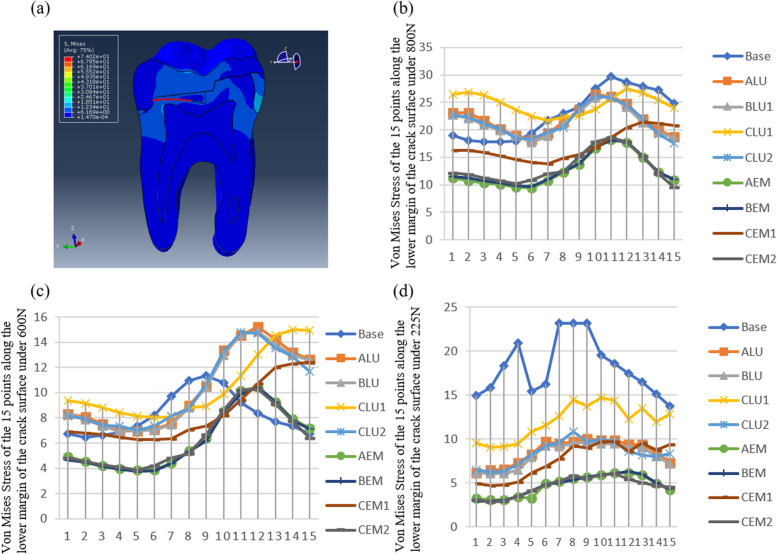
Fig. 8.
The maximum concentrated von Mises stress value at 15 nodes along the lower margin of the crack surface under 800 N, 600 N and 225 N. a the 15 points of stress measurement along the lower margin of the crack surface; b Von Mises stress of the 15 points long the lower margin of the crack surface under 800 N; c Von Mises stress of the 15 points long the lower margin of the crack surface under 600 N; d Von Mises stress of the 15 points long the lower margin of the crack surface under 225 N
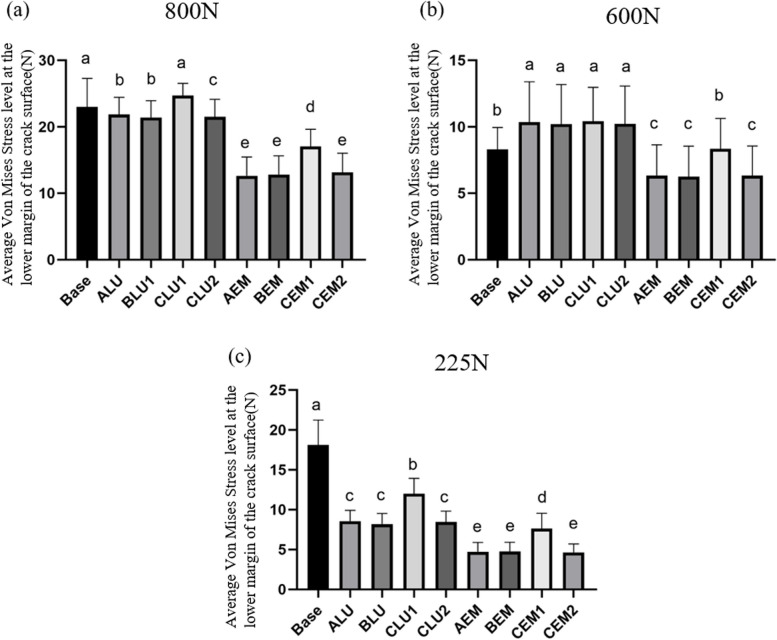
Fig. 9.
The average von Mises stress level at the lower margin of the crack surface under 800 N, 600 N and 225 N (P < 0.05)
Results
Stress patterns and distribution of occlusal surface, dentin and restoration surface
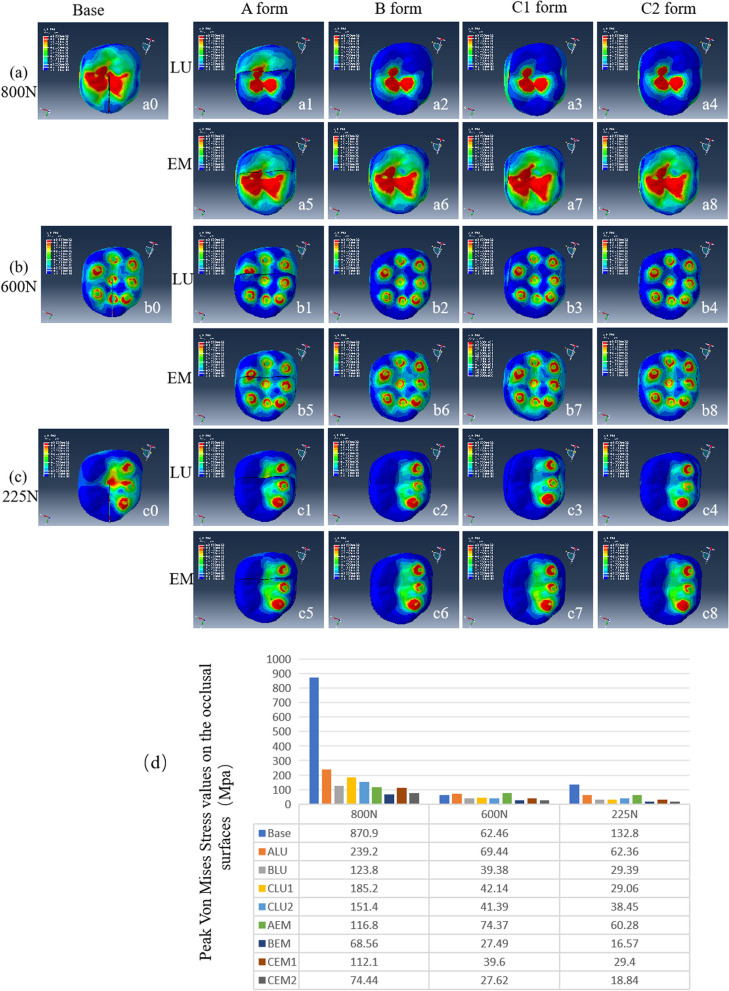
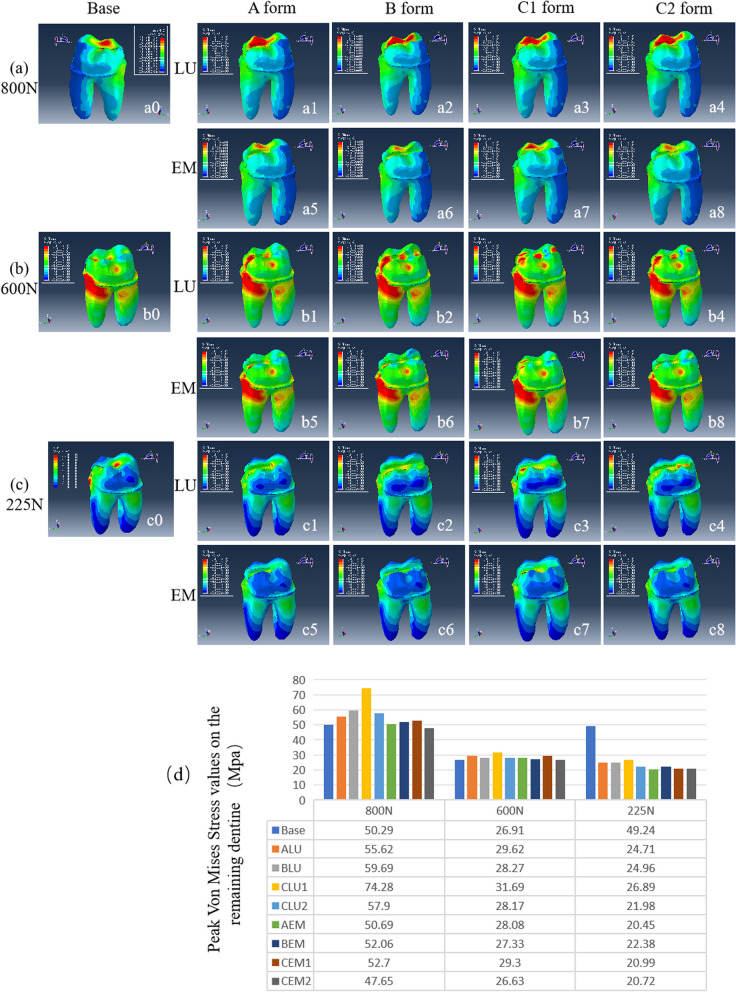
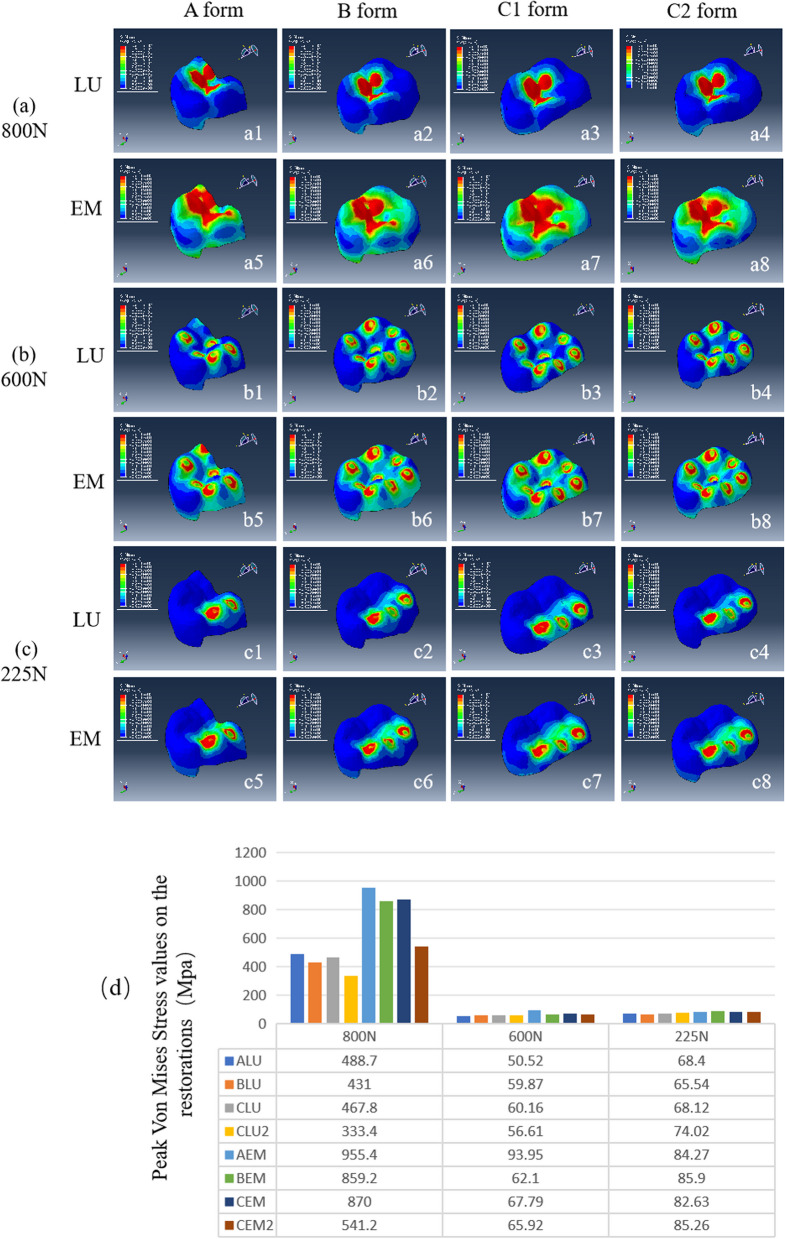
Figure 3 shows a horizontal view of the mechanical behavior of the occlusal surface of a cracked tooth. These images indicate that the stress concentration and absorption modes under load vary significantly depending on the material and restoration design. The choice of repair material also affects the distribution and magnitude of stresses when the same repair method is applied under forces of 800 N, 600 N, and 225 N (Fig. 3d). Overlay and Occlusal veneer 2 covering the occlusal surfaces with IPS e.max CAD (EMX) showed the minimal stress values under the three loads. The results showed that Lava Ultimate restorations under 800 N vertical stress all had higher dentin stress than the control group, and only the occlusal veneer 2 group with IPS e.max CAD (EMX) was lower than the control group. The distribution of Von Mises stresses on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) was reduced under a total multipoint lateral force of 225 N (Fig. 4). Figure 5 shows the stress distribution of the restoration surface, and it was found that restorations with IPS e.max CAD (EMX) showed higher Peak Von Mises Stress values than Lava Ultimate restorations under 800 N. However, there was no significant difference between the groups for force loading of 600 N and 225 N (Fig. 5).
Fig. 3.
Stress patterns and distribution in a cracked tooth according to various treatment modalities of occlusal surface. (a1-a4) (b1-b4) (c1-c4) the porcelain material is Lava Ultimate; (a5-a8) (b5-b8) (c5-c8) the porcelain material is IPS e.max CAD (EMX); (a1-a8) The distribution of VM stress on the occlusal surfaces of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total vertical force of 800 N; (b1–b8) The distribution of VM stress on the occlusal surfaces of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) A total force of 600 N with direction aligned vertically along the tooth axis was applied to 8 different points; (c1–c8) The distribution of VM stress on the occlusal surfaces of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total multipoint lateral force of 225 N; d The maximum von Mises stress values (MPa) on the occlusal surface
Fig. 4.
Stress patterns and distribution in a cracked tooth according to various treatment modalities of dentine. (a1-a4) (b1-b4) (c1-c4) the porcelain material is Lava Ultimate; (a5-a8) (b5-b8) (c5-c8) the porcelain material is IPS e.max CAD (EMX); (a1-a8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total vertical force of 800 N; (b1–b8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) A total force of 600 N with direction aligned vertically along the tooth axis was applied to 8 different points; (c1–c8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total multipoint lateral force of 225 N; d The maximum von Mises stress values (MPa) of the dentin
Fig. 5.
Stress patterns and distribution of the restorations in a cracked tooth according to various treatment modalities. (a1-a4) (b1-b4) (c1-c4) the porcelain material is Lava Ultimate; (a5-a8) (b5-b8) (c5-c8) the porcelain material is IPS e.max CAD (EMX); (a1-a8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total vertical force of 800 N; (b1–b8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) A total force of 600 N with direction aligned vertically along the tooth axis was applied to 8 different points; (c1–c8) The distribution of VM stress along the cracked line of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total multipoint lateral force of 225 N; d The maximum von Mises stress values (MPa) of various restorations
Stress distribution and measurement along the bottom of the crack line
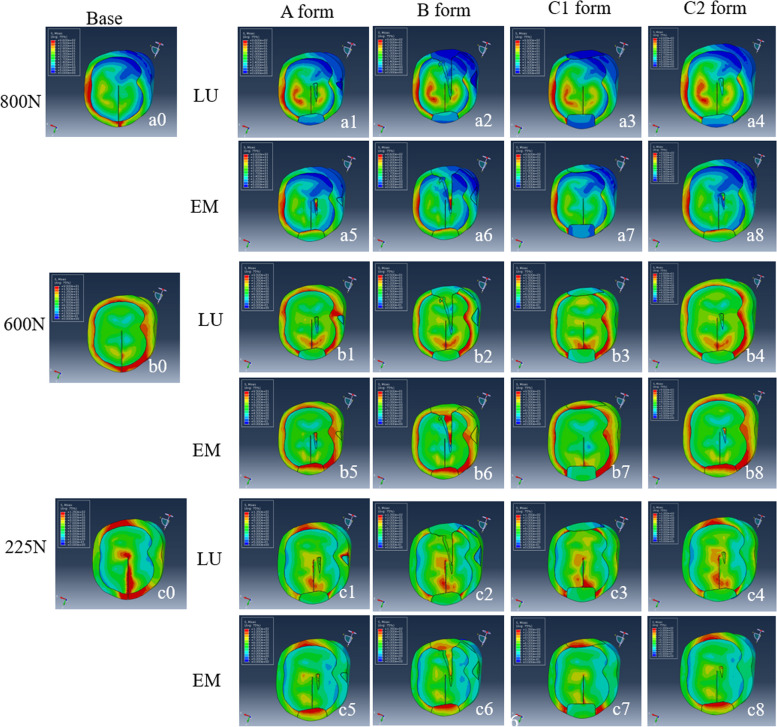
Figure 6 shows the horizontal view of the stress distributions along the bottom of the crack line. It is pronounced that with Lava Ultimate, the stresses at the cracks are rather more aggregated under a total force of 600 N, with IPS e.max CAD (EMX), the stresses at the cracks become less noticeable, especially for Fig. 6 (b5, b6, b8). Under a total multi-point lateral force of 225 N, the stresses are mainly concentrated at the crack and near the edge ridge in the base group. Regardless of the restorative materials and designs, the stress value at the crack line all decreased. Still, it was more evident in the IPS e.max CAD (EMX) material group (Fig. 6 c5-c8), especially in c5, c6, and c8 where the stress concentration at the crack was no longer visible.
Fig. 6.
Stress patterns and distribution of the bottom level of the restoration in a cracked tooth according to various treatment modalities. (a1-a4) (b1-b4) (c1-c4)the porcelain material is Lava Ultimate; (a5-a8)(b5-b8)(c5-c8) the porcelain material is IPS e.max CAD (EMX); (a1-a8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total vertical force of 800 N; (b1–b8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) A total force of 600 N with direction aligned vertically along the tooth axis was applied to 8 different points; (c1–c8) The distribution of VM stress along the cracked line of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total multipoint lateral force of 225 N
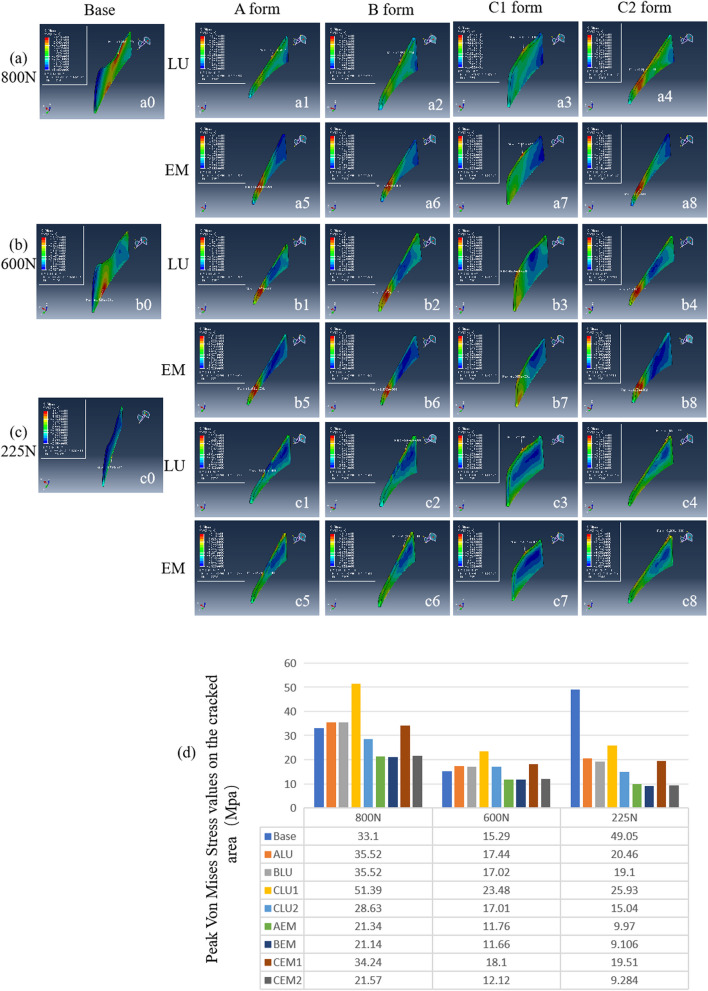
Whether the stress at the hidden crack can be reduced after repair is crucial. Figure 7 shows the stress patterns along the crack surface after various treatments. In the base model, at 600 N and 225 N, the stress was concentrated entirely at the bottom of the crack. With restoration, the stress value moves up to the upper part of the crack. After comparing the maximum stress values of the groups after restoration, it was found that the onlay, overlay, and occlusal veneer 2 using IPS e.max CAD (EMX) were the lowest.
Fig. 7.
Stress patterns and distribution in the crack area in a cracked tooth according to various treatment modalities. (a1-a4) (b1-b4) (c1-c4) the porcelain material is Lava Ultimate; (a5-a8) (b5-b8) (c5-c8) the porcelain material is IPS e.max CAD (EMX); (a1-a8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total vertical force of 800 N; (b1–b8) The distribution of VM stress on the remaining dentine of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) A total force of 600 N with direction aligned vertically along the tooth axis was applied to 8 different points; (c1–c8) The distribution of VM stress in the crack area of the models (ALU, BLU, CLU, CLU 2, AEM, BEM, CEM, CEM 2) under a total multipoint lateral force of 225 N; d The maximum von Mises stress values (MPa) of the crack area
In addition, to detect changes in stress at the bottom of cracks, we measured the von Mises stress levels at 15 nodes with lower remaining crack lines in the basic model and after restorations (Fig. 8). Under the action of forces of 800 N and 600 N, the concentrated stress level at node 1 was the lowest, gradually increasing towards node 11 and then decreasing. Under 225 N lateral force, it was highest at nodes 7, 8, and 9, and the value steadily reduced. At 800N, there was no significant difference in any of the repair modes of Lava Ultimate compared to the primary mode. However, when repaired with IPS e.max CAD (EMX) of the same repair design, the Von Mises stress levels decreased significantly. The Von Mises stress level from node 1 to node 11 decreased significantly when repaired with IPS e.max CAD (EMX) under 600N force, but the Von Mises stress level from node 12 to node 15 was higher than that of the base model. It was more evident that the von Mises stress values decreased significantly in all models when subjected to 225 lateral forces, more significantly in the case of onlays, overlays, and occlusal veneer 2. Among the various treatment models, the occlusal veneer 1 of Lava Ultimate (CLU 1) showed the highest stress levels at most measurement nodes. At node 15, IPS e.max CAD (EMX) inlay (AEM), overlay (BEM), and occlusal veneer 2 (CEM 2) showed the lowest stress levels.
Figure 9 shows the average stress level at the lower margin of the remaining crack surface. The stress level was significantly different between Lava Ultimate (ALU/BLU/CLU/CLU 2) and IPS e.max CAD (EMX) (AEM/BEM/CEM/CEM 2) under the three Loading Processes (P < 0.05). Among the three stress loading modes, AEM, BEM, and CEM 2 had the lowest stress values (P < 0.05) (Fig. 9).
Discussion
The diversity of treatment options and the uncertainty of the prognosis of the affected cracked tooth make it challenging for clinical dentists. Delayed treatment may lead to crack propagation, bacterial invasion, and pulp infection, ultimately leading to severe pulp and periapical diseases, which become the main causes of tooth loss. However, the early symptoms of a cracked tooth are not obvious and are easily confused with other diseases. It is difficult for dentists to diagnose and determine the degree of crack progression [37]. One of the goals of treatment is to immobilize cracked tooth fragments that move during the loading process. This can be accomplished by covering the cusps. Cusp coverage is equivalent to overlapping the cusp of a cracked tooth, and indirect restoration can protect and strengthen the remaining tooth structure. Indirect restorations of cracked teeth usually include inlay, overlay, and full or partial crown restoration [38]. The study on the treatment decisions for cracked teeth found that the treatment options chosen vary greatly, especially for asymptomatic cracked teeth [39]. Therefore, the treatment method for early cracked teeth is challenging.
With the development of adhesive bonding techniques in recent years, minimally invasive dentistry has become a hot topic in clinical restorative dentistry research. Previous studies have shown that molars restored with occlusal veneers exhibit satisfactory mechanical properties, which supports the use of non-retentive occlusal veneers to treat occlusal abrasion and erosion [19, 40]. However, there is no direct comparison of the outcomes of the onlay, overlay, and occlusal veneers, and there is a need fora consensus on the optimal materials and designs to prevent the expansion of residual crack propagation. Therefore, we used three-dimensional finite element analysis to compare the crack line and residual dentin stress pattern before and after treatment with various materials and designs in the same cracked tooth model. Our study examined two materials and four preparatory designs for mandibular first molar cracked teeth, including onlay, overlay, and two occlusal veneers. Regarding restoration materials, our analysis included MOE, Poisson’s ratio, and layer thickness, with MOE being the parameter that has the most significant impact on the results.
In the results, we found that materials have a more significant impact on the stress of dentin and cracks, and different preparation methods for the same veneer also affect the stress. Regarding repair methods, the stress values on the tooth surface of the overlay and the occlusal veneer 2 are relatively low. Under lateral force, there was no significant difference between the two groups, and the IPS e.max CAD (EMX) restoration was slightly smaller. The maximum internal stress results of the restoration indicate that under the action of 800 N force, the internal stress of the IPS restoration is higher than that of Lava Ultimate. If the MOE value of the restoration is lower than that of dentin or enamel, it will be more easily distorted by occlusal force and can then increase the horizontal vector. The increased horizontal vector will result in stress concentration at the lower edge of the remaining crack surface. After restorations with higher MOE, the deformation of the restoration is relatively small, which may reduce the stress in the buccal and lingual directions.
The primary purpose of cracked teeth restoration is to prevent the crack line from further penetrating the proximal root and spreading to the pulp. The most critical aspect is the stress value of the crack line. We evaluated the residual stress values in the crack area after various treatments to evaluate the possibility of crack propagation. The results indicate that IPS e.max CAD (EMX) onlays, overlays, and occlusal veneers 2 were better treatments for reducing stress concentrations in the remaining cracked areas. Compared with Lava Ultimate, IPS e.max CAD (EMX) restoration showed a lower stress value on the remaining crack. The results indicate that IPS e.max CAD (EMX) overlay, overlay, and occlusal veneer 2 are better treatment methods to reduce the remaining crack area stress concentration. Compared to Lava Ultimate, IPS e.max CAD (EMX) showed a lower stress values at the remaining cracks. This result may be due to the stiff ceramic material with high MOE firmly splinting the cracks and preventing horizontal separation of crack lines. However, high stress is transmitted vertically at the bottom of the restorations. In the case of occlusal veneer filled with resin internally, the highest average stress was found at the lower edge of the crack. Therefore, when using occlusal veneer to treat cracked teeth, removing cracks and avoiding resin fillers is necessary to minimize stress concentration in the remaining crack lines.
A previous study demonstrated that when marginal ridge cracks are detected early in teeth diagnosed with reversible pulpitis and crowns are placed, approximately 20% of cases require root canal treatment within six months [6], and crown restoration is not a conservative method to avoid root canal treatment for cracked teeth [26]. Therefore, finding restorations that can reduce stress at the crack line and minimize tooth preparation is significant. Research has found that when external stress is applied to the material, high MOE materials transfer stress to the other side due to their hardness and non-absorbability. However, low MOE materials can absorb stress and deform the material [41]. The practice-based study revealed that low MOE resin-based restoration may have a stress-absorbing effect, such that shock on the weakened cusp is reduced by cuspal coverage [11]. The stresses at the crack line were concentrated before operation under the 600 N and lateral force 225 N. Still, after high MOE onlay, overlay, and occlusal veneer 2, the stresses at the cracks were significantly reduced (Fig. 6). The type of material substantially affects the stress at the crack for the same form of preparation, which provides direction for selecting materials for the restoration of cracked teeth.
This study is the first to verify the stress effect of occlusal veneers on cracked teeth. No published research includes an FEA-based comparison of occlusal veneer designs. This study verified the stress effect of veneer restoration on cracked teeth for the first time. In this study, two preparation forms were verified, and it was found that different preparation methods under the same material and whether the resin base was significantly used affected the results. The combination of improved dental restorative materials and dental adhesive techniques can be used for thin-thickness restorations, thereby replacing lost hard tooth structure in a minimally invasive manner [42, 43]. Occlusal veneers as a treatment technique for live pulp cracked teeth have begun to be used in clinics [44]. The reduction and cuspal coverage allow the reduction of flexion during loading and minimize stress, thereby increasing the fracture toughness of the restored tooth to that of an intact tooth [10, 45]. It is important to note that cuspal restorations reinforce the tooth but may increase the risk of pulp exposure failure [46]. Cusp overlapping also involves eliminating significant amounts of healthy enamel and dentin. Occlusal veneer appears to have more advantages in this regard. The study results showed no significant difference in the average Von Mises Stress levels between the two occlusal veneer restorations and the onlay and overlay at the lower margin of the crack surface. Therefore, the occlusal veneer not only removes less tooth tissue but also achieves the goal of reducing stress in the cracked line.
The present study has several limitations. FEA is a widely used technique. However, many details are idealized, simplified, or overlooked, which results in some errors in the experimental results. In finite element analysis, three-dimensional modeling is a necessary procedure. However, not all structures can be fully simulated; for example, dentin is anisotropic. However, in this study, all structures were assumed to be homogeneous and isotropic. In this process, the effects of dentin tubules, endodontic static pressure, and elastic modulus gradient on the mechanical properties of dentin are ignored. As with previous finite element analysis studies, this is an essential limitation of this study. Additionally, temperature changes can generate thermal stress on the restored dental tissues. When hot foods or beverages enter the mouth, dental tissues and restorative materials expand, while they contract when cold substances come into contact with them. Due to the mismatch in the thermal expansion coefficient, differences in physical properties between restorative materials and tooth structures can also contribute to stress development. However, this study did not explore the effects of temperature changes on cracked molar teeth.
Tooth chewing is a dynamic process in which the force acting on the first mandibular molar is not unidirectional and plays a significant role. This experiment was only simplified as a static load, which limits the experimental results to some extent. In clinical practice, the forms of hidden cracks in teeth vary greatly. In this study, only a few representative forms of cracks were selected, and future research should be conducted on hidden cracks in other forms and locations. In conclusion, there may be some differences between the finite element method analysis and the actual situation, and further clinical trials are required to verify them.
Conclusion
This study employed three-dimensional finite element analysis to analyze the occlusal stress patterns at the lower margin of the remaining crack surface after treatment with various materials and designs in the cracked tooth model. We found that using higher MOE materials to repair onlay, overlay, and occlusal veneer 2 is a favorable method to prevent further crack propagation. It can transmit lower stress to the lower part of the restoration and result in lower internal stress concentration at the lower margin of the remaining crack surface. Occlusal veneer 1 with resin filling inside showed poor stress distribution and higher stress concentration on the remaining cracked area. This provides direction for selecting the composite resin as a substrate for cracked tooth restorations. Despite the limitations of this study, this finite element analysis study offers a new basis for clinical guidance on selecting materials and restoration methods suitable for cracked teeth.
Acknowledgements
Not applicable.
Clinical trial number
A protocol was specified and registered with the Chinese Clinical Trial Registry (ChiCTR) on 2022–04-12 (registration number: ChiCTR2200058630).
Authors’ contributions
TL, FHY, XW, and YNZ conceived this study and designed the study. TL collected, analyzed, and interpreted the data; wrote the paper; and prepared the figures. YHH and JLM performed contributed partially to the data acquisition. YW, YH and QZ contributed partially to the figure preparation and data analysis. TL, WDY, FHY, XW, and YNZ reviewed the manuscript, and supervised this project and revised the final manuscript. All authors read and approved the final manuscript.
Funding
This work was supported by the National Natural Scientific Foundation of China (82201055), Nanjing Medical Science and Technique Development Foundation (ZKX23053) and “2015” Cultivation Program for Reserve Talents for Academic Leaders of Nanjing Stomatological School, Medical School of Nanjing Univeristy (0223A205, 0223A207), “3456” Cultivation Program for Junior Talents of Nanjing Stomatological School, Medical School of Nanjing University (0222C116), the National Key R&D of Program of China (No. 2023YFC2506300).
Data availability
The datasets analysed during the current study are available from the corresponding author on reasonable request.
Declarations
Ethics approval and consent to participate
All the procedures were approved by the Ethics committee of Nanjing Stomatological Hospital (No. NJSH-2023NL-014–1) and conducted by the relevant guidelines and regulations. Informed consent was obtained from the subject. A protocol was specified and registered with the Chinese Clinical Trial Registry (ChiCTR) on 2022–04-12 (registration number: ChiCTR2200058630).
Consent for publication
Patients signed informed consent regarding publishing their data and photographs.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Contributor Information
Fuhua Yan, Email: yanfh@nju.edu.cn.
Xiang Wang, Email: wangxiang@nju.edu.cn.
Yanan Zhu, Email: zh-yanan@163.com.
References
- 1.Leong DJX, de Souza NN, Sultana R, Yap AU. Outcomes of endodontically treated cracked teeth: a systematic review and meta-analysis. Clin Oral Investig. 2020;24(1):465–73. [DOI] [PubMed] [Google Scholar]
- 2.Nuamwisudhi P, Jearanaiphaisarn T. Oral functional behaviors and tooth factors associated with cracked teeth in asymptomatic patients. J Endod. 2021;47(9):1383–90. [DOI] [PubMed] [Google Scholar]
- 3.Hilton TJ, Funkhouser E, Ferracane JL, Gilbert GH, Baltuck C, Benjamin P, Louis D, Mungia R, Meyerowitz C. Correlation between symptoms and external characteristics of cracked teeth: Findings from The National Dental Practice-Based Research Network. J Am Dent Assoc. 2017;148(4):246–256.e241. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 4.Krell KV, Caplan DJ. 12-month Success of Cracked Teeth Treated with Orthograde Root Canal Treatment. J Endod. 2018;44(4):543–8. [DOI] [PubMed] [Google Scholar]
- 5.Davis MC, Shariff SS. Success and survival of endodontically treated cracked teeth with radicular extensions: a 2- to 4-year prospective cohort. J Endod. 2019;45(7):848–55. [DOI] [PubMed] [Google Scholar]
- 6.Krell KV, Rivera EM. A six year evaluation of cracked teeth diagnosed with reversible pulpitis: treatment and prognosis. J Endod. 2007;33(12):1405–7. [DOI] [PubMed] [Google Scholar]
- 7.Kang SH, Kim BS, Kim Y. Cracked teeth: distribution, characteristics, and survival after root canal treatment. J Endod. 2016;42(4):557–62. [DOI] [PubMed] [Google Scholar]
- 8.Seo DG, Yi YA, Shin SJ, Park JW. Analysis of factors associated with cracked teeth. J Endod. 2012;38(3):288–92. [DOI] [PubMed] [Google Scholar]
- 9.Zhang S, Xu Y, Ma Y, Zhao W, Jin X, Fu B. The treatment outcomes of cracked teeth: A systematic review and meta-analysis. J Dent. 2024;142:104843. [DOI] [PubMed] [Google Scholar]
- 10.Banerji S, Mehta SB, Millar BJ: Cracked tooth syndrome. Part 2: restorative options for the management of cracked tooth syndrome. Br Dent J 2010, 208(11):503–514. [DOI] [PubMed]
- 11.Opdam NJ, Roeters JJ, Loomans BA, Bronkhorst EM. Seven-year clinical evaluation of painful cracked teeth restored with a direct composite restoration. J Endod. 2008;34(7):808–11. [DOI] [PubMed] [Google Scholar]
- 12.Naka O, Millar BJ, Sagris D, David C. Do composite resin restorations protect cracked teeth? An in-vitro study Br Dent J. 2018;225(3):223–8. [DOI] [PubMed] [Google Scholar]
- 13.Wu S, Lew HP, Chen NN. Incidence of Pulpal Complications after Diagnosis of Vital Cracked Teeth. J Endod. 2019;45(5):521–5. [DOI] [PubMed] [Google Scholar]
- 14.Banerji S, Mehta SB, Kamran T, Kalakonda M, Millar BJ. A multi-centred clinical audit to describe the efficacy of direct supra-coronal splinting–a minimally invasive approach to the management of cracked tooth syndrome. J Dent. 2014;42(7):862–71. [DOI] [PubMed] [Google Scholar]
- 15.Yap EXY, Chan PY, Yu VSH, Lui JN. Management of cracked teeth: Perspectives of general dental practitioners and specialists. J Dent. 2021;113:103770. [DOI] [PubMed] [Google Scholar]
- 16.Signore A, Benedicenti S, Covani U, Ravera G. A 4- to 6-year retrospective clinical study of cracked teeth restored with bonded indirect resin composite onlays. Int J Prosthodont. 2007;20(6):609–16. [PubMed] [Google Scholar]
- 17.Veneziani M. Posterior indirect adhesive restorations: updated indications and the Morphology Driven Preparation Technique. Int J Esthet Dent. 2017;12(2):204–30. [PubMed] [Google Scholar]
- 18.Wang M, Hong Y, Hou X, Pu Y. Biting and thermal sensitivity relief of cracked tooth restored by occlusal veneer: A 12-to 24 months prospective clinical study. J Dent. 2023;138:104694. [DOI] [PubMed] [Google Scholar]
- 19.Alghauli M, Alqutaibi AY, Wille S, Kern M. Clinical outcomes and influence of material parameters on the behavior and survival rate of thin and ultrathin occlusal veneers: A systematic review. J Prosthodont Res. 2023;67(1):45–54. [DOI] [PubMed] [Google Scholar]
- 20.Ladino L, Sanjuan ME, Valdez DJ, Eslava RA. Clinical and Biomechanical Performance of Occlusal Veneers: A Scoping Review. J Contemp Dent Pract. 2021;22(11):1327–37. [PubMed] [Google Scholar]
- 21.Pacquet W, Delebarre C, Browet S, Gerdolle D. Therapeutic strategy for cracked teeth. Int J Esthet Dent. 2022;17(3):340–55. [PubMed] [Google Scholar]
- 22.Valera-Jiménez JF, Burgueño-Barris G, Gómez-González S, López-López J, Valmaseda-Castellón E, Fernández-Aguado E. Finite element analysis of narrow dental implants. Dent Mater. 2020;36(7):927–35. [DOI] [PubMed] [Google Scholar]
- 23.Matuda AGN, Silveira MPM, Andrade GS, Piva A, Tribst JPM, Borges ALS, Testarelli L, Mosca G, Ausiello P: Computer Aided Design Modelling and Finite Element Analysis of Premolar Proximal Cavities Restored with Resin Composites. Materials (Basel) 2021, 14(9). [DOI] [PMC free article] [PubMed]
- 24.Barbosa FT, Zanatta LCS, de Souza RE, Gehrke SA. Comparative analysis of stress distribution in one-piece and two-piece implants with narrow and extra-narrow diameters: A finite element study. PLoS ONE. 2021;16(2):e0245800. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Eram A, Zuber M, Keni LG, Kalburgi S, Naik R, Bhandary S, Amin S, Badruddin IA. Finite element analysis of immature teeth filled with MTA, Biodentine and Bioaggregate. Comput Methods Programs Biomed. 2020;190:105356. [DOI] [PubMed] [Google Scholar]
- 26.Kim SY, Kim BS, Kim H, Cho SY. Occlusal stress distribution and remaining crack propagation of a cracked tooth treated with different materials and designs: 3D finite element analysis. Dent Mater. 2021;37(4):731–40. [DOI] [PubMed] [Google Scholar]
- 27.Dejak B, Młotkowski A. 3D-Finite element analysis of molars restored with endocrowns and posts during masticatory simulation. Dent Mater. 2013;29(12):e309–317. [DOI] [PubMed] [Google Scholar]
- 28.Lin C, Hu H, Zhu J, Wu Y, Rong Q, Tang Z. Influence of sagittal root positions on the stress distribution around custom-made root-analogue implants: a three-dimensional finite element analysis. BMC Oral Health. 2021;21(1):443. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 29.Couegnat G, Fok SL, Cooper JE, Qualtrough AJ. Structural optimization of dental restorations using the principle of adaptive growth. Dent Mater. 2006;22(1):3–12. [DOI] [PubMed] [Google Scholar]
- 30.Martins LM, de Lima LM, da Silva LM, Cohen-Carneiro F, Noritomi PY, Lorenzoni FC: The crown materials and the occlusal thickness affect the load stress dissipation on 3D molar crown: Finite Element Analysis. Int J Prosthodont 2021. [DOI] [PubMed]
- 31.He J, Zheng Z, Wu M, Zheng C, Zeng Y, Yan W. Influence of restorative material and cement on the stress distribution of endocrowns: 3D finite element analysis. BMC Oral Health. 2021;21(1):495. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Tribst JPM, Lo Giudice R, Dos Santos AFC, Borges ALS, Silva-Concílio LR, Amaral M, Lo Giudice G: Lithium Disilicate Ceramic Endocrown Biomechanical Response According to Different Pulp Chamber Extension Angles and Filling Materials. Materials (Basel) 2021, 14(5). [DOI] [PMC free article] [PubMed]
- 33.Wimmer T, Erdelt KJ, Raith S, Schneider JM, Stawarczyk B, Beuer F. Effects of differing thickness and mechanical properties of cement on the stress levels and distributions in a three-unit zirconia fixed prosthesis by FEA. J Prosthodont. 2014;23(5):358–66. [DOI] [PubMed] [Google Scholar]
- 34.Zhang Z, Zheng K, Li E, Li W, Li Q, Swain M. Mechanical benefits of conservative restoration for dental fissure caries. J Mech Behav Biomed Mater. 2016;53:11–20. [DOI] [PubMed] [Google Scholar]
- 35.Liu B, Lu C, Wu Y, Zhang X, Arola D, Zhang D. The effects of adhesive type and thickness on stress distribution in molars restored with all-ceramic crowns. J Prosthodont. 2011;20(1):35–44. [DOI] [PubMed] [Google Scholar]
- 36.Jiang Q, Huang Y, Tu X, Li Z, He Y, Yang X. Biomechanical properties of first maxillary molars with different endodontic cavities: a finite element analysis. J Endod. 2018;44(8):1283–8. [DOI] [PubMed] [Google Scholar]
- 37.Xu M, Zhou L, Zheng L, Zhou Q, Liu K, Mao Y, Song S. Sonodynamic therapy-derived multimodal synergistic cancer therapy. Cancer Lett. 2021;497:229–42. [DOI] [PubMed] [Google Scholar]
- 38.Kakka A, Gavriil D, Whitworth J. Treatment of cracked teeth: A comprehensive narrative review. Clin Exp Dent Res. 2022;8(5):1218–48. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 39.Alkhalifah S, Alkandari H, Sharma PN, Moule AJ. Treatment of cracked teeth. J Endod. 2017;43(9):1579–86. [DOI] [PubMed] [Google Scholar]
- 40.Sasse M, Krummel A, Klosa K, Kern M. Influence of restoration thickness and dental bonding surface on the fracture resistance of full-coverage occlusal veneers made from lithium disilicate ceramic. Dent Mater. 2015;31(8):907–15. [DOI] [PubMed] [Google Scholar]
- 41.Sun J, Jiang J, Xue Z, Ma H, Pan J, Qian K. Mechanical properties of cracked teeth with different dental materials and crown parameters: An in vitro proof-of-concept. J Mech Behav Biomed Mater. 2023;145:106045. [DOI] [PubMed] [Google Scholar]
- 42.Al-Akhali M, Kern M, Elsayed A, Samran A, Chaar MS. Influence of thermomechanical fatigue on the fracture strength of CAD-CAM-fabricated occlusal veneers. J Prosthet Dent. 2019;121(4):644–50. [DOI] [PubMed] [Google Scholar]
- 43.Bajraktarova-Valjakova E, Korunoska-Stevkovska V, Kapusevska B, Gigovski N, Bajraktarova-Misevska C, Grozdanov A. Contemporary dental ceramic materials, a review: chemical composition, physical and mechanical properties, indications for use. Open Access Maced J Med Sci. 2018;6(9):1742–55. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 44.Fitzsimonds ZR, Rodriguez-Hernandez CJ, Bagaitkar J, Lamont RJ. From beyond the pale to the pale riders: the emerging association of bacteria with oral cancer. J Dent Res. 2020;99(6):604–12. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 45.Guess PC, Schultheis S, Wolkewitz M, Zhang Y, Strub JR. Influence of preparation design and ceramic thicknesses on fracture resistance and failure modes of premolar partial coverage restorations. J Prosthet Dent. 2013;110(4):264–73. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 46.Fennis WM, Kuijs RH, Kreulen CM, Verdonschot N, Creugers NH. Fatigue resistance of teeth restored with cuspal-coverage composite restorations. Int J Prosthodont. 2004;17(3):313–7. [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
The datasets analysed during the current study are available from the corresponding author on reasonable request.