ETHY. A Theory of Fruit Climacteric Ethylene Emission

Abstract

A theory of fruit climacteric ethylene emission was developed and used as the basis of a simulation model called ETHY. According to the theory, the biosynthetic pathway of ethylene is supplied by ATP and is regulated by 1-aminocyclopropane-1-carboxylic acid (ACC) synthase and ACC oxidase. The conjugation of ACC with malonate to form MACC was taken into account as a way to decrease the availability of ACC. Because of the seasonal increase of fruit volume, the dilution of biochemical compounds used in ETHY was taken into account. Finally, the ethylene diffusion across the skin was considered. The theory took into account the effect of temperature and O₂ and CO₂ internal concentrations on ethylene. The model was applied to peach (Prunus persica) fruit over 3 years, several leaf:fruit ratios, and irrigation conditions. An adequate ethylene increase was predicted without considering any increase in respiration during the ripening period, which suggests that the respiratory climacteric may not be required for ripening. Another important result of this study is the high sensitivity of ETHY to the parameters involved in the calculation of ACC oxidase and ACC synthase activities, ATP production, and skin surface and permeability. ETHY was also highly sensitive to changes in fruit growth and temperature.

The plant hormone ethylene (C₂H₄) plays a major role in the ripening process of climacteric fruits. Ripening parameters such as flesh softening (Haji et al., 2003; Hiwasa et al., 2003), color change (Flores et al., 2001), and production of aromas depend strongly on C₂H₄ production (Rupasinghe et al., 2000; Alexander and Grierson, 2002; Flores et al., 2002).

C₂H₄ production in plant tissues has been studied extensively (Arshad and Frankenberger, 2002). It proceeds via a biosynthetic pathway, which was first established in apple (Malus domestica) fruit by Adams and Yang (1977). This pathway proceeds from Met, through S-adenosylmethionine (SAM) and 1-aminocyclopropane-1-carboxylic acid (ACC) to C₂H₄. The pathway enables high rates of C₂H₄ production without high intracellular concentrations of Met. This is achieved by recycling 5′-methylthioadenosine (MTA) to Met through the Yang cycle. The respiration produces the ATP needed for the Yang cycle. Thanks to this recycling process, high levels of C₂H₄ can be produced with a constant pool of Met (Arshad and Frankenberger, 2002). It is known that C₂H₄ production rates in ripening fruit are controlled by the tissue's capability to synthesize ACC and to convert ACC to C₂H₄. The two key controls are ACC synthase (ACCs) and ACC oxidase (ACCo; Tucker, 1993). The C₂H₄ is diluted in fruit tissue and then diffused into the atmosphere. Thus, fruit volume and permeability of skin to gas are important biophysical traits of fruit to consider when analyzing C₂H₄ emission (Ben-Yehoshua and Cameron, 1989).

The aim of this work was to develop a theory of C₂H₄ emission, based on a mathematical representation of the respiration process and C₂H₄ pathway. This representation was chosen to be as simple as possible because a lot of quantitative information about the regulation of this pathway is missing. The theory relates C₂H₄ production to fruit growth and environmental conditions. Indeed, fruit C₂H₄ production is known to vary during the development of the fruit depending on environmental conditions such as temperature; levels of O₂ and CO₂ in the air (Lelièvre et al., 1997); and fruit growth manipulated by changing leaf:fruit ratio (Poll et al., 1996; Souty et al., 1999), fruit thinning (Johnson, 1995), or branch ringing (Agusti et al., 1998). The theory was the basis of the ETHY simulation model, which predicts the seasonal C₂H₄ production. The model was tested by comparing simulations to experimental data recorded on peach (Prunus persica) fruit during 3 years under various leaf:fruit ratios and irrigation conditions. The importance of the different processes and environmental factors involved in C₂H₄ production was analyzed through a sensitivity analysis.

THEORETICAL ANALYSIS OF THE SYSTEM

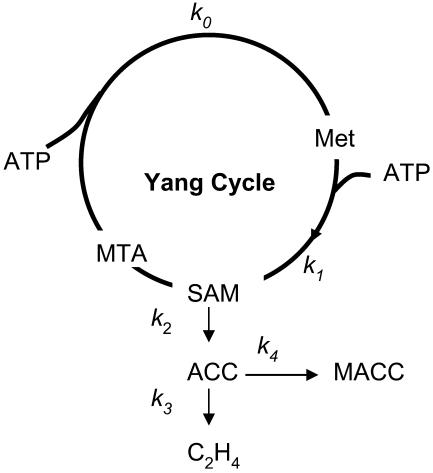
The theory concerns C₂H₄ emission during the last period of fruit development (for a peach, this is the last 2–3 months before maturity). It concerns (1) the biosynthetic pathway of C₂H₄, which includes the Yang cycle and the SAM-C₂H₄ pathway schematized in Figure 1, and (2) dilution and diffusion of C₂H₄ through the fruit skin.

Figure 1.

Schematic representation of the Yang cycle and SAM-C₂H₄ pathway. The metabolites considered are ATP, Met, SAM, MTA, MACC, and C₂H₄. Chains of reactions involved in their conversions are represented as simple reactions. The k_i are the rate constants of the reactions.

It is known that Met and SAM pools are too low in plant tissues to sustain normal rates of ACC production, implying that they must be continuously regenerated (Baur and Yang, 1972). This is the role of the Yang cycle. As this cycle is supplied by ATP (Kushad et al., 1983; Theologis, 1992), ATP production through respiration is an important aspect of ETHY. The SAM-C₂H₄ pathway is regulated by ACCs and ACCo, which are the two key enzymes considered in ETHY. The stress was laid on the autocatalytic C₂H₄ production, which results in up-regulation by C₂H₄ of these two enzymes. The conjugation of ACC with malonate to form malonyl-ACC (MACC) was taken into account in ETHY as a way to decrease the availability of ACC for C₂H₄ production. Because of the seasonal increase of fruit volume, the dilution of biochemical compounds used in ETHY was taken into account. Finally, C₂H₄ diffusion across the skin was considered.

Metabolic and biophysical processes are strongly connected in ETHY. For example, the diffusion of C₂H₄ in the ambient atmosphere depends on the internal C₂H₄ concentration, which depends itself on the intensity of the diffusion and on other processes (dilution, metabolism). Yang cycle, respiration, production of MACC, regulation of ACCs and ACCo, dilution, and C₂H₄ release in ambient atmosphere are the basic processes represented in ETHY. The fruit is described as one compartment separated from the exterior by the skin. The ambient atmosphere plays the role of the exterior compartment. Gas exchange occurs by diffusion through the fruit skin. The temperature of the fruit is assumed to be equal to that of the ambient atmosphere.

Fruit growth is considered to influence C₂H₄ production because it is related to respiration, dilution, and to the skin area through which C₂H₄ is released. The temperature is an important external factor controlling respiration in ETHY. The model also takes into account the effect of O₂ and CO₂ internal concentrations on C₂H₄ through their action on ACCo, which requires O₂ and is inhibited by high concentrations of CO₂ (Lelièvre et al., 1997).

The main state variables of the system are MACC, ACC, CO₂, O₂, and C₂H₄ concentrations in the fruit. The hourly information coming from the external compartment is the temperature and the concentrations of O₂ and CO₂ in the ambient atmosphere. This information and the fruit growth components (dry and fresh mass, and dry growth rate) represent the ETHY inputs. Using the inputs together with a theoretical analysis quantified by the governing equations, we compute the main reaction rates of the C₂H₄ biosynthesis pathway and gas transfer processes. The lists of the ETHY variables and parameters are presented in “Materials and Methods.”

Governing Equations

Dilution versus Metabolism and/or Diffusion

In the following, the amount of a reactant X per fruit will be noted X and its concentration [X] (mol m⁻³).

As [X] = X/V, with V (m⁻³) ≈ 10⁻⁶ M_fresh and M_fresh (g) the fruit fresh mass, we get:

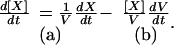(1, a and b)

This equation, which shows that the rate of variation of the concentration of a reactant, depends on two components related to metabolism and/or diffusion of X outside the fruit (a) and to dilution (b), will be used in the following to take into account the increase of fruit fresh mass during growth.

Yang Cycle, and the SAM-C₂H₄ and ACC-MACC Pathways

The Yang cycle and the SAM-C₂H₄ pathway (Fig. 1) are described according to the “rate law” of chemical kinetics (Chang, 2000), which states that the rate of a reaction is proportional to the reactant X in the fruit. The rate constant is denoted k_i. The following set of equations was used for the Yang cycle:

(2)

In keeping with the recycling scheme,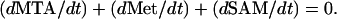

The variation of ACC results from the balance between its synthesis, its degradation in C₂H₄ controlled by ACCo (k₃; h⁻¹), and its conjugation with malonate to form MACC catalyzed by the ACC N-malonyltransferase (k₄; h⁻¹). The rate law equation for ACC is then:

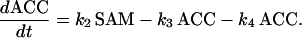(3)

Assuming the Yang cycle at steady-state 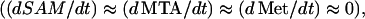Equation 2 shows that the rate of synthesis of ACC from SAM is proportional to the amount of ATP present:

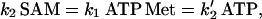where k′₂ (h⁻¹) is a rate constant related to the activity of ACCs.

The ACC concentration depends on the amount of ACC per fruit and on dilution by water according to Equations 1 and 3. The rate law equation for ACC concentration is then:

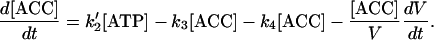(4)

The variation of MACC concentration is:

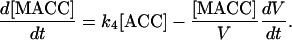(5)

The C₂H₄ concentration in the fruit depends on the one hand on the balance between C₂H₄ biosynthesis and diffusion to the external atmosphere (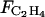; mol h⁻¹), and on the other hand on dilution:

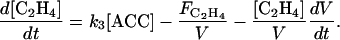(6)

Enzyme Regulations

Although the ACC N-malonyltransferase is developmentally regulated (Arshad and Frankenberger, 2002), the rate constant k₄ was considered invariant for the sake of simplicity. Both ACCs and ACCo are encoded by multigene families (Lelièvre et al., 1997) and are regulated by a number of regulating factors. The values of the rate constants k′₂ and k₃ depend on the level of regulating factors, which influence the activities of both enzymes. The rate constants were considered to be the product of a parameter (respectively, k_S and k_O; h⁻¹) by functions of regulating factors. Both enzymes are regulated by C₂H₄ (Lelièvre et al., 1997). For the sake of simplicity, the effect was considered to be proportional to the C₂H₄ concentration. The oxygen concentration in the tissues plays an important role in C₂H₄ biosynthesis since oxygen is a cosubstrate of ACCo. It has been assumed that ACCo is inhibited by carbon dioxide (Rothan and Nicolas, 1994). To describe this regulation of ACCo, a generalized form of the Michaelis-Menten equation used by de Wild et al. (1999) for pear (Pyrus communis) was applied.

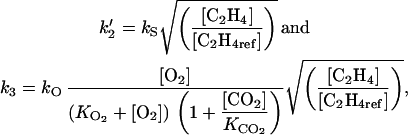(7)

with [C₂H_4ref] a reference concentration equal to 1 mol m⁻³ and 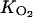(mol m⁻³) and 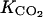(mol m⁻³) K_ms.

C₂H₄ Diffusion to the External Atmosphere

C₂H₄ diffusion to the external atmosphere has been described by Fick's first law for flat surfaces, which has often been applied to the study of gas exchange in bulky organs (Ben-Yehoshua and Cameron, 1989). Considering that C₂H₄ concentration in the ambient atmosphere is nil, C₂H₄ diffusion to the external atmosphere is:

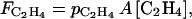(8)

where 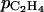(m h⁻¹) is the apparent skin permeability and A (m²) is the skin area.

A is estimated from fruit mass through an empirical equation:

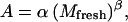(9)

where α and β are parameters.

According to Lescourret et al. (2001), skin permeability can vary with fruit size. It was assumed to vary linearly with fruit size:

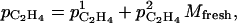(10)

where 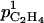(m h⁻¹) and 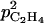(m g⁻¹ h⁻¹) are parameters.

ACC and C₂H₄ Concentrations, and ACCs and ACCo Activities

The rate of variation of ACC and C₂H₄ concentration may be obtained by combining Equations 4 and 6 to 8:

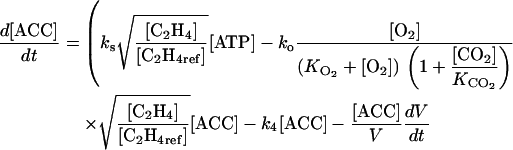(11)

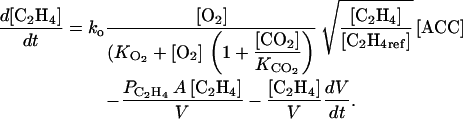(12)

The activity of ACCs is represented by ACC synthesis for a given concentration of ATP ([ATP⁰]). The activity of ACCo is represented by C₂H₄ synthesis for a given concentration of 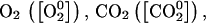and ACC ([ACC⁰]).

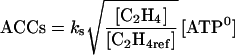(13)

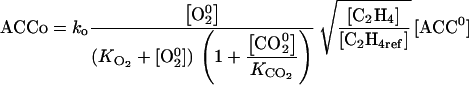(14)

ATP, O₂, and CO₂ Concentrations

The concentration of ATP in the fruit results from the balance between ATP production during the respiration process and consumption for energy-requiring processes with a rate constant λ (h⁻¹) and dilution in water. One mol of CO₂ produced by respiration was assumed to be coupled with the production of 5 mol of ATP (Cannell and Thornley, 2000). Accordingly, the variation of ATP concentration is:

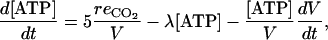(15)

where 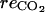is the rate of CO₂ production caused by respiration.

Spoelstra et al. (2002) have shown in tomato (Lycopersicon esculentum) embryos that the change of ATP concentration is negligible compared to the actual amount of ATP synthesized and turned over (i.e. 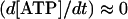). Assuming that such a property is valid for the fruit, the ATP concentration can be approximated by the following:

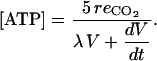(16)

This equation means that the ATP concentration is proportional to the respiration per unit of fruit mass when the fruit growth is negligible, which has been shown in ripening avocado (Persea americana) fruit (Bennett et al., 1987).

The oxygen and carbon dioxide concentrations are calculated assuming that they are under steady-state condition [i.e. respiration rates for O₂ (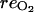) and CO₂ are equal to the fluxes of these gases through the skin to the external atmosphere], and the gas diffusion to the external atmosphere can be described by Fick's first law:

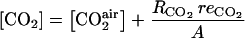(17)

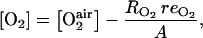(18)

where 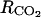(h m⁻¹) and 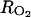(h m⁻¹) are resistances to diffusion of CO₂ and O₂, respectively. The air CO₂ and O₂ concentrations in mol m⁻³ are calculated from the percentage of O₂ in the air using the ideal-gas equation.

The respired oxygen is calculated from the CO₂ produced by the respiration using the respiratory quotient (RQ) concept:

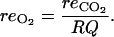(19)

The growth-maintenance paradigm (Cannell and Thornley, 2000) was used to calculate the respiration in terms of CO₂. The growth respiration is considered proportional to fruit growth rate and the maintenance respiration to dry mass and temperature (Penning de Vries and van Laar, 1982; Thornley and Johnson, 1990). The effect of temperature is described with the Q₁₀ concept. The fruit respiration in terms of CO₂ production (mol h⁻¹) is then calculated as:

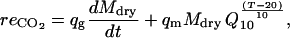(20)

where M_dry (g) is fruit dry mass, q_g (mol g⁻¹) the growth respiration coefficient, q_m (mol g⁻¹ h⁻¹) the maintenance respiration coefficient at 20°C, Q₁₀ the temperature ratio of maintenance respiration, and T (°C) the temperature.

Equations 16 to 20 are combined to calculate ATP, CO₂, and O₂ concentrations as a function of fruit dry mass, volume, area, and temperature:

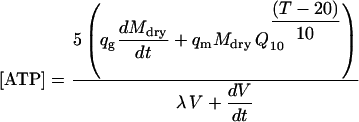(21)

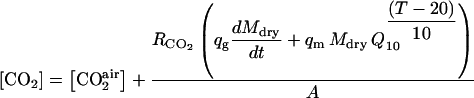(22)

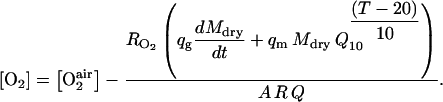(23)

Equations 5, 8 to 12, and 21 to 23 form a system of equations for [ACC], [MACC], [C₂H₄], [ATP], [CO₂], [O₂], and 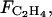which can be solved numerically with given (inputted) functions of time M_dry(t), M_fresh(t) and T(t); O₂ and CO₂ concentrations in the air (assumed to be constant); and the initial values of respective variables.

Initial Conditions and Parameterization

The MACC concentration reported in the literature during the early growth of fruits is very variable, from 3 10⁻⁵ to 8 10⁻³ mol m⁻³ according to the authors (Amoros et al., 1989; Tonutti et al., 1991; Lara and Vendrell, 2000). We chose an initial value of 5 10⁻⁴ mol m⁻³ to run the model. The ACC concentration can be very low before the rise of C₂H₄ production. ACC concentrations lower than 10⁻⁵ mol m⁻³ have been reported (Amoros et al., 1989; Lara and Vendrell, 2000). An initial value of 10⁻⁵ mol m⁻³ was chosen to run the model. The internal C₂H₄ concentration was reported to stay between almost zero and 2 10⁻⁶ mol m⁻³ before the C₂H₄ crisis for different fruit species (Lyons et al., 1962; Lau et al., 1986; Saltveit, 1993; Johnston et al., 2002). An initial value of 10⁻⁶ mol m⁻³ was chosen for the internal C₂H₄ concentration.

The Michaelis-Menten constant for O₂ and CO₂ inhibition of C₂H₄ production were deduced from the experiment of de Wild et al. (1999) on pear fruit (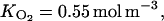 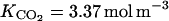). The apparent peach skin permeability to C₂H₄ was measured by Cameron and Reid (1982). Considering at first approximation that it was unvarying with fruit development, we took their estimation (2 10⁻³ m h⁻¹) for 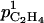and zero for 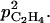Parameters α = 6.049 10⁻⁴ and β = 0.601 in Equation 9, relating the fruit surface area to the fruit mass, were taken from Fishman and Génard (1998) for the peach cultivar Suncrest. To estimate λ, the rate constant of ATP consumption, we considered that the mean ATP concentration in the fruit tissues was 0.015 mol m⁻³ according to the data of Saquet et al. (2000, 2003a, 2003b). Then Equation 21 was used to get an estimation of λ = 500 h⁻¹. The temperature ratio of maintenance respiration Q₁₀ was taken equal to 2 as estimated by Pavel and DeJong (1993). The q_g and q_m were estimated using nonlinear least squares regression, Equation 20, and respiration measurements. The growth respiration coefficient was estimated to be equal to q_g = 0.0025 ± 2 10⁻⁴ mol g⁻¹, which is lower than the value (0.007 mol g⁻¹) obtained by DeJong and Goudriaan (1989) for peach fruits but close to coefficients obtained for fleshy fruits such as mango (Mangifera indica; 0.0033 mol g⁻¹) and cucumber (Cucumis sativus; 0.0036 mol g⁻¹) by Léchaudel et al. (2005) and Marcelis and Baan Hofman-Eijer (1995). The maintenance respiration coefficient at 20°C was estimated to be equal to q_m = 12 10⁻⁵ ± 5 10⁻⁶ mol g⁻¹ h⁻¹, a value higher than that estimated for peach (5.6 10⁻⁵ mol g⁻¹ d⁻¹) by DeJong and Goudriaan (1989), lower than that measured on cucumber (34 10⁻⁵ mol g⁻¹ d⁻¹) by Marcelis and Baan Hofman-Eijer (1995) and on tomato (27 10⁻⁵ mol g⁻¹ d⁻¹) by Walker and Thornley (1977), but comparable to that obtained on mango (9.6 10⁻⁵ mol g⁻¹ d⁻¹) by Léchaudel et al. (2005). The respiratory quotient (RQ) was calculated as the mean of the measured ratio 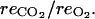The estimation was RQ = 0.834 ± 0.006. The ranges of CO₂ and O₂ concentrations for fleshy fruits are 0.4% to 8% and 15% to 19%, respectively, according to measurements obtained for cantaloupe (Cucumis melo; Lyons et al., 1962), orange (Citrus sinensis; Ben-Yehoshua et al., 1985), avocado (Ben-Yehoshua et al., 1963), pear (Williams and Patterson, 1962), and tomato (Saltveit, 1993). To estimate the CO₂ and O₂ resistances to diffusion ( 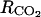and 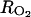), we considered that the mean concentrations in the fruit tissues have to be in these ranges. Using Equations 22 and 23, we obtaine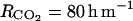d and 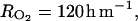which are close to the minimal resistance values obtained by Cameron and Reid (1982) for different fruit species (R_CO2 = 117–583 h m⁻¹, R_O2 = 80–833 h m⁻¹). The air O₂ and CO₂ concentrations used in Equations 22 and 23 were 20.97% and 0.03%, respectively. The rate constant for ACC malonyltransferase was taken at k₄ = 0.001 h⁻¹ in order to obtain coherent MACC concentrations with what is mentioned in the literature with regard to peach (3 10⁻⁵ to 10⁻² mol m⁻³; Amoros et al., 1989; Tonutti et al., 1991). The other parameters of the model (k_s and k_o) were estimated using nonlinear least squares, the model (to predict the C₂H₄ emission), and C₂H₄ measurements.

ETHY Inputs

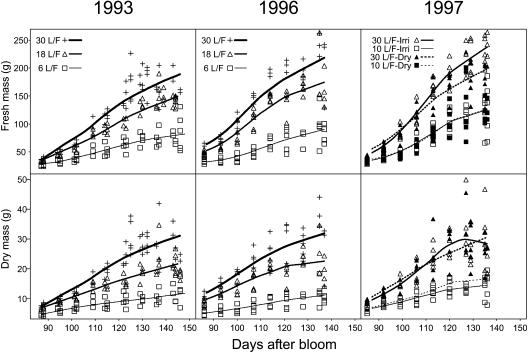
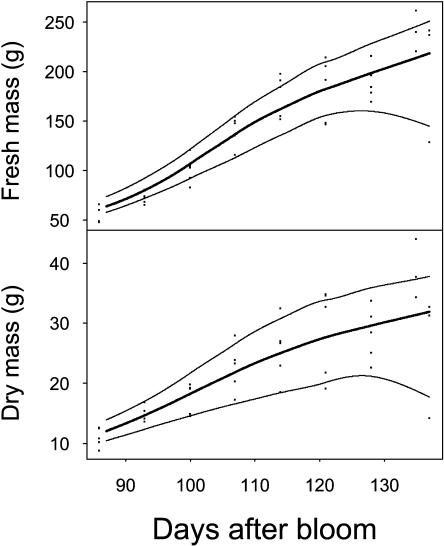
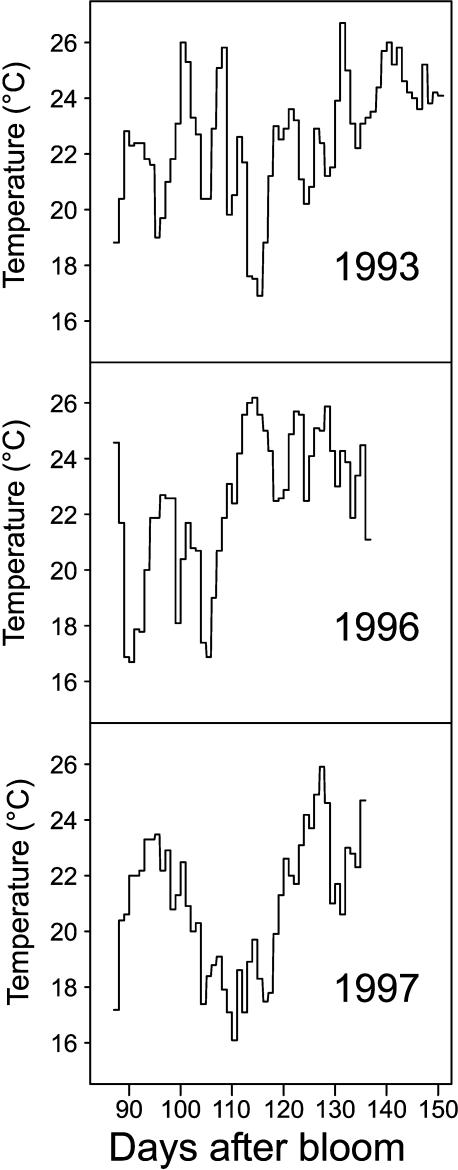
The seasonal trends of fruit masses are shown in Figure 2. Fresh and dry masses increased with both fruit age and leaf:fruit ratio. The suppression of tree irrigation in 1997 had only a minor negative effect on fresh mass for the 30 leaf:fruit ratio. There was high variability between fruit masses for a given treatment and date. That is why the mean, maximal, and minimal growth curves (Fig. 3) were used for each treatment and year as inputs of ETHY. The mean temperature was 22.5°C in 1993 and 1996, and 21°C in 1997. The mean daily temperature fluctuated greatly each year between 16°C and 26°C (Fig. 4).

Figure 2.

Seasonal variation of peach fruit (cv Suncrest) fresh and dry mass over the 3-year experimental period. Points are experimental data and lines smooth curves. The leaf:fruit and irrigation treatments are indicated on the graphs.

Figure 3.

Seasonal variation of peach fruit (cv Suncrest) fresh and dry mass in 1996 for the treatment involving 30 leaves per fruit. The maximal, mean, and minimum curves are drawn. Points are experimental data and lines smooth curves.

Figure 4.

Seasonal variation of mean daily temperature over the 3-year experimental period.

RESULTS

ETHY Goodness-of-Fit and Predictive Quality

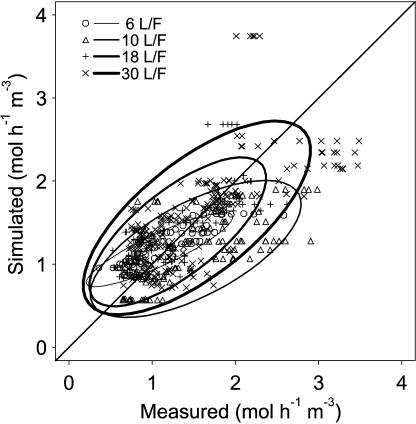
The model was able to simulate the order of magnitude of fruit respiration, the lack of irrigation effect (data not shown), and the increase of maximal respiration with the leaf:fruit ratio (Fig. 5). Root mean squared errors (RMSE) varied from 0.16 to 0.38 mol h⁻¹ m⁻³ according to year and leaf:fruit ratio treatment, and the mean value of the error was 0.27 mol h⁻¹ m⁻³. The predictive quality of the model was quite correct: the root mean squared errors of prediction (RMSEP), which ranged from 0.16 to 0.54 mol h⁻¹ m⁻³ with a mean equal to 0.3 mol h⁻¹ m⁻³, were close to the RMSE.

Figure 5.

Simulated versus measured respiration rate over the 3-year experimental period. Variance ellipses indicating 90% of the data for a given leaf:fruit ratio are drawn (the thickness of the ellipse line is proportional to the leaf:fruit ratio).

With regard to C₂H₄, the RMSE varied from 8 to 234 μmol h⁻¹ m⁻³, according to year and leaf:fruit ratio, and the mean value of the error was 89 μmol h⁻¹ m⁻³. The RMSEP ranged from 7 to 260 μmol h⁻¹ m⁻³ with an average of 107 μmol h⁻¹ m⁻³. The parameters related to ACCs and ACCo were estimated at k_s = 0.778 ± 0.042 h⁻¹, k_o = 0.268 ± 0.017 h⁻¹.

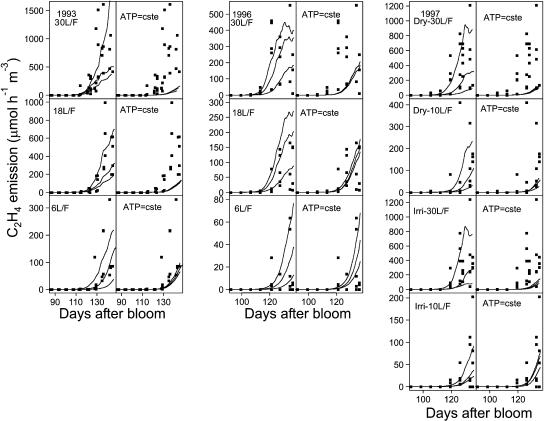
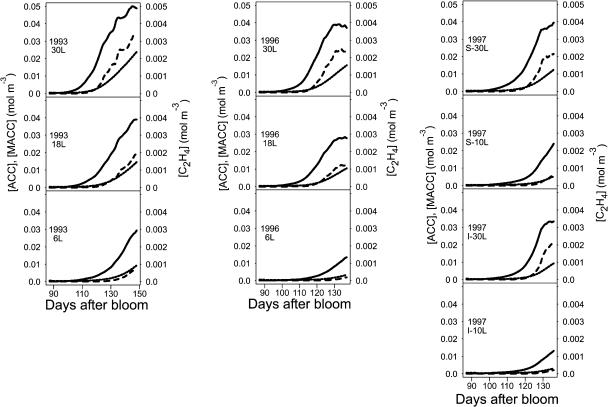
In accordance with the measured data, the model predicted an increase in C₂H₄ production with the leaf:fruit ratio, no effect of irrigation treatment, and lower C₂H₄ production in 1996 than in the other two years (Fig. 6). The intratreatment variation of C₂H₄ production was high (Fig. 6), which was fairly well depicted by the model using as input the maximal and minimal growth curves of each treatment.

Figure 6.

Observed (points) and simulated (lines) C₂H₄ emission for each treatment over the 3-year experimental period. The three lines per graph originate from three simulations corresponding to the maximum, mean, and minimum masses of fruits. For each year and treatment, the case where the ATP concentration varies as a function of respiration according to the model assumption (graphs on the left for each experiment) and the case where it is constant were considered (graphs on the right).

Seasonal Variation of ATP, Internal O₂ and CO₂, ACC, MACC, and C₂H₄ Concentrations in Response to Year and Fruit Growth

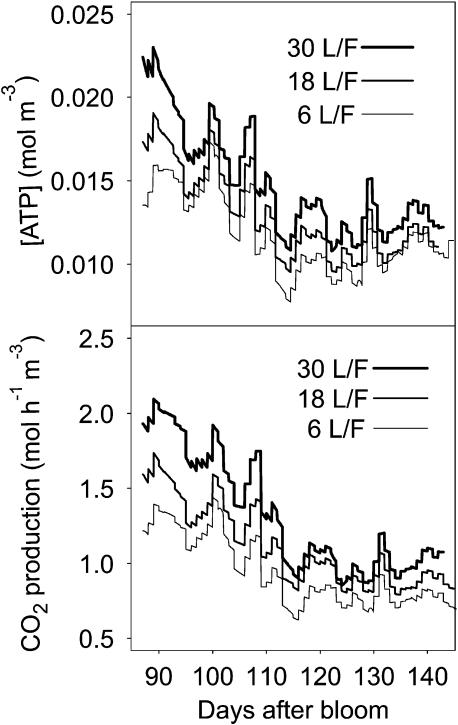
The model predicted significant variations of ATP concentration from 0.008 to 0.023 mol m⁻³ with a general decrease following that of fruit respiration per unit of fruit volume (Fig. 7). The increase of fruit growth from six to 30 leaves per fruit has for consequence a 25% increase of ATP concentration. At a short time step, fairly high fluctuations of ATP concentrations are simulated, which results mainly from the effect of temperature on fruit respiration (Figs. 4 and 7).

Figure 7.

Seasonal variation of ATP concentration and respiration rate simulated by ETHY over the three leaf:fruit ratios studied in 1993.

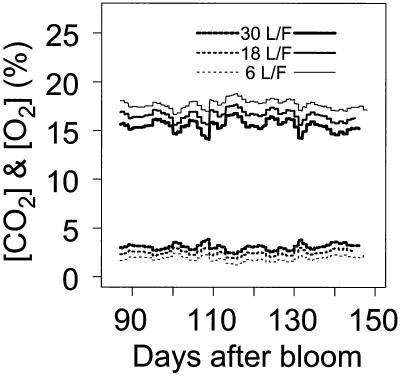
The predicted concentrations of O₂ in the fruit for the different treatments and years were in the14% to 18.7% range, which were slightly lower than in the air (21%). The predicted CO₂ concentrations in the fruit (1.3%–3.9%) were much higher than in the air (0.03%). Under the steady-state assumption, the O₂ and CO₂ concentrations in the fruit were fairly stable during the season, but short time variations were observed in response to temperature fluctuations (Fig. 8). The increase of fruit growth with leaf:fruit ratio resulted in an [O₂] decrease and [CO₂] increase in the fruit.

Figure 8.

Seasonal variation of O₂ (continuous lines) and CO₂ (dashed lines) concentrations simulated by ETHY over the three leaf:fruit ratios studied in 1993.

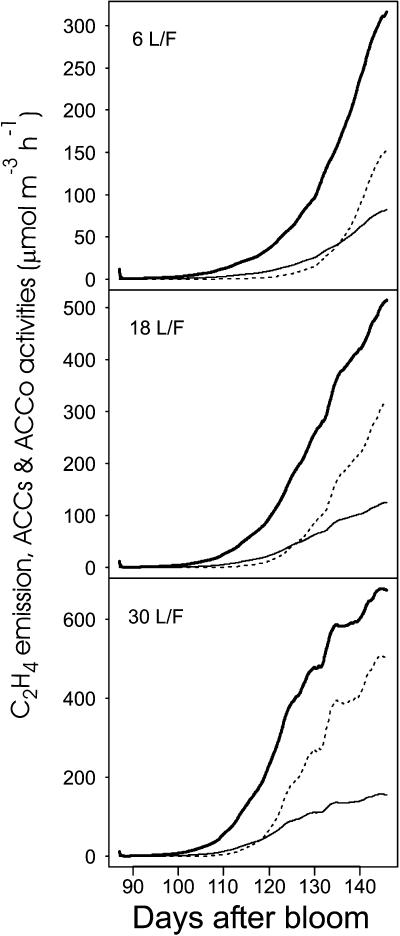
The model predicted a seasonal increase of ACC, MACC, and C₂H₄ concentrations and a positive effect of leaf number per fruit (Fig. 9). The concentration of C₂H₄ was 10 times lower than that of MACC and ACC, and MACC concentrations were always lower than those of ACC. The ACC reached its maximal concentration before MACC and C₂H₄, especially in the case of high leaf:fruit ratio. The activity of ACCs and ACCo was simulated for [ATP⁰] = 0.015 mol m⁻³, [ACC] = 0.015 mol m⁻³, and ambient [O₂] and [CO₂] for the treatment with six and 30 leaves per fruit in 1993. The activity of the two enzymes increased from 100 to 140 d after bloom (DAB) and this all the more strongly as the leaf:fruit ratio was high (Fig. 10). The C₂H₄ production started much later and this more especially as the leaf:fruit ratio was low.

Figure 9.

Seasonal variation of ACC (continuous lines), MACC (dotted line), and C₂H₄ (dashed lines) concentrations simulated by ETHY over the treatments and years studied.

Figure 10.

Seasonal variation of ACCo (continuous thin lines) and ACCs (continuous thick lines) activities, and C₂H₄ (dashed lines) emission simulated by ETHY over the treatments in 1993.

Key Internal Variables for C₂H₄ Production

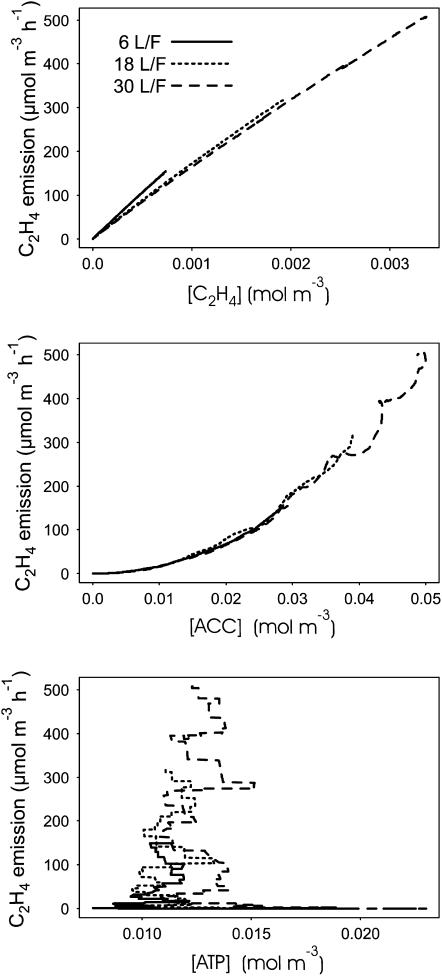
According to ETHY, C₂H₄ emission depends on three main variables, the concentration of ATP on which ACCs depends, the concentration of ACC on which C₂H₄ synthesis depends, and the concentration of C₂H₄ itself on which the intensity of the diffusion in the external atmosphere depends. The link between C₂H₄ emission and these three variables was analyzed using the simulations of the leaf:fruit ratio done in 1993. As expected, there was, whatever the leaf:fruit ratio, a unique very strong positive linear link between internal C₂H₄ concentration and C₂H₄ emission (Fig. 11). A strong positive exponential link was also found with ACC concentration. There was no obvious connection between C₂H₄ emission and the ATP concentration, which shows that there is no link between C₂H₄ emission at a given time and ATP concentration at this time. This could be due to the low ATP cost of C₂H₄ production. The amount of ATP needed for C₂H₄ production was computed considering that two ATP are needed to produce one ACC molecule (Fig. 1). The computed ATP cost of C₂H₄ production was always below 0.04% of ATP production by respiration. Nevertheless, ATP concentration is a key variable for C₂H₄ production. Indeed, keeping ATP concentration as a constant (0.013 mol m⁻³), whatever the leaf:fruit ratio, and re-estimating the parameters (k_s = 0.5 ± 0.08 h⁻¹ and k_o = 0.36 ± 0.06 h⁻¹) led to a poor adjustment to the experimental data (RMSE = 131 μmol h⁻¹ m⁻³). With a constant concentration of ATP, the model was able to simulate correct C₂H₄ production for low leaf:fruit ratio but predicted too low the C₂H₄ production for high leaf:fruit ratio (Fig. 6).

Figure 11.

Relationships between C₂H₄ emission and C₂H₄, ACC, and ATP concentrations simulated by ETHY over the three leaf:fruit ratios studied in 1993.

Key Parameters of Fruit Metabolism

A sensitivity analysis to the model parameters was performed to look for the key parameters, i.e. the parameters whose variation has a strong effect on the model outputs. The analysis was performed using the inputs of 1993. To see if the model was sensitive to different degrees to small or large parameter variations, each parameter was varied from X = ±1%, 5%, 10%, 20%, 40%, 60%, 80% and 100% around the value reported in “Materials and Methods.” The effect of this variation on the average value of (1) O₂, CO₂, ATP, ACC, MACC, and C₂H₄ concentrations and (2) fruit respiration and C₂H₄ emission was assessed. The model was considered sensitive to a parameter when an X percentage of variation of this parameter resulted in at least an X/2 percentage of variation of one or more of the above-mentioned variables. As the model was similarly sensitive to parameters whatever the level of X (data not shown), only the results obtained for X = ±20% are presented (Table I). The sensitivity increased from six to 30 leaves per fruit. However, as the general trends were very similar, only the results obtained for 18 leaf:fruit ratio in 1993 were presented (Table I). The oxygen internal concentration was only slightly sensitive to parameter β relating fruit surface area to fruit mass. The higher the fruit surface was, the higher the internal O₂ concentration was. The carbon dioxide internal concentration was sensitive to β but also to α (Eq. 9), to the resistance to CO₂ diffusion (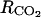), and to the maintenance respiration coefficient (q_m). The lower the fruit surface and the higher 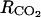and q_m were, the higher the carbon dioxide internal concentration was. The respiration was only sensitive to q_m. The ATP concentration was only slightly sensitive to the maintenance respiration coefficient and to λ, which is the rate constant of ATP consumption. The ACC, MACC, and C₂H₄ concentrations and C₂H₄ emission were sensitive to the same set of parameters, which are growth and maintenance respiration coefficients; λ; parameters k_s and k_o, which are involved in the activities of ACCs and ACCo; the skin permeability to C₂H₄ 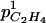; and parameters α and β. They were sensitive to a lesser degree to 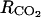and 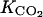involved in CO₂ diffusion and in ACCo inhibition by CO₂. The ACC, MACC, and C₂H₄ concentrations and C₂H₄ emission were only slightly sensitive to the rate constant k₄, which determines MACC synthesis. This means that the possible variation of ACC malonyltransferase activity would have a small impact on C₂H₄ synthesis, except if this variation is large enough to compensate for the low sensitivity.

Table I.

Effect of a ± 20% variation of the ETHY parameters on the average value of O₂, CO₂, ATP, ACC, MACC, and C₂H₄ concentrations, and fruit respiration and C₂H₄ emission

Values are expressed as a percentage of the reference condition. The analysis was performed using the inputs of treatment 18 leaves per fruit in 1993.

Parameters		[O2]	[CO2]	[ATP]	[MACC]	[ACC]	[C2H4]	Respiration	C2H4 Emission
Respiration parameters
qg	+20	−1	5	5	21	17	26	5	26
	−20	1	−5	−5	−20	−16	−24	−5	−24
qm	+20	−4	15	15	50	45	81	15	82
	−20	4	−15	−15	−40	−40	−57	−15	−58
Q10	+20	−1	4	4	8	10	17	4	17
	−20	1	−4	−4	−9	−10	−17	−5	−17
RQ	+20	4	0	0	1	1	1	0	1
	−20	−7	0	0	−1	−1	−2	0	−2
ATP parameter
λ	+20	0	0	−17	−54	−52	−74	0	−74
	−20	0	0	25	118	89	186	0	191
MACC, ACC, and C2H4 metabolic parameters
k4	+20	0	0	0	9	−7	−12	0	−12
	−20	0	0	0	−12	7	13	0	13
ks	+20	0	0	0	92	71	143	0	146
	−20	0	0	0	−61	−61	−82	0	−82
ko	+20	0	0	0	44	23	76	0	79
	−20	0	0	0	−52	−47	−78	0	−78
KO2	+20	0	0	0	−4	−3	−6	0	−6
	−20	0	0	0	4	3	6	0	7
KCO2	+20	0	0	0	10	6	17	0	17
	−20	0	0	0	−15	−11	−24	0	−24
Permeability and resistances to gas
RO2	+20	−5	0	0	−1	−1	−2	0	−2
	−20	5	0	0	1	1	2	0	2
RCO2	+20	0	20	0	−12	−8	−19	0	−19
	−20	0	−20	0	12	8	20	0	21
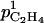	+20	0	0	0	−50	−47	−76	0	−71
	−20	0	0	0	81	54	188	0	134
Allometric parameters
α	+20	4	−16	0	−42	−38	−66	0	−60
	−20	−7	25	0	65	47	150	0	102
β	+20	11	−41	0	−81	−85	−99	0	−98
	−20	−19	70	0	153	108	582	0	291

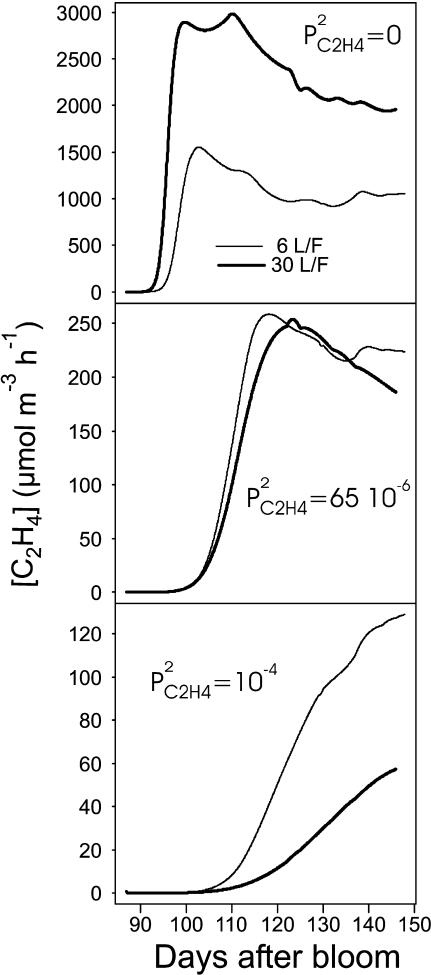
It is interesting to note that an increase in skin permeability to C₂H₄ induced a decrease in ACC, MACC, and C₂H₄ concentrations as well as in C₂H₄ emission. Equation 10 allows an increase of skin permeability to C₂H₄ with fruit mass if 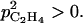For small values of 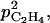the C₂H₄ emission started earlier and reached a higher level for high than for low leaf:fruit ratio as observed in our experiments. But for high values of 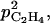the opposite situation was predicted by the model. In the latter case, the increase of C₂H₄ synthesis with the leaf:fruit ratio was overcompensated by the increase of C₂H₄ diffusion to the external atmosphere (Fig. 12). For medium values of 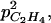no effect of leaf:fruit ratios was predicted.

Figure 12.

Effect of 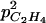increase on seasonal variation of C₂H₄ emission simulated by ETHY at 23°C over six (thin lines) and 30 (thick lines) leaves per fruit.

Effect of O₂ and CO₂ Air Concentration and Temperature on C₂H₄ Production

Sensitivity to environmental variables was analyzed using, as previously, the results obtained with an 18 leaf:fruit ratio in 1993. The environmental variables had a variation of ±X% throughout the season, and the effect of this variation on the average value of C₂H₄ emission was assessed. The values of X considered in the analysis were the same as those used when examining the sensitivity to parameters. The model was similarly sensitive whatever the level of X. Considering a ±20% variation, the effect was very small for CO₂ (±0.2%), and no effect of the natural variation of CO₂ concentration in the air seemed possible. Although larger, the effect of O₂ remained negligible (−11% to +7%). On the contrary, sensitivity to temperature was high (−71% to +170%). Indeed, a temperature increase as low as +5% had a large effect on C₂H₄ production (+31%).

DISCUSSION

The theoretical framework developed here enables us to provide a description of the relationship between environment, fruit growth, and C₂H₄ emission. It pieces together elements of present knowledge on autocatalytic C₂H₄ in a biophysical and metabolic theory focusing on processes of regulation by C₂H₄ itself, ATP, CO₂, O₂, and temperature. The simulation model based on this theory was able to predict the effect of fruit growth intensity on C₂H₄ emission for several years in peach.

ETHY predicted significant variations of ATP concentration from 0.007 to 0.023 mol m⁻³ with a general decrease following that of fruit respiration per fruit mass. Such a variation of more than 100% of ATP concentration is commonly observed during postharvest fruit storage (Saquet et al., 2003a, 2003b). The internal O₂ and CO₂ concentrations were sensitive to parameters relating fruit surface area to fruit mass and much less to parameters implicated in fruit respiration and permeability to gas. This means that changes in fruit shape (e.g. between cultivars) might have important consequences. The predicted internal O₂ and CO₂ concentrations were fairly stable during the season, which is in conflict with the results on tomato and cantaloupe of Lyons et al. (1962), Saltveit (1993), and Shellie and Saltveit (1993), who found a regular increase of CO₂ and decrease of O₂ concentrations before and during ripening. This discrepancy was not ascribable to our steady-state assumption as indicated by additional simulations done in no steady-state conditions (data not shown). On the other hand, it could be explained by the higher maintenance respiration coefficient of these fruits. Indeed, when the model was run using the maintenance respiration coefficient of tomato in place of that of peach, a seasonal increase of CO₂ and decrease of O₂ concentrations were obtained (data not shown). As predicted by the model, Tonutti et al. (1991, 1997) and Agusti et al. (1999) observed a sharp increase of ACC and C₂H₄ in peach during maturation and an increase of ACCs and ACCo. Similar trends are observed in species such as apple (Suzuki et al., 1999), which means that our model could be extended to species other than peach. The model also predicts that the ACC concentration reaches a plateau before the final increase in C₂H₄ emission, as usually reported (Tonutti et al., 1997; Agusti et al., 1999). When the fruit growth is promoted, Johnson (1995) and Agusti et al. (1999) found that ACCs, ACCo, and C₂H₄ and ACC biosynthesis occur earlier, which is in accordance with our experimental results and model. As predicted by the model, they also found that the increase of the enzyme activities began earlier than the C₂H₄ burst. As shown by Amoros et al. (1989) on peach and Lara and Vendrell (2000) on apple, our model predicts an increase of MACC during ripening. However, a decrease of MACC has also been observed by Tonutti et al. (1991) on peach fruit. This plasticity in MACC seasonal variation reflects the effect of regulations of the rate constant k₄ in Equation 5 that are not considered in the model. As the model is not very sensitive to k₄, only strong variations of this rate constant could explain these different patterns of MACC kinetics. Future research could focus more on the regulation of ACC N-malonyltransferase, which generates MACC.

The different steps leading to C₂H₄ production have been considered, with a focus on ACCs and ACCo. The strong sensitivity to parameters related to these enzymes shows the importance of metabolism in the control of C₂H₄ production. In agreement with our results, studies on gene expression showed the importance of these enzymes in controlling the rate of endogenous C₂H₄ production (Lelièvre et al., 1997; Alexander and Grierson, 2002).

Our main assumption is that C₂H₄ production is highly dependent on ATP availability and thus on fruit respiration. This assumption is in agreement with results of Saquet et al. (2003b) on stored apples. They observed simultaneous variations in ATP and CO₂ concentrations and C₂H₄ production when the composition of the controlled atmosphere changed. This aspect is not often considered in the works on autocatalytic C₂H₄. Our simulations showed that the control of C₂H₄ production by ATP availability makes it possible to account for the differences observed between treatments leading to contrasted growths. The activities of ACCs and ACCo were different between treatments, but this was primarily due to differences in ATP availability. Surprisingly, the model was not very sensitive to the respiration parameters, whereas ATP production was directly dependent on respiration. Indeed, there is no simple connection between respiration or ATP concentration and C₂H₄ concentration because the ATP concentrations decrease during the season when the C₂H₄ concentration increases in response to the autocatalytic process.

Another important assumption of the model is that the diffusion process through the skin has to be considered as shown by the high sensitivity of the model to the permeability to C₂H₄ and to the skin area. The importance of such a process has been put forward by postharvest scientists studying the effect of film permeability of packaging on gas concentrations (Fishman et al., 1995). This is all the more important as permeability and skin area are highly variable between species and within the same species (Cameron and Reid, 1982). Considering that skin permeability could vary during fruit development, the model predicted either no effect of fruit size (or leaf number per fruit) on C₂H₄ or a decrease of this C₂H₄ with increasing fruit size. This lack of effect has been observed on apple by Johnston et al. (2002). A negative effect has also been observed on apple (Poll et al., 1996) and on cantaloupe by Valantin (1998).

It was also assumed that C₂H₄ production was regulated by factors such as O₂ and CO₂ concentrations in the tissues and temperature. Effects of O₂, CO₂, and temperature have been well established, especially in postharvest studies. Our model is only slightly sensitive to air O₂ concentrations and almost insensitive to CO₂ concentrations. Only strong variations, such as those encountered during postharvest fruit storage in packages, can be effective. On the contrary, the temperature has a strong effect on C₂H₄ emission, and small variations can have a significant effect.

One interesting result of our study is that it was possible to simulate the onset of C₂H₄ production without considering any initial event such as the increase of endogenous concentrations of jasmonates (Fan et al., 1998), auxins (Miller et al., 1987; Agusti et al., 1999), or abscisic acid as mentioned above. Moreover, adequate C₂H₄ increase for different years and treatments was predicted without considering any increase in respiration during the ripening period. The respiration was only calculated as a function of temperature, fruit mass, and growth rate. These results are in agreement with those of Saltveit (1993) and Shellie and Saltveit (1993), who suggest that the respiratory climacteric may not be required for the ripening of climacteric fruit. Indeed, the increase of energy demand for the climacteric rise of C₂H₄ is not so strong that a simultaneous increase of respiration is needed. According to our predictions, the ATP requirement for C₂H₄ production in peach fruit would represent less than 0.04% of the available ATP.

An interesting perspective of this work is based on the high ETHY sensitivity to parameters involved in calculating ACCo and ACCs activities, ATP production, and skin surface and permeability. This would enable the use of ETHY to analyze comprehensively the high genetic variability in C₂H₄ production (Miccolis and Saltveit, 1991; Klozenbucher et al., 1994; Xu et al., 1998) following the approach initiated by Quilot et al. (2004) for sugar concentrations.

MATERIALS AND METHODS

Plant Material and Experimental Design

The ETHY model was parameterized, calibrated, and validated for the late-maturing peach (Prunus persica L. Batsch) cv Suncrest/GF 677. The measurements were performed on peach trees planted in 1981 in the orchard of the Institut National de la Recherche Agronomique (INRA) Avignon Centre. Trees were goblet trained and received routine horticultural care suitable for commercial orchards. This care included winter and summer pruning, weekly irrigation from June to harvest, hand thinning in April, and pest control. Fungicides and insecticides were applied every 2 weeks from February to July. Weeds were destroyed by weed-killer.

The first experiments in 1993 and 1996 varied assimilate supply to the fruits. Treatments were applied to fruit-bearing shoots located on the southern part of each tree and isolated from the tree by girdling. Three treatments set leaf:fruit ratios at six, 18, and 30 leaves per fruit to obtain minimum, mean, and maximum growth curves that were representative of the Suncrest cultivar. Shoots with six or 18 leaves per fruit were thinned to four fruits, and shoots with 30 leaves per fruit were thinned to two or three fruits. In 1993 and 1996, 240 fruit-bearing shoots were prepared on 64 and 36 trees, respectively, to provide 80 sets of three neighboring shoots with leaf:fruit ratios of six, 18, and 30.

Fruits were harvested from five replicates per treatment each week from mid-June to the beginning of fruit maturation. A replicate was made up of fruits from two shoots when fruits were small and from one shoot later in the season. The last harvests were on August 16, 1993, and August 9, 1996.

Experiment 2, performed in 1997, varied both assimilate and water supply to the fruits. The treatments were applied to 240 fruit-bearing shoots isolated from 40 trees by girdling and located on the southern part of each tree. Half of the trees were irrigated in June and July, whereas irrigation was withheld from the remaining trees. For each irrigated treatment, leaf:fruit ratio of the selected fruit-bearing shoots was 10 or 30. Sixty pairs of neighboring shoots with leaf:fruit ratios of 10 and 30 were prepared for each irrigation treatment. Fruits from five replicates per treatment were harvested each week from mid-June to fruit maturation (July 24).

Gas Measurements

At each harvest, O₂ consumption and CO₂ and C₂H₄ production were measured individually at a constant room temperature (23°C) by confining intact fruit in a gas-tight 400-mL jar. The internal atmosphere of the jar was analyzed by gas chromatography. CO₂ and O₂ were separated on a Porapak Q column at 110°C, followed by a 13× molecular sieve column at room temperature (Chambroy et al., 1984), and quantified using a catharometric detector. The C₂H₄ released by the fruits was determined by a flame ionization detector gas chromatograph with an activated alumina column at 120°C.

Inputs of the Model

After C₂H₄ measurement, the stone was removed and the fresh mass of fruit flesh was measured. The dry mass was measured by drying the stone and the flesh at 70°C for 72 h. The hourly dry and fresh fruit masses, which were inputs of the model, were interpolated from our measurements by local regression (Chambers and Hastie, 1992). For each treatment, a local regression was applied to the whole set of data, to the upper range of data (i.e. the set of maximal masses per measurement date), and to the lower range of data (i.e. the set of minimal masses per measurement date) to get mean, maximal, and minimal growth curves, respectively. Hourly temperatures of the air were collected in INRA weather stations close to the experimental fields.

Modeling Technique

Simulation of both CO₂ and C₂H₄ emission were based on an hourly scale. Accordingly, the results were displayed hourly on the figures, using DAB as units (e.g. for hour 12 of DAB 110, the x value was 110.5). An hour was also used as the time frame in the numerical integration. The computer program was written using Splus simulation language (Becker et al., 1988). The differential equations were solved numerically by the first-order Runge-Kutta method. The “nonlinear least squares regression” Splus function was used for model calibration to estimate parameters that could not be determined in independent experiments. Parameters were estimated by minimizing the sum of squared differences between the response and the prediction using the Gauss-Newton algorithm.

For testing model quality, a goodness-of-fit criterion was calculated separately for C₂H₄ and CO₂ emission in each of the 10 year×treatment combinations. The basic criterion was a RMSE, a very common criterion describing the mean distance between simulation and measurement (Kobayashi and Us Salam, 2000), here computed as:

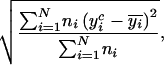where N is the number of measurement dates over the growing period and n_i the number of measures at date i. 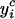is the C₂H₄ (or the CO₂) emission at date i calculated by the model, and 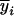the average of the n_i measures.

The smaller the RMSE in comparison to measurements, the better the goodness-of-fit.

The predictive quality of the model, which is not the same as adjustment quality (Wallach et al., 2001), is very important to assess to be able to use the model outside the range of its development. For C₂H₄ and CO₂ emission, we computed the classical RMSEP using the 10 year × treatment combinations, by cross-validation as did Batchelor et al. (1994) and Wallach et al. (2001). The principles of cross-validation are as follows (Wallach et al., 2001). A situation being one year × treatment combination, the data are split into two parts: one part includes a single situation (the target situation), and the other part includes all the data independent of the target situation, i.e. in our case, not from the same year. Parameters are adjusted using the second part of the data. The RMSE of the resulting model is calculated on the target situation, as presented before. The procedure is repeated using every situation in turn as the target situation, and averaging the RMSE over all the target situations gives the estimate of prediction error.

ETHY Variables

The ETHY variables are as follows: M_fresh (g), fruit fresh mass; M_dry (g), fruit dry mass; V (m⁻³), fruit volume; A (m²), skin area; T (°C), temperature; 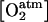(mol m⁻³), atmospheric O₂ concentration; 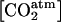(mol m⁻³), atmospheric CO₂ concentration; 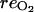(mol h⁻¹), respired oxygen; 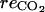(mol h⁻¹), CO₂ produced through respiration; [O₂] (mol m⁻³), internal O₂ concentration; [CO₂] (mol m⁻³), internal CO₂ concentration; [ATP] (mol m⁻³), ATP concentration; [MACC] (mol m⁻³), MACC concentration; [ACC] (mol m⁻³), ACC concentration; [C₂H₄] (mol m⁻³), C₂H₄ concentration; and 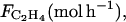C₂H₄ diffusion to the external atmosphere.

ETHY Parameters

The ETHY parameters are as follows: q_g = 0.0025 (mol g⁻¹), growth respiration coefficient; q_m = 12 10⁻⁵ (mol g⁻¹ h⁻¹), maintenance respiration coefficient at 20°C; Q₁₀ = 2, temperature ratio of maintenance respiration; RQ = 0.834, respiratory quotient; λ = 500 (h⁻¹), ATP parameter; k₄ = 0.001 (h⁻¹), MACC parameter; k_s = 0.778 (h⁻¹), ACCs parameter; k_o = 0.268 (h⁻¹), ACCo parameter; Michaelis constant involved in effect of O₂ on ACCo; 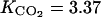(mol m⁻³), Michaelis constant involved in effect of CO₂ on ACCo; 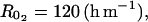resistances to diffusion of O₂; 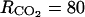(h m⁻¹), resistances to diffusion of CO₂; 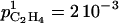(m h⁻¹), skin permeability; and α = 6.049 10⁻⁴ and β = 0.601, empirical parameter relating fruit area (m²) to fruit mass (g).