Resistance To Change Of Forgetting Functions And Response Rates

Abstract

This experiment examined the effects of reinforcement probability on resistance to change of remembering and response rate. Pigeons responded on a two-component multiple schedule in which completion of a variable-interval 20-s schedule produced delayed matching-to-sample trials in both components. Each session included four delays (0.1 s, 2 s, 4 s, and 8 s) between sample termination and presentation of comparison stimuli in both components. The two components differed in the probability of reinforcement arranged for correct matches (i.e., rich, p  =  .9; lean, p  =  .1). Response rates during the variable-interval portion of the procedure were higher in the rich component during baseline and more resistant to the disruptive effects of intercomponent food and extinction. Forgetting functions were constructed by examining matching accuracy as a function of delay duration. Baseline accuracy was higher in the rich component than in the lean component as measured by differences in the y-intercept of the forgetting functions (i.e., initial discrimination), rather than from differences in the slope of the forgetting function (i.e., rate of forgetting). Intercomponent food increased the rate of forgetting relatively more in the lean component than in the rich component, but initial discrimination was not systematically affected. Extinction reduced initial discrimination relatively more in the lean component than in the rich component, but did not systematically affect rate of forgetting. These results are consistent with our previous data suggesting that, as for response rate, accuracy and resistance to change of discriminating are positively related to rate of reinforcement. These data also suggest that the disruptability of remembering depends on the conditions of reinforcement, but the way in which remembering is disrupted depends on the nature of the disruptor.

Substantial evidence indicates that the persistence of response rate is related to conditions of reinforcement prior to the introduction of a disruptor (see Nevin, 1992; Nevin & Grace, 2000; for review). For example, Nevin (1974, Experiments 1 to 3) maintained the key pecking of pigeons on a multiple schedule of variable-interval (VI) food delivery. The two components of the multiple schedule differed in the rate at which food was available or the amount of food delivered. Nevin introduced response-independent food during the intercomponent interval (ICI) or extinction (no food for key pecking) across conditions. He found that response rates were more resistant to change, relative to baseline performance, in the component that delivered food at a higher rate or in larger amounts. Subsequent research has determined more specifically that the overall rate of reinforcement in a context (response-dependent plus any response-independent reinforcers; see Nevin, Tota, Torquato, & Shull, 1990) is most predictive of resistance to change of response rates.

Despite a few systematic exceptions (see Nevin & Grace, 2000), this basic finding has broad generality. For example, greater resistance to change with higher rates of reinforcement has been shown with pigeons, rats, college students, older adults, and people with mental retardation (e.g., Harper, 1996; Mace et al., 1990; Plaud, Gaither, & Lawrence, 1997; Plaud, Plaud, & von Duvillard, 1999; Shull, Gaynor, & Grimes, 2002). Furthermore, this finding has been demonstrated with a variety of disruptors, including prefeeding, response-independent food in the ICI, and extinction with nonhumans (e.g., Grace & Nevin, 2000; Grimes & Shull, 2001; Shahan, Magee, & Dobberstein, 2003), as well as extinction and various alternative sources of reinforcement with people (e.g., Ahearn, Clark, Gardenier, Chung, & Dube, 2003; Dube, McIlvane, Mazzitelli, & McNamara, 2003; Mace et al., 1990; Mace, Mauro, Boyajian, & Eckert, 1997; Plaud et al., 1999).

Recently, Nevin, Milo, Odum, and Shahan (2003) found that accuracy of conditional discrimination performance, like response rate, was more resistant to change when it was more frequently reinforced. In that study, pigeons responded under a multiple schedule in which pecks to a center key produced 0-s delayed matching-to-sample (DMTS) trials on a VI schedule (i.e., a multiple VI-DMTS schedule). In this procedure, based on one developed by Schaal, Odum, and Shahan (2000), one component had a high probability that a correct match would produce food (the rich component), and the other component had a low probability that a correct match would produce food (the lean component). Response rates during the VI and matching accuracy during DMTS trials were higher in the component that had a higher probability of reinforcement. When ICI food, prefeeding, extinction, and a delay between the sample and comparisons were introduced, DMTS accuracy was more resistance to change in the rich component in each case. Response rates during the VI also were more resistant to change in the rich component except during the delay to the comparisons, which had relatively small and inconsistent effects on response rates. In other words, the accuracy of conditional discrimination was largely governed by the same factors that determined resistance to change of response rates.

In the present experiment, we sought to extend the analysis of resistance to change to forgetting functions (accuracy as a function of delay to comparisons). White and colleagues (e.g., White, 1985, 1991, 2001; White, Ruske, & Colombo, 1996) have suggested that forgetting functions have two separable aspects: initial discrimination (i.e., accuracy at 0-s delay) and slope (i.e., rate of forgetting). They have shown that some manipulations, characterized as factors related to attending to the sample stimulus, affect initial discrimination without affecting the slope of the function. Other manipulations, characterized as factors related to remembering the sample, affect the slope of the function without affecting initial discrimination.

In what is termed the signaled magnitude effect, forgetting functions maintained by higher amounts of reinforcement are above and parallel to forgetting functions maintained by lower amounts within sessions (Jones, White, & Alsop, 1995; McCarthy & Voss, 1995; Nevin & Grosch, 1990). White and Wixted (1999) obtained similar results for forgetting functions maintained by higher and lower probabilities of reinforcement for matches across conditions. In other words, increasing overall reinforcement for correct matches increases initial discrimination, but does not alter the rate of forgetting.

There has been little investigation, however, of how baseline reinforcement conditions affect the degree to which forgetting functions are altered under conditions of disruption. Nevin and Grosch (1990) explored with pigeon subjects the effects of various disruptors on matching accuracy for trials in which different stimuli signaled whether a relatively large or small reinforcer would follow a correct match. They found that a houselight interpolated during the delay between samples and comparisons, administration of pentobarbital, and shorter sample durations produced disruptions in forgetting functions that did not differ across the two kinds of trials. This effect occurred despite higher baseline accuracies in the larger-magnitude trials. In the present experiment, we determined how disruptors commonly used in the study of resistance to change of response rates (ICI food, prefeeding, and extinction) affected resistance to change of response rates and forgetting functions maintained by different probabilities of reinforcement in the multiple VI-DMTS procedure with pigeons.

METHOD

Subjects

Four White Carneau pigeons served as subjects in this experiment. All pigeons had previous histories with related operant procedures. Prior to the present experiment, the pigeons performed for several months on a similar procedure in which they discriminated vertical and horizontal lines. Reliable forgetting functions could not be obtained using this procedure because accuracy was at near-chance levels with even short retention intervals. The pigeons were maintained at 80% ± 15 g of free-feeding weights by postsession feeding of pelleted pigeon chow as necessary. Between sessions, pigeons were individually housed in a temperature-controlled colony room with free access to water under a 12:12 hr light/dark cycle.

Apparatus

Four Lehigh Valley Electronics pigeon chambers constructed of painted metal with aluminum front panels were used. The chambers were 35 cm long, 35 cm high, and 30 cm wide. Each front panel had three translucent plastic keys in a row that could be lit from behind with green, red, blue, or yellow light and required a force of about 0.10 N to record a response. Keys were 2.5 cm in diameter and 24 cm from the floor. A lamp (28 V, 1.1 W) mounted 4.5 cm above the center key served as a houselight. A rectangular opening 10 cm above the chamber floor provided access through a 5-cm by 5.5-cm aperture to a solenoid-operated hopper filled with pelleted pigeon chow. During hopper presentations, the opening was lit with white light and the houselight and keylights were extinguished. White noise and chamber ventilation fans masked extraneous noise. Contingencies were programmed and data collected by a microcomputer located in an adjacent room using Med Associates® interfacing and software.

Procedure

Baseline

The procedure was similar to that used by Nevin et al. (2003). Due to the pigeons' previous experience, no pretraining was required. Two components of a multiple schedule, signaled by the color of the center key (either red or green), alternated. Pecks to the lit center key changed it to yellow or blue (the sample) on a VI 20-s schedule. The VI schedule was composed of 20 intervals generated based on the method described by Fleshler and Hoffman (1962). Separate lists of intervals were maintained for the two multiple-schedule components. If no peck occurred within 80 s (the longest interval duration plus 20 s), the schedule progressed to sample presentation without a key peck. The sample remained on until the first peck after 3 s or until 6 s had elapsed, whichever occurred first. After the sample, the center key returned to the color present during the VI for a 0.1, 2, 4, or 8-s delay. Each delay duration was presented 12 times per session during each component, with each sample color (blue or yellow) appearing an equal number of times at each delay.

Following the delay, the center key was extinguished, and the side keys were lit, one yellow and one blue (the comparisons). The key that was lit with each color varied randomly across trials. A single peck turned off the side keys and was followed by food or blackout. Components differed in the probability that choice of the comparison color that matched the sample color would produce food. In one component (the rich component, signaled by the red key for Pigeons P51 and P97), correct matches had a probability of .9 of producing 2-s access to food. Nonreinforced matches and incorrect choices produced a 2-s blackout. In the other component (the lean component, signaled by the green key for Pigeons P51 and P97), correct matches had a probability of .1 of producing 2-s access to food. As in the rich component, nonreinforced matches and incorrect choices produced a 2-s blackout. The color assignments were reversed for Pigeons P74 and P85. The component that began the session was chosen randomly. Components alternated after blocks of four trials that contained one presentation of each delay duration. The order of the delays was chosen randomly within each block. Components were separated by a 15-s ICI during which the houselight was on and the keys were dark. Pigeons experienced 30 sessions under the baseline conditions prior to the introduction of the first disruptor. Experimental sessions were conducted daily at approximately the same time, and ended after 96 trials (48 per component). Data from the last 10 sessions of this and subsequent baselines were used in analyses.

Resistance Tests

To examine resistance to change of matching accuracy and rates of key pecking during the VI portion of the schedule, a variety of disruptors were introduced. Each disruptor was in effect for 10 consecutive sessions. Twenty baseline sessions were conducted between disruptors, which were presented in the order described below. The number of sessions of the initial baseline and in between disruptors was chosen based on our prior experience that behavior under the VI-DMTS procedure typically stabilizes in this time frame for pigeons with prior extensive exposure. For each baseline exposure, the mean response rates during the VI in the first 5 and second 5 of the last 10 sessions did not differ by more than 5% from the mean of all these 10 sessions, thus indicating that response rates were relatively stable. Performance in the DMTS portion of the procedure also appeared stable.

Ici Food

During the ICI, food was presented on average every 5 s on a random time (RT) schedule. The houselight was extinguished and the hopper was raised for 2 s during food presentations.

Prefeeding

During prefeeding, pigeons were fed either 20 g or 40 g of pigeon chow in the home cage 30 min prior to 10 consecutive sessions. Which amount was fed first was counterbalanced across pigeons, with 20 baseline sessions between prefeeding amounts. Twenty grams had little effect on behavior, whereas 40 g tended to suppress behavior almost completely. Thus results are not shown for prefeeding.

Extinction

During extinction, correct matches were never followed by food but instead were always followed by blackout. During extinction, if no peck was made to a comparison stimulus within 20 s, the comparisons were extinguished and a 2-s blackout ensued (i.e., there was a 20-s limited hold). If a limited hold expired, that trial was not counted as correct or incorrect. Sessions ended after 96 trials or 90 min, whichever occurred first. Pigeon P74 finished at least 90 trials during the first four sessions, but only five in the fifth session, and even fewer on subsequent sessions. Data from the DMTS and VI portion of the procedure for this pigeon were used only for the first five sessions of extinction. Pigeon P51 finished all trials for the first seven sessions, then 95, 80, and 40 on the 8th, 9th, and 10th sessions, respectively. Pigeon P85 finished all trials during all extinction sessions. Finally, Pigeon P97 finished all trials for the first eight sessions and then 95 and 94 trials on the 9th and 10th sessions, respectively.

Measures

To assess performance during the VI portion of the schedule, response rates on the center key were calculated separately for the rich and lean components. Time and pecks during DMTS trials were excluded from VI response rates. To assess performance during the DMTS portion of the schedule, choices of the blue or yellow comparison given a blue or yellow sample were recorded separately for each delay duration for each component. These values were used to calculate log d, a measure of conditional discrimination performance (see Davison & Tustin, 1978; Nevin, 1981). This measure is calculated as:

in which By is the number of choices of the yellow comparison and Bb is the number of choices of the blue comparison, each totaled separately for trials on which a yellow sample (Sy) or blue sample (Sb) was presented.

Log d is the geometric mean of the ratio of correct to incorrect choices, and has several desirable properties. Unlike percentage correct, log d is independent of bias for one comparison stimulus over another and has a range of 0 (no discrimination) to infinity (all choices correct on an infinitely large number of trials). It is often the case, however, that one or more of the terms of the equation equals zero (e.g., if there are no errors of a particular type), and it is therefore common to add a small number (in the present case, 0.25) to each term to allow calculation of log d (Hautus, 1995; see Alsop, 2004, for discussion). With this correction, for 12 trials (i.e., six yellow and six blue) per delay per session, pooled over 10 sessions, the maximum value of log d in the present experiment is 2.38 at each delay.

Forgetting functions (log d as a function of delay to the comparison for each component) were then characterized by the following exponential decay model (e.g., White, 2001) using nonlinear regression:

in which a is the estimated performance level when there is no delay to the comparisons (initial discrimination, log d₀), b is the slope of the function (the rate of forgetting over the delays), and t is the duration of the retention interval (the delay). As a result of the fitting procedure, estimated values of a may be greater than the empirical maximum of log d described above.

RESULTS

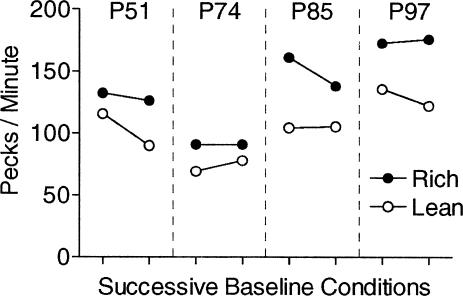
Figures 1 and 2 focus on response rates during the VI portion of the schedule. Figure 1 shows average baseline response rates during the VI for the rich and lean components for each pigeon. The data are averages over the last 10 sessions of the baseline conditions that preceded disruption by ICI food and extinction, respectively. Response rates during the VI were higher in each baseline during the rich component (in which correct matches had a .9 probability of producing food) than in the lean component (in which correct matches had a .1 probability of producing food). Across these successive baseline conditions, response rates within components were not systematically different across pigeons. A two-way (component × condition) repeated measures analysis of variance (ANOVA) found a significant effect of component, F(1, 3)  =  23.19, p  =  .017, but not of condition, F(1, 3)  =  2.56, p  =  .21, with no interaction, F(1, 3)  =  .005, p  =  .95.

Fig. 1. Response rates in the VI portion of the procedure for the rich and lean components.

Data are means of the last 10 sessions of successive exposure to baseline conditions.

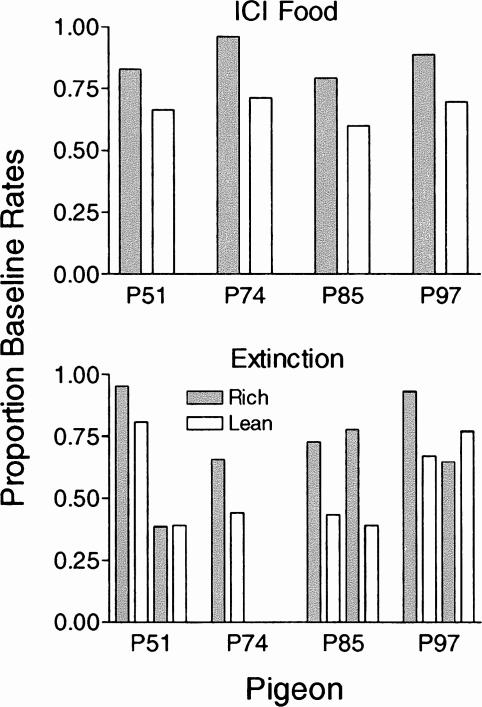
Fig. 2. Response rates in the rich and lean components for the 10 sessions of exposure to intercomponent food (top panel) and extinction (bottom panel) presented as a proportion of the immediately preceding baseline.

Data are shown separately for the first five and last five sessions of extinction.

Figure 2 expresses average response rates during the VI for each component during the ICI food (top panel) and extinction (bottom panel) conditions as a proportion of the average preceding baseline rate (shown in Figure 1) for each pigeon. Adding free food during the ICI decreased response rates in both the rich and lean components relative to baseline. In each case, proportion baseline response rates were lower during the lean component than during the rich component. A paired t test determined that this difference was statistically significant, t(3)  =  11.79, p  =  .0013. Extinction also reduced response rates during the VI relative to baseline for each pigeon. For the first five sessions of extinction, the decrease was greater in the lean component than during the rich component, t(3)  =  7.1, p  =  .0058, but during the second five sessions of extinction, there was no significant difference across components, t(2)  =  0.56, p  =  .632. Data are shown for Pigeon P74 for only the first five sessions because this pigeon did not respond after the fifth session.

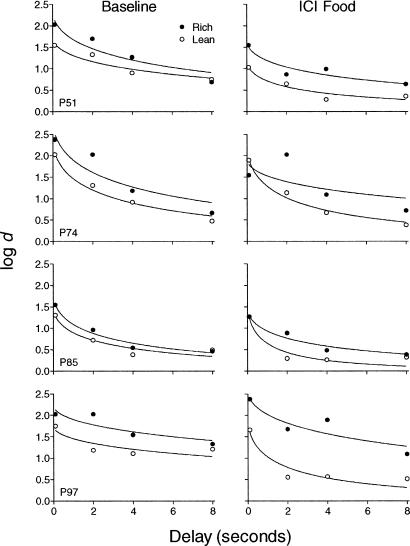
Figures 3 through 6 focus on performance during the DMTS portion of the procedure. Figure 3 shows forgetting functions during the first baseline condition (left column) and during exposure to ICI food (right column) for each pigeon. Data were pooled over the last 10 sessions for baseline and for the 10 sessions of exposure to ICI food. The curves in the figure show the fit of Equation 2 to the data using nonlinear regression performed with GraphPad Prism®. During baseline, log d usually decreased as the delay duration increased for each component. The function for the rich component was above and roughly parallel to that of the lean component for each pigeon. During baseline, initial discrimination was higher for the rich component, but rate of forgetting was not systematically different for the two components. During ICI food, the forgetting function for the rich component remained above that of the lean component, but the function for the lean component became steeper than during baseline for each pigeon. Table 1 shows the parameters a (initial discrimination), b (rate of forgetting), and the variance accounted for (VAC) by Equation 2 for each pigeon for ICI food and the baseline that preceded it. For baseline conditions, the VAC for Equation 2 was within a generally accepted range, with a median of 0.87 for the rich component and 0.91 for the lean component. The VAC for Equation 2 during ICI food was similar to that during the preceding baseline for most pigeons, with a median of 0.85 during the rich component and 0.91 during the lean component.

Fig. 3. Forgetting function for the rich and lean components during the final 10 sessions of baseline (left column) and during sessions with intercomponent food deliveries (right column).

Accuracy (i.e., log d) is plotted as a function of the delay between sample offset and presentation of the comparison stimuli. Forgetting functions (i.e., Equation 2) were fit to the data from both components.

Table 1. Parameter values for a (initial discrimination) and b (rate of forgetting), and variance accounted for (VAC) for Equation 2 (see text) for each pigeon for the intercomponent interval (ICI) food and extinction conditions, preceded by the prior baseline conditions.

Pigeon	Condition
	Baseline		ICI food		Baseline		Extinction
	Rich	Lean	Rich	Lean	Rich	Lean	Rich	Lean
a
P51	2.37	1.74	1.67	1.22	1.64	1.66	0.88	0.63
P74	2.86	2.42	1.93	2.32	2.75	2.69	2.24	1.74
P85	1.83	1.52	1.50	1.68	1.48	0.85	0.88	0.26
P97	2.24	1.75	2.59	1.97	2.55	2.31	1.44	1.00
b
P51	0.34	0.28	0.34	0.53	0.23	0.25	0.20	0.22
P74	0.41	0.50	0.23	0.58	0.28	0.44	0.23	0.58
P85	0.52	0.53	0.48	0.96	0.40	0.40	0.38	0.12
P97	0.16	0.19	0.25	0.65	0.22	0.38	0.34	0.23
VAC
P51	0.88	0.91	0.88	0.91	0.78	0.61	0.74	0.95
P74	0.86	0.98	0.41	0.98	0.75	0.98	0.49	0.84
P85	0.97	0.91	0.95	0.91	0.96	0.94	0.59	0.44
P97	0.77	0.71	0.81	0.89	0.92	0.93	0.97	0.63

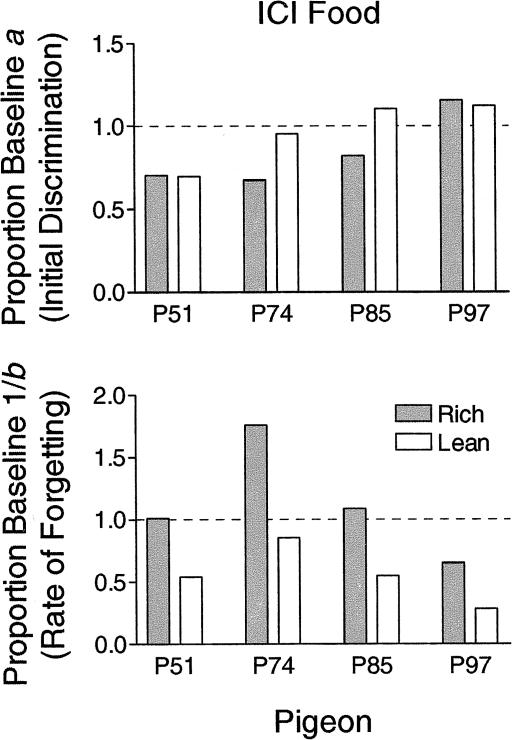
Figure 4 shows the parameters of the forgetting functions during ICI food relative to baseline. The top panel of Figure 4 shows that proportion baseline a (initial discrimination) was not systematically affected by ICI food across pigeons. A paired t test showed that the values for the rich and lean component were not significantly different from each other, t(3)  =  1.51, p  =  .27. The conclusions were similar when the analysis was based on log d for the 0.1 s delay, rather than for the fitted parameter a. The bottom panel of Figure 4, however, shows that b (rate of forgetting) was systematically affected by the delivery of ICI food. Because a larger value of b indicates more rapid forgetting, the inverse of the value of b was used to compute proportion baseline b for this figure so that larger values indicate less disruption as with response rates. Proportion baseline 1/b was lower for the lean component than the rich component in each case. A paired t test showed that the values for the rich and lean component were significantly different from each other, t(3)  =  4.83, p  =  .017. Thus, relative to baseline, rate of forgetting increased in the lean component but not in the rich component.

Fig. 4. Parameters of the forgetting functions for the rich and lean components during sessions with intercomponent food presented as a proportion of the parameter values obtained from fits to the immediately preceding baseline data.

Rate of forgetting (bottom panel) is presented as a proportion of baseline 1/b.

Figure 5 shows forgetting functions for extinction (right column) and the baseline that preceded it (left column) for each pigeon. Data were pooled over the last 10 sessions of baseline and for the 10 sessions of exposure to extinction. The exception is Pigeon P74, for which data during extinction are from the first five sessions only. The curves in the figure show the fit of Equation 2 to the data. During baseline, log d usually decreased as the delay duration increased for each component. Initial discrimination typically was higher for the rich component. The function for the rich component was above that of the lean component for 3 of 4 pigeons. The slope of the functions (rate of forgetting) was similar for the two components for 2 pigeons (P51 and P85), but steeper in the lean component for the other 2 pigeons (P74 and P97). During extinction, the forgetting function for the rich component remained above that of the lean component. Initial discrimination was reduced by extinction, but rate of forgetting was not systematically affected across pigeons. The functions were similar for the first five and last five sessions of extinction in overall form, but the degree of reduction in initial discrimination was greater for both components in the last five sessions (data not shown). Table 1 shows the values of the parameters a and b as well as VAC for the functions fit to the entire extinction exposure (except for Pigeon P74 as noted previously). Overall, the VAC for Equation 2 was similar to the baseline that preceded ICI food, with a median of 0.85 for the rich component and 0.94 for the lean component. The VAC for Equation 2 was lower during extinction than the preceding baseline, with a median of 0.67 for the rich component and 0.74 for the lean component.

Fig. 5. Forgetting function for the rich and lean components during the final 10 sessions of baseline (left column) and during extinction (right column) sessions.

Accuracy (i.e., log d) is plotted as a function of the delay between sample offset and presentation of the comparison stimuli. Forgetting functions (i.e., Equation 2) were fit to the data from both components.

Figure 6 shows the parameters of the forgetting functions during extinction relative to baseline. The top panel of Figure 6 shows that proportion baseline a (initial discrimination) was lower in the lean component than in the rich component in each case. A paired t test showed that the values for the rich and lean component were significantly different from each other, t(3)  =  5.41, p  =  .012. Thus, relative to baseline, initial discrimination decreased more in the lean component than in the rich component. The conclusions were similar when the analysis was based on log d for the 0.1 s delay, rather than for the fitted parameter a. The bottom panel of Figure 6, however, shows that b (rate of forgetting) was not systematically affected by extinction. The high value for Pigeon P85 in the lean component most likely reflects a floor effect in that component during extinction. Accuracy was poor at each delay duration, making the rate of forgetting across delays necessarily low (see Figure 5). A paired t test showed that the values for the rich and lean component were not significantly different from each other, t(3)  =  1.15, p  =  .33.

Fig. 6. Parameters of the forgetting functions for the rich and lean components during extinction sessions presented as a proportion of the parameter values obtained from fits to the immediately preceding baseline data.

Rate of forgetting (bottom panel) is presented as a proportion of baseline 1/b.

DISCUSSION

Baseline response rates during the VI portion of the multiple schedule were higher in the rich component, in which correct matches had a .9 probability of producing food, than in the lean component, in which correct matches had a .1 probability of producing food. This finding is similar to that of Nevin et al. (2003), who arranged a VI-DMTS procedure with 0-s delays to comparisons. The forgetting functions for the DMTS portion of the procedure in the rich component were above and parallel to the forgetting functions in the lean component. In other words, probability of reinforcement for a correct match affected initial discrimination but not rate of forgetting. This result is consistent with the signaled magnitude effect, in which forgetting functions for trials on which a larger reinforcer is signaled for correct matches are above and parallel to forgetting functions maintained by a smaller reinforcer within sessions (Jones et al., 1995; McCarthy & Voss, 1995; Nevin & Grosch, 1990).

Disruption by ICI food and extinction reduced response rates during the VI portion of the procedure more in the lean component than in the rich component. This finding replicates the results of Nevin et al. (2003), which used the VI-DMTS procedure with 0-s delays to comparisons. Furthermore, results from these studies, in which the consequence for responding during the VI was the opportunity to complete a DMTS trial with a high or low probability of reinforcer delivery for correct matches, extend the generality of findings from research with simple VI schedules of reinforcement that differ in terms of the rate or amount of food delivered for key pecking (e.g., Nevin, 1974; see Nevin & Grace, 2000, for review).

The forgetting functions were affected differently by the two disruptors. Intercomponent food increased the rate of forgetting in the lean component but did not systematically affect initial discrimination. This result is similar to that obtained by Spetch (1985). Although she did not examine forgetting functions with different probabilities of food delivery, Spetch found that ICI food increased the rate of forgetting in DMTS trials. The present experiment appears to be the first to demonstrate that the increase in the rate of forgetting depends on the baseline reinforcement conditions for matching to sample.

Extinction, however, reduced initial discrimination more in the lean component than in the rich component, but did not increase rate of forgetting. There seems to be little precedent for this result. To our knowledge, previous research has not examined the effects of extinction on forgetting functions in general and those maintained by different probabilities of reinforcement in particular. Nevin et al. (2003) previously found that extinction reduced matching accuracy relatively more in the lean component than in the rich component in the VI-DMTS procedure with 0-s delays to comparisons. The few studies that have implemented extinction for simultaneous matching (in which the sample remains on during comparison presentation) with pigeons have found no effect of extinction on matching accuracy (e.g., Cumming, Berryman, Cohen, & Lanson, 1967; Nevin, 1967). Simultaneous matching, obviously, does not involve forgetting because the sample is present when the choice is made. That aspect of the procedure may be related to the difference between results with simultaneous matching and those with DMTS.

The differing effects of ICI food and extinction on the aspects of the forgetting functions provide support for White's (e.g., 1985, 1991, 2001) assertion that initial discrimination and rate of forgetting are separable aspects of delayed conditional discriminations. He and his colleagues have demonstrated that some manipulations, characterized as factors related to attending to the sample stimulus, affect initial discrimination without affecting the slope of the function. For example, White and Wixted (1999) required either five pecks or one peck to the sample stimulus in a DMTS procedure with pigeons. When five pecks were required, the forgetting function was above and parallel to that of the forgetting function generated when only one peck was required to the sample. In other words, requiring additional responses to the sample increased initial discrimination but had no effect on the rate of forgetting. In the present experiment, initial discrimination was higher under baseline conditions than under extinction, but there were no systematic differences in rate of forgetting between baseline and extinction conditions. Therefore, one could conclude that extinction decreases accuracy on DMTS trials by reducing attending to samples, not by affecting remembering of samples.

Other manipulations, characterized as factors related to remembering the sample (i.e., interference), affect the slope of the function without affecting initial discrimination (e.g., White, 1985, 1991, 2001). For example, Harper and White (1997) determined forgetting functions in pigeons when the houselight was normally off during the delay to the comparisons and when it was on. Although initial discrimination was similar under these conditions, rate of forgetting was higher when the houselight was on during the delay. In the present experiment, interpolation of food during the ICI increased the rate of forgetting in the lean component relative to baseline conditions, but had no systematic effect on initial discrimination in the two components. According to this interpretation, ICI food disrupts accuracy by interfering with remembering the samples (see also Spetch, 1985), not by affecting attending to the samples.

In summary, ICI food and extinction reduced both rates of key pecking during the VI that produced DMTS trials and accuracy on those trials to a lesser extent in the rich component than in the lean component. These results support the earlier suggestion (Nevin et al., 2003) that discriminating between stimuli may be strengthened by reinforcement in much the same way as reinforcement strengthens free-operant responding. The analysis of aspects of forgetting functions, in addition to overall accuracy, however, suggests a more subtle relation. The separate aspects of forgetting functions, initial discrimination and rate of forgetting, can be differentially affected by disruption. Thus, when considering resistance to change of forgetting functions, both the nature of the disruptor and the baseline conditions of reinforcement must be taken into account.